
(Financial: credit card number validation) Credit card numbers follow certain patterns. A credit card number must have between 13 and 16 digits. It must start with
- for Visa cards
- for Master cards
- for American Express cards
- for Discover cards
In 1954, Hans Luhn of IBM proposed an
- 1. Double every second digit from right to left. If doubling of a digit results in a two-digit number, add up the two digits to get a single-digit number.
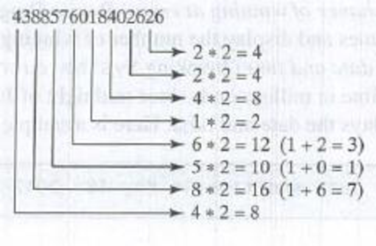
- 2. Now add all single-digit numbers from Step 1.
4 + 4 + 8 + 2 + 3 + 1 + 7 + 8 = 37
- 3. Add all digits in the odd places from right to left in the card number.
6 + 6 + 0 + 8 + 0 + 7 + 8 + 3 = 38
- 4. Sum the results from Step 2 and Step 3.
37+38=75
- 5. If the result from Step 4 is divisible by 10, the card number is valid; otherwise, it is invalid. For example, the number 4388576018402626 is invalid, but the number 4388576018410707 is valid.
Write a
/** Return true if the card number is valid */
public static boolean isValid(long number)
/** Get the result from Step 2 */
public static int sumOfDoubleEvenPlace(long number)
/** Return this number if it is a single digit, otherwise, • return the sum of the two digits */
public static int getDigit(int number)
/** Return sum of odd-place digits in number */
public static int sumOfOddPlace(long number)
/** Return true if the number dis a prefix for number *I
public static boolean prefixMatched(long number, int d)
/** Return the number of digits in d */
public static int getSize(long d)
/ ** Return the first k number of digits from number. If the • number of digits in number is less than k, return number. */
public static long getPrefix(long number , int k)
Here are sample runs of the program: (You may also implement this program by reading the input as a string and processing the string to validate the credit card.)

Trending nowThis is a popular solution!

Chapter 6 Solutions
Introduction to Java Programming and Data Structures, Comprehensive Version (11th Edition)
Additional Engineering Textbook Solutions
Starting Out with C++ from Control Structures to Objects (9th Edition)
Starting Out with Java: From Control Structures through Data Structures (4th Edition) (What's New in Computer Science)
Electric Circuits. (11th Edition)
INTERNATIONAL EDITION---Engineering Mechanics: Statics, 14th edition (SI unit)
Starting Out with Java: From Control Structures through Objects (7th Edition) (What's New in Computer Science)
- Describe three (3) Multiplexing techniques common for fiber optic linksarrow_forwardCould you help me to know features of the following concepts: - commercial CA - memory integrity - WMI filterarrow_forwardBriefly describe the issues involved in using ATM technology in Local Area Networksarrow_forward
- For this question you will perform two levels of quicksort on an array containing these numbers: 59 41 61 73 43 57 50 13 96 88 42 77 27 95 32 89 In the first blank, enter the array contents after the top level partition. In the second blank, enter the array contents after one more partition of the left-hand subarray resulting from the first partition. In the third blank, enter the array contents after one more partition of the right-hand subarray resulting from the first partition. Print the numbers with a single space between them. Use the algorithm we covered in class, in which the first element of the subarray is the partition value. Question 1 options: Blank # 1 Blank # 2 Blank # 3arrow_forward1. Transform the E-R diagram into a set of relations. Country_of Agent ID Agent H Holds Is_Reponsible_for Consignment Number $ Value May Contain Consignment Transports Container Destination Ф R Goes Off Container Number Size Vessel Voyage Registry Vessel ID Voyage_ID Tonnagearrow_forwardI want to solve 13.2 using matlab please helparrow_forward
- a) Show a possible trace of the OSPF algorithm for computing the routing table in Router 2 forthis network.b) Show the messages used by RIP to compute routing tables.arrow_forwardusing r language to answer question 4 Question 4: Obtain a 95% standard normal bootstrap confidence interval, a 95% basic bootstrap confidence interval, and a percentile confidence interval for the ρb12 in Question 3.arrow_forwardusing r language to answer question 4. Question 4: Obtain a 95% standard normal bootstrap confidence interval, a 95% basic bootstrap confidence interval, and a percentile confidence interval for the ρb12 in Question 3.arrow_forward
 C++ Programming: From Problem Analysis to Program...Computer ScienceISBN:9781337102087Author:D. S. MalikPublisher:Cengage Learning
C++ Programming: From Problem Analysis to Program...Computer ScienceISBN:9781337102087Author:D. S. MalikPublisher:Cengage Learning C++ for Engineers and ScientistsComputer ScienceISBN:9781133187844Author:Bronson, Gary J.Publisher:Course Technology Ptr
C++ for Engineers and ScientistsComputer ScienceISBN:9781133187844Author:Bronson, Gary J.Publisher:Course Technology Ptr Microsoft Visual C#Computer ScienceISBN:9781337102100Author:Joyce, Farrell.Publisher:Cengage Learning,
Microsoft Visual C#Computer ScienceISBN:9781337102100Author:Joyce, Farrell.Publisher:Cengage Learning, Systems ArchitectureComputer ScienceISBN:9781305080195Author:Stephen D. BurdPublisher:Cengage Learning
Systems ArchitectureComputer ScienceISBN:9781305080195Author:Stephen D. BurdPublisher:Cengage Learning EBK JAVA PROGRAMMINGComputer ScienceISBN:9781337671385Author:FARRELLPublisher:CENGAGE LEARNING - CONSIGNMENTProgramming Logic & Design ComprehensiveComputer ScienceISBN:9781337669405Author:FARRELLPublisher:Cengage
EBK JAVA PROGRAMMINGComputer ScienceISBN:9781337671385Author:FARRELLPublisher:CENGAGE LEARNING - CONSIGNMENTProgramming Logic & Design ComprehensiveComputer ScienceISBN:9781337669405Author:FARRELLPublisher:Cengage





