Chapter 6, Problem 1RCC

(a) Draw two typical curves y = f(x) and y = g(x), where f(x) ≥ g(x) for a ≤ x ≤ b. Show how to approximate the area between these curves by a Riemann sum and sketch the corresponding approximating rectangles. Then write an expression for the exact area.

(b) Explain how the situation changes if the curves have equations x = f(y) and x = g(y), where f(y) ≥ g(y) for c ≤ y ≤ d.

To determine

To Draw: the two typical curves $y=f\left(x\right)$ and $y=g\left(x\right)$.

To define: A Riemann sum that approximates the area between the two typical curves with drawing of the corresponding approximating rectangles and exact area between the two typical curves and the expression for the exact area.

Explanation of Solution

Consider the two curves $y=f\left(x\right)$ and $y=g\left(x\right)$.

Here, the top curve function is $f\left(x\right)$ and the bottom curve function is $g\left(x\right)$.

Assume f and g are continuous function and $f\left(x\right)\ge g\left(x\right)$ for $a\le x\le b.$

Here, the lower limit is a and the upper limit is b.

Show the approximate i^th strip rectangle with base $\Delta x$ and height $f\left(x_i^{*}\right)-g\left(x_i^{*}\right)$ in the region between a and b.

Sketch the two typical curves $y=f\left(x\right)$ and $y=g\left(x\right)$ as shown in Figure 1.

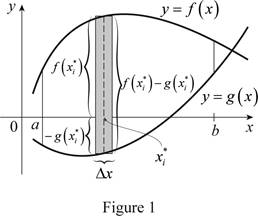
Refer to figure 1.

The two typical curves $y=f\left(x\right)$ and $y=g\left(x\right)$ showing the approximate i^th strip rectangle is drawn.

The expression for the exact area is $A=\underset{n\to \infty }{\lim }{\displaystyle \sum _{i=1}^n\left[f\left(x_i^{*}\right)-g\left(x_i^{*}\right)\right]}\Delta x$.

Divide the area between the two typical curves into n strips of equal width and take the entire sample points to be right endpoints, in which $x_i^{*}$ as $x_i.$ Hence the Riemann sum is

${\displaystyle \sum _{i=1}^n\left[f\left(x_i^{*}\right)-g\left(x_i^{*}\right)\right]}\Delta x$

Sketch thecorresponding approximating rectangles as shown in Figure 2.

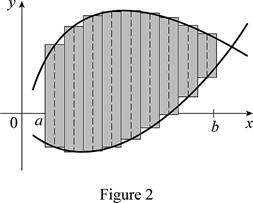
The better and better approximation occurs in $n\to \infty$. Hencethe exact areaA, between the two typical curves is the sum of the areas of the corresponding approximating rectangles as shown below.

$A=\underset{n\to \infty }{\lim }{\displaystyle \sum _{i=1}^n\left[f\left(x_i^{*}\right)-g\left(x_i^{*}\right)\right]}\Delta x$

Thus, the Riemann sum with the sketch of corresponding approximating rectangles and the exact area between the two typical curves shown.

Therefore, the approximation of the area between the two typical curves using Riemann sum with the sketch of the corresponding approximating rectangles and the sum of the areas corresponding approximating rectangles is the exact area.

To determine

To Draw: The two typical curves with the changing the situation as $x=f\left(y\right)$ and $x=g\left(y\right)$.

To define: The situation if the curves changes from $y=f\left(x\right)$ and $y=g\left(x\right)$ to $x=f\left(y\right)$ and $x=g\left(y\right)$ the expression for the exact area.

The expression for the exact area is $A={\displaystyle {\int }_c^d\left[f\left(y\right)-g\left(y\right)\right]dy}$.

Explanation of Solution

Consider the two curves $x=f\left(y\right)$ and $x=g\left(y\right)$.

Here, the right curve function is $f\left(y\right)$ and the left curve function is $g\left(y\right)$.

Assume f and g are continuous function and $f\left(y\right)\ge g\left(y\right)$ for $c\le y\le d$.

Here, the bottom limit is c and the top limit is d.

Sketch the two typical curves $x=f\left(y\right)$ and $x=g\left(y\right)$ is shown in Figure 3.

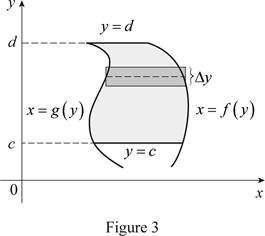
Thus, the two typical curves $y=f\left(x\right)$ and $y=g\left(x\right)$ are drawn.

Normally the height calculated from the top function minus bottom one and integrating from left to right. Instead of normal calculation, use “right minus left” and integrating from bottom to top. Therefore the exact area, A written as

$A={\displaystyle {\int }_c^d\left[f\left(y\right)-g\left(y\right)\right]dy}$

Therefore, the changes of the situation if the curves have equations $x=f\left(y\right)$ and $x=g\left(y\right)$ is explained.