Chapter 58, Problem 23AR

To determine

The measure of angle $\angle 1$.

The measure of angle $\angle 2$.

The measure of angle $\angle 3$.

The measure of angle $\angle 4$.

The measure of angle $\angle 5$.

The measure of angle $\angle 6$.

The measure of angle $\angle 7$.

The measure of angle $\angle 8$.

The measure of angle $\angle 9$.

The measure of angle $\angle 10$.

Answer to Problem 23AR

The measure of angle $\angle 1$ is $25°$

The measure of angle $\angle 2$ is $67°$.

The measure of angle $\angle 3$ is $48°$.

The measure of angle $\angle 4$ is $29°$.

The measure of angle $\angle 5$ is $48°$.

The measure of angle $\angle 6$ is $86°$.

The measure of angle $\angle 7$ is $94°$.

The measure of angle $\angle 8$ is $126°$.

The measure of angle $\angle 9$ is $54°$.

The measure of angle $\angle 10$ is $88°$.

Explanation of Solution

Given information:

The angle $\angle CAD$ is $38°$, angle $\angle BEC$ is $40°$, arc $\stackrel{⌢}{AC}$ is $130°$ and arc $\stackrel{⌢}{CE}$ is $=134°$.

The given figure is as shown below.

  

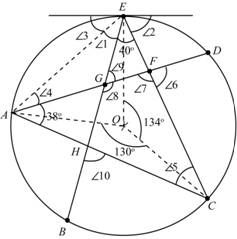
        Figure -(1)

Write the expression for angle $\angle CAE$.

 $\angle CAE=\frac{\angle COE}{2}$ ...... (I)

Write the expression for the angle $\angle 4$.

 $\angle 4+38°=\angle CAE$ ...... (II)

Write the expression for angle $\angle AOE$.

 $\begin{array}{c}\angle AOE=360°-\left(130°+134°\right)\\ =360°-264°\\ =96°\end{array}$

Write the expression for angle $\angle ACE$.

 $\angle ACE=\frac{\angle AOE}{2}$ ...... (III)

Write the expression for the angle sum property of a triangle $XYZ$.

 $\angle X+\angle Y+\angle Z=180°$ ...... (IV)

Here, the first angle of the triangle is $\angle X$, the second angle of the triangle is $\angle Y$ and the third angle of the triangle is $\angle Z$

Write the expression for angle $\angle EFG$.

 $\angle EFG=180°-\angle 7$ ...... (V)

Write the expression for angle $\angle AGH$.

 $\angle AGH=\angle 9$

Write the expression for angle $\angle AHG$.

 $\angle AHG=\angle 10$

Write the expression for angle $\angle OEC$.

 $\begin{array}{c}\angle OEC=\frac{180°-134°}{2}\\ =23°\end{array}$

Write the expression for angle $\angle 2$.

 $\angle OEC+\angle 2=90°$ ...... (VI)

Write the expression for the angle sum property of a line.

 $\theta +\phi +\alpha +\beta +\mathrm{....}=180°$ ...... (VII)

Here, the respective angles on the lines are $\theta$, $\phi$, $\alpha$, $\beta$.

Calculation:

Substitute $134°$ for $\angle COE$ in Equation (I)

$\begin{array}{c}\angle CAE=\frac{134°}{2}\\ =67°\end{array}$

Substitute $67°$ for $\angle CAE$ in Equation (II).

 $\begin{array}{l}\angle 4+38°=67°\\ \angle 4=67°-38°\\ \angle 4=29°\end{array}$

Substitute $96°$ for $\angle AOE$ and $\angle 5$ for $\angle ACE$ in Equation (III).

 $\begin{array}{c}\angle 5=\frac{96°}{2}\\ =48°\end{array}$

Substitute $\left(\angle 4+38°\right)$ for $\angle X$, $\angle 5$ for $\angle Y$, $\left(40°+\angle 1\right)$ for $\angle Z$, $29°$ for $\angle 4$ and $48°$ for $\angle 5$, for triangle ACE in Equation (IV).

$\begin{array}{l}\left(\angle 4+38°\right)+\angle 5+\left(40°+\angle 1\right)=180°\\ 29°+38°+48°+40°+\angle 1=180°3\\ \angle 1=25°\end{array}$

Substitute $38°$ for $\angle X$, $\angle 5$ for $\angle Y$, $\angle 7$ for $\angle Z$ and $48°$ for $\angle 5$, for triangle ACF in Equation (IV).

$\begin{array}{l}38°+\angle 5+\angle 7=180°\\ \angle 7=180°-38°-48°\\ \angle 7=94°\end{array}$

Substitute $\angle 7$ for $\angle X$, $\angle 6$ for $\angle Y$, $0°$ for $\angle Z$ and $94°$ for $\angle 7$, for line AFD in Equation (IV).

$\begin{array}{l}\angle 7+\angle 6+0°=180°\\ 94°+\angle 6=180°\\ \angle 6=180°-94°\\ \angle 6=86°\end{array}$

Substitute $94°$ for $\angle 7$ in Equation (V).

$\begin{array}{c}\angle EFG=180°-94°\\ =86°\end{array}$

Substitute $40°$ for $\angle X$, $\angle 9$ for $\angle Y$, $\angle EFG$ for $\angle Z$ and $86°$ for $\angle EFG$, for triangle EFG in Equation (IV).

$\begin{array}{l}\angle EFG+\angle 9+40°=180°\\ \angle 9=180°-40°-86°\\ \angle 9=54°\end{array}$

Substitute $\angle 8$ for $\angle X$, $\angle 9$ for $\angle Y$, $0°$ for $\angle Z$ and $54°$ for $\angle 8$, for line BGE in Equation (IV).

$\begin{array}{l}\angle 8+\angle 9+0°=180°\\ \angle 8=180°-54°\\ \angle 8=126°\end{array}$

Substitute $40°$ for $\angle X$, $\angle 9$ for $\angle Y$, $\angle EFG$ for $\angle Z$ and $86°$ for $\angle EFG$, for triangle EFG in Equation (IV).

$\begin{array}{l}\angle EFG+\angle 9+40°=180°\\ \angle 9=180°-40°-86°\\ \angle 9=54°\end{array}$

Substitute $38°$ for $\angle X$, $\angle 9$ for $\angle Y$, $\angle 10$ for $\angle Z$ and $54°$ for $\angle 9$, for triangle AGH in Equation (IV).

$\begin{array}{l}38°+\angle 9+\angle 10=180°\\ \angle 10=180°-38°-54°\\ \angle 10=88°\end{array}$

Substitute $23°$ for $\angle OEC$ in Equation (VI).

$\begin{array}{l}23°+\angle 2=90°\\ \angle 2=90°-23°\\ \angle 2=67°\end{array}$

Substitute $\angle 1$ for $\theta$, $\angle 2$ for $\phi$, $\angle 3$ for $\alpha$, $40°$ for $\beta$, $25°$ for $\angle 1$ and $67°$ for $\angle 2$ in Equation (VII).

$\begin{array}{l}\angle 1+\angle 2+\angle 3+40°=180°\\ \angle 3=180°-40°-25°-67°\\ \angle 3=48°\end{array}$

Conclusion:

The measure of angle $\angle 1$ is $25°$

The measure of angle $\angle 2$ is $67°$.

The measure of angle $\angle 3$ is $48°$.

The measure of angle $\angle 4$ is $29°$.

The measure of angle $\angle 5$ is $48°$.

The measure of angle $\angle 6$ is $86°$.

The measure of angle $\angle 7$ is $94°$.

The measure of angle $\angle 8$ is $126°$.

The measure of angle $\angle 9$ is $54°$.

The measure of angle $\angle 10$ is $88°$.