
Concept explainers
Given the following
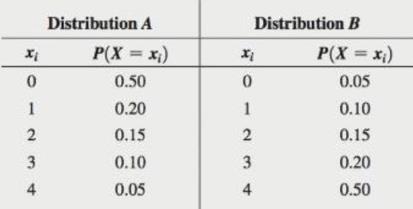
a. Compute the expected value for each distribution.
b. Compute the standard deviation for each distribution.
c. Compare the results of distribution A and B.
a.
Find the expected value for distribution A and distribution B.
Answer to Problem 1PS
The expected value of distribution A is 1 and distribution B is 3.
Explanation of Solution
Calculation:
The table of probability distributions for distribution A and B is given.
The formula for calculating expected value is,
Here,
The expected value of distribution A is calculated by substituting the values in the above formula. It is calculated as,
The expected value of distribution B is,
Thus, the expected value of distribution A and distribution B are 1 and 3, respectively.
b.
Find the standard deviation of distribution A and B.
Answer to Problem 1PS
The standard deviation for both distributions A and B is 1.22474.
Explanation of Solution
Calculation:
The standard deviation is calculated using the formula,
Here,
For distribution A,
For distribution B,
Thus, the standard deviation of distribution A and B is same, that is, 1.2247.
c.
Compare the results of the two distributions.
Answer to Problem 1PS
The mean value for distribution B is higher than distribution A but the standard deviation is same for both the distributions.
Explanation of Solution
From part a, the expected value for distribution A and B are obtained as 1 and 3, respectively. From part b, the standard deviation for both the distributions is obtained as 1.2247.
Thus, it can be said that the mean value or the expected value of distribution B is greater than that of distribution A. However, both of them have the same spread as the value of standard deviation is same.
Want to see more full solutions like this?
Chapter 5 Solutions
Basic Business Statistics, Student Value Edition (13th Edition)
- Given your fitted regression line, what would be the residual for snake #5 (10 C)?arrow_forwardCalculate the 95% confidence interval around your estimate of r using Fisher’s z-transformation. In your final answer, make sure to back-transform to the original units.arrow_forwardCalculate Pearson’s correlation coefficient (r) between temperature and heart rate.arrow_forward
- A researcher wishes to estimate, with 90% confidence, the population proportion of adults who support labeling legislation for genetically modified organisms (GMOs). Her estimate must be accurate within 4% of the true proportion. (a) No preliminary estimate is available. Find the minimum sample size needed. (b) Find the minimum sample size needed, using a prior study that found that 65% of the respondents said they support labeling legislation for GMOs. (c) Compare the results from parts (a) and (b). ... (a) What is the minimum sample size needed assuming that no prior information is available? n = (Round up to the nearest whole number as needed.)arrow_forwardThe table available below shows the costs per mile (in cents) for a sample of automobiles. At a = 0.05, can you conclude that at least one mean cost per mile is different from the others? Click on the icon to view the data table. Let Hss, HMS, HLS, Hsuv and Hмy represent the mean costs per mile for small sedans, medium sedans, large sedans, SUV 4WDs, and minivans respectively. What are the hypotheses for this test? OA. Ho: Not all the means are equal. Ha Hss HMS HLS HSUV HMV B. Ho Hss HMS HLS HSUV = μMV Ha: Hss *HMS *HLS*HSUV * HMV C. Ho Hss HMS HLS HSUV =μMV = = H: Not all the means are equal. D. Ho Hss HMS HLS HSUV HMV Ha Hss HMS HLS =HSUV = HMVarrow_forwardQuestion: A company launches two different marketing campaigns to promote the same product in two different regions. After one month, the company collects the sales data (in units sold) from both regions to compare the effectiveness of the campaigns. The company wants to determine whether there is a significant difference in the mean sales between the two regions. Perform a two sample T-test You can provide your answer by inserting a text box and the answer must include: Null hypothesis, Alternative hypothesis, Show answer (output table/summary table), and Conclusion based on the P value. (2 points = 0.5 x 4 Answers) Each of these is worth 0.5 points. However, showing the calculation is must. If calculation is missing, the whole answer won't get any credit.arrow_forward
 Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
