Chapter 4.5, Problem 58E

Use Table 4, Appendix 3, to find the following probabilities for a standard normal random variable Z:

a P(0 ≤ Z ≤ 1.2)

b P(−.9 ≤ Z ≤ 0)

c P(.3 ≤ Z ≤ 1.56)

d P(−.2 ≤ Z ≤ .2)

e P(−1.56 ≤ Z ≤ −.2)

f Applet Exercise Use the applet Normal Probabilities to obtain P(0 ≤ Z ≤ 1.2). Why are the values given on the two horizontal axes identical?

To determine

Find the value of $P\left(0\le Z\le 1.2\right)$.

Answer to Problem 58E

The value of $P\left(0\le Z\le 1.2\right)$ is 0.3849.

Explanation of Solution

Calculation:

It is given that Z is normally distributed with mean 0 and standard deviation 1.

The following can be observed for Z:

$P\left(Z<0\right)+P\left(0\le Z\le 1.2\right)+P\left(Z>1.2\right)=1$

Since, the standard normal distribution is symmetric about 0, $P\left(Z>0\right)=P\left(Z<0\right)$.

$P\left(Z>0\right)+P\left(0\le Z\le 1.2\right)+P\left(Z>1.2\right)=1$

Use Table 4: Normal Curve Areas Standard normal probability in right-hand tail to obtain the probability as follows:

• Locate 0.0 along the z column.
• Locate 0 along the Second decimal place of z.
• The intersection of row and column gives the probability value of 0.5000.

Use Table 4: Normal Curve Areas Standard normal probability in right-hand tail to obtain the probability as follows:

• Locate 1.2 along the z column
• Locate 0 along the Second decimal place of z
• The intersection of row and column gives the probability value of 0.1151.

$\begin{array}{c}P\left(Z>0\right)+P\left(0\le Z\le 1.2\right)+P\left(Z>1.2\right)=1\\ P\left(0\le Z\le 1.2\right)=1-P\left(Z>0.0\right)-P\left(Z>1.2\right)\\ =1-A\left(0.0\right)-A\left(1.2\right)\\ =1-0.5000-0.1151\\ =0.3849\end{array}$

Thus, the value of $P\left(0\le Z\le 1.2\right)$ is 0.3849.

To determine

Find the value of $P\left(-0.9\le Z\le 0\right)$.

Answer to Problem 58E

The value of $P\left(-0.9\le Z\le 0\right)$ is 0.3159.

Explanation of Solution

Calculation:

The following can be observed for Z:

$P\left(Z<-0.9\right)+P\left(-0.9\le Z\le 0\right)+P\left(Z>0\right)=1$

Since, the standard normal distribution is symmetric about 0, $P\left(Z<-0.9\right)=P\left(Z>0.9\right)$.

$P\left(Z>0.9\right)+P\left(-0.9\le Z\le 0\right)+P\left(Z>0\right)=1$

Use Table 4: Normal Curve Areas Standard normal probability in right-hand tail to obtain the probability as follows:

• Locate 0.0 along the z column.
• Locate 0 along the Second decimal place of z.
• The intersection of row and column gives the probability value of 0.5000.

Use Table 4: Normal Curve Areas Standard normal probability in right-hand tail to obtain the probability as follows:

• Locate 0.9 along the z column.
• Locate 0 along the Second decimal place of z.
• The intersection of row and column gives the probability value of 0.1841.

$\begin{array}{c}P\left(Z>0.9\right)+P\left(-0.9\le Z\le 0\right)+P\left(Z>0\right)=1\\ P\left(-0.9\le Z\le 0\right)=1-P\left(Z>0.0\right)-P\left(Z>1.2\right)\\ =1-A\left(0.0\right)-A\left(1.2\right)\\ =1-0.5000-0.1841\\ =0.3159\end{array}$

Thus, the value of $P\left(-0.9\le Z\le 0\right)$ is 0.3159.

To determine

Find the value of $P\left(0.3\le Z\le 1.56\right)$.

Answer to Problem 58E

The value of $P\left(0.3\le Z\le 1.56\right)$ is 0.3227.

Explanation of Solution

Calculation:

The following can be observed for Z:

$\begin{array}{l}P\left(Z<0.3\right)+P\left(0.3\le Z\le 1.56\right)+P\left(Z>1.56\right)=1\\ 1-P\left(Z>0.3\right)+P\left(0.3\le Z\le 1.56\right)+P\left(Z>1.56\right)=1\end{array}$

Use Table 4: Normal Curve Areas Standard normal probability in right-hand tail to obtain the probability as follows:

• Locate 0.3 along the z column
• Locate 0 along the Second decimal place of z
• The intersection of row and column gives the probability value of 0.3821.

Use Table 4: Normal Curve Areas Standard normal probability in right-hand tail to obtain the probability as follows:

• Locate 1.5 along the z column
• Locate 6 along the Second decimal place of z
• The intersection of row and column gives the probability value of 0.0594.

$\begin{array}{c}1-P\left(Z>0.3\right)+P\left(0.3\le Z\le 1.56\right)+P\left(Z>1.56\right)=1\\ P\left(0.3\le Z\le 1.56\right)=1-1+P\left(Z>0.3\right)-P\left(Z>1.56\right)\\ =P\left(Z>0.3\right)-P\left(Z>1.56\right)\end{array}$

                                                                                              $\begin{array}{c}=A\left(0.3\right)-A\left(1.56\right)\\ =0.3821-0.0594\\ =0.3227\end{array}$

Thus, The value of $P\left(-0.9\le Z\le 0\right)$ is 0.3227.

To determine

Find the value of $P\left(-0.2\le Z\le 0.2\right)$.

Answer to Problem 58E

The value of $P\left(-0.2\le Z\le 0.2\right)$ is 0.1586.

Explanation of Solution

Calculation:

The following can be observed for Z:

$P\left(Z<-0.2\right)+P\left(-0.2\le Z\le 0.2\right)+P\left(Z>0.2\right)=1$

Since, the standard normal distribution is symmetric about 0, $P\left(Z<-0.2\right)=P\left(Z>0.2\right)$.

$P\left(Z>0.2\right)+P\left(-0.2\le Z\le 0.2\right)+P\left(Z>0.2\right)=1$

Use Table 4: Normal Curve Areas Standard normal probability in right-hand tail to obtain the probability as follows:

• Locate 0.2 along the z column
• Locate 0 along the Second decimal place of z
• The intersection of row and column gives the probability value of 0.4207.

$\begin{array}{c}P\left(Z>0.2\right)+P\left(-0.2\le Z\le 0.2\right)+P\left(Z>0.2\right)=1\\ P\left(-0.2\le Z\le 0.2\right)=1-2\times P\left(Z>0.2\right)\\ =1-2\times A\left(0.2\right)\end{array}$

                                                                                        $\begin{array}{c}=1-2\left(0.4207\right)\\ =1-0.8414\\ =0.1586\end{array}$

Thus, the value of $P\left(-0.2\le Z\le 0.2\right)$ is 0.1586.

To determine

Find the value of $P\left(-1.56\le Z\le -0.2\right)$.

Answer to Problem 58E

The value of $P\left(-1.56\le Z\le -0.2\right)$ is 0.3613.

Explanation of Solution

Calculation:

The following can be observed for Z:

$P\left(Z<-1.56\right)+P\left(-1.56\le Z\le -0.2\right)+P\left(Z>-0.2\right)=1$

Since, the standard normal distribution is symmetric about 0, $P\left(Z<-1.56\right)=P\left(Z>1.56\right)$. Again, $P\left(Z>-0.2\right)=1-P\left(Z<-0.2\right)=1-P\left(Z>0.2\right)$.

$P\left(Z>1.56\right)+P\left(-1.56\le Z\le -0.2\right)+1-P\left(Z>0.2\right)=1$

Use Table 4: Normal Curve Areas Standard normal probability in right-hand tail to obtain the probability as follows:

• Locate 0.2 along the z column
• Locate 0 along the Second decimal place of z
• The intersection of row and column gives the probability value of 0.4207.

Use Table 4: Normal Curve Areas Standard normal probability in right-hand tail to obtain the probability as follows:

• Locate 1.5 along the z column
• Locate 6 along the Second decimal place of z
• The intersection of row and column gives the probability value of 0.0594.

$\begin{array}{c}P\left(Z>1.56\right)+P\left(-1.56\le Z\le -0.2\right)+1-P\left(Z>0.2\right)=1\\ P\left(-1.56\le Z\le -0.2\right)=P\left(Z>0.2\right)-P\left(Z>1.56\right)\\ =A\left(0.2\right)-A\left(1.56\right)\\ =0.4207-0.0594\\ =0.3613\end{array}$

Thus, The value of $P\left(-1.56\le Z\le -0.2\right)$ is 0.3613.

To determine

Find the value of $P\left(0\le Z\le 1.2\right)$ by using Applet.

Explain the values given on the two horizontal axes identical or not.

Answer to Problem 58E

The value of $P\left(0\le Z\le 1.2\right)$ is 0.3849.

Explanation of Solution

Calculation:

The following can be observed for Z:

Step-by-step procedure to obtain the probability value using Applets as follows:

• Enter Mean = 0 and StDev = 1 values in the provided boxes.
• Enter Start  = 0 and End = 1.2 value in the provided boxes.

Output obtained using Applets software is represented as follows:

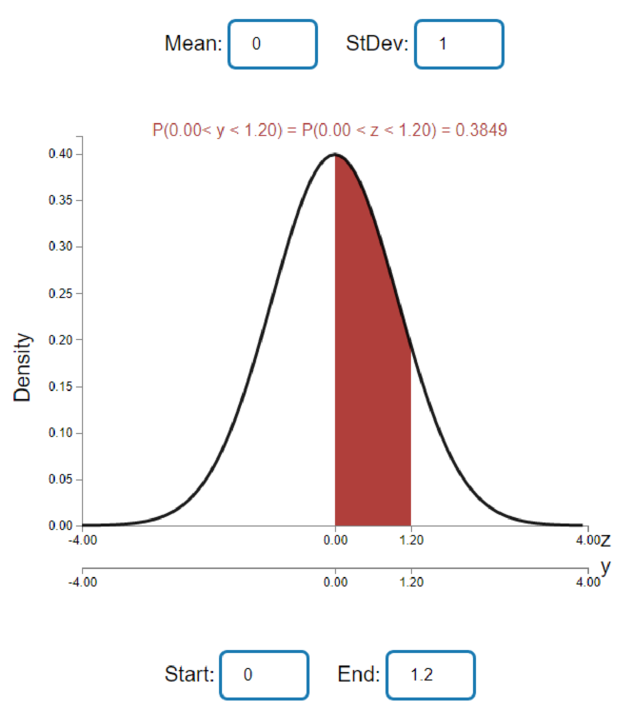
From the above output, the value of $P\left(0\le Z\le 1.2\right)$ is 0.3849.

Thus, the value of $P\left(0\le Z\le 1.2\right)$ is 0.3849.

Explanation:

The values given on the two horizontal axes are identical. It is because, in the above output the top Z axes represent the standard normal variable and they y axes represents the variable of interest. In the given situation the standard normal variable is only used. Hence as a result the both axes provide the same Z value of $P\left(0\le Z\le 1.2\right)$ as 0.3849.