
a.
Draw a histogram for the travel time distribution.
a.
Answer to Problem 40E
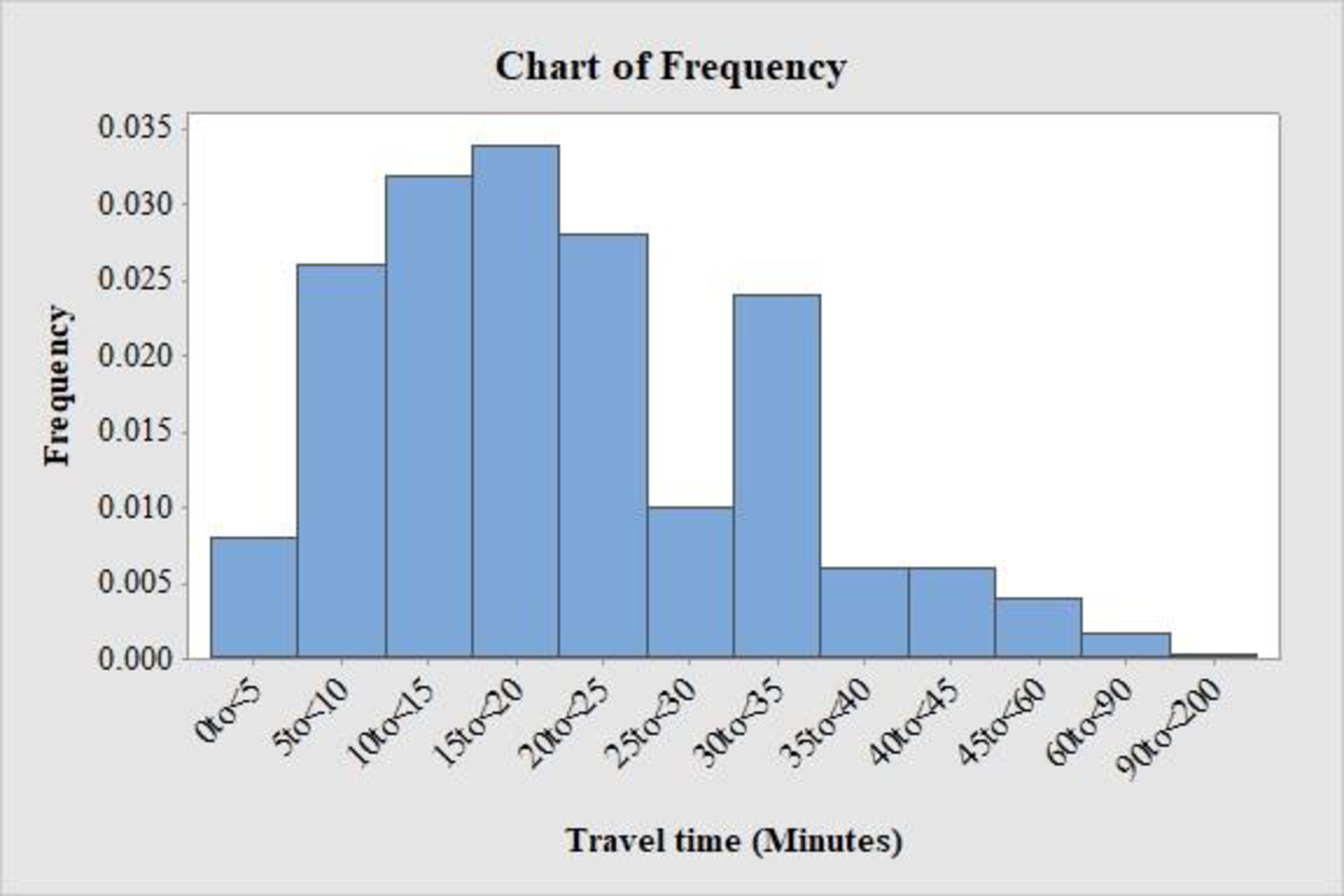
The histogram for the travel time distribution is given below:
Explanation of Solution
Calculation:
The data represent the relative frequency for travel time to work for a large sample of adults who did not work at home.
Software procedure:
Step-by-step procedure to obtain the width using MINITAB software:
- Choose Calc > Calculator.
- Enter the column of width under Store result in variable.
- Enter the formula ‘Upper’-‘Lower’ under Expression.
- Click OK.
The
Step-by-step procedure to obtain the density using MINITAB software:
- Choose Calc > Calculator.
- Enter the column of Frequency under Store result in variable.
- Enter the formula ‘Frequency’/‘width’ under Expression.
- Click OK.
The density is stored in the column “Frequency”.
Step by step procedure to draw the relative frequency histogram using MINITAB software:
- Select Graph > Bar chart.
- In Bars represent select Values from a table.
- In One column of values select Simple.
- Enter Frequency in Graph variables.
- Enter Travel Time (Minutes) in categorical variable.
- Select OK.
- Click on the axis of the graph and in Gap between clusters enter 0.
Thus, the histogram for travel time is obtained.
b.
Delineate the features, such as center, shape, and variability of the histogram from Part (a).
b.
Explanation of Solution
From the histogram, it is observed that the distribution of travel time is slightly positively skewed with a single maximum frequency density, that is, a single
c.
Explain whether it is appropriate to use the
c.
Answer to Problem 40E
No, it is not appropriate to use empirical rule to make statements about the travel time distribution.
Explanation of Solution
By observing the histogram, the travel time data are skewed right. However, the empirical rule is applicable for data that are distributed normally, which is bell-shaped and symmetric.
Therefore, in this context, it is not appropriate to use the empirical rule to make the statements about the travel time distribution.
d.
Elucidate the reason why the travel time distribution could not be well approximated by a normal curve.
d.
Explanation of Solution
It is given that the approximate
The observation of the travel time should not be negative. In general, the travel time begins from 0.
If the distribution of the travel time is approximately normal, then the percentage of the travel time that fall below 0 is obtained as given below:
Use the standard normal probabilities (cumulative z-curve area) table to find the z-value:
Procedure:
For z at –1.12:
- Locate –1.1 in the left column of the table.
- Obtain the value in the corresponding row below 0.02.
That is,
Therefore, the percentage of the travel time that fall below 0 is 13.14% that is not possible.
Hence, the distribution of the travel time cannot be approximated by the normal curve.
e.
Find the percentage of travel time between 0 and 75 minutes using Chebyshev’s rule.
Obtain the percentage of travel time between 0 and 47 minutes using Chebyshev’s rule.
e.
Answer to Problem 40E
The percentage of travel time between 0 and 75 minutes is at least 75%.
The percentage of travel time between 0 and 47 minutes is at least 21%.
Explanation of Solution
Calculation:
The approximate mean and standard deviation for the travel time distribution are 27 minutes and 24 minutes, respectively.
The values at 2 standard deviations away from the mean is obtained as follows:
The observation of the travel time should not be negative. Therefore, the travel time that begins at –21 minutes lies in 2 standard deviation below the mean that is not possible. Travel time 75 minutes lies 2 standard deviations above from the mean.
Chebyshev’s rule:
For any number k, where
The z-score gives the number of standard deviations that an observation of 75 minutes is away from the mean. Here, the z-scores for 0 minutes and 75 minutes are –2 and 2, respectively. Thus, these observations of time are 2 standard deviations from the mean that is
(i).
The percentage of travel time between 0 and 75 minutes is obtained as given below:
Use Chebyshev’s rule as follows:
In this context, at least 0.75 proportion of observations lie within 2 standard deviations of the mean.
Therefore, the percentage of travel time between 0 and 75 minutes is 75%.
(ii).
A travel time of 47 minutes is
A travel time of 0 minutes is
Since 1.125 is larger than 0.833, these observations of time are 1.125 standard deviations from the mean that is
The percentage of the travel times between 0 and 47 minutes is obtained as given below:
Use Chebyshev’s rule as follows:
Thus, the percentage of the travel time between 0 and 47 minutes is 21%.
f.
Explain the way why the statements in Part (e) agree with the actual percentage for the travel time distribution.
f.
Explanation of Solution
Calculation:
The actual percentage of travel time between 0 and 75 minutes is obtained as given below:
Therefore, the actual percentage of travel time between 0 and 75 minutes is approximately 95.5% and differs a lot from the percentage obtained using Chebyshev’s rule.
The actual percentage of travel times between 0 and 47 minutes is obtained as given below:
Therefore, the actual percentage of travel time between 0 and 47 minutes is approximately 90% and differs a lot from the percentage obtained using Chebyshev’s rule.
Want to see more full solutions like this?
Chapter 4 Solutions
Bundle: Introduction to Statistics and Data Analysis, 5th + WebAssign Printed Access Card: Peck/Olsen/Devore. 5th Edition, Single-Term
- A company found that the daily sales revenue of its flagship product follows a normal distribution with a mean of $4500 and a standard deviation of $450. The company defines a "high-sales day" that is, any day with sales exceeding $4800. please provide a step by step on how to get the answers in excel Q: What percentage of days can the company expect to have "high-sales days" or sales greater than $4800? Q: What is the sales revenue threshold for the bottom 10% of days? (please note that 10% refers to the probability/area under bell curve towards the lower tail of bell curve) Provide answers in the yellow cellsarrow_forwardFind the critical value for a left-tailed test using the F distribution with a 0.025, degrees of freedom in the numerator=12, and degrees of freedom in the denominator = 50. A portion of the table of critical values of the F-distribution is provided. Click the icon to view the partial table of critical values of the F-distribution. What is the critical value? (Round to two decimal places as needed.)arrow_forwardA retail store manager claims that the average daily sales of the store are $1,500. You aim to test whether the actual average daily sales differ significantly from this claimed value. You can provide your answer by inserting a text box and the answer must include: Null hypothesis, Alternative hypothesis, Show answer (output table/summary table), and Conclusion based on the P value. Showing the calculation is a must. If calculation is missing,so please provide a step by step on the answers Numerical answers in the yellow cellsarrow_forward
 Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,





