Chapter 4.1, Problem 46E

To determine

To graph: The two approximate solutions of the differential equation $\frac{dy}{dx}=-\frac{1}{x^2}$ on the slope field, one of which passes through the indicated point $\left(1,3\right)$.

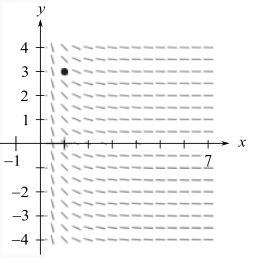
(b)

To determine

To calculate: The particular solution of differential equation $\frac{dy}{dx}=-\frac{1}{x^2}$ by using integration and the point $\left(1,3\right)$ and then graph the solution by graphing utility and then compare the result obtained with the sketch in part(a) that passes through given point $\left(1,3\right)$.