
(a)
Interpretation:
The average velocity of the given liquid flowing in the pipe is to be calculated.
Concept Introduction:
The formula to calculate the average velocity of a stream which is flowing through a pipe of cross-sectional area S is:
Here, u is the local velocity of the stream in the differential cross-sectional area dS .
(a)
Answer to Problem 4.1P
The average velocity of the given liquid flowing in the pipe is
Explanation of Solution
Given information:
In a
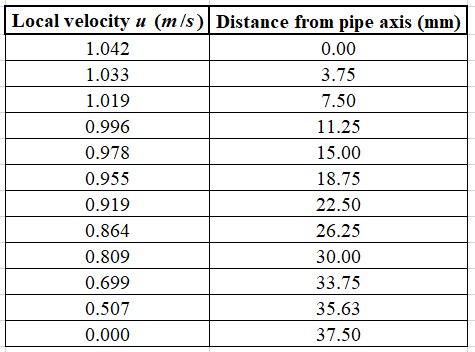
The cross-sectional area of the pipe is defined as:
Here,
Here,
Use equation (2) and (3) in equation (1) and simplify further as
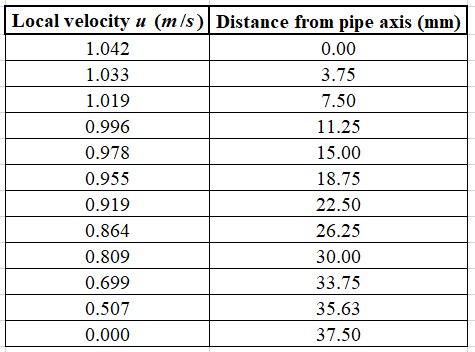
The table for
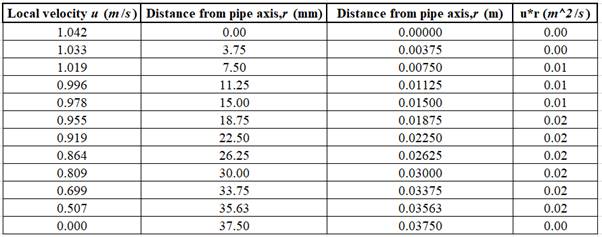
Now, plot the graph of
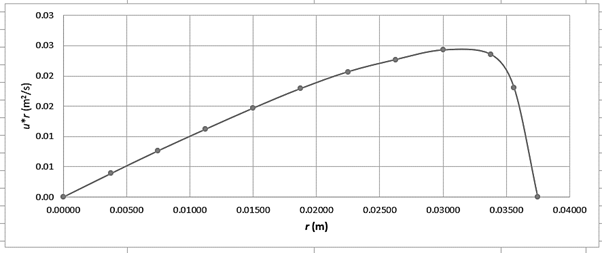
The area under this curve is defined as
Now, use this value to calculate the average velocity as:
(b)
Interpretation:
The correction factor for the kinetic energy of the given liquid flowing in the pipe is to be calculated.
Concept Introduction:
The formula to calculate the correction factor
Here, u is the local velocity of the stream in the differential cross-sectional area dS and
(b)
Answer to Problem 4.1P
The correction factor for the kinetic energy of the given liquid flowing in the pipe is
Explanation of Solution
Given information:
In a
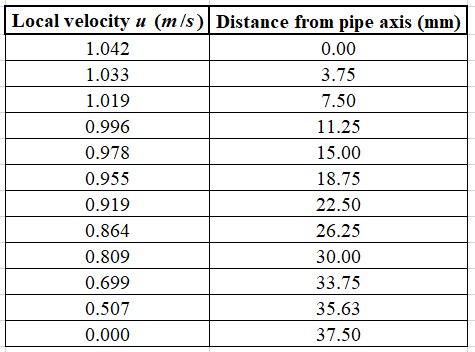
The cross-sectional area of the pipe is defined as:
Here,
Here,
Use equation (2) and (3) in equation (4) and simplify further as:
Use the value of
The table for
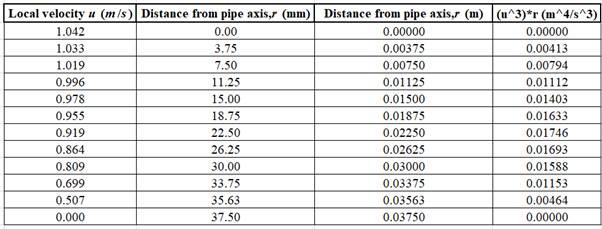
Now, plot the graph of
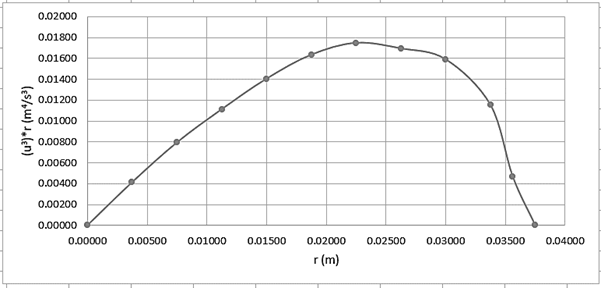
The area under this curve is defined as
Now, use this value to calculate the correction factor
(c)
Interpretation:
The correction factor for the momentum of the given liquid flowing in the pipe is to be calculated.
Concept Introduction:
The formula to calculate the correction factor
Here, u is the local velocity of the stream in the differential cross-sectional area dS and
(c)
Answer to Problem 4.1P
The correction factor for the momentum of the given liquid flowing in the pipe is
Explanation of Solution
Given information:
In a
The cross-sectional area of the pipe is defined as:
Here,
Here,
Use equation (2) and (3) in equation (5) and simplify further as:
Use the value of
The table for
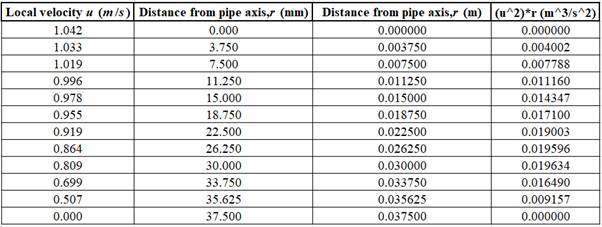
Now, plot the graph of
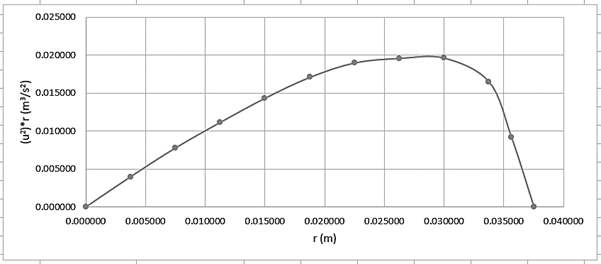
The area under this curve is defined as
Now, use this value to calculate the correction factor
Want to see more full solutions like this?
Chapter 4 Solutions
Unit Operations of Chemical Engineering
- chemical engineering. The answer is minus 1.26 KJ/mol for H(3). Demonstrate the reference state to the process state and calculations. I only need help for determing that variable.arrow_forwardExhaust gas from a power plant passes through a 15-by-20-it rectangular duct at an average velocity of 50 ft/s. The total length of duct is 250 ft and there are two 90° bends.The gas is at 180°F and about 1 atm, and the properties are similar to those of air. Calculate the pressure drop in the duet and the power required to overcome pressure losses.arrow_forwardUntuk sistem gas etilena (1)/propilena (2), estimasi (f^1, f^2, $^1, dan ^2 pada t = 150°C, P = 30 bar, dan y1 = 0,35; kij = 0. (a) Dengan menerapkan Persamaan (10.63). (b) Dengan asumsi bahwa campuran adalah lingkungan idealarrow_forward
- Only focus on H(3), which is the specific enthalpy for nitrogen gas. chemical engineeringarrow_forwardchemical engineering. Only focus on H(3), which is the nitrogen gas. Start with the reference state to the process state. Be thorough to the fullestarrow_forwardacetone with these parameters: po:=101325; #Standard atmospheric pressure in PaTfo:=273.15-94.45; #Melting temperature in K Tvo:=273.15+56.15; #Boiling temperature in K Hv:=31270; #Enthalpy of vaporization in J/molR:=8.314; #Gas Constant in J/mol*KNLe:=1.76; #Lewis number for acetoneMw:= 0.05808 ; #kg/mol molecular weight of acetoneW0:= 0.15; Wsp:=0.005;Am:= 0.12; #m^2/kg dry solid for the exposed wet areah:= 11; #W/m^2K for heat transfer coefficienttau__min:= Hv*(W0-Wsp)/Mw/Am/h/(T8-TS); tau__min/60;arrow_forward
- chemical engineering Material-energy balance. Only focus on the nitrogen gas, which is H(3)arrow_forward1. The settling chamber, shown schematically in Figure 2E1.1, is used as a primary separation device in the removal of dust particles of density 1500 kg/m³ from a gas of density 0:7 kg/m³ and viscosity 1.90 x 10-5 Pa s. Gas inlet Elevation Gas Gas exit exit H Collection surface -W Section X-X Dimensions: H=3m L = 10 m W=2m Figure 2E1.1 Schematic diagram of settling chamber Assuming Stokes' law applies, show that the efficiency of collection of particles of size x is given by the expression collection efficiency, x = x²8(pp - Pi)L 18μHU where U is the uniform gas velocity through the parallel-sided section of the chamber. State any other assumptions made. (b) What is the upper limit of particle size for which Stokes' law applies? (c) When the volumetric flow rate of gas is 0.9 m³/s, and the dimensions of the chamber are those shown in Figure 2E1.1, determine the collection efficiency for spherical particles of diameter 30 mm.arrow_forwardCan you answer this sequantially correct like show me the full process. Also, since it is chemical engineering related problem a perry's handbook is used. Thank youarrow_forward
- chemical engineering Demonstrate how each specific enthalpy was calculated, from the reference state to the process state. Be thorough to the fullest. This is a material-energy balance. The answers are H(1) = 35.7 KJ/kmol, H(2) = 32.0 KJ/kmol, and H(3) = -1.26 KJ/kmol.arrow_forwardheat and mass transfer:arrow_forwardChemical Engineering. Be thorough to the fullest for the three enthalpies. H(1) = 35.7 kj/kmol H(2) =32.0 Kj/kmol H(3)= -1.26 Kj/kmolarrow_forward
 Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education
Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY
Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
 Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning
Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The
Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The





