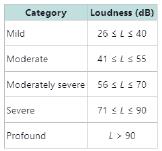
Problem 1SP: Solve. a.42x3=64b.272w+5=1325w Problem 2SP: Solve. 5x=83 Problem 3SP: Solve. a.400+104x1=63,000b.100=700e0.2k Problem 4SP: Solve. 35x6=24x+1 Problem 5SP: Solve. e2x5ex14=0 Problem 6SP: Solve. log27x4=log22x+1 Problem 7SP: Solve. lnx+lnx8=lnx20 Problem 8SP: Solve. 8log4w+6=24 Problem 9SP: Solve. logt18=1.4 Problem 10SP: Solve. 2log7x=log7x48 Problem 11SP: Determine how long it will take $8000 compounded monthly at 6 to double. Round to 1 decimal place. Problem 12SP: a. Find the intensity of sound form a leaf blower it the decibel level is 115 dB. b. Is the... Problem 1PE: An equation such as 4x=9 is called an equation because the equation contains a variable in the... Problem 2PE Problem 3PE: The equivalence property of logarithmic expressions states that if logbx=logby,then=. Problem 4PE: An equation containing a variable within a logarithmic expression is called a equation. Problem 5PE: For Exercises 5-16, solve the equation. (See Example 1) 3x=81 Problem 6PE: For Exercises 5-16, solve the equation. (See Example 1) 2x=32 Problem 7PE: For Exercises 5-16, solve the equation. (See Example 1) 53=5t Problem 8PE: For Exercises 5-16, solve the equation. (See Example 1) 3=3w Problem 9PE: For Exercises 5-16, solve the equation. (See Example 1) 23y+1=16 Problem 10PE: For Exercises 5-16, solve the equation. (See Example 1) 52z+2=625 Problem 11PE: For Exercises 5-16, solve the equation. (See Example 1) 113c+1=111c5 Problem 12PE: For Exercises 5-16, solve the equation. (See Example 1) 72x3=149x+1 Problem 13PE: For Exercises 5-16, solve the equation. (See Example 1) 82x5=32x6 Problem 14PE: For Exercises 5-16, solve the equation. (See Example 1) 27x4=92x+1 Problem 15PE: For Exercises 5-16, solve the equation. (See Example 1) 1003t5=10003t Problem 16PE: For Exercises 5-16, solve the equation. (See Example 1) 100,0002w+1=10,0004w Problem 17PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 18PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 19PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 20PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 21PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 22PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 23PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 24PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 25PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 26PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 27PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 28PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 29PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 30PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 31PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 32PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 33PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 34PE: For Exercises 17-34, solve the equation. Write the solution set the exact values given in terms of... Problem 35PE: For Exercises 35-36, determine if the given value of x is a solution to the logarithmic equation.... Problem 36PE: For Exercises 35-36, determine if the given value of x is a solution to the logarithmic equation.... Problem 37PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 38PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 39PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 40PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 41PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 42PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 43PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 44PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 45PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 46PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 47PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 48PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 49PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 50PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 51PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 52PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 53PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 54PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 55PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 56PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 57PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 58PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 59PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 60PE: For Exercises 37-60, solve the equation. Write the solution set with the set with the exact... Problem 61PE: For Exercises 61-70, use the model A=PertorA=P1+rnnt, where A is the future value of P dollars... Problem 62PE: For Exercises 61-70, use the model A=PertorA=P1+rnnt, where A is the future value of P dollars... Problem 63PE: For Exercises 61-70, use the model A=PertorA=P1+rnnt, where A is the future value of P dollars... Problem 64PE: For Exercises 61-70, use the model A=PertorA=P1+rnnt, where A is the future value of P dollars... Problem 65PE: For Exercises 61-70, use the model A=PertorA=P1+rnnt, where A is the future value of P dollars... Problem 66PE: For Exercises 61-70, use the model A=PertorA=P1+rnnt, where A is the future value of P dollars... Problem 67PE: For Exercises 61-70, use the model A=PertorA=P1+rnnt, where A is the future value of P dollars... Problem 68PE: For Exercises 61-70, use the model A=PertorA=P1+rnnt, where A is the future value of P dollars... Problem 69PE: For Exercises 61-70, use the model A=PertorA=P1+rnnt, where A is the future value of P dollars... Problem 70PE: For Exercises 61-70, use the model A=PertorA=P1+rnnt, where A is the future value of P dollars... Problem 71PE: Physicians often treat thyroid cancer with a radioactive form of iodine called iodiro-131 131I . The... Problem 72PE: Caffeine occurs naturally in a variety of food products such as coffee, tea, and chocolate. The... Problem 73PE: Sunlight is absorbed in water, and as a result the light intensity in oceans, lakes, and ponds... Problem 74PE: Sunlight is absorbed in water, and as a result the light intensity in oceans, lakes, and ponds... Problem 75PE: Sunlight is absorbed in water, and as a result the light intensity in oceans, lakes, and ponds... Problem 76PE: Sunlight is absorbed in water, and as a result the light intensity in oceans, lakes, and ponds... Problem 77PE: Forge welding is a process in which two pieces of steel are joined together by heating the pieces of... Problem 78PE: A pie comes out of the oven at 325oF and is placed to cool in a 70oF kitchen. The temperature of the... Problem 79PE Problem 80PE: For Exercises 79-80, the formula L=10logII0 gives the loudness of sound L (in dB) based on the... Problem 81PE Problem 82PE Problem 83PE: A new teaching method to teach vocabulary to sixth-graders involves having students work in group on... Problem 84PE Problem 85PE: Radiated seismic energy from an earthquake is estimated by logE=4.4+1.5M, where E is the energy in... Problem 86PE: On August 31, 1854, an epidemic of cholera was discovered in London, England, resulting from a... Problem 87PE: For Exercises 87-94, find an equation for the inverse function. fx=2x7 Problem 88PE: For Exercises 87-94, find an equation for the inverse function. fx=5x+6 Problem 89PE: For Exercises 87-94, find an equation for the inverse function. fx=lnx+5 Problem 90PE: For Exercises 87-94, find an equation for the inverse function. fx=lnx7 Problem 91PE: For Exercises 87-94, find an equation for the inverse function. fx=10x3+1 Problem 92PE: For Exercises 87-94, find an equation for the inverse function. fx=10x+24 Problem 93PE: For Exercises 87-94, find an equation for the inverse function. fx=logx+79 Problem 94PE: For Exercises 87-94, find an equation for the inverse function. fx=logx11+8 Problem 95PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 96PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 97PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 98PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 99PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 100PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 101PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 102PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 103PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 104PE Problem 105PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 106PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 107PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 108PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 109PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 110PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 111PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 112PE: For Exercises 95-112, solve the equation. Write the solution set with exact solutions. Also give... Problem 113PE: Explain the process to solve the equation 4x=11. Problem 114PE Problem 115PE Problem 116PE: For Exercises 115-126, solve the equation. ex9ex2=4 Problem 117PE: For Exercises 115-126, solve the equation. lnx2lnx5=4 Problem 118PE Problem 119PE: For Exercises 115-126, solve the equation. logx2=logx2 Problem 120PE: For Exercises 115-126, solve the equation. logx2=logx3 Problem 121PE: For Exercises 115-126, solve the equation. logw+4logw12=0 Problem 122PE: For Exercises 115-126, solve the equation. lnx+3lnx10=0 Problem 123PE: For Exercises 115-126, solve the equation. e2x8ex+6=0 Problem 124PE: For Exercises 115-126, solve the equation. e2x6ex+4=0 Problem 125PE: For Exercises 115-126, solve the equation. log56c+5+log5c=1 Problem 126PE: For Exercises 115-126, solve the equation. log3x8+log3x=1 Problem 127PE: For Exercises 127-130, an equation is given in the form Y1x=Y2x. Graph Y1andY2 on a graphing utility... Problem 128PE: For Exercises 127-130, an equation is given in the form Y1x=Y2x. Graph Y1andY2 on a graphing utility... Problem 129PE: For Exercises 127-130, an equation is given in the form Y1x=Y2x. Graph Y1andY2 on a graphing utility... Problem 130PE: For Exercises 127-130, an equation is given in the form Y1x=Y2x. Graph Y1andY2 on a graphing utility... format_list_bulleted


 Algebra for College StudentsAlgebraISBN:9781285195780Author:Jerome E. Kaufmann, Karen L. SchwittersPublisher:Cengage Learning
Algebra for College StudentsAlgebraISBN:9781285195780Author:Jerome E. Kaufmann, Karen L. SchwittersPublisher:Cengage Learning
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning






