
Concept explainers
(A)
To explain:
The given assumption of more is better is sufficient for both goods.
Answer to Problem 3.21P
The given assumption more is better is not sufficient for both goods.
Explanation of Solution
Given that −
And,
In the above equation both MUx and MUy are greater than zero. Therefore, the given assumption more is better is not sufficient for both goods.
Introduction:
(B)
To explain:
Whether the marginal utility of x decrease, or remain constant or increase with the consumer buying more of x, if the preferences of consumer are represented by the utility function of Cobb Douglas,
Answer to Problem 3.21P
The marginal utility of x will diminish will not diminish but increases with an increase in the consumption of x by the individual.
Explanation of Solution
The given marginal utility function of x will not diminish but increases, as the individual consumes more of good x.
Introduction:
Marginal utility shows the additional utility that the consumer is deriving from consuming additional units of that product or service.
(C)
Tofind:
The MRSxy.
Answer to Problem 3.21P
After necessary derivations, the MRSxy is given by
Explanation of Solution
Marginal utility shows the additional utility that the consumer is deriving from consuming additional units of that product or service.
Through dividing the marginal utility of x with the marginal utility of y, the marginal rate of substitution can be arrived at.
Implies,
Thus, the MRS of x for y will be
Now, it can be concluded that the MRSxy will decrease when x increases, and MRSxy also will decrease when y decreases. Since, in both these cases MRSxy decreases, it stays decreasing.
(D)
To evaluate:
Whether the MRSxy will decrease or increase or remain constant, if the individual decides to substitute x for y along an indifference curve.
Answer to Problem 3.21P
After necessary observations, it can be said that the MRSxy will be diminishing.
Explanation of Solution
Marginal utility shows the additional utility that the consumer is deriving from consuming additional units of that product or service.
MRSxy, denoting the marginal rate of substitution of x for y, equaling to
(E)
To plot:
A graph with x denoted by horizontal axis and y denoted by vertical axis, an indifference curve, and to label the curve U1.
Answer to Problem 3.21P
As can be seen in the graph plotted in the next section, the indifference curve is bowed towards the origin, owing to the diminishing MRS.
Explanation of Solution
Marginal utility shows the additional utility that the consumer is deriving from consuming additional units of that product or service.
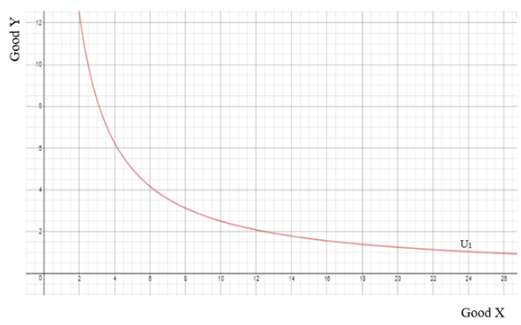
In the graph above, as explained in the requirements, it can be seen that with the diminishing MRS, the indifference curve is bowed towards the origin.
(F)
Toplot:
On the same graph, with x denoted by horizontal axis and y denoted by vertical axis, another indifference curve, U2, as U2> U1.
Answer to Problem 3.21P
As can be seen in the graph plotted in the next section, the indifference curves, U1 and U2, as U2> U1.
Explanation of Solution
Marginal utility shows the additional utility that the consumer is deriving from consuming additional units of that product or service.
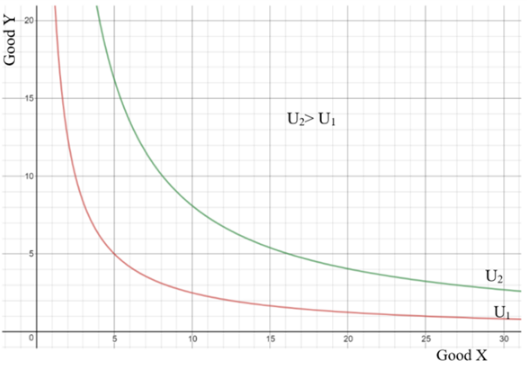
In the graph above, as explained in the requirements, it can be seen that with the diminishing MRS, the indifference curves are bowed towards the origin, satisfying the rule that U2> U1.
Want to see more full solutions like this?
Chapter 3 Solutions
EBK MICROECONOMICS
- 4. Supply and Demand. The table gives hypothetical data for the quantity of electric scooters demanded and supplied per month. Price per Electric Scooter Quantity Quantity Demanded Supplied $150 500 250 $175 475 350 $200 450 450 $225 425 550 $250 400 650 $275 375 750 a. Graph the demand and supply curves. Note if you prefer to hand draw separately, you may and insert the picture separately. Price per Scooter 300 275 250 225 200 175 150 250 400 375425475 350 450 550 650 750 500 850 Quantity b. Find the equilibrium price and quantity using the graph above. c. Illustrate on your graph how an increase in the wage rate paid to scooter assemblers would affect the market for electric scooters. Label any new lines in the same graph above to distinguish changes. d. What would happen if there was an increase in the wage rate paid to scooter assemblers at the same time that tastes for electric scooters increased? 1ངarrow_forward3. Production Costs Clean 'n' Shine is a competitor to Spotless Car Wash. Like Spotless, it must pay $150 per day for each automated line it uses. But Clean 'n' Shine has been able to tap into a lower-cost pool of labor, paying its workers only $100 per day. Clean 'n' Shine's production technology is given in the following table. To determine its short-run cost structure, fill in the blanks in the table. Fill in the columns below. Outpu Capita Labor TFC TVC TC MC AFC AVC ATC 1 0 30 1 1 70 1 2 120 1 3 160 1 4 190 1 5 210 1 6 a. Over what range of output does Clean 'n' Shine experience increasing marginal returns to labor? Over what range does it experience diminishing marginal returns to labor? (*answer both questions) b. As output increases, do average fixed costs behave as described in the text? Explain. C. As output increases, do marginal cost, average variable cost, and average total cost behave as described in the text? Explain. d. Looking at the numbers in the table, but without…arrow_forward2. Elasticity and the Minimum Wage - The following graph depicts two labor markets for cashiers. We assume the same supply curve (cashiers respond similarly to wage offers in each city) but different demand functions (employer demand is more elastic – more responsive to wages - in one city than the other, perhaps because one has higher quality retail stores than the other). The y-axis shows hourly wages in dollars; the x-axis shows the number of employees in hundreds. Wage 12 11 29 10 9 00 8 7 Supply 5 4 3 2 1 D2 12 D1 0 0 1 2 3 4 5 6 7 8 9 10 11 12 Employment 11 With minimum wage of 8 dollars: A. What is the equilibrium level of employment before the minimum wage is imposed? B. A) According to the graph and given a minimum wage of 8 dollars, how many workers would employers want to hire if the demand for workers in City #1 looked like D1? B) How does that number compare to the market equilibrium employment? C. A) In City #1 (with demand curve D1), would there be an excess supply of…arrow_forward
- The demand function for organic apples is given by Qd = 20 – 2P while the supply function is given by Qs = 4P – 10.a. Solve for the equilibrium P* and Q*.b. Carefully graph the D & S curves. Include all intercepts and P* and Q* (**enlarge your graph so you can better show the questions below use graphing paper**)i. Suppose that the government legislates a $1/gallon to be collected from the buyer. Identify the new equation for the demand curve. Plot the new demand curve (on the same graph as b).ii. Solve for the new equilibrium PT* and QT* and indicate on your graph. On the same graph, indicate the P that consumers pay (PC) and the P that producers get to keep (PS).c. On another graph with the original D and S curves, impose the same tax ($1/gallon) to sellers. Identify the new equation for the supply curve. Plot the new supply curve. i. Solve for the new equilibrium PT* and QT* and indicate on your graph. On thesame graph, indicate the P that consumers pay (PC) and the P that…arrow_forwardDon't use ai to answer I will report you answerarrow_forwardExplain and evaluate the impact of legislation on the U.S. criminal justice system, specifically on the prison population and its impact on poverty and the U.S. economy. Include significant elements and limitations such as the War on Drugs and the First Step Act.arrow_forward
- Given the following petroleum tax details, calculate the marginal tax rate and explain its significance: Total Revenue: $500 million Cost of Operations: $200 million Tax Rate: 40% Additional Royalty: 5% Profit-Based Tax: 10%arrow_forwardUse a game tree to illustrate why an aircraft manufacturer may price below the current marginal cost in the short run if it has a steep learning curve. (Hint: Show that learning by doing lowers its cost in the second period.) Part 2 Assume for simplicity the game tree is illustrated in the figure to the right. Pricing below marginal cost reduces profits but gives the incumbent a cost advantage over potential rivals. What is the subgame perfect Nash equilibrium?arrow_forwardAnswerarrow_forward
- M” method Given the following model, solve by the method of “M”. (see image)arrow_forwardAs indicated in the attached image, U.S. earnings for high- and low-skill workers as measured by educational attainment began diverging in the 1980s. The remaining questions in this problem set use the model for the labor market developed in class to walk through potential explanations for this trend. 1. Assume that there are just two types of workers, low- and high-skill. As a result, there are two labor markets: supply and demand for low-skill workers and supply and demand for high-skill workers. Using two carefully drawn labor-market figures, show that an increase in the demand for high skill workers can explain an increase in the relative wage of high-skill workers. 2. Using the same assumptions as in the previous question, use two carefully drawn labor-market figures to show that an increase in the supply of low-skill workers can explain an increase in the relative wage of high-skill workers.arrow_forwardPublished in 1980, the book Free to Choose discusses how economists Milton Friedman and Rose Friedman proposed a one-sided view of the benefits of a voucher system. However, there are other economists who disagree about the potential effects of a voucher system.arrow_forward
 Exploring EconomicsEconomicsISBN:9781544336329Author:Robert L. SextonPublisher:SAGE Publications, Inc
Exploring EconomicsEconomicsISBN:9781544336329Author:Robert L. SextonPublisher:SAGE Publications, Inc


 Economics (MindTap Course List)EconomicsISBN:9781337617383Author:Roger A. ArnoldPublisher:Cengage Learning
Economics (MindTap Course List)EconomicsISBN:9781337617383Author:Roger A. ArnoldPublisher:Cengage Learning





