Single Variable Calculus: Early Transcendentals
8th Edition
ISBN: 9781305270336
Author: James Stewart
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 2.8, Problem 1E
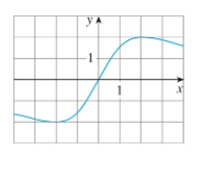
Use the given graph to estimate the value of each derivative. Then sketch the graph of f'.
(a) f'(–3)
(b) f' (–2)
(c) f'(–1)
(d) f'(0)
(e) f'(l)
(f) f'(2)
(g) f'(3)
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
An airplane flies due west at an airspeed of 428 mph. The wind blows in the direction of 41° south of west
at 50 mph. What is the ground speed of the airplane? What is the bearing of the airplane?
428 mph
41°
50 mph
a. The ground speed of the airplane is
b. The bearing of the airplane is
mph.
south of west.
Rylee's car is stuck in the mud. Roman and Shanice come along in a truck to help pull her out. They attach
one end of a tow strap to the front of the car and the other end to the truck's trailer hitch, and the truck
starts to pull. Meanwhile, Roman and Shanice get behind the car and push. The truck generates a
horizontal force of 377 lb on the car. Roman and Shanice are pushing at a slight upward angle and generate
a force of 119 lb on the car. These forces can be represented by vectors, as shown in the figure below. The
angle between these vectors is 20.2°. Find the resultant force (the vector sum), then give its magnitude
and its direction angle from the positive x-axis.
119 lb
20.2°
377 lb
a. The resultant force is
(Tip: omit degree notations from your answers; e.g. enter cos(45) instead of cos(45°))
b. It's magnitude is
lb.
c. It's angle from the positive x-axis is
Find a plane containing the point (3, -3, 1) and the line of intersection of the planes 2x + 3y - 3z = 14
and -3x - y + z = −21.
The equation of the plane is:
Chapter 2 Solutions
Single Variable Calculus: Early Transcendentals
Ch. 2.1 - A Lank holds 1000 gallons o f water, which drains...Ch. 2.1 - A cardiac monitor is used to measure the heart...Ch. 2.1 - The point P(2, 1) lies on the curve y = 1/(1 x)....Ch. 2.1 - The point P(0.5, 0) lies on the curve y = cos x....Ch. 2.1 - If a ball is thrown into the air with a velocity...Ch. 2.1 - If a rock is thrown upward on the planet Mars with...Ch. 2.1 - The table shows the position of a motorcyclist...Ch. 2.1 - The displacement (in centimeters) of a particle...Ch. 2.1 - The point P(1, 0) lies on the curve y = sin(l0/x)....Ch. 2.2 - Prob. 1E
Ch. 2.2 - Explain what it means to say that...Ch. 2.2 - Explain the meaning of each of the following. (a)...Ch. 2.2 - Use the given graph of f to state the value of...Ch. 2.2 - For the function f whose graph is given, state the...Ch. 2.2 - For the function h whose graph is given, state the...Ch. 2.2 - For the function g whose graph is given, state the...Ch. 2.2 - For the function A whose graph is shown, state the...Ch. 2.2 - For the function f whose graph is shown, state the...Ch. 2.2 - Prob. 10ECh. 2.2 - Sketch the graph of the function and use it to...Ch. 2.2 - Sketch the graph of the function and use it to...Ch. 2.2 - Prob. 13ECh. 2.2 - Prob. 14ECh. 2.2 - Prob. 15ECh. 2.2 - Sketch the graph of an example of a function f...Ch. 2.2 - Sketch the graph of an example of a function f...Ch. 2.2 - Prob. 18ECh. 2.2 - Prob. 19ECh. 2.2 - Guess the value of the limit (if it exists) by...Ch. 2.2 - Prob. 21ECh. 2.2 - Prob. 22ECh. 2.2 - Prob. 23ECh. 2.2 - Prob. 24ECh. 2.2 - Prob. 25ECh. 2.2 - Prob. 26ECh. 2.2 - Prob. 27ECh. 2.2 - Prob. 28ECh. 2.2 - Prob. 29ECh. 2.2 - Prob. 30ECh. 2.2 - Prob. 31ECh. 2.2 - Prob. 32ECh. 2.2 - Determine the infinite limit. limx12x(x1)2Ch. 2.2 - Prob. 34ECh. 2.2 - Prob. 35ECh. 2.2 - Prob. 36ECh. 2.2 - Prob. 37ECh. 2.2 - Prob. 38ECh. 2.2 - Prob. 39ECh. 2.2 - Prob. 40ECh. 2.2 - Prob. 41ECh. 2.2 - Prob. 42ECh. 2.2 - Prob. 43ECh. 2.2 - Prob. 44ECh. 2.2 - Determine limx11x31 and limx1+1x31 (a) by...Ch. 2.2 - Prob. 46ECh. 2.2 - (a) Estimate the value of the limit limx0 (1 +...Ch. 2.2 - Prob. 49ECh. 2.2 - Prob. 50ECh. 2.2 - Prob. 51ECh. 2.2 - Prob. 52ECh. 2.2 - Prob. 53ECh. 2.2 - Prob. 54ECh. 2.2 - Prob. 55ECh. 2.3 - Given that limx2f(x)=4limx2g(x)=2limx2h(x)=0 find...Ch. 2.3 - Tire graphs of f and g are given. Use them to...Ch. 2.3 - Prob. 3ECh. 2.3 - Prob. 4ECh. 2.3 - Prob. 5ECh. 2.3 - Prob. 6ECh. 2.3 - Prob. 7ECh. 2.3 - Prob. 8ECh. 2.3 - Prob. 9ECh. 2.3 - (a) What is wrong with the following equation?...Ch. 2.3 - Prob. 11ECh. 2.3 - Evaluate the limit, if it exists. limx3x2+3xx2x12Ch. 2.3 - Prob. 13ECh. 2.3 - Prob. 14ECh. 2.3 - Prob. 15ECh. 2.3 - Evaluate the limit, if it exists....Ch. 2.3 - Prob. 17ECh. 2.3 - Prob. 18ECh. 2.3 - Prob. 19ECh. 2.3 - Prob. 20ECh. 2.3 - Prob. 21ECh. 2.3 - Prob. 22ECh. 2.3 - Prob. 23ECh. 2.3 - Evaluate the limit, if it exists. limh0(3+h)131hCh. 2.3 - Prob. 25ECh. 2.3 - Prob. 26ECh. 2.3 - Prob. 27ECh. 2.3 - Prob. 28ECh. 2.3 - Prob. 29ECh. 2.3 - Evaluate the limit, if it exists. limx4x2+95x+4Ch. 2.3 - Prob. 31ECh. 2.3 - Evaluate the limit, if it exists. limh01(xh)21x2hCh. 2.3 - Prob. 33ECh. 2.3 - Prob. 34ECh. 2.3 - Prob. 35ECh. 2.3 - Prob. 36ECh. 2.3 - If 4x 9 f(x) x2 4x + 7 for x 0, find limx4f(x)Ch. 2.3 - If 2x g(x) x4 x2 + 2 for all x, evaluate...Ch. 2.3 - Prove that limx0x4cos2x=0.Ch. 2.3 - Prob. 40ECh. 2.3 - Prob. 41ECh. 2.3 - Find the limit, if it exists. If the limit does...Ch. 2.3 - Prob. 43ECh. 2.3 - Prob. 44ECh. 2.3 - Prob. 45ECh. 2.3 - Prob. 46ECh. 2.3 - Prob. 47ECh. 2.3 - Let g(x) =sgn(sinx). (a) Find each of the...Ch. 2.3 - Prob. 49ECh. 2.3 - Prob. 50ECh. 2.3 - Prob. 51ECh. 2.3 - l.et g(x)={xifx13ifx=12xif1x2x3ifx2 (a) Evaluate...Ch. 2.3 - Prob. 53ECh. 2.3 - Prob. 54ECh. 2.3 - Prob. 55ECh. 2.3 - Prob. 56ECh. 2.3 - Prob. 57ECh. 2.3 - Prob. 58ECh. 2.3 - If limx1f(x)8x1=10, find limx1f(x).Ch. 2.3 - If limx0f(x)x2=5, find the following limits. (a)...Ch. 2.3 - If f(x)={x2ifxisrational0ifxisirrational prove...Ch. 2.3 - Show by means of an example that limxa[f(x)+g(x)]...Ch. 2.3 - Prob. 63ECh. 2.3 - Prob. 64ECh. 2.3 - Prob. 65ECh. 2.3 - Prob. 66ECh. 2.4 - Use the given graph of f to find a number such...Ch. 2.4 - Use the given graph of f to find a number such...Ch. 2.4 - Use the given graph of f(x)=x to find a number ...Ch. 2.4 - Use the given graph of f(x) =x2 to find a number ...Ch. 2.4 - Use a graph to find a number such that if...Ch. 2.4 - Prob. 6ECh. 2.4 - Prob. 7ECh. 2.4 - Prob. 8ECh. 2.4 - Prob. 9ECh. 2.4 - Prob. 10ECh. 2.4 - Prob. 11ECh. 2.4 - Prob. 12ECh. 2.4 - Prob. 13ECh. 2.4 - Prob. 14ECh. 2.4 - Prob. 15ECh. 2.4 - Prob. 16ECh. 2.4 - Prob. 17ECh. 2.4 - Prob. 18ECh. 2.4 - Prob. 19ECh. 2.4 - Prob. 20ECh. 2.4 - Prob. 21ECh. 2.4 - Prob. 22ECh. 2.4 - Prob. 23ECh. 2.4 - Prob. 24ECh. 2.4 - Prob. 25ECh. 2.4 - Prob. 26ECh. 2.4 - Prob. 27ECh. 2.4 - Prob. 28ECh. 2.4 - Prob. 29ECh. 2.4 - Prove the statement using the , definition of a...Ch. 2.4 - Prob. 31ECh. 2.4 - Prob. 32ECh. 2.4 - Prob. 33ECh. 2.4 - Prob. 34ECh. 2.4 - Prob. 35ECh. 2.4 - Prob. 36ECh. 2.4 - Prob. 37ECh. 2.4 - Prob. 38ECh. 2.4 - Prob. 39ECh. 2.4 - Prob. 40ECh. 2.4 - Prob. 41ECh. 2.4 - Prob. 42ECh. 2.4 - Prob. 43ECh. 2.4 - Prob. 44ECh. 2.5 - Write an equation that expresses the fact that a...Ch. 2.5 - Prob. 2ECh. 2.5 - (a) From the graph of f , state the numbers at...Ch. 2.5 - Prob. 4ECh. 2.5 - Sketch the graph of a function f that is...Ch. 2.5 - Sketch the graph of a function f that is...Ch. 2.5 - Sketch the graph of a function f that is...Ch. 2.5 - Prob. 8ECh. 2.5 - Prob. 9ECh. 2.5 - Prob. 10ECh. 2.5 - Use the definition of continuity and the...Ch. 2.5 - Prob. 12ECh. 2.5 - Prob. 13ECh. 2.5 - Prob. 14ECh. 2.5 - Prob. 15ECh. 2.5 - Prob. 16ECh. 2.5 - Prob. 17ECh. 2.5 - Prob. 18ECh. 2.5 - Prob. 19ECh. 2.5 - Prob. 20ECh. 2.5 - Prob. 21ECh. 2.5 - Prob. 22ECh. 2.5 - Prob. 23ECh. 2.5 - Prob. 24ECh. 2.5 - Prob. 25ECh. 2.5 - Prob. 26ECh. 2.5 - Prob. 27ECh. 2.5 - Prob. 28ECh. 2.5 - Prob. 29ECh. 2.5 - Prob. 30ECh. 2.5 - Prob. 31ECh. 2.5 - Prob. 32ECh. 2.5 - Prob. 33ECh. 2.5 - Prob. 34ECh. 2.5 - Prob. 35ECh. 2.5 - Prob. 36ECh. 2.5 - Use continuity to evaluate the limit....Ch. 2.5 - Prob. 38ECh. 2.5 - Show that f is continuous on ( , )....Ch. 2.5 - Prob. 40ECh. 2.5 - Find the numbers at which f is discontinuous. At...Ch. 2.5 - Prob. 42ECh. 2.5 - Prob. 43ECh. 2.5 - The gravitational force exerted by the planet...Ch. 2.5 - Prob. 45ECh. 2.5 - Prob. 46ECh. 2.5 - Suppose f and g are continuous functions such that...Ch. 2.5 - Prob. 48ECh. 2.5 - Prob. 49ECh. 2.5 - Prob. 50ECh. 2.5 - If f(x) = x2 + 10 sin x, show that there is a...Ch. 2.5 - Suppose f is continuous on [1, 5] and the only...Ch. 2.5 - Prob. 53ECh. 2.5 - Prob. 54ECh. 2.5 - Prob. 55ECh. 2.5 - Prob. 56ECh. 2.5 - Prob. 57ECh. 2.5 - Prob. 58ECh. 2.5 - (a) Prove that the equation has at least one real...Ch. 2.5 - (a) Prove that the equation has at least one real...Ch. 2.5 - Prob. 61ECh. 2.5 - Prob. 62ECh. 2.5 - Prob. 63ECh. 2.5 - Prob. 64ECh. 2.5 - Prob. 65ECh. 2.5 - Prob. 66ECh. 2.5 - Prob. 67ECh. 2.5 - Prob. 68ECh. 2.5 - Prob. 69ECh. 2.5 - Prob. 70ECh. 2.5 - Prob. 71ECh. 2.5 - Prob. 72ECh. 2.6 - Explain in your own words tile meaning of each of...Ch. 2.6 - Prob. 2ECh. 2.6 - For the function f whose graph is given, state the...Ch. 2.6 - For the function g whose graph is given, state the...Ch. 2.6 - Prob. 5ECh. 2.6 - Prob. 6ECh. 2.6 - Prob. 7ECh. 2.6 - Prob. 8ECh. 2.6 - Prob. 9ECh. 2.6 - Sketch the graph of an example of a function f...Ch. 2.6 - Prob. 11ECh. 2.6 - Prob. 12ECh. 2.6 - Prob. 13ECh. 2.6 - Prob. 14ECh. 2.6 - Prob. 15ECh. 2.6 - Prob. 16ECh. 2.6 - Prob. 17ECh. 2.6 - Prob. 18ECh. 2.6 - Prob. 19ECh. 2.6 - Prob. 20ECh. 2.6 - Prob. 21ECh. 2.6 - Prob. 22ECh. 2.6 - Prob. 23ECh. 2.6 - Prob. 24ECh. 2.6 - Prob. 25ECh. 2.6 - Prob. 26ECh. 2.6 - Prob. 27ECh. 2.6 - Prob. 28ECh. 2.6 - Prob. 29ECh. 2.6 - Prob. 30ECh. 2.6 - Prob. 31ECh. 2.6 - Prob. 32ECh. 2.6 - Prob. 33ECh. 2.6 - Prob. 34ECh. 2.6 - Prob. 35ECh. 2.6 - Prob. 36ECh. 2.6 - Prob. 37ECh. 2.6 - Prob. 38ECh. 2.6 - Prob. 39ECh. 2.6 - Prob. 40ECh. 2.6 - Prob. 41ECh. 2.6 - Prob. 42ECh. 2.6 - Prob. 43ECh. 2.6 - Prob. 44ECh. 2.6 - (a) Estimate the value of limx(x2+x+1+x) by...Ch. 2.6 - Prob. 46ECh. 2.6 - Prob. 47ECh. 2.6 - Find the horizontal and vertical asymptotes of...Ch. 2.6 - Prob. 49ECh. 2.6 - Prob. 50ECh. 2.6 - Prob. 51ECh. 2.6 - Prob. 52ECh. 2.6 - Prob. 53ECh. 2.6 - Prob. 54ECh. 2.6 - Prob. 55ECh. 2.6 - Prob. 56ECh. 2.6 - Find a formula for a function f that satisfies the...Ch. 2.6 - Prob. 58ECh. 2.6 - A function f is a ratio of quadratic functions and...Ch. 2.6 - Prob. 60ECh. 2.6 - Prob. 61ECh. 2.6 - Prob. 62ECh. 2.6 - Prob. 63ECh. 2.6 - Prob. 64ECh. 2.6 - Prob. 65ECh. 2.6 - Prob. 66ECh. 2.6 - Prob. 67ECh. 2.6 - Prob. 68ECh. 2.6 - Prob. 69ECh. 2.6 - Prob. 70ECh. 2.6 - Prob. 71ECh. 2.6 - Prob. 72ECh. 2.6 - Prob. 73ECh. 2.6 - Prob. 74ECh. 2.6 - Prob. 75ECh. 2.6 - Prob. 76ECh. 2.6 - Prob. 77ECh. 2.6 - Prob. 78ECh. 2.6 - Prob. 79ECh. 2.6 - Prob. 80ECh. 2.6 - Prob. 81ECh. 2.7 - A curve has equation y = f(x) (a) Write an...Ch. 2.7 - Graph the curve y = ex in the viewing rectangles [...Ch. 2.7 - Prob. 3ECh. 2.7 - Prob. 4ECh. 2.7 - Find an equation of the tangent line to the curve...Ch. 2.7 - Prob. 6ECh. 2.7 - Prob. 7ECh. 2.7 - Prob. 8ECh. 2.7 - Prob. 9ECh. 2.7 - Prob. 10ECh. 2.7 - Prob. 11ECh. 2.7 - Prob. 12ECh. 2.7 - Prob. 13ECh. 2.7 - If a rock is thrown upward on the planet Mars with...Ch. 2.7 - The displacement (in meters) of a particle moving...Ch. 2.7 - Prob. 16ECh. 2.7 - For the function g whose graph is given, arrange...Ch. 2.7 - Prob. 18ECh. 2.7 - For the function f graphed in Exercise 18: (a)...Ch. 2.7 - Prob. 20ECh. 2.7 - Prob. 21ECh. 2.7 - If the tangent line to y= f(x) at (4, 3) passes...Ch. 2.7 - Sketch the graph of a function f for which f(0) =...Ch. 2.7 - Prob. 24ECh. 2.7 - Sketch the graph of a function q that is...Ch. 2.7 - Prob. 26ECh. 2.7 - Prob. 27ECh. 2.7 - Prob. 28ECh. 2.7 - Prob. 29ECh. 2.7 - Prob. 30ECh. 2.7 - Prob. 31ECh. 2.7 - Prob. 32ECh. 2.7 - Prob. 33ECh. 2.7 - Prob. 34ECh. 2.7 - Prob. 35ECh. 2.7 - Prob. 36ECh. 2.7 - Each limit represents the derivative of some...Ch. 2.7 - Prob. 38ECh. 2.7 - Prob. 39ECh. 2.7 - Prob. 40ECh. 2.7 - Prob. 41ECh. 2.7 - Each limit represents the derivative of some...Ch. 2.7 - Prob. 43ECh. 2.7 - Prob. 44ECh. 2.7 - Prob. 45ECh. 2.7 - Prob. 46ECh. 2.7 - Prob. 47ECh. 2.7 - Prob. 48ECh. 2.7 - Prob. 49ECh. 2.7 - The table shows values of the viral load V(r) in...Ch. 2.7 - Prob. 51ECh. 2.7 - Prob. 52ECh. 2.7 - Prob. 53ECh. 2.7 - Prob. 54ECh. 2.7 - Prob. 55ECh. 2.7 - Prob. 56ECh. 2.7 - The quantity of oxygen that can dissolve in water...Ch. 2.7 - The graph shows the influence of the temperature T...Ch. 2.7 - Prob. 59ECh. 2.7 - Prob. 60ECh. 2.7 - (a) Graph the function f(x)=sinx11000sin(1000x) in...Ch. 2.8 - Use the given graph to estimate the value of each...Ch. 2.8 - Prob. 2ECh. 2.8 - Match the graph of each function in (a)(d) with...Ch. 2.8 - Trace or copy the graph of the given function .f....Ch. 2.8 - Trace or copy the graph of the given function .f....Ch. 2.8 - Prob. 6ECh. 2.8 - Trace or copy the graph of the given function .f....Ch. 2.8 - Trace or copy the graph of the given function .f....Ch. 2.8 - Trace or copy the graph of the given function .f....Ch. 2.8 - Trace or copy the graph of the given function .f....Ch. 2.8 - Prob. 11ECh. 2.8 - Prob. 12ECh. 2.8 - Prob. 13ECh. 2.8 - Prob. 14ECh. 2.8 - The graph shows how the average age of first...Ch. 2.8 - Prob. 16ECh. 2.8 - Prob. 17ECh. 2.8 - Prob. 18ECh. 2.8 - Prob. 19ECh. 2.8 - Prob. 20ECh. 2.8 - Prob. 21ECh. 2.8 - Prob. 22ECh. 2.8 - Prob. 23ECh. 2.8 - Prob. 24ECh. 2.8 - Prob. 25ECh. 2.8 - Prob. 26ECh. 2.8 - Prob. 27ECh. 2.8 - Prob. 28ECh. 2.8 - Prob. 29ECh. 2.8 - Prob. 30ECh. 2.8 - Prob. 31ECh. 2.8 - Prob. 32ECh. 2.8 - Prob. 33ECh. 2.8 - Prob. 34ECh. 2.8 - Prob. 35ECh. 2.8 - Prob. 36ECh. 2.8 - Prob. 37ECh. 2.8 - Water temperature affects the growth rate of brook...Ch. 2.8 - Let P represent the percentage of a city's...Ch. 2.8 - Prob. 40ECh. 2.8 - Prob. 41ECh. 2.8 - Prob. 42ECh. 2.8 - Prob. 43ECh. 2.8 - Prob. 44ECh. 2.8 - Prob. 45ECh. 2.8 - Prob. 46ECh. 2.8 - Prob. 47ECh. 2.8 - Prob. 48ECh. 2.8 - Prob. 49ECh. 2.8 - Prob. 50ECh. 2.8 - Prob. 51ECh. 2.8 - Prob. 52ECh. 2.8 - Prob. 53ECh. 2.8 - Prob. 54ECh. 2.8 - Prob. 55ECh. 2.8 - Prob. 56ECh. 2.8 - Prob. 57ECh. 2.8 - Prob. 58ECh. 2.8 - Prob. 59ECh. 2.8 - Where is the greatest integer function f(x) = [[ x...Ch. 2.8 - Prob. 61ECh. 2.8 - (a) Sketch the graph of the function g(x) = x +...Ch. 2.8 - Prob. 63ECh. 2.8 - Prob. 64ECh. 2.8 - Prob. 65ECh. 2.8 - Prob. 66ECh. 2.8 - Prob. 67ECh. 2 - Explain what each of the following means and...Ch. 2 - Prob. 2RCCCh. 2 - Prob. 3RCCCh. 2 - Prob. 4RCCCh. 2 - Prob. 5RCCCh. 2 - Prob. 6RCCCh. 2 - Prob. 7RCCCh. 2 - Prob. 8RCCCh. 2 - Prob. 9RCCCh. 2 - Prob. 10RCCCh. 2 - Prob. 11RCCCh. 2 - Prob. 12RCCCh. 2 - Prob. 13RCCCh. 2 - Prob. 14RCCCh. 2 - Prob. 15RCCCh. 2 - Prob. 16RCCCh. 2 - Prob. 1RQCh. 2 - Prob. 2RQCh. 2 - Prob. 3RQCh. 2 - Prob. 4RQCh. 2 - Prob. 5RQCh. 2 - Prob. 6RQCh. 2 - Determine whether the statement is true or false....Ch. 2 - Prob. 8RQCh. 2 - Prob. 9RQCh. 2 - Prob. 10RQCh. 2 - Prob. 11RQCh. 2 - Prob. 12RQCh. 2 - Prob. 13RQCh. 2 - Prob. 14RQCh. 2 - Prob. 15RQCh. 2 - Prob. 16RQCh. 2 - Prob. 17RQCh. 2 - Determine whether the statement is true or false....Ch. 2 - Prob. 19RQCh. 2 - Prob. 20RQCh. 2 - Prob. 21RQCh. 2 - Determine whether the statement is true or false....Ch. 2 - Prob. 23RQCh. 2 - Determine whether the statement is true or false....Ch. 2 - Prob. 25RQCh. 2 - Prob. 26RQCh. 2 - Prob. 1RECh. 2 - Prob. 2RECh. 2 - Prob. 3RECh. 2 - Prob. 4RECh. 2 - Prob. 5RECh. 2 - Prob. 6RECh. 2 - Prob. 7RECh. 2 - Prob. 8RECh. 2 - Prob. 9RECh. 2 - Prob. 10RECh. 2 - Prob. 11RECh. 2 - Prob. 12RECh. 2 - Prob. 13RECh. 2 - Prob. 14RECh. 2 - Prob. 15RECh. 2 - Prob. 16RECh. 2 - Prob. 17RECh. 2 - Prob. 18RECh. 2 - Prob. 19RECh. 2 - Prob. 20RECh. 2 - Prob. 21RECh. 2 - Prob. 22RECh. 2 - If 2x 1 f(x) x2 for 0 x 3, find limx1f(x).Ch. 2 - Prob. 24RECh. 2 - Prob. 25RECh. 2 - Prob. 26RECh. 2 - Prob. 27RECh. 2 - Prob. 28RECh. 2 - Prob. 29RECh. 2 - Prob. 30RECh. 2 - Prob. 31RECh. 2 - Prob. 32RECh. 2 - Prob. 33RECh. 2 - Use the Intermediate Value Theorem to show that...Ch. 2 - Prob. 35RECh. 2 - Prob. 36RECh. 2 - Prob. 37RECh. 2 - According to Boyle's Law, if the temperature of a...Ch. 2 - Prob. 39RECh. 2 - Prob. 40RECh. 2 - Prob. 41RECh. 2 - Prob. 42RECh. 2 - Prob. 43RECh. 2 - Prob. 44RECh. 2 - Prob. 45RECh. 2 - Prob. 46RECh. 2 - Prob. 47RECh. 2 - The figure shows the graphs of f, f', and f"....Ch. 2 - Prob. 49RECh. 2 - Prob. 50RECh. 2 - Prob. 51RECh. 2 - Prob. 52RECh. 2 - Prob. 53RECh. 2 - Prob. 54RECh. 2 - Prob. 1PCh. 2 - Find numbers a and b such that limx0ax+b2x=1.Ch. 2 - Prob. 3PCh. 2 - The figure shows a point P on the parabola y = x2...Ch. 2 - Prob. 5PCh. 2 - Prob. 6PCh. 2 - Prob. 7PCh. 2 - Prob. 8PCh. 2 - Prob. 9PCh. 2 - Prob. 10PCh. 2 - Prob. 11PCh. 2 - Prob. 12PCh. 2 - Prob. 13PCh. 2 - Suppose f is a function with the property that |...
Additional Math Textbook Solutions
Find more solutions based on key concepts
1. How much money is Joe earning when he’s 30?
Pathways To Math Literacy (looseleaf)
For each hour of class time, how many hours outside of class are recommended for studying and doing homework?
Elementary Algebra For College Students (10th Edition)
First Derivative Test a. Locale the critical points of f. b. Use the First Derivative Test to locale the local ...
Calculus: Early Transcendentals (2nd Edition)
Silvia wants to mix a 40% apple juice drink with pure apple juice to make 2 L of a juice drink that is 80% appl...
Beginning and Intermediate Algebra
Find all solutions of each equation in the interval .
Precalculus: A Unit Circle Approach (3rd Edition)
Fill in each blank so that the resulting statement is true.
1. The degree of the polynomial function is _____....
Algebra and Trigonometry (6th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- Determine whether the lines L₁ : F(t) = (−2, 3, −1)t + (0,2,-3) and L2 : ƒ(s) = (2, −3, 1)s + (−10, 17, -8) intersect. If they do, find the point of intersection. ● They intersect at the point They are skew lines They are parallel or equalarrow_forwardAnswer questions 2arrow_forwardHow does a fourier transform works?arrow_forward
- Determine the radius of convergence of a power series:12.6.5, 12.6.6, 12.6.7, 12.6.8Hint: Use Theorem12.5.1 and root test, ratio test, integral testarrow_forwardCan you answer this question and give step by step and why and how to get it. Can you write it (numerical method)arrow_forwardCan you answer this question and give step by step and why and how to get it. Can you write it (numerical method)arrow_forward
- There are three options for investing $1150. The first earns 10% compounded annually, the second earns 10% compounded quarterly, and the third earns 10% compounded continuously. Find equations that model each investment growth and use a graphing utility to graph each model in the same viewing window over a 20-year period. Use the graph to determine which investment yields the highest return after 20 years. What are the differences in earnings among the three investment? STEP 1: The formula for compound interest is A = nt = P(1 + − − ) n², where n is the number of compoundings per year, t is the number of years, r is the interest rate, P is the principal, and A is the amount (balance) after t years. For continuous compounding, the formula reduces to A = Pert Find r and n for each model, and use these values to write A in terms of t for each case. Annual Model r=0.10 A = Y(t) = 1150 (1.10)* n = 1 Quarterly Model r = 0.10 n = 4 A = Q(t) = 1150(1.025) 4t Continuous Model r=0.10 A = C(t) =…arrow_forwardUse a graphing utility to find the point of intersection, if any, of the graphs of the functions. Round your result to three decimal places. (Enter NONE in any unused answer blanks.) y = 100e0.01x (x, y) = y = 11,250 ×arrow_forward5. For the function y-x³-3x²-1, use derivatives to: (a) determine the intervals of increase and decrease. (b) determine the local (relative) maxima and minima. (e) determine the intervals of concavity. (d) determine the points of inflection. (e) sketch the graph with the above information indicated on the graph.arrow_forward
- Can you solve this 2 question numerical methodarrow_forward1. Estimate the area under the graph of f(x)-25-x from x=0 to x=5 using 5 approximating rectangles Using: (A) right endpoints. (B) left endpoints.arrow_forward9. Use fundamental theorem of calculus to find the derivative d a) *dt sin(x) b)(x)√1-2 dtarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendentals
Calculus
ISBN:9781285741550
Author:James Stewart
Publisher:Cengage Learning
Thomas' Calculus (14th Edition)
Calculus
ISBN:9780134438986
Author:Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:9780134763644
Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:PEARSON
Calculus: Early Transcendentals
Calculus
ISBN:9781319050740
Author:Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:W. H. Freeman
Calculus: Early Transcendental Functions
Calculus
ISBN:9781337552516
Author:Ron Larson, Bruce H. Edwards
Publisher:Cengage Learning
Derivatives of Trigonometric Functions - Product Rule Quotient & Chain Rule - Calculus Tutorial; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=_niP0JaOgHY;License: Standard YouTube License, CC-BY