Concept explainers
Videos
(A)
To express the dimensions in inches upto 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given,the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
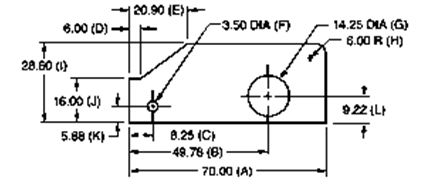
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(B)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
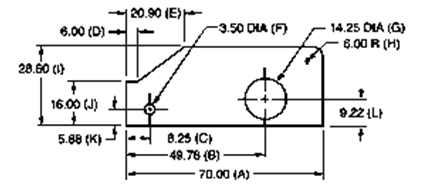
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(C)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
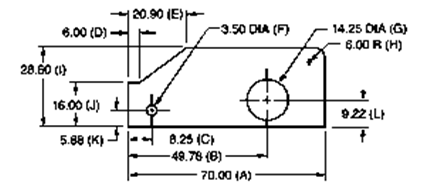
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(D)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
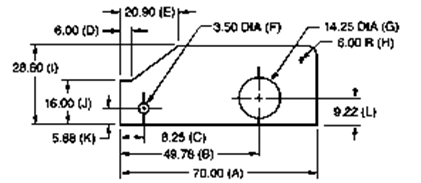
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(E)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
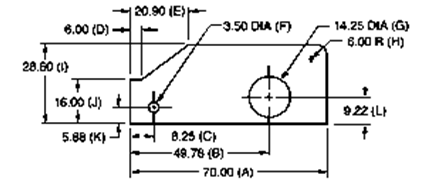
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(F)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
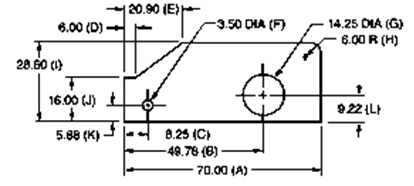
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(G)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
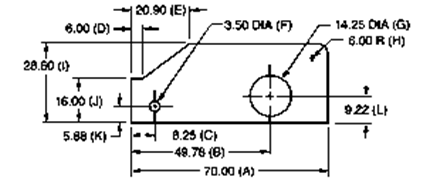
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(H)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
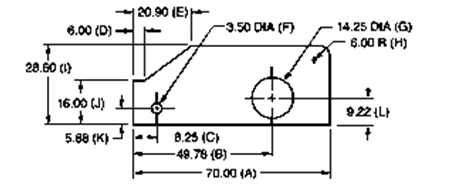
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(I)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
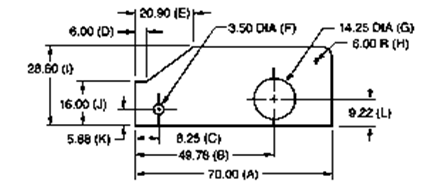
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(J)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
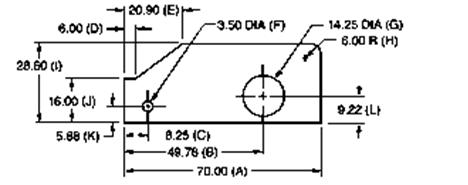
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(K)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
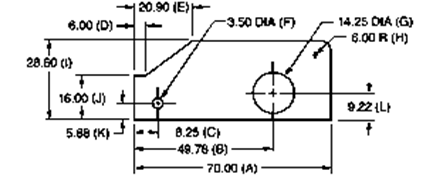
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
(L)
To express the dimensions in inches up to 3 decimal places.
Answer
The dimension
Explanation of Solution
Given Information:
Given, the part shown in figure is to be made in a machine shop using decimal-inch machinery and tools. All dimensions are in millimeters.
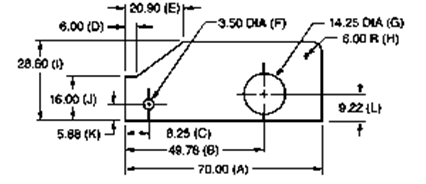
Calculation:
To express a unit in one system as an equivalent unit in the other system, either use unity fractions or multiply the given measurements by the appropriate conversion factor in the metric-Customary Linear Equivalent Table
As we know that,
Converting
Hence, the dimension
Want to see more full solutions like this?
Chapter 27 Solutions
Mathematics For Machine Technology
- 2. You manage a chemical company with 2 warehouses. The following quantities of Important Chemical A have arrived from an international supplier at 3 different ports: Chemical Available (L) Port 1. 400 Port 2 110 Port 3 100 The following amounts of Important Chemical A are required at your warehouses: Warehouse 1 Warehouse 2 Chemical Required (L) 380 230 The cost in £ to ship 1L of chemical from each port to each warehouse is as follows: Warehouse 1 Warehouse 2 Port 1 £10 £45 Port 2 £20 £28 Port 3 £13 £11 (a) You want to know how to send these shipments as cheaply as possible. For- mulate this as a linear program (you do not need to formulate it in standard inequality form). (b) Suppose now that all is as in the previous question but that only 320L of Important Chemical A are now required at Warehouse 1. Any excess chemical can be transported to either Warehouse 1 or 2 for storage, in which case the company must pay only the relevant transportation costs, or can be disposed of at the…arrow_forwardchoose true options in these from given question a) always full and always crossing. b) always full and sometimes crossing. c) always full and never crossing. d) sometimes full and always crossing. e) sometimes full and sometimes crossing. f) sometimes full and never crossing. g) never full and always crossing. h) never full and sometimes crossing. i) never full and never crossing.arrow_forwardAt a Noodles & Company restaurant, the probability that a customer will order a nonalcoholic beverage is 0.49. Find the probability that in a sample of 13 customers, at least 7 will order a nonalcoholic beveragearrow_forward
- 10. In the general single period market model with = {W1, W2, W3}, one risky asset, S, and a money market account, we have So = 4 for the risky asset. Moreover, the effective rate of interest on the money market account is 5% and at time t = 1 we have W1 W2 W3 S₁ 100 50 40 21 21 21 (a) Calculate all risk-neutral probability measures for this model. [4 Marks] (b) State if the model is arbitrage-free. Give a brief reason for your answer. [2 Marks] (c) A large bank has designed an investment product with payoff X at time t = 1. Given W₁ W2 W3 X 0 1 1.5 show that X is an attainable contingent claim. [4 marks]arrow_forwardadded 2 imagesarrow_forwardA movie studio wishes to determine the relationship between the revenue generated from the streaming of comedies and the revenue generated from the theatrical release of such movies. The studio has the following bivariate data from a sample of fifteen comedies released over the past five years. These data give the revenue x from theatrical release (in millions of dollars) and the revenue y from streaming (in millions of dollars) for each of the fifteen movies. The data are displayed in the Figure 1 scatter plot. Theater revenue, x Streaming revenue, y (in millions of (in millions of dollars) dollars) 13.2 10.3 62.6 10.4 20.8 5.1 36.7 13.3 44.6 7.2 65.9 10.3 49.4 15.7 31.5 4.5 14.6 2.5 26.0 8.8 28.1 11.5 26.1 7.7 28.2 2.8 60.7 16.4 6.7 1.9 Streaming revenue (in millions of dollars) 18+ 16+ 14 12+ xx 10+ 8+ 6+ 2- 0 10 20 30 40 50 60 70 Theater revenue (in millions of dollars) Figure 1 Send data to calculator Send data to Excel The least-squares regression line for these data has a slope…arrow_forward
- help on this, results givenarrow_forwarda) Suppose that we are carrying out the 1-phase simplex algorithm on a linear program in standard inequality form (with 3 variables and 4 constraints) and suppose that we have reached a point where we have obtained the following tableau. Apply one more pivot operation, indicating the highlighted row and column and the row operations you carry out. What can you conclude from your updated tableau? x1 x2 x3 81 82 83 84 81 -2 0 1 1 0 0 0 3 82 3 0 -2 0 1 2 0 6 12 1 1 -3 0 0 1 0 2 84 -3 0 2 0 0 -1 1 4 -2 -2 0 11 0 0-4 0 -8arrow_forwardb) Solve the following linear program using the 2-phase simplex algorithm. You should give the initial tableau, and each further tableau produced during the execution of the algorithm. If the program has an optimal solution, give this solution and state its objective value. If it does not have an optimal solution, say why. maximize ₁ - 2x2+x34x4 subject to 2x1+x22x3x41, 5x1 + x2-x3-×4 ≤ −1, 2x1+x2-x3-34 2, 1, 2, 3, 40.arrow_forward
- 9. An elementary single period market model contains a risk-free asset with interest rate r = 5% and a risky asset S which has price 30 at time t = 0 and will have either price 10 or 60 at time t = 1. Find a replicating strategy for a contingent claim with payoff h(S₁) = max(20 - S₁, 0) + max(S₁ — 50, 0). Total [8 Marks]arrow_forward8. An elementary single period market model has a risky asset with price So = 20 at the beginning and a money market account with interest rate r = 0.04 compounded only once at the end of the investment period. = = In market model A, S₁ 10 with 15% probability and S₁ 21 with 85% probability. In market model B, S₁ = 25 with 10% probability and S₁ = 30 with 90% probability. For each market model A, B, determine if the model is arbitrage-free. If not, construct an arbitrage. Total [9 Marks]arrow_forwardb) Solve the following linear program using the 2-phase simplex algorithm. You should give the initial tableau, and each further tableau produced during the execution of the algorithm. If the program has an optimal solution, give this solution and state its objective value. If it does not have an optimal solution, say why. maximize ₁ - 2x2+x34x4 subject to 2x1+x22x3x41, 5x1 + x2-x3-×4 ≤ −1, 2x1+x2-x3-34 2, 1, 2, 3, 40.arrow_forward
- Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill 
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,