
The following nonlinear, parasitic ODE was suggested by Hornbeck (1975):
If the initial condition is
(a) Analytically
(b) Using the fourth-order RK method with a constant step size of 0.03125.
(c) Using the MATLAB function ode45.
(d) Using the MATLAB function ode23s.
(e) Using the MATLAB function ode23tb.
Present your results in graphical form.
(a)
To calculate: The analytical solution of the nonlinear, parasitic ordinary differential equation,
Answer to Problem 27P
Solution:
The analytical solution of the differential equation is
Explanation of Solution
Given Information:
A nonlinear, parasitic ordinary differential equation,
Formula used:
The general linear differential equation is,
Calculation:
Consider the nonlinear ordinary differential equation,
Rearrange the above differential equationto get,
Now, compare the above differential equation with the general linear differential equation
Thus,
Now, find the integrating factor (I. F.) as shown below,
Therefore, the solution of the linear differential equation is given as,
Substitute the value of integrating factor (I. F.) in above equation,
Now, integrate the right-hand-side of the above equation,
Solve further, to get
Thus, the solution is
Now, to determine the constant c, use the initial condition
Substitute
Therefore,
Hence, the solution of the differential equation is
(b)
To calculate: Thesolution of the nonlinear, parasitic ordinary differential equation,
Answer to Problem 27P
Solution:
The graph of the solution of the differential equation is,
Explanation of Solution
Given Information:
A nonlinear, parasitic ordinary differential equation,
Calculation:
Consider the nonlinear ordinary differential equation,
The VBA code to solve the above differential equation with fourth-order RK method with a constant step size of 0.03125 is given below,
The output given below is obtained in the Excel after the execution of the above code:
| 4th order RK | ||
| t | y1 | |
| 0 | 0.08 | 0.08 |
| 0.03125 | 0.093476 | 0.093477 |
| 0.0625 | 0.108906 | 0.108906 |
| 0.09375 | 0.126289 | 0.126289 |
| 0.125 | 0.145625 | 0.145625 |
| 0.15625 | 0.166914 | 0.166914 |
| 0.1875 | 0.190156 | 0.190156 |
| 0.21875 | 0.215351 | 0.215352 |
| 0.25 | 0.242499 | 0.2425 |
| 0.28125 | 0.2716 | 0.271602 |
| 0.3125 | 0.302655 | 0.302656 |
| 0.34375 | 0.335662 | 0.335664 |
| 0.375 | 0.370622 | 0.370625 |
| 0.40625 | 0.407536 | 0.407539 |
| 0.4375 | 0.446403 | 0.446406 |
| 0.46875 | 0.487222 | 0.487227 |
| 0.5 | 0.529995 | 0.53 |
| 0.53125 | 0.57472 | 0.574727 |
| 0.5625 | 0.621399 | 0.621406 |
| 0.59375 | 0.670031 | 0.670039 |
| 0.625 | 0.720615 | 0.720625 |
| 0.65625 | 0.773152 | 0.773164 |
| 0.6875 | 0.827642 | 0.827656 |
| 0.71875 | 0.884085 | 0.884102 |
| 0.75 | 0.942481 | 0.9425 |
| 0.78125 | 1.002829 | 1.002852 |
| 0.8125 | 1.06513 | 1.065156 |
| 0.84375 | 1.129383 | 1.129414 |
| 0.875 | 1.195589 | 1.195625 |
| 0.90625 | 1.263747 | 1.263789 |
| 0.9375 | 1.333857 | 1.333906 |
| 0.96875 | 1.405919 | 1.405977 |
| 1 | 1.479932 | 1.48 |
| 1.03125 | 1.555897 | 1.555977 |
| 1.0625 | 1.633814 | 1.633906 |
| 1.09375 | 1.713681 | 1.713789 |
| 1.125 | 1.795498 | 1.795625 |
| 1.15625 | 1.879266 | 1.879414 |
| 1.1875 | 1.964983 | 1.965156 |
| 1.21875 | 2.052649 | 2.052852 |
| 1.25 | 2.142263 | 2.1425 |
| 1.28125 | 2.233824 | 2.234102 |
| 1.3125 | 2.327332 | 2.327656 |
| 1.34375 | 2.422785 | 2.423164 |
| 1.375 | 2.520181 | 2.520625 |
| 1.40625 | 2.61952 | 2.620039 |
| 1.4375 | 2.7208 | 2.721406 |
| 1.46875 | 2.824017 | 2.824727 |
| 1.5 | 2.929171 | 2.93 |
| 1.53125 | 3.036257 | 3.037227 |
| 1.5625 | 3.145273 | 3.146406 |
| 1.59375 | 3.256214 | 3.257539 |
| 1.625 | 3.369075 | 3.370625 |
| 1.65625 | 3.483852 | 3.485664 |
| 1.6875 | 3.600538 | 3.602656 |
| 1.71875 | 3.719125 | 3.721602 |
| 1.75 | 3.839605 | 3.8425 |
| 1.78125 | 3.961966 | 3.965352 |
| 1.8125 | 4.086198 | 4.090156 |
| 1.84375 | 4.212287 | 4.216914 |
| 1.875 | 4.340215 | 4.345625 |
| 1.90625 | 4.469964 | 4.476289 |
| 1.9375 | 4.601512 | 4.608906 |
| 1.96875 | 4.734831 | 4.743477 |
| 2 | 4.869893 | 4.88 |
| 2.03125 | 5.00666 | 5.018477 |
| 2.0625 | 5.145091 | 5.158906 |
| 2.09375 | 5.285137 | 5.301289 |
| 2.125 | 5.426742 | 5.445625 |
| 2.15625 | 5.569837 | 5.591914 |
| 2.1875 | 5.714346 | 5.740156 |
| 2.21875 | 5.860176 | 5.890352 |
| 2.25 | 6.007221 | 6.0425 |
| 2.28125 | 6.155356 | 6.196602 |
| 2.3125 | 6.304436 | 6.352656 |
| 2.34375 | 6.454288 | 6.510664 |
| 2.375 | 6.604715 | 6.670625 |
| 2.40625 | 6.755482 | 6.832539 |
| 2.4375 | 6.906318 | 6.996406 |
| 2.46875 | 7.056903 | 7.162227 |
| 2.5 | 7.206864 | 7.33 |
| 2.53125 | 7.355766 | 7.499727 |
| 2.5625 | 7.503099 | 7.671406 |
| 2.59375 | 7.648268 | 7.845039 |
| 2.625 | 7.790577 | 8.020625 |
| 2.65625 | 7.92921 | 8.198164 |
| 2.6875 | 8.063218 | 8.377656 |
| 2.71875 | 8.191486 | 8.559102 |
| 2.75 | 8.312714 | 8.7425 |
| 2.78125 | 8.425381 | 8.927852 |
| 2.8125 | 8.527709 | 9.115156 |
| 2.84375 | 8.617619 | 9.304414 |
| 2.875 | 8.69268 | 9.495625 |
| 2.90625 | 8.750052 | 9.688789 |
| 2.9375 | 8.786412 | 9.883906 |
| 2.96875 | 8.797877 | 10.08098 |
| 3 | 8.779905 | 10.28 |
| 3.03125 | 8.727189 | 10.48098 |
| 3.0625 | 8.633523 | 10.68391 |
| 3.09375 | 8.491649 | 10.88879 |
| 3.125 | 8.293087 | 11.09563 |
| 3.15625 | 8.027917 | 11.30441 |
| 3.1875 | 7.684546 | 11.51516 |
| 3.21875 | 7.249417 | 11.72785 |
| 3.25 | 6.706683 | 11.9425 |
| 3.28125 | 6.037815 | 12.1591 |
| 3.3125 | 5.221152 | 12.37766 |
| 3.34375 | 4.231369 | 12.59816 |
| 3.375 | 3.038857 | 12.82063 |
| 3.40625 | 1.609002 | 13.04504 |
| 3.4375 | -0.09867 | 13.27141 |
| 3.46875 | -2.13146 | 13.49973 |
| 3.5 | -4.5447 | 13.73 |
| 3.53125 | -7.40305 | 13.96223 |
| 3.5625 | -10.7821 | 14.19641 |
| 3.59375 | -14.7703 | 14.43254 |
| 3.625 | -19.4709 | 14.67063 |
| 3.65625 | -25.0048 | 14.91066 |
| 3.6875 | -31.5132 | 15.15266 |
| 3.71875 | -39.1613 | 15.3966 |
| 3.75 | -48.1421 | 15.6425 |
| 3.78125 | -58.6814 | 15.89035 |
| 3.8125 | -71.043 | 16.14016 |
| 3.84375 | -85.5354 | 16.39191 |
| 3.875 | -102.519 | 16.64563 |
| 3.90625 | -122.417 | 16.90129 |
| 3.9375 | -145.72 | 17.15891 |
| 3.96875 | -173.006 | 17.41848 |
| 4 | -204.949 | 17.68 |
| 4.03125 | -242.336 | 17.94348 |
| 4.0625 | -286.088 | 18.20891 |
| 4.09375 | -337.283 | 18.47629 |
| 4.125 | -397.179 | 18.74563 |
| 4.15625 | -467.248 | 19.01691 |
| 4.1875 | -549.211 | 19.29016 |
| 4.21875 | -645.079 | 19.56535 |
| 4.25 | -757.205 | 19.8425 |
| 4.28125 | -888.338 | 20.1216 |
| 4.3125 | -1041.69 | 20.40266 |
| 4.34375 | -1221.03 | 20.68566 |
| 4.375 | -1430.74 | 20.97063 |
| 4.40625 | -1675.96 | 21.25754 |
| 4.4375 | -1962.71 | 21.54641 |
| 4.46875 | -2297.99 | 21.83723 |
| 4.5 | -2690.02 | 22.13 |
| 4.53125 | -3148.39 | 22.42473 |
| 4.5625 | -3684.34 | 22.72141 |
| 4.59375 | -4310.97 | 23.02004 |
| 4.625 | -5043.62 | 23.32063 |
| 4.65625 | -5900.23 | 23.62316 |
| 4.6875 | -6901.75 | 23.92766 |
| 4.71875 | -8072.7 | 24.2341 |
| 4.75 | -9441.73 | 24.5425 |
| 4.78125 | -11042.3 | 24.85285 |
| 4.8125 | -12913.7 | 25.16516 |
| 4.84375 | -15101.5 | 25.47941 |
| 4.875 | -17659.5 | 25.79563 |
| 4.90625 | -20650.1 | 26.11379 |
| 4.9375 | -24146.4 | 26.43391 |
| 4.96875 | -28234.2 | 26.75598 |
Now, plot the following chart using the data obtained in Excel.
In the above plot, the series1 represent the numerical solution whereas the series2 represent the exact solution.
(c)
The solution of the nonlinear, parasitic ordinary differential equation,
Answer to Problem 27P
Solution:
The graph of the solution of the differential equation is,
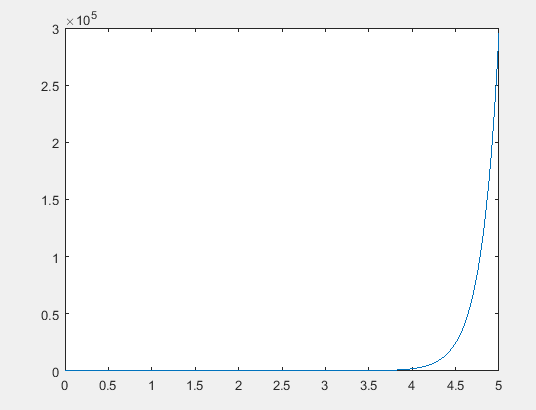
Explanation of Solution
Given Information:
A nonlinear, parasitic ordinary differential equation,
Consider the nonlinear ordinary differential equation,
Use the MATLAB function
Write the code given below in MATLAB editor window and save it.
function
Now, write the following code in MATLAB command window

Following graph is obtained after the execution of the above MATLAB code.

(d)
The solution of the nonlinear, parasitic ordinary differential equation,
Answer to Problem 27P
Solution:
The graph of the solution of the differential equation is,
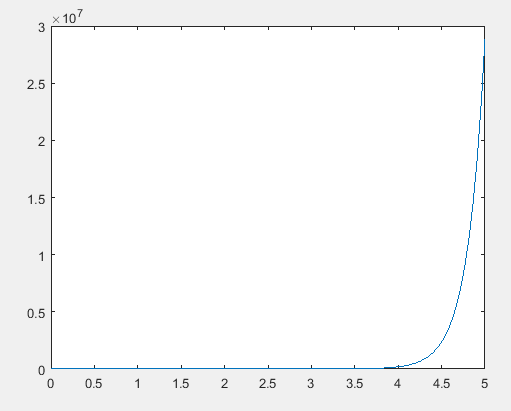
Explanation of Solution
Given Information:
A nonlinear, parasitic ordinary differential equation,
Consider the nonlinear ordinary differential equation,
Use the MATLAB function
Write the code given below in MATLAB editor window and save it.
function
Now, write the following code in MATLAB command window

Following graph is obtained after the execution of the above MATLAB code.

(e)
The solution of the nonlinear, parasitic ordinary differential equation,
Answer to Problem 27P
Solution:
The graph of the solution of the differential equation is,
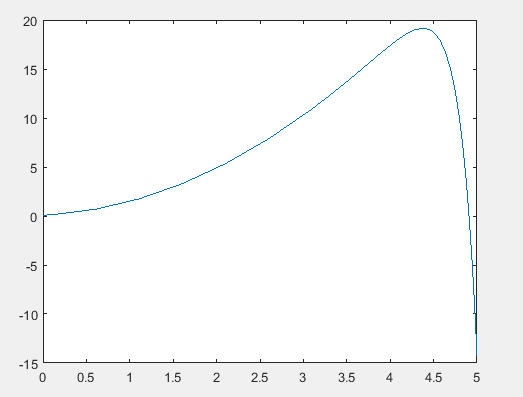
Explanation of Solution
Given Information:
A nonlinear, parasitic ordinary differential equation,
Consider the nonlinear ordinary differential equation,
Use the MATLAB function
Write the code given below in MATLAB editor window and save it.
function
Now, write the following code in MATLAB command window

Following graph is obtained after the execution of the above MATLAB code.

Want to see more full solutions like this?
Chapter 27 Solutions
EBK NUMERICAL METHODS FOR ENGINEERS
Additional Engineering Textbook Solutions
Introductory Statistics
Calculus: Early Transcendentals (2nd Edition)
Precalculus: A Unit Circle Approach (3rd Edition)
A First Course in Probability (10th Edition)
Precalculus: Mathematics for Calculus (Standalone Book)
Elementary Statistics ( 3rd International Edition ) Isbn:9781260092561
- 18) Find all the complex cube roots of -2i. Leave your answers in polar form with the argument in degrees.arrow_forward9) Write an equation for the hyperbola. 2+ -6-5-4-3-2 -2- -4- -5+ + 23 45 6xarrow_forward8) Find an equation for the hyperbola with vertices at vertices at (±7, 0) and foci at (±9, 0).arrow_forward
- 3) Find an equation for the parabola with a vertex at (1, 2) and focus at (1, 4). A) (x - 1)² = 8(y-2) C) (x - 1)² = -8(y - 2) B) (y - 2)² = -12(x − 1) - D) (y - 2)² = 12(x-1)arrow_forward2) Graph the equation. y² = 12x 5 10 -10 -5 5 + 10 xarrow_forward15) Solve the triangles if possible. B = 11°, b = 15.5, a = 40.62arrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning


