Chapter 26, Problem 1P

(a)

Program Plan Intro

To explain the determining of the maximum flow in a network with edge and vertex capacities can be reduced to an ordinary maximum-flow problem on a flow network of comparable size.

Explanation of Solution

Given Information:The network is given below:

  

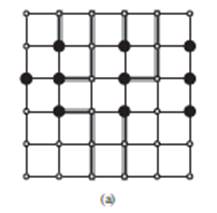
Explanation:

The constraints can be covered by splitting the vertex into two then the edge in between two vertices of the network will be the vertex capacity for the graph.

The maximum flow of the network is determined by consider the path so that the graph has maximum capacity.

The steps to determine the maximum-flow is given below:

Step 1: Split the vertex into 2 and formed new network of vertices u and v.

Step 2: The splitting results the new vertices of new network is $\{0,1\}\times V$ so it has some edges $(v,u)$ .

Step 3: Check the graph for edge $(v,u)$ then there exist an edge in the original network as $1\times V$ and $0\times u$

Step 4: The new network consists of edge as $(u,v)$ such that there are total of $2\left|V\right|$ and total of $(V+E)$ edge comparing to original network.

Step 5: To obtain the maximum flow consider the v as it goes from $(0\times v)$ and $(1\times v)$ .

Thus, in this way the new flow network is formed having maximum flow.

(b)

Program Plan Intro

To describes an efficient algorithm to solve the escape problem.

Explanation of Solution

Given Information:The figure with no escape grid is given below:

  

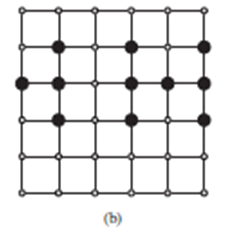
Explanation:

The escape problem can be solved as follows:

Step 1: Built a flow network with unit capacity by considering vertex constraints.

Step 2: Consider the above figure select the unit edge that intersecting the grid lines of adjacent grid.

Step 3: The available network has bidirectional edges with unit capacity so put a unit capacity corresponding to edges going to source s to other vertices.

Step 4: The capacity of the edges is unity so all the augmented paths will be unity.

Step 5: The augmented path defines the path to escape that is escape path is equal to m , where m is vertex disjoint path.

Step 6: Checks the value of maximum flow if it is less than the m then it is not the required escape path.

The path obtained from the above algorithm can be crossed verified by the vertex disjoint path it satisfy the equality then it is required escape path otherwise the flow network cannot have any escape path.