
Concept explainers
(A)
The dimension of A in inches.
Answer to Problem 16A
The dimension of A in inches is
Explanation of Solution
Given information:
The schematic diagram of the system is shown below.
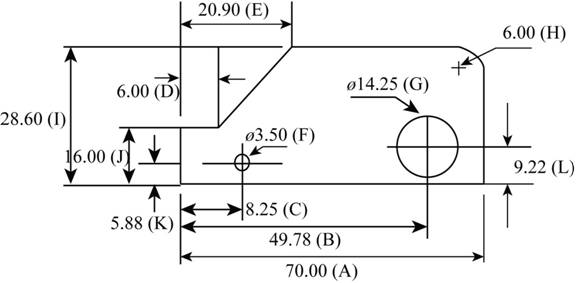
Figure-(1)
Write the expression for the dimension in inches.
Here, the dimension in inches is
Calculation:
Substitute
Conclusion:
The dimension of A in inches is
(B)
The dimension of B in inches.
Answer to Problem 16A
The dimension of B in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of B in inches is
(C)
The dimension of C in inches.
Answer to Problem 16A
The dimension of C in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of C in inches is
(D)
The dimension of D in inches.
Answer to Problem 16A
The dimension of D in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of D in inches is
(E)
The dimension of E in inches.
Answer to Problem 16A
The dimension of E in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of E in inches is
(F)
The dimension of F in inches.
Answer to Problem 16A
The dimension of F in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of F in inches is
(G)
The dimension of G in inches.
Answer to Problem 16A
The dimension of G in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of G in inches is
(H)
The dimension of H in inches.
Answer to Problem 16A
The dimension of H in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of H in inches is
(I)
The dimension of I in inches.
Answer to Problem 16A
The dimension of I in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of I in inches is
(J)
The dimension of J in inches.
Answer to Problem 16A
The dimension of J in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of J in inches is
(K)
The dimension of K in inches.
Answer to Problem 16A
The dimension of K in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of K in inches is
(L)
The dimension of L in inches.
Answer to Problem 16A
The dimension of L in inches is
Explanation of Solution
Substitute
Conclusion:
The dimension of L in inches is
Want to see more full solutions like this?
Chapter 25 Solutions
EBK MATHEMATICS FOR MACHINE TECHNOLOGY
- 5. Find the solution to each of the following by using an appropriate formula developed in the lecture slides: (a) + 3y = 2, y(0) = 4; (b) dy - 7y = 7, y(0) = 7; (c) 3d+6y= 5, y(0) = 0arrow_forward1. Evaluate the following improper integrals: (a) fe-rt dt; (b) fert dt; (c) fi da dxarrow_forward8. Given the rate of net investment I(t) = 9t¹/2, find the level of capital formation in (i) 16 years and (ii) between the 4th and the 8th years.arrow_forward
- 9. If the marginal revenue function of a firm in the production of output is MR = 40 - 10q² where q is the level of output, and total revenue is 120 at 3 units of output, find the total revenue function. [Hints: TR = √ MRdq]arrow_forward6. Solve the following first-order linear differential equations; if an initial condition is given, definitize the arbitrary constant: (a) 2 + 12y + 2et = 0, y(0) = /; (b) dy+y=tarrow_forward4. Let A = {a, b, c, d, e, f}, B = {e, f, g, h} and C = {a, e, h,i}. Let U = {a, b, c, d, e, f, g, h, i, j, k}. • Draw a Venn Diagram to describe the relationships between these sets Find (AB) NC • Find (AC) UB Find AUBUC • Find (BC) N (A - C)arrow_forward
- 7. A consumer lives on an island where she produces two goods x and y according to the production possibility frontier x² + y² < 200 and she consumes all the goods. Her utility function is U(x, y) = x y³. She faces an environmental constraint on her total output of both goods. The environmental constraint is given by x + y ≤20. • (a) Write down the consumer's optimization problem. (b) Write out the Kuhn-Tucker first order conditions. (c) Find the consumer's optimal consumption bundle (x*, y*).arrow_forward3. Answer the following questions: (a) Given the marginal propensity to import M'(Y) = 0.1 and the information that M = 20 when Y = 0, find the import function M(Y). (b) Given a continuous income stream at the constant rate of $1,000 per year, what will be the present value II if the income stream terminates after exactly 3 years and the discount rate is 0.04? (c) What is the present value of a perpetual cash flow of $2,460 per year, discounted at r = 8%?arrow_forward5. Let A and B be arbitrary sets. Prove AnB = AUB.arrow_forward
- 2. Answer the following questions: (a) Given the marginal-revenue function R'(Q) = 28Q - €0.3Q, find the total-revenue function R(Q). What initial condition can you introduce to definitize the constant of integration? = (b) Given the marginal propensity to consume C'(Y) 0.80.1Y-1/2 and the information that C = Y when Y = 100, find the consumption function C(Y).arrow_forward7. Let X, A, and B be arbitrary sets such that ACX and BC X. Prove AUB CX.arrow_forward1. Write out the following sets as a list of elements. If necessary you may use ... in your description. {x EZ: |x|< 10 A x < 0} {x ЄN: x ≤ 20 A x = 2y for some y = N} {n EN: 3 | n^ 1 < n < 20} {y Є Z: y² <0}arrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,  Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill




