Elementary Geometry For College Students, 7e
7th Edition
ISBN: 9781337614085
Author: Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher: Cengage,
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 2.4, Problem 21E
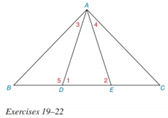
| Given: |
|
Find: |
|
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
4) Find a proposition with three variables p, q, and r that is never true.
5) Determine whether this proposition is a tautology using propositional
equivalence and laws of logic: ((p
(bv (bL ←
→¬p
[1
6) Explain why the negation of "Some students in my class use e-mail” is not "Some
students in my class do not use e-mail".
Milgram lemma
B) Consider
Show that
-Au= f in a
u=0 on on
llu-ulls Chllullz
02 Prove that
Where
||ul| = a(u, u) = vu. Vu dx + fonu.u ds
Q3: Let V = H' (2),
a(u,v) =
CR, a(u,v) = (f,v)
where
Vu. Vv dx + Ja cuv dx and ||u|=||||
Show that a(u, v) is V-ellipiticly and continuity.
7) Is the following statement True or False: AU BUA = Ā. Justify your answer.
8) Suppose g: A → B and f: B → C where A =
{2,3,6,8}, and g and f are defined by g =
{a, b, c, d}, B = {1,2,3}, C =
{(a,2), (b,1), (c, 3), (d, 2)} and
f = {(1,8),(2,3), (3,2)}. Find fog, gof and f−¹.
9) Verify that a₁ = 7(3") -π is a solution to the recurrence relation an =
an
4an-1 -3an-2
Chapter 2 Solutions
Elementary Geometry For College Students, 7e
Ch. 2.1 - Prob. 1ECh. 2.1 - For Exercises 1 to 4, lm with transversal v. See...Ch. 2.1 - For Exercises 1 to 4, lm with transversal v. See...Ch. 2.1 - For Exercises 1 to 4, lm with transversal v. See...Ch. 2.1 - Use drawings, as needed, to answer each question....Ch. 2.1 - Use drawings, as needed, to answer each question....Ch. 2.1 - Use drawings, as needed, to answer each question....Ch. 2.1 - Use drawings, as needed, to answer each question....Ch. 2.1 - Use drawings, as needed, to answer each question....Ch. 2.1 - Use drawings, as needed, to answer each question....
Ch. 2.1 - Use drawings, as needed, to answer each question....Ch. 2.1 - In Euclidean geometry, how many lines can be drawn...Ch. 2.1 - Lines r and s are cut by the transversal t. Which...Ch. 2.1 - ADBC, ABDC, and mA=92. Find: a mB b mC c mDCh. 2.1 - lm, with transversal t and OQ bisects MNO. If...Ch. 2.1 - Given: lm Transversal t m1=4x+2 m6=4x2 Find: x and...Ch. 2.1 - Given: mn Transversal k m3=x23x m6=(x+4)(x5) Find:...Ch. 2.1 - Given: mn Transversal k m1=5x+y m2=3x+y m8=3x+5y...Ch. 2.1 - Given: mn Transversal k m3=6x+y m5=8x+2y m6=4x+7y...Ch. 2.1 - In the three-dimensional figure, CAAB and BEAB....Ch. 2.1 - Given: lmand34 Prove: 14 See figure below. PROOF...Ch. 2.1 - Given: lmandmn Prove: 14 PROOF Statements Reasons...Ch. 2.1 - Given: CEDF Transversal AB CX bisects ACE DE...Ch. 2.1 - Given: CEDF Transversal AB DE bisects CDF Prove:...Ch. 2.1 - Given: rs Transversal t 1 is a right Prove: 2 is...Ch. 2.1 - Given: ABDEmBAC=42mEDC=54 Find: mACD HINT: There...Ch. 2.1 - Given: ABDEmBAC+mCDE=93 Find: mACD See Hint in...Ch. 2.1 - Prob. 28ECh. 2.1 - In triangle ABC, line t is drawn through vertex A...Ch. 2.1 - In Exercises 30 to 32, write a formal proof of...Ch. 2.1 - In Exercises 30 to 32, write a formal proof of...Ch. 2.1 - In Exercises 30 to 32, write a formal proof of...Ch. 2.1 - Suppose that two lines are cut by a transversal in...Ch. 2.1 - Prob. 34ECh. 2.1 - Prob. 35ECh. 2.1 - Given: Triangle MNQ with obtuse MNQ Construct:...Ch. 2.1 - Prob. 37ECh. 2.1 - Given: A line m and a point T not on m Suppose...Ch. 2.1 - Prob. 39ECh. 2.2 - Note: Exercises preceded by an asterisk are of a...Ch. 2.2 - Note: Exercises preceded by an asterisk are of a...Ch. 2.2 - Prob. 3ECh. 2.2 - Note: Exercises preceded by an asterisk are of a...Ch. 2.2 - In Exercises 5 to 10, draw a conclusion where...Ch. 2.2 - Prob. 6ECh. 2.2 - Prob. 7ECh. 2.2 - Prob. 8ECh. 2.2 - Prob. 9ECh. 2.2 - In Exercises 5 to 10, draw a conclusion where...Ch. 2.2 - Which of the following statements would you prove...Ch. 2.2 - Which of the following statements would you prove...Ch. 2.2 - Prob. 13ECh. 2.2 - In Exercises 13 to 16, write the first statement...Ch. 2.2 - In Exercises 13 to 16, write the first statement...Ch. 2.2 - In Exercises 13 to 16, write the first statement...Ch. 2.2 - Prob. 17ECh. 2.2 - Prob. 18ECh. 2.2 - Prob. 19ECh. 2.2 - Prob. 20ECh. 2.2 - Prob. 21ECh. 2.2 - In Exercise 21 and 22, state a conclusion for the...Ch. 2.2 - A periscope uses an indirect method of...Ch. 2.2 - Some stores use an indirect method of observation....Ch. 2.2 - Prob. 25ECh. 2.2 - Prob. 26ECh. 2.2 - Prob. 27ECh. 2.2 - Prob. 28ECh. 2.2 - Prob. 29ECh. 2.2 - Prob. 30ECh. 2.2 - In Exercises 25 to 36, give the indirect proof for...Ch. 2.2 - Prob. 32ECh. 2.2 - Prob. 33ECh. 2.2 - Prob. 34ECh. 2.2 - Prob. 35ECh. 2.2 - Prob. 36ECh. 2.3 - In Exercises 1 to 6, l and m are cut by...Ch. 2.3 - In Exercises 1 to 6, l and m are cut by...Ch. 2.3 - Prob. 3ECh. 2.3 - In Exercises 1 to 6, l and m are cut by...Ch. 2.3 - In Exercises 1 to 6, l and m are cut by...Ch. 2.3 - In Exercises 1 to 6, l and m are cut by...Ch. 2.3 - In Exercise 7 to 16, name the lines if any that...Ch. 2.3 - In Exercise 7 to 16, name the lines if any that...Ch. 2.3 - In Exercise 7 to 16, name the lines if any that...Ch. 2.3 - In Exercise 7 to 16, name the lines if any that...Ch. 2.3 - In Exercise 7 to 16, name the lines if any that...Ch. 2.3 - In Exercise 7 to 16, name the lines if any that...Ch. 2.3 - In Exercise 7 to 16, name the lines if any that...Ch. 2.3 - In Exercise 7 to 16, name the lines if any that...Ch. 2.3 - In Exercise 7 to 16, name the lines if any that...Ch. 2.3 - In Exercise 7 to 16, name the lines if any that...Ch. 2.3 - In Exercise 17 and 18 , complete each proof by...Ch. 2.3 - In Exercises 17 and 18, complete each proof by...Ch. 2.3 - In Exercises 19 to 22, complete the proof. Given:...Ch. 2.3 - In Exercise 19 to 22 complete the proof. Given: 1 ...Ch. 2.3 - In Exercise 19 to 22 complete the proof. Given: DE...Ch. 2.3 - In Exercise 19 to 22 complete the proof. Given: XY...Ch. 2.3 - In Exercise 23 to 30, determine the value of x so...Ch. 2.3 - In Exercise 23 to 30, determine the value of x so...Ch. 2.3 - In Exercise 23 to 30, determine the value of x so...Ch. 2.3 - In Exercise 23 to 30, determine the value of x so...Ch. 2.3 - In Exercise 23 to 30, determine the value of x so...Ch. 2.3 - In Exercise 23 to 30, determine the value of x so...Ch. 2.3 - In Exercise 23 to 30, determine the value of x so...Ch. 2.3 - In Exercise 23 to 30, determine the value of x so...Ch. 2.3 - Prob. 31ECh. 2.3 - In Exercises 31 to 33, give a formal proof for...Ch. 2.3 - Prob. 33ECh. 2.3 - Prob. 34ECh. 2.3 - Prob. 35ECh. 2.3 - Prob. 36ECh. 2.3 - Prob. 37ECh. 2.3 - Given: m2+m3=90 BE bisects ABC CE bisects BCD...Ch. 2.3 - Prob. 39ECh. 2.4 - In Exercise 1 to 4, refer to ABC . On the basis of...Ch. 2.4 - In Exercise 1 to 4, refer to ABC . On the basis of...Ch. 2.4 - In Exercise 1 to 4, refer to ABC . On the basis of...Ch. 2.4 - In Exercise 1 to 4, refer to ABC . On the basis of...Ch. 2.4 - Describe the auxiliary line segment as determined,...Ch. 2.4 - Describe the auxiliary line segment as determined,...Ch. 2.4 - In Exercises 7 and 8, classify the trianglenot...Ch. 2.4 - In Exercises 7 and 8, classify the trianglenot...Ch. 2.4 - In Exercises 9 and 10, classify the triangle not...Ch. 2.4 - In Exercises 9 and 10, classify the triangle not...Ch. 2.4 - In Exercises 11 and12, make drawings as needed....Ch. 2.4 - In Exercises 11 and 12, make drawings as needed....Ch. 2.4 - In Exercises 13 to 15, jk and ABC. Given:...Ch. 2.4 - In Exercises 13 to 15, jk and ABC. Given:...Ch. 2.4 - In Exercises 13 to 15, jk and. ABC. Given:...Ch. 2.4 - Given: MNNQ and s as shown Find: x, y, and zCh. 2.4 - Given: ABDC DB bisects ADC mA=110 Find: m3Ch. 2.4 - Given: ABDC DB bisects ADC m1=36 Find: mACh. 2.4 - Prob. 19ECh. 2.4 - Given: ABC with BDCE m1=2xm3=x Find: mB in terms...Ch. 2.4 - Given: ADE with m1=m2=x Find: mDAE=x2 x, m1, and...Ch. 2.4 - Given: ABC with mB=mC=x2 Find: mBAC=x x, mBAC, and...Ch. 2.4 - Consider any triangle and one exterior angle at...Ch. 2.4 - Given: Right ABC with right C m1=7x+4m2=5x+2 Find:...Ch. 2.4 - In Exercises 25 to 27 , see the figure for...Ch. 2.4 - In Exercises 25 to 27 , see the figure for...Ch. 2.4 - Prob. 27ECh. 2.4 - Given: m1=8(x+2)m3=5x3m5=5(x+1)2 Find: xCh. 2.4 - Given: Find: , , andCh. 2.4 - Given: Equiangular RST Prove: RV bisects SRT RVS...Ch. 2.4 - Prob. 31ECh. 2.4 - The sum of the measures of two angles of a...Ch. 2.4 - Draw, if possible, an a isosceles obtuse triangle....Ch. 2.4 - Prob. 34ECh. 2.4 - Along a straight shoreline, two houses are located...Ch. 2.4 - An airplane has leveled off is flying horizontally...Ch. 2.4 - Prob. 37ECh. 2.4 - The roofline of a house shows the shape of a right...Ch. 2.4 - A lamppost has design such that mC=110 and AB....Ch. 2.4 - For the lamppost of Exercise 39, Suppose that...Ch. 2.4 - Prob. 41ECh. 2.4 - Prob. 42ECh. 2.4 - Prob. 43ECh. 2.4 - Explain why the following statement is true. The...Ch. 2.4 - Prob. 45ECh. 2.4 - Prob. 46ECh. 2.4 - Prob. 47ECh. 2.4 - Given: AB, DE and CF ABDE CG bisects BCF FG...Ch. 2.4 - Given: NQ bisects MNP PQ bisects MPR mQ=42 Find:...Ch. 2.4 - Given: In right ABC, AD bisects CAB and BF bisects...Ch. 2.5 - For Exercises 1 and 2, consider a group of regular...Ch. 2.5 - For Exercises 1 and 2, consider a group of regular...Ch. 2.5 - Given: ABDC, ADBC, AEFC, with angle measures as...Ch. 2.5 - In pentagon ABCDE with BDE, find the measure of...Ch. 2.5 - Find the total number of diagonals for a polygon...Ch. 2.5 - Find the total number of diagonals for a polygon...Ch. 2.5 - Find the sum of the measures of the interior...Ch. 2.5 - Find the sum of the measures of the interior...Ch. 2.5 - Find the measure of each interior angle of a...Ch. 2.5 - Find the measure of each interior angle of a...Ch. 2.5 - Find the sum of the measures of the exterior...Ch. 2.5 - Find the sum of the measures of the exterior...Ch. 2.5 - Find the measures of each exterior angle of a...Ch. 2.5 - Find the measures of each exterior angle of a...Ch. 2.5 - Find the number of sides for a polygon whose sum...Ch. 2.5 - Find the number of sides for a polygon whose sum...Ch. 2.5 - Find the number of sides for a regular polygon...Ch. 2.5 - Find the number of sides for a regular polygon...Ch. 2.5 - Find the number of sides for a regular polygon...Ch. 2.5 - Find the number of sides for a regular polygon...Ch. 2.5 - What is the measure of each interior angle of a...Ch. 2.5 - Lug bolts are equally spaced about the wheel to...Ch. 2.5 - In Exercises 23 to 28 , with P={allpolygons} as...Ch. 2.5 - Prob. 24ECh. 2.5 - Prob. 25ECh. 2.5 - Prob. 26ECh. 2.5 - Prob. 27ECh. 2.5 - Prob. 28ECh. 2.5 - Given: Quadrilateral RSTQ with exterior s at R and...Ch. 2.5 - Given: Regular hexagon ABCDEF with diagonal AC and...Ch. 2.5 - Given: Quadrilateral RSTV with diagonals RT and SV...Ch. 2.5 - Given: Quadrilateral ABCD with BAAD and BCDC...Ch. 2.5 - A father wishes to make a baseball home plate for...Ch. 2.5 - The adjacent interior and exterior angles of a...Ch. 2.5 - Find the measure of each a acute interior angle of...Ch. 2.5 - Find the measure of each a acute interior angle of...Ch. 2.5 - Consider any regular polygon; find and join in...Ch. 2.5 - Consider a regular hexagon RSTUVW. What does...Ch. 2.5 - The face of a clock has the shape of a regular...Ch. 2.5 - The top surface of a picnic table is in the shape...Ch. 2.5 - Prob. 41ECh. 2.5 - For the concave quadrilateral ABCD, explain why...Ch. 2.5 - If mA=20, mB=88 and mC=31, find the measure of the...Ch. 2.5 - Is it possible for a polygon to have the following...Ch. 2.5 - Is it possible for a regular polygon to have the...Ch. 2.5 - Draw a concave hexagon that has: a one interior...Ch. 2.5 - Draw a concave pentagon that has: a one interior...Ch. 2.5 - For concave pentagon ABCDE, find the measure of...Ch. 2.5 - For concave hexagon HJKLMN, mH=y and the measure...Ch. 2.5 - In concave hexagon HJKLMN, mH=72. Exterior angles...Ch. 2.6 - Prob. 1ECh. 2.6 - Prob. 2ECh. 2.6 - Prob. 3ECh. 2.6 - Which letters have symmetry with respect to a...Ch. 2.6 - Prob. 5ECh. 2.6 - Prob. 6ECh. 2.6 - Prob. 7ECh. 2.6 - Prob. 8ECh. 2.6 - Prob. 9ECh. 2.6 - Prob. 10ECh. 2.6 - Prob. 11ECh. 2.6 - Prob. 12ECh. 2.6 - Prob. 13ECh. 2.6 - Prob. 14ECh. 2.6 - Prob. 15ECh. 2.6 - Suppose that square RSTV slides point for point to...Ch. 2.6 - Prob. 17ECh. 2.6 - Prob. 18ECh. 2.6 - Prob. 19ECh. 2.6 - Prob. 20ECh. 2.6 - Prob. 21ECh. 2.6 - Prob. 22ECh. 2.6 - In which direction clockwise or counterclockwise...Ch. 2.6 - In which direction clockwise or counterclockwise...Ch. 2.6 - Considering that the consecutive dials on the...Ch. 2.6 - Considering that the consecutive dials on the...Ch. 2.6 - Prob. 27ECh. 2.6 - Prob. 28ECh. 2.6 - Prob. 29ECh. 2.6 - Prob. 30ECh. 2.6 - A regular hexagon is rotated about a centrally...Ch. 2.6 - A regular octagon is rotated about a centrally...Ch. 2.6 - Prob. 33ECh. 2.6 - XYZ is the image of XYZ following a 100...Ch. 2.6 - Hexagon ABCBAD is determined when the open figure...Ch. 2.6 - Prob. 36ECh. 2.CR - If m1=m2, which line segments are parallel?Ch. 2.CR - Prob. 2CRCh. 2.CR - Prob. 3CRCh. 2.CR - Prob. 4CRCh. 2.CR - Given: mDCA=130mBAC=2x+ymBCE=150mDEC=2xy Find: x...Ch. 2.CR - Prob. 6CRCh. 2.CR - Prob. 7CRCh. 2.CR - Prob. 8CRCh. 2.CR - For Review Exercises 7 to 11, use the given...Ch. 2.CR - For Review Exercises 7 to 11, use the given...Ch. 2.CR - For Review Exercises 7 to 11, use the given...Ch. 2.CR - Prob. 12CRCh. 2.CR - Prob. 13CRCh. 2.CR - Prob. 14CRCh. 2.CR - For Review Exercises 12 to 15 , find the values of...Ch. 2.CR - Given: m1=x212 m4=x(x2) Find: x so that ABCDCh. 2.CR - Prob. 17CRCh. 2.CR - Prob. 18CRCh. 2.CR - Prob. 19CRCh. 2.CR - For Review Exercises 19 to 24, decide whether the...Ch. 2.CR - Prob. 21CRCh. 2.CR - Prob. 22CRCh. 2.CR - Prob. 23CRCh. 2.CR - Prob. 24CRCh. 2.CR - Complete the following table for regular polygons....Ch. 2.CR - Prob. 26CRCh. 2.CR - For Review Exercises 26 to 29, sketch, if...Ch. 2.CR - For Review Exercises 26 to 29, sketch, if...Ch. 2.CR - Prob. 29CRCh. 2.CR - For Review Exercises 30 and 31, write the...Ch. 2.CR - For Review Exercises 30 and 31, write the...Ch. 2.CR - Which statementthe converse, the inverse, or the...Ch. 2.CR - Given: ABCF23 Prove: 13Ch. 2.CR - Given: 1 is complementary to 2; 2 is complementary...Ch. 2.CR - Prob. 35CRCh. 2.CR - Given: ACDCAB Prove: DACBCh. 2.CR - Prob. 37CRCh. 2.CR - Prob. 38CRCh. 2.CR - Prob. 39CRCh. 2.CR - Prob. 40CRCh. 2.CR - Prob. 41CRCh. 2.CR - Construct an equilateral triangle ABC with side...Ch. 2.CR - Prob. 43CRCh. 2.CR - Prob. 44CRCh. 2.CR - Prob. 45CRCh. 2.CR - Complete the drawing so that the figure is...Ch. 2.CR - Prob. 47CRCh. 2.CT - Consider the figure shown at the right. a Name the...Ch. 2.CT - In the accompanying figure, m2=68, m8=112, and...Ch. 2.CT - To prove a theorem of the form "If P, then Q" by...Ch. 2.CT - Assuming that statements 1 and 2 are true, draw a...Ch. 2.CT - Let all of the lines named be coplanar. Make a...Ch. 2.CT - Prob. 6CTCh. 2.CT - For ABC, find mB if a mA=65 and mC=79. b mA=2x,...Ch. 2.CT - Prob. 8CTCh. 2.CT - a Given that the polygon shown has six congruent...Ch. 2.CT - Prob. 10CTCh. 2.CT - Prob. 11CTCh. 2.CT - In the figure shown, suppose that ABDC and ADBC....Ch. 2.CT - Prob. 13CTCh. 2.CT - Prob. 14CTCh. 2.CT - Prob. 15CTCh. 2.CT - In Exercises 16 and 18 , complete the missing...Ch. 2.CT - Prob. 17CTCh. 2.CT - Prob. 18CTCh. 2.CT - Prob. 19CT
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, geometry and related others by exploring similar questions and additional content below.Similar questions
- 1) Find the prime factorization of 111111. 2) Find (-88 mod 13) 5 mod 7. 3) Use the Euclidean algorithm to find gcd(144,233).arrow_forward3 10) Suppose B = 2 4 5) and C = (b 2 prove that no such matrix exists. (6 1) . Find a matrix A such that AB = C orarrow_forwardi let V-H.) R aluv) = (v) where alu)=SU. V dx and { and (v) sv Show that the finite element solution Un Unique Prove that U-Un ll≤ch llull A²=f and U= Ju =0 on on a with bili near from a (u,v) = SAU. Av dr 32 Prove that aluv) is countinous and V-expitic ii Prove that 2 Mete ||(U-U|| ²== ||||²+|| || ² where -Auf in U=0 on 2arrow_forward
- Concdsider the following problem in R² ди -MAU+B+P+4= f inv ax U= 0 of 2 Bi-1, 2 are so prove. that al) is continous and, V-elliptic ⑥Provethat 14-Ull, s chllull zudx Where lull" = a(u,u) = £14. Ju 2 dx+ u.uds an let U= x(1-x)y (1-y) is gol. to -Au=f Compute || Ull Li and lull in are Ju, Duarrow_forwardMake Varitional for Malation for Problem and prove al.,.) is continous and, V-elliptic 224 2x² - -40² 4+24=1 ayz Xe=oonT 1 - €4 +x4 +4 = f XE I = (0,2), 4(a)=(()=0 (iii) - Au+S4=f Xen yo U=0, XE INarrow_forward4) Given: LMNP is a parallelogram, PRSM, LR 1 PM, and NS 1 PM Prove: PS MR Statement Reason P L S R N Marrow_forward
- 5) Given: Quadrilateral MNPQ with Fill in the missing reasons to show MNPQ is a parallelogram. 1/4 and 2 = 3. M N 1 2 4 3 Reason P Statement 1. 1 ≈ 4 and 2 = <3 1. Given 2. NQ=NQ 2. 3. AMNQ = APQN 3. 4. MN QP and NP = MQ 4. 5. MNPQ is a parallelogram 5.arrow_forward3) Given: EFGH is a parallelogram and ZH is a right angle. Prove: EFGH is a rectangle. E F G Harrow_forwardDirections: In parallelogram QRST shown, mZQRS = 61°, mZQST = 52°, and point U is located on QR such that TU is perpendicular to diagonal QS. (a) Determine the measure of TQR. (b) Determine the measure of /TQS. (c) Determine the measure of ZQTU. U R Sarrow_forward
- onsider the variational form a(u,v) = (f,v) where a(u,v) = 'u'v' dx Prove that VvE H(0, 1), i = 1, 2,...., n - 1 a(v,q)=-v(x-1) + 2v(x) = v(x+1)] h - where (,), CV, is usual basis of hat functions Q2: A: Consider the problem A²u = f and u= ди On = 0 on an With bilinear form a(u,v) = f Au. Av dn prove that a(u,v) V-ellpitic. B: Prove that Where lu-ulls Chulz lul = a(u, u) = f vu.vu dx + fonu. uds Q3: Consider the problem -V. (Vu) + u = 0, in n.Vu=gN on an 1- Show that the solution u of the problem satisfies the stability lulul≤ Clignlin (use 2abarrow_forwardIf r/s=t/u then r+s/t+u=?arrow_forwardGiven square ABCD with diagonals AC, BC. the measurement of DEC = 2a+b and measurement of ABC is a+2b. Find a and b.arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
Recommended textbooks for you
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Functions and Change: A Modeling Approach to Coll...
Algebra
ISBN:9781337111348
Author:Bruce Crauder, Benny Evans, Alan Noell
Publisher:Cengage Learning
What are the Different Types of Triangles? | Don't Memorise; Author: Don't Memorise;https://www.youtube.com/watch?v=1k0G-Y41jRA;License: Standard YouTube License, CC-BY
Law of Sines AAS, ASA, SSA Ambiguous Case; Author: Mario's Math Tutoring;https://www.youtube.com/watch?v=FPVGb-yWj3s;License: Standard YouTube License, CC-BY
Introduction to Statistics..What are they? And, How Do I Know Which One to Choose?; Author: The Doctoral Journey;https://www.youtube.com/watch?v=HpyRybBEDQ0;License: Standard YouTube License, CC-BY
Triangles | Mathematics Grade 5 | Periwinkle; Author: Periwinkle;https://www.youtube.com/watch?v=zneP1Q7IjgQ;License: Standard YouTube License, CC-BY
What Are Descriptive Statistics And Inferential Statistics?; Author: Amour Learning;https://www.youtube.com/watch?v=MUyUaouisZE;License: Standard Youtube License