Discrete Mathematics with Graph Theory (Classic Version) (3rd Edition) (Pearson Modern Classics for Advanced Mathematics Series)
3rd Edition
ISBN: 9780134689555
Author: Edgar Goodaire, Michael Parmenter
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 2.3, Problem 4E
With a table like that in Fig. 2.2, illustrate a relation on the set
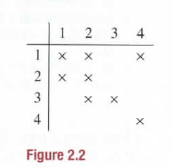
(a) reflexive and symmetric.
(b) not symmetric and not antisymmetric.
(c) not symmetric but antisymmetric.
(d) transitive.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Chapter 2 Solutions
Discrete Mathematics with Graph Theory (Classic Version) (3rd Edition) (Pearson Modern Classics for Advanced Mathematics Series)
Ch. 2.1 - 5{x+2y|x{0,1,2},y{2,0,2}}Ch. 2.1 - 5{x+2y|x{0,1,2},y{2,0,2}}Ch. 2.1 - If A={a,b}, then bA.Ch. 2.1 - Prob. 4TFQCh. 2.1 - {,{}}Ch. 2.1 - {,{}}Ch. 2.1 - True/False Questions
7.
Ch. 2.1 - Prob. 8TFQCh. 2.1 - (Assume A and B are sets). ABBA.Ch. 2.1 - If A has two elements, then P(P(A)) has eight...
Ch. 2.1 - Prob. 1ECh. 2.1 - 2. List five elements in each of the following...Ch. 2.1 - Let A={1,2,3,4}. List all the subsets B of A such...Ch. 2.1 - 4. Let . Are the following statements true or...Ch. 2.1 - 5. Determine which of the following are true and...Ch. 2.1 - Prob. 6ECh. 2.1 - Determine which of the following are true and...Ch. 2.1 - Prob. 8ECh. 2.1 - a) List all the subset of set {a,b,c,d} that...Ch. 2.1 - (a) How many elements are in the power set of the...Ch. 2.1 - Prob. 11ECh. 2.1 - Prob. 12ECh. 2.1 - Prob. 13ECh. 2.1 - Prob. 14ECh. 2.2 - Prob. 1TFQCh. 2.2 - Prob. 2TFQCh. 2.2 - Prob. 3TFQCh. 2.2 - Prob. 4TFQCh. 2.2 - If A and B are setsand AB, then AB.Ch. 2.2 - Prob. 6TFQCh. 2.2 - The name of Augustus De Morgan appears in both...Ch. 2.2 - (AC)(BcAc).Ch. 2.2 - True/False Questions
9.
Ch. 2.2 - Prob. 10TFQCh. 2.2 - List A={xN|x7},B={xZ||x2|4} and C={xR|x34x=0}:...Ch. 2.2 - Prob. 2ECh. 2.2 - Prob. 3ECh. 2.2 - Prob. 4ECh. 2.2 - For A={a,b,c,{a,b}}, find (a) A{a,b} (b) {}P(A)...Ch. 2.2 - Prob. 6ECh. 2.2 - 7. Let , and . List the elements in the...Ch. 2.2 - Let n3 and A={1,2,3,.....n}. (a) How many subsets...Ch. 2.2 - Prob. 9ECh. 2.2 - 10. The universal set for this problem is the set...Ch. 2.2 - Prob. 11ECh. 2.2 - 12. Let P denote the set of primes and E the set...Ch. 2.2 - For nZ, let An={aZ|an}. Find each of the following...Ch. 2.2 - Prob. 14ECh. 2.2 - 15. Let , and .
(a) Draw a Venn diagram showing...Ch. 2.2 - Prob. 16ECh. 2.2 - Prob. 17ECh. 2.2 - Prob. 18ECh. 2.2 - Prob. 19ECh. 2.2 - Prob. 20ECh. 2.2 - Prob. 21ECh. 2.2 - Prob. 22ECh. 2.2 - Prob. 23ECh. 2.2 - Prob. 24ECh. 2.2 - Prob. 25ECh. 2.2 - Prob. 26ECh. 2.2 - Prob. 27ECh. 2.2 - Prob. 28ECh. 2.2 - Prob. 29ECh. 2.2 - Prob. 30ECh. 2.2 - Prob. 31ECh. 2.3 - {(x,x+1)|xN} is a binary relation on N, the set of...Ch. 2.3 - True/False Questions
2. is a binary relation on...Ch. 2.3 - Let be a binary relation on a set A and let aA....Ch. 2.3 - Let R be a binary relation on a set A and let aA....Ch. 2.3 - Let R be a binary relation on a set A. If R is not...Ch. 2.3 - True/False Questions
6. Let be a binary relation...Ch. 2.3 - True/False Questions
7. Let be a binary relation...Ch. 2.3 - If a binary relation R is antisymmetric, then R is...Ch. 2.3 - If a binary relation R is notsymmetric, then it is...Ch. 2.3 - True/False Questions
10. Let be a binary relation...Ch. 2.3 - Let B denote the set of books in a college library...Ch. 2.3 - 2. Let A denote the set of names of streets in St....Ch. 2.3 - Determine which of the properties reflexive,...Ch. 2.3 - 4. With a table like that in Fig. 2.2, illustrate...Ch. 2.3 - 5. Let . List the ordered pairs in a relation on A...Ch. 2.3 - 6. Is it possible for a binary relation to be both...Ch. 2.3 - What is wrong with the following argument, which...Ch. 2.3 - Determine whether each of the binary relations R...Ch. 2.3 - Determine whether each of the binary relations R...Ch. 2.3 - Prob. 10ECh. 2.3 - Prob. 11ECh. 2.3 - Prob. 12ECh. 2.3 - Prob. 13ECh. 2.4 - True/False Questions
“” defines an equivalence...Ch. 2.4 - An equivalence relation on a set A is a binary...Ch. 2.4 - Prob. 3TFQCh. 2.4 - Prob. 4TFQCh. 2.4 - Prob. 5TFQCh. 2.4 - Prob. 6TFQCh. 2.4 - Prob. 7TFQCh. 2.4 - Prob. 8TFQCh. 2.4 - Prob. 9TFQCh. 2.4 - Prob. 10TFQCh. 2.4 - Prob. 11TFQCh. 2.4 - Prob. 1ECh. 2.4 - 2. Explain why each of the following binary...Ch. 2.4 - Prob. 3ECh. 2.4 - Prob. 4ECh. 2.4 - Prob. 5ECh. 2.4 - For natural numbers a and b, define a~b if and...Ch. 2.4 - Prob. 7ECh. 2.4 - 8. For integers a, b, define if and only if for...Ch. 2.4 - 9. Define ~ on Z by if and only if is a multiple...Ch. 2.4 - 10. For integers a and b, define if for some...Ch. 2.4 - 11. For , define if and only if .
(a) Prove that...Ch. 2.4 - For a,bZ, define a~b if and only if a2b2 is...Ch. 2.4 - Prob. 13ECh. 2.4 - Prob. 14ECh. 2.4 - Prob. 15ECh. 2.4 - Define ~ on R2 by (x,y)~(u,v) if and only if...Ch. 2.4 - Prob. 17ECh. 2.4 - Determine which of the following define...Ch. 2.4 - Prob. 19ECh. 2.4 - Prob. 20ECh. 2.4 - Prob. 21ECh. 2.4 - 22. Let A be the set of all natural numbers and ~...Ch. 2.4 - Prob. 23ECh. 2.4 - Prob. 24ECh. 2.4 - Prob. 25ECh. 2.5 - A partial order on a set A is a reflexive,...Ch. 2.5 - Prob. 2TFQCh. 2.5 - True/False Questions
3. The binary relation “” on...Ch. 2.5 - Prob. 4TFQCh. 2.5 - Hasse diagrams are used to identify the...Ch. 2.5 - Prob. 6TFQCh. 2.5 - Prob. 7TFQCh. 2.5 - Prob. 8TFQCh. 2.5 - Prob. 9TFQCh. 2.5 - The statement in Question 9 is the contrapositive...Ch. 2.5 - 1. Determine whether each of the following...Ch. 2.5 - 2. (a) List the elements of the set in...Ch. 2.5 - List all pairs (x,y) with xy in the partial orders...Ch. 2.5 - Prob. 4ECh. 2.5 - Prob. 5ECh. 2.5 - Prob. 6ECh. 2.5 - In the poset (P(S),) of subsets of a set S, under...Ch. 2.5 - Prob. 8ECh. 2.5 - Prob. 9ECh. 2.5 - 10. (a) Let and, for and in A, define if and...Ch. 2.5 - Prob. 11ECh. 2.5 - Prob. 12ECh. 2.5 - Prob. 13ECh. 2.5 - Prob. 14ECh. 2.5 - Prob. 15ECh. 2.5 - Prob. 16ECh. 2.5 - Prob. 17ECh. 2.5 - Prob. 18ECh. 2 - If A={xN|x7},B={xZ||x5|3}andC={2,3}, find (AB)C.Ch. 2 - Let A={xZ|1x2},B={2x3|xA},andC={xR|x=ab,aA,bB}....Ch. 2 - Prob. 3RECh. 2 - Prob. 4RECh. 2 - 5. This exercise refers to the Venn diagram shown...Ch. 2 - Prob. 6RECh. 2 - Let A={1}. Find P(P(A)).Ch. 2 - Prob. 8RECh. 2 - Give an example showing that the statement...Ch. 2 - Prob. 10RECh. 2 - 11. Let A be a set.
(a) What is meant by the term...Ch. 2 - Let A={xR||x|1} and, for a,bA, define (a,b)R if...Ch. 2 - Determine whether ~ is reflexive, symmetric,...Ch. 2 - For a,bN, define a~b if and only if ab. Determine...Ch. 2 - 15. Define a relation R on Z by if is a multiple...Ch. 2 - 16. Define a relation R on Z by if is a multiple...Ch. 2 - 17. Let ~ denote an equivalence relation on a set...Ch. 2 - 18. Let ~ denote an equivalence relation on a set...Ch. 2 - Prob. 19RECh. 2 - Let A be the set of points different from the...Ch. 2 - Show that (P(Z),) is a partially ordered set.Ch. 2 - Let A={1,2,4,6,8} and, for a,bA, define a_b if and...Ch. 2 - 23. Let be a poset and . Can a and b have two...
Additional Math Textbook Solutions
Find more solutions based on key concepts
147. Draining a tank Water drains from the conical tank shown in the accompanying figure at the rate .
a. What...
University Calculus
Silvia wants to mix a 40% apple juice drink with pure apple juice to make 2 L of a juice drink that is 80% appl...
Beginning and Intermediate Algebra
The largest polynomial that divides evenly into a list of polynomials is called the _______.
Elementary & Intermediate Algebra
Complete each statement with the correct term from the column on the right. Some of the choices may not be used...
Intermediate Algebra (13th Edition)
Find all solutions of each equation in the interval .
Precalculus: A Unit Circle Approach (3rd Edition)
Evaluate the integrals in Exercises 1–46.
1.
University Calculus: Early Transcendentals (4th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- Give an example of a relation R on a nonempty set A that is symmetric and transitive, but not reflexive.arrow_forwardIn each of the following parts, a relation is defined on the set of all human beings. Determine whether the relation is reflective, symmetric, or transitive. Justify your answers. xRy if and only if x lives within 400 miles of y. xRy if and only if x is the father of y. xRy if and only if x is a first cousin of y. xRy if and only if x and y were born in the same year. xRy if and only if x and y have the same mother. xRy if and only if x and y have the same hair colour.arrow_forwardLabel each of the following statements as either true or false. Let R be a relation on a nonempty set A that is symmetric and transitive. Since R is symmetric xRy implies yRx. Since R is transitive xRy and yRx implies xRx. Hence R is alsoreflexive and thus an equivalence relation on A.arrow_forward
- True or False Label each of the following statements as either true or false. Let be an equivalence relation on a nonempty setand let and be in. If, then.arrow_forward13. Consider the set of all nonempty subsets of . Determine whether the given relation on is reflexive, symmetric or transitive. Justify your answers. a. if and only if is subset of . b. if and only if is a proper subset of . c. if and only if and have the same number of elements.arrow_forwardIn Exercises 610, a relation R is defined on the set Z of all integers, In each case, prove that R is an equivalence relation. Find the distinct equivalence classes of R and least four members of each. xRy if and only if x2+y2 is a multiple of 2.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
Elements Of Modern AlgebraAlgebraISBN:9781285463230Author:Gilbert, Linda, JimmiePublisher:Cengage Learning,
Elements Of Modern Algebra
Algebra
ISBN:9781285463230
Author:Gilbert, Linda, Jimmie
Publisher:Cengage Learning,
What is a Relation? | Don't Memorise; Author: Don't Memorise;https://www.youtube.com/watch?v=hV1_wvsdJCE;License: Standard YouTube License, CC-BY
RELATIONS-DOMAIN, RANGE AND CO-DOMAIN (RELATIONS AND FUNCTIONS CBSE/ ISC MATHS); Author: Neha Agrawal Mathematically Inclined;https://www.youtube.com/watch?v=u4IQh46VoU4;License: Standard YouTube License, CC-BY