
Concept explainers
(a)
To find: The proportions of failures for businesses headed by women and businesses headed by men.
To test: Whether the same proportion of women’s and men’s businesses fails or not.
To find: The hypotheses, the test statistic, P-value and conclusion.
(a)
Answer to Problem 23.25E
The proportion of failures for businesses headed by men is 0.1415.
The proportion of failures for businesses headed by women is 0.1666.
The null hypothesis is
The alternative hypothesis is
The value of the z-statistic is –0.39.
The P-value is 0.698.
The conclusion is that, the same proportion of women’s and men’s businesses fails.
Explanation of Solution
Given info:
In the survey, 15 out of 106 were headed by men failed and 7 out of 42 were headed by women failed.
Calculation:
PLAN:
Check whether the same proportion of women’s and men’s businesses fails or not.
State the test hypotheses.
Null hypothesis:
Alternative hypothesis:
SOLVE:
Test statistic and P-value:
Software procedure:
Step by step procedure to obtain proportion, test statistic and P-value using the MINITAB software:
- Choose Stat > Basic Statistics > 2 Proportions.
- Choose Summarized data.
- In First sample, enter Trials as 106 and
Events as 15. - In Second sample, enter Trials as 42 and Events as 7.
- Choose Options.
- Choose Pooled proportions.
- In Confidence level, enter 95.
- In Alternative, select not equal.
- Click OK in all the dialogue boxes.
Output using the MINITAB software is given below:

From the MINITAB output,
The proportion of failures for businesses headed by men is 0.1415.
The proportion of failures for businesses headed by women is 0.1666.
The value of the z-statistic is –0.39.
The P-value is 0.698.
CONCLUDE:
The P-value is 0.698 and the significance level is 0.05.
Here, the P-value is greater than the significance level.
That is,
Therefore, using the rejection rule, it can be concluded that there is no evidence to reject
Thus, the same proportion of women’s and men’s businesses fails.
(b)
To find: The proportions of failures for businesses headed by women and businesses headed by men.
To test: Whether the same proportion of women’s and men’s businesses fails or not.
To find: The hypotheses, the test statistic, P-value and conclusion.
(b)
Answer to Problem 23.25E
The proportion of failures for businesses headed by men is 0.1415.
The proportion of failures for businesses headed by women is 0.1666.
The null hypothesis is
The alternative hypothesis is
The value of the z-statistic is –2.12.
The P-value is 0.034.
The conclusion is that, there is no evidence that the same proportion of women’s and men’s businesses fails.
Explanation of Solution
Given info:
In the survey, 210 out of 1,260 were headed by women failed and 450 out of 3,180 were headed by men failed.
Calculation:
PLAN:
Check whether the same proportion of women’s and men’s businesses fails or not.
State the test hypotheses.
Null hypothesis:
Alternative hypothesis:
SOLVE:
Test statistic and P-value:
Software procedure:
Step by step procedure to obtain proportion, test statistic and P-value using the MINITAB software:
- Choose Stat > Basic Statistics > 2 Proportions.
- Choose Summarized data.
- In First sample, enter Trials as 3,180 and Events as 450.
- In Second sample, enter Trials as 1,260 and Events as 210.
- Choose Options.
- Choose Pooled proportions.
- In Confidence level, enter 95.
- In Alternative, select not equal.
- Click OK in all the dialogue boxes.
Output using the MINITAB software is given below:
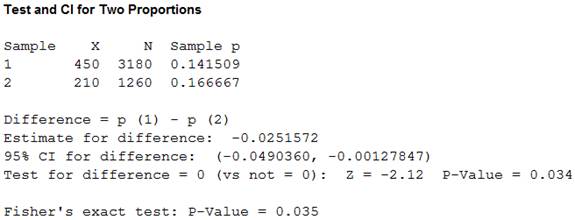
From the MINITAB output,
The proportion of failures for businesses headed by men is 0.1415.
The proportion of failures for businesses headed by women is 0.1666.
The value of the z-statistic is –2.12.
The P-value is 0.034.
CONCLUDE:
The P-value is 0.034 and the significance level is 0.05.
Here, the P-value is less than the significance level.
That is,
Therefore, using the rejection rule, it can be concluded that there is evidence to reject
Thus, there is no evidence that the same proportion of women’s and men’s businesses fails.
(c)
To find: The 95% confidence interval for the difference between the proportions of women’s and men’s businesses that fail for the settings of part (a).
To find: The 95% confidence interval for the difference between the proportions of women’s and men’s businesses that fail for the settings of part (b).
To identify: The effect of larger samples on the confidence interval.
(c)
Answer to Problem 23.25E
The 95% confidence interval for the difference between the proportions of women’s and men’s businesses that fail for the settings of part (a) is
The 95% confidence interval for the difference between the proportions of women’s and men’s businesses that fail for the settings of part (b) is
The effect of larger samples on the confidence interval is that the confidence interval is narrow.
Explanation of Solution
Given info:
In the survey, 210 out of 1,260 were headed by women failed and 450 out of 3,180 were headed by men failed.
Calculation:
From part (a), the 95% confidence interval for the difference between the proportions of women’s and men’s businesses that fail for the settings of part (a) is
From part (b), the 95% confidence interval for the difference between the proportions of women’s and men’s businesses that fail for the settings of part (b) is
Justification:
From the results, it can be observed that the confidence interval is narrow if the sample is 30 times large.
Want to see more full solutions like this?
Chapter 23 Solutions
Basic Practice of Statistics (Instructor's)
- ons 12. A sociologist hypothesizes that the crime rate is higher in areas with higher poverty rate and lower median income. She col- lects data on the crime rate (crimes per 100,000 residents), the poverty rate (in %), and the median income (in $1,000s) from 41 New England cities. A portion of the regression results is shown in the following table. Standard Coefficients error t stat p-value Intercept -301.62 549.71 -0.55 0.5864 Poverty 53.16 14.22 3.74 0.0006 Income 4.95 8.26 0.60 0.5526 a. b. Are the signs as expected on the slope coefficients? Predict the crime rate in an area with a poverty rate of 20% and a median income of $50,000. 3. Using data from 50 workarrow_forward2. The owner of several used-car dealerships believes that the selling price of a used car can best be predicted using the car's age. He uses data on the recent selling price (in $) and age of 20 used sedans to estimate Price = Po + B₁Age + ε. A portion of the regression results is shown in the accompanying table. Standard Coefficients Intercept 21187.94 Error 733.42 t Stat p-value 28.89 1.56E-16 Age -1208.25 128.95 -9.37 2.41E-08 a. What is the estimate for B₁? Interpret this value. b. What is the sample regression equation? C. Predict the selling price of a 5-year-old sedan.arrow_forwardian income of $50,000. erty rate of 13. Using data from 50 workers, a researcher estimates Wage = Bo+B,Education + B₂Experience + B3Age+e, where Wage is the hourly wage rate and Education, Experience, and Age are the years of higher education, the years of experience, and the age of the worker, respectively. A portion of the regression results is shown in the following table. ni ogolloo bash 1 Standard Coefficients error t stat p-value Intercept 7.87 4.09 1.93 0.0603 Education 1.44 0.34 4.24 0.0001 Experience 0.45 0.14 3.16 0.0028 Age -0.01 0.08 -0.14 0.8920 a. Interpret the estimated coefficients for Education and Experience. b. Predict the hourly wage rate for a 30-year-old worker with four years of higher education and three years of experience.arrow_forward
- 1. If a firm spends more on advertising, is it likely to increase sales? Data on annual sales (in $100,000s) and advertising expenditures (in $10,000s) were collected for 20 firms in order to estimate the model Sales = Po + B₁Advertising + ε. A portion of the regression results is shown in the accompanying table. Intercept Advertising Standard Coefficients Error t Stat p-value -7.42 1.46 -5.09 7.66E-05 0.42 0.05 8.70 7.26E-08 a. Interpret the estimated slope coefficient. b. What is the sample regression equation? C. Predict the sales for a firm that spends $500,000 annually on advertising.arrow_forwardCan you help me solve problem 38 with steps im stuck.arrow_forwardHow do the samples hold up to the efficiency test? What percentages of the samples pass or fail the test? What would be the likelihood of having the following specific number of efficiency test failures in the next 300 processors tested? 1 failures, 5 failures, 10 failures and 20 failures.arrow_forward
- The battery temperatures are a major concern for us. Can you analyze and describe the sample data? What are the average and median temperatures? How much variability is there in the temperatures? Is there anything that stands out? Our engineers’ assumption is that the temperature data is normally distributed. If that is the case, what would be the likelihood that the Safety Zone temperature will exceed 5.15 degrees? What is the probability that the Safety Zone temperature will be less than 4.65 degrees? What is the actual percentage of samples that exceed 5.25 degrees or are less than 4.75 degrees? Is the manufacturing process producing units with stable Safety Zone temperatures? Can you check if there are any apparent changes in the temperature pattern? Are there any outliers? A closer look at the Z-scores should help you in this regard.arrow_forwardNeed help pleasearrow_forwardPlease conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points. 4. One-Way ANOVA: Analyze the customer satisfaction scores across four different product categories to determine if there is a significant difference in means. (Hints: The null can be about maintaining status-quo or no difference among groups) H0 = H1=arrow_forward
- Please conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points 2. Two-Sample T-Test: Compare the average sales revenue of two different regions to determine if there is a significant difference. (Hints: The null can be about maintaining status-quo or no difference among groups; if alternative hypothesis is non-directional use the two-tailed p-value from excel file to make a decision about rejecting or not rejecting null) H0 = H1=arrow_forwardPlease conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points 3. Paired T-Test: A company implemented a training program to improve employee performance. To evaluate the effectiveness of the program, the company recorded the test scores of 25 employees before and after the training. Determine if the training program is effective in terms of scores of participants before and after the training. (Hints: The null can be about maintaining status-quo or no difference among groups; if alternative hypothesis is non-directional, use the two-tailed p-value from excel file to make a decision about rejecting or not rejecting the null) H0 = H1= Conclusion:arrow_forwardPlease conduct a step by step of these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points. The data for the following questions is provided in Microsoft Excel file on 4 separate sheets. Please conduct these statistical tests on separate sheets of Microsoft Excel. If the calculations in Microsoft Excel are incorrect, the null and alternative hypotheses, as well as the conclusions drawn from them, will be meaningless and will not receive any points. 1. One Sample T-Test: Determine whether the average satisfaction rating of customers for a product is significantly different from a hypothetical mean of 75. (Hints: The null can be about maintaining status-quo or no difference; If your alternative hypothesis is non-directional (e.g., μ≠75), you should use the two-tailed p-value from excel file to…arrow_forward
 MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman





