Chapter 2.2, Problem 43E

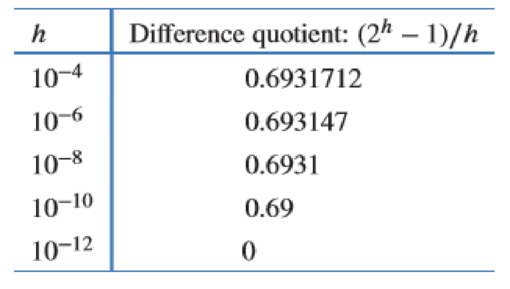
Suppose Table 2.3 on page 94 is continued with smaller values of h. A particular calculator gives the results in Table 2.4. (Your calculator may give slightly different results.) Comment on the values of the difference quotient in Table 2.4. In particular, why is the last value of (2^h − 1)∕h zero? What do you expect the calculated value of (2^h − 1)∕h to be when h = 10⁻²⁰?

Table 2.4 Questionable values of difference quotients of 2^x near x = 0