
(a)
Draw the PV diagram of the closed cycle.
(a)
Answer to Problem 34P
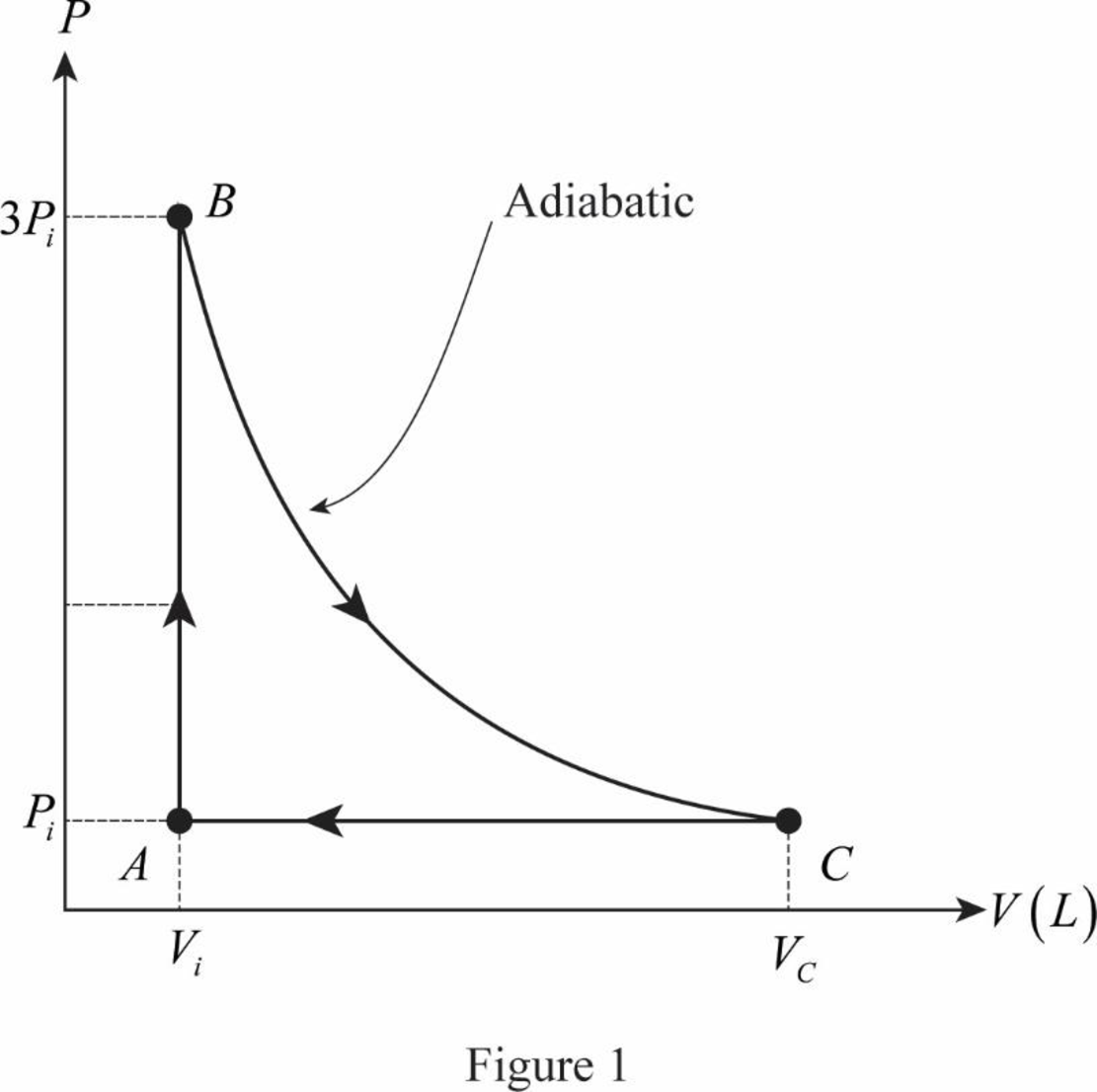
The PV diagram for the closed cycle is drawn.
Explanation of Solution
An adiabatic process occurs without
An Isobaric process is a thermodynamic process in which the pressure stays constant. The heat transferred to the system does work, but also changes the internal energy of the system.
Conclusion:
The figure 1 shows the PV diagram for the ideal gas expands adiabatically to its original pressure and compressed isobarically to its original volume.
(b)
The volume of the gas at the end of the adiabatic expansion.
(b)
Answer to Problem 34P
The volume of the gas at the end of the adiabatic expansion is
Explanation of Solution
Write the expression from PV diagram at the point B and C.
Here,
Conclusion:
Rewrite the above expression from the PV diagram to find volume.
Here,
Therefore, the volume of the gas at the end of the adiabatic expansion is
(c)
The temperature of the gas in an adiabatic expansion.
(c)
Answer to Problem 34P
The temperature of the gas in an adiabatic expansion is
Explanation of Solution
Write the expression from
Here,
Write the expression from the PV diagram
Here,
Conclusion:
Compare equation (II) and (III) to find
Therefore, the temperature of the gas in an adiabatic expansion is
(d)
The temperature at the end of the cycle.
(d)
Answer to Problem 34P
The temperature at the end of the cycle is
Explanation of Solution
Write the expression for temperature for whole cycle.
Here,
Conclusion:
Therefore, the temperature at the end of the cycle is
(e)
The net work done on the gas.
(e)
Answer to Problem 34P
The net work done on the gas is
Explanation of Solution
Write the expression for energy transfer between the points AB.
Here,
Replace
Write the expression for energy transfer for adiabatic process between the points BC.
Write the expression from ideal gas law.
Here,
Replace
Write the expression for energy transfer between the points CA.
Here,
Replace
Conclusion:
Find the energy transfer for whole cycle.
Substitute the equation (V), (VI) and (VII) in above equation.
Rewrite the above equation.
Find the internal energy for whole cycle.
Here,
Substitute equation (VIII) in the above equation.
Therefore, the net work done on the gas is
Want to see more full solutions like this?
Chapter 21 Solutions
Physics for Scientists and Engineers with Modern Physics, Technology Update
- 3. If the force of gravity stopped acting on the planets in our solar system, what would happen? a) They would spiral slowly towards the sun. b) They would continue in straight lines tangent to their orbits. c) They would continue to orbit the sun. d) They would fly straight away from the sun. e) They would spiral slowly away from the sun. 4. 1 The free-body diagram of a wagon being pulled along a horizontal surface is best represented by A F N B C 0 Ꭰ FN E a) A b) B c) C app app The app 10 app d) e) ס ח D E 10 apparrow_forwardPls help ASAParrow_forwardPls help asaparrow_forward
- Pls help asaparrow_forwardThe acceleration of an object sliding along a frictionless ramp is inclined at an angle 0 is 9. a) g tano b) g cose c) g sino 10. d) g e) zero A 1.5 kg cart is pulled with a force of 7.3 N at an angle of 40° above the horizontal. If a kinetic friction force of 3.2 N acts against the motion, the cart's acceleration along the horizontal surface will be a) 5.0 m/s² b) 1.6 m/s² c) 2.4 m/s² 11. d) 1.0 m/s² e) 2.7 m/s² What is the net force acting on an object with a mass of 10 kg moving at a constant velocity of 10 m/s [North]? a) 100 N [North] b) 100 N [South] 10 N [North} d) 10 N [South] e) None of these.arrow_forwardModified True/False - indicate whether the sentence or statement is true or false. If the statement is false, correct the statement to make it true. 12. An object in uniform circular motion has a constant velocity while experiencing centripetal acceleration. 13. An object travelling in uniform circular motion experiences an outward centrifugal force that tends to pull the object out of the circular path. 14. An object with less inertia can resist changes in motion more than an object with more inertia. 15. For an object sliding on a horizontal surface with a horizontal applied force, the frictional force will always increase as the applied force increases.arrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
 Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





