a)
To determine: Value of one month call option with $40 as an exercise price.
a)
Explanation of Solution
Given information:
Company H stock prices changes once in a month either by increase in 20% or decreases by 16.7%.
Current price level is $40 and interest rate is 1% per month.
Calculation of value of option:
First, it is necessary to find out the probabilities by using risk-neutral method.
Therefore, the value of p is 48% and,
Therefore, there is 48% of chances that price of stock will rise by 20% and a 52% chances that option will worth of $0 when it matures.
Therefore, the value of call is $3.82
b)
To determine: Value of delta.
b)
Explanation of Solution
Calculation of value of delta:
Hence, the value of option delta is 0.545
c)
To determine: The way payoffs of this call option be replicated based on replicated portfolio method.
c)
Explanation of Solution
Calculation of value of call by using replicating portfolio method:
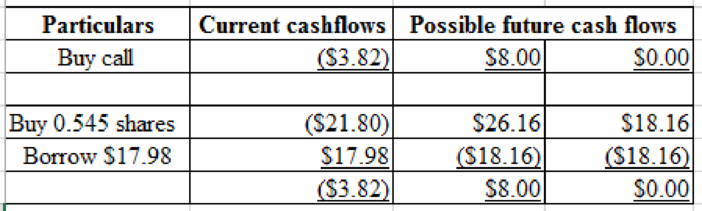
Hence, the value of call is $3.82
d)
To determine: Value of two month call option with $40 as an exercise price.
d)
Explanation of Solution
Calculation of value of option:
The following option possibilities are as follows,
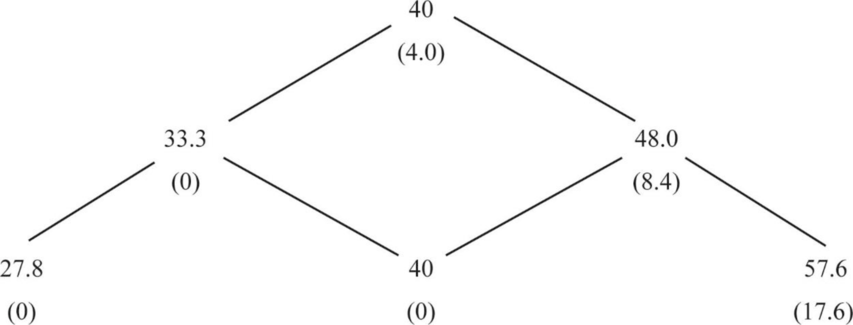
Month 1-a:
Hence the value of call under month 1-a is ‘0’
Month 1-b:
Therefore, value of the call under month 1-b is $8.4
Month 0:
Therefore, value of the call under month 0 is $4.0
e)
To determine: Value of delta.
e)
Explanation of Solution
Calculation of delta:
Hence, the delta value is 0.572
Want to see more full solutions like this?
Chapter 21 Solutions
Principles of Corporate Finance (Mcgraw-hill/Irwin Series in Finance, Insurance, and Real Estate)
- An investment that is worth $27,200 is expected to pay you $62,280 in 5 years and has an expected return of X percent per year. What is X?arrow_forwardDon't used Ai solution and don't used hand raitingarrow_forward3-7. (Working with an income statement and balance sheet) Prepare a balance sheet and income statement for Kronlokken Company from the following scrambled list of items. a. Prepare a common-sized income statement and a common-sized balance sheet. Interpret your findings. Depreciation expense $66,000 Cash 225,000 Long-term debt 334,000 Sales 573,000 Accounts payable 102,000 General and administrative expense 79,000 Buildings and equipment 895,000 Notes payable 75,000 Accounts receivable 153,000 Interest expense 4,750 Accrued expenses 7,900 Common stock 289,000 Cost of goods sold 297,000 Inventory 99,300 Taxes 50,500 Accumulated depreciation 263,000 Prepaid expenses 14,500 Taxes payable 53,000 Retained earnings 262,900 ||arrow_forward
- x3-3. (Preparing an income statement) Prepare an income statement and a common- sized income statement from the following information. MyLab Sales Cost of goods sold General and administrative expenses Depreciation expenses Interest expense Income taxes $525,000 200,000 62,000 8,000 12,000 97,200arrow_forward3-9. (Working with a statement of cash flows) Given the following information, prepare LO3 a statement of cash flows. Increase in accounts receivable Increase in inventories Operating income Interest expense Increase in accounts payable Dividends $25 30 75 25 25 15 20 Increase in net fixed assets 23 Depreciation expense Income taxes 12 17 Beginning cash 20 Increase in common stockarrow_forward3-4. (Preparing a balance sheet) Prepare a balance sheet from the following informa- LO2 tion. What is the net working capital and debt ratio? Cash $50,000 Account receivables 42,700 Accounts payable 23,000 Short-term notes payable 10,500 Inventories 40,000 Gross fixed assets 1,280,000 Other current assets 5,000 Long-term debt 200,000 Common stock 490,000 Other assets 15,000 Accumulated depreciation 312,000 Retained earnings ? MyLabarrow_forward
- Consider a situation involving determining right and wrong. Do you believe utilitarianism provides a more objective viewpoint than moral rights in this context? Why or why not? How about when comparing utilitarianism to principles of justice? Share your thoughts. Reflect on this statement: "Every principle of distributive justice, whether that of the egalitarian, the capitalist, the socialist, the libertarian, or Rawls, in the end is illegitimately advocating some type of equality." Do you agree or disagree with this assertion? Why might someone claim this, and how would you respond?arrow_forwardI need help checking my spreadsheet. Q: Assume that Temp Force’s dividend is expected to experience supernormal growth of 73%from Year 0 to Year 1, 47% from Year 1 to Year 2, 32% from Year 2 to Year 3 and 21% from year3 to year 4. After Year 4, dividends will grow at a constant rate of 2.75%. What is the stock’sintrinsic value under these conditions? What are the expected dividend yield and capital gainsyield during the first year? What are the expected dividend yield and capital gains yield duringthe fifth year (from Year 4 to Year 5)?arrow_forwardwhat are the five components of case study design? Please help explain with examplesarrow_forward
Intermediate Financial Management (MindTap Course...FinanceISBN:9781337395083Author:Eugene F. Brigham, Phillip R. DavesPublisher:Cengage Learning
EBK CONTEMPORARY FINANCIAL MANAGEMENTFinanceISBN:9781337514835Author:MOYERPublisher:CENGAGE LEARNING - CONSIGNMENT