EBK INTRODUCTION TO THE PRACTICE OF STA
9th Edition
ISBN: 8220103674638
Author: Moore
Publisher: YUZU
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Question
Chapter 2, Problem 144E
(a)
To determine
To graph: The
(a)
Expert Solution
Explanation of Solution
Graph: To draw the scatterplot for the provided data set, the below steps are followed in Minitab software.
Step 1: Open the “MEIS” file in Minitab software.
Step 2: Go to Graph
Step 3: Choose the option “Simple” and click “OK”.
Step 4: Select “Sales” as Y variables and “Dwellpermit” as X variables.
Step 5: Click “OK” twice.
The scatterplot is obtained as:
To determine
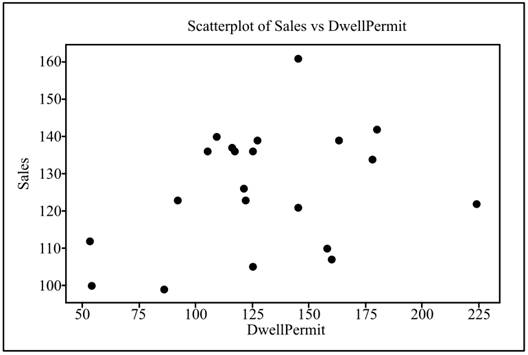
To explain: The relationship between the variables and the presence of the outliers.
Expert Solution
Answer to Problem 144E
Solution: Though the relationship between the variables is very weak, but there is no outlier in the data set.
Explanation of Solution
The scatterplot obtained in part (a) shows that the data are very much scattered. That is there is very weak relationship between the response variable sales and the explanatory variable, issued permits. But there is no outlier present in the data set.
(b)
To determine
To find: The least-squares regression line for the data.
(b)
Expert Solution
Answer to Problem 144E
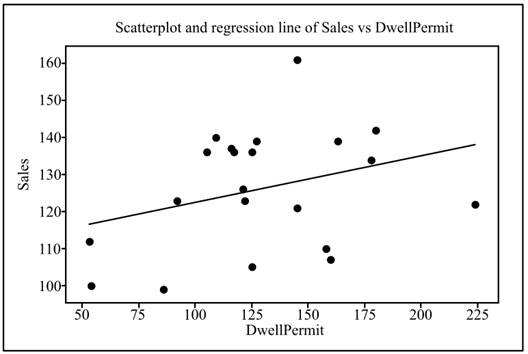
Solution: The least-squares regression line is obtained as
Explanation of Solution
Calculation: To draw the regression line and obtain the sketch of the regression line, the below steps are followed in the Minitab software.
Step 1: Right click on the obtained graph in the part (a).
Step 2: Go to Add
Step 3: Click on “Linear” under the menu “Model Order” and tick the “Fit intercept”.
Step 4: Click on “OK” to obtain the regression line.
The obtained regression equation is
Graph: The below graph shows the regression line.
(c)
To determine
To explain: The slope of the obtained line.
(c)
Expert Solution
Answer to Problem 144E
Solution: The slope can be interpreted as if there is an increase of 1unit of the variable, issued permit for dwelling then there is an increase of 0.1263 units in the value of the variable sales.
Explanation of Solution
The slope of the least-square regression line indicates the amount of increase (decrease) in the response variable due to the 1-unit increase (decrease) in the value of the explanatory variable. In the provided equation, the amount of increase in the value of the response variable, sales is 0.1263 due to the 1-unit increase in the value of the variable issued permit for dwelling.
(d)
To determine
To explain: The intercept of the line.
(d)
Expert Solution
Answer to Problem 144E
Solution: The intercept can be interpreted as the value of the response variable when the value of the explanatory variable is zero. As the value of the response variable is 109.8 for the zero issued permits, it is appropriate to use the intercept for explaining the relationship between the variables.
Explanation of Solution
The intercept of the least-squares linear regression provided the value of the response variable when the value of the explanatory variable is zero. The explanatory variable can be zero because the index of issued permits for new dwelling can be zero and the value of the response variable for zero issued permit is 109.8. Therefore, it is appropriate to use the intercept for explaining the relationship between the variables.
(e)
To determine
The sales for an index of 224 dwelling permits.
(e)
Expert Solution
Answer to Problem 144E
Solution: The predicted value of the sales is 138.0912.
Explanation of Solution
The predicted value of the sales can be obtained by substituting the value of the index of dwelling permit in the least-squares linear regression equation.
The predicted value can be calculated as:
(f)
To determine
To find: The residual value for Canada.
(f)
Expert Solution
Answer to Problem 144E
Solution: The obtained value of the residual is
Explanation of Solution
Calculation: The sale for the Canada is provided as 122. The residual value for the sales for Canada whose index of dwelling permits is 224 is calculated as:
(g)
To determine
The percentage of variability in sales is explained by dwelling permit.
(g)
Expert Solution
Answer to Problem 144E
Solution: The explained percentage of variability in sales is 10.30%.
Explanation of Solution
The variability is explained by r 2 r 2
Therefore, the proportion of variation that is explained by the explanatory variables of the model is 10.30%.
Want to see more full solutions like this?
Subscribe now to access step-by-step solutions to millions of textbook problems written by subject matter experts!
Students have asked these similar questions
0|0|0|0
-
Consider the time series X₁ and Y₁ = (I – B)² (I – B³)Xt. What transformations were
performed on Xt to obtain Yt?
seasonal difference of order 2
simple difference of order 5
seasonal difference of order 1
seasonal difference of order 5
simple difference of order 2
Calculate the 90% confidence interval for the population mean difference using the data in the attached image. I need to see where I went wrong.
Microsoft Excel snapshot for random sampling: Also note the formula used for the last
column
02
x✓ fx =INDEX(5852:58551, RANK(C2, $C$2:$C$51))
A
B
1
No.
States
2
1
ALABAMA
Rand No.
0.925957526
3
2
ALASKA
0.372999976
4
3
ARIZONA
0.941323044
5
4 ARKANSAS
0.071266381
Random Sample
CALIFORNIA
NORTH CAROLINA
ARKANSAS
WASHINGTON
G7
Microsoft Excel snapshot for systematic sampling:
xfx INDEX(SD52:50551, F7)
A
B
E
F
G
1
No.
States
Rand No. Random Sample
population
50
2
1 ALABAMA
0.5296685 NEW HAMPSHIRE
sample
10
3
2 ALASKA
0.4493186 OKLAHOMA
k
5
4
3 ARIZONA
0.707914 KANSAS
5
4 ARKANSAS 0.4831379 NORTH DAKOTA
6
5 CALIFORNIA 0.7277162 INDIANA
Random Sample
Sample Name
7
6 COLORADO 0.5865002 MISSISSIPPI
8
7:ONNECTICU 0.7640596 ILLINOIS
9
8 DELAWARE 0.5783029 MISSOURI
525
10
15
INDIANA
MARYLAND
COLORADO
Chapter 2 Solutions
EBK INTRODUCTION TO THE PRACTICE OF STA
Ch. 2.1 - Prob. 1UYKCh. 2.1 - Prob. 2UYKCh. 2.1 - Prob. 3UYKCh. 2.1 - Prob. 4UYKCh. 2.1 - Prob. 5ECh. 2.1 - Prob. 6ECh. 2.1 - Prob. 7ECh. 2.1 - Prob. 8ECh. 2.1 - Prob. 9ECh. 2.2 - Prob. 10UYK
Ch. 2.2 - Prob. 11UYKCh. 2.2 - Prob. 12UYKCh. 2.2 - Prob. 13UYKCh. 2.2 - Prob. 14UYKCh. 2.2 - Prob. 15UYKCh. 2.2 - Prob. 16ECh. 2.2 - Prob. 17ECh. 2.2 - Prob. 18ECh. 2.2 - Prob. 19ECh. 2.2 - Prob. 20ECh. 2.2 - Prob. 21ECh. 2.2 - Prob. 22ECh. 2.2 - Prob. 23ECh. 2.2 - Prob. 24ECh. 2.2 - Prob. 25ECh. 2.2 - Prob. 26ECh. 2.2 - Prob. 27ECh. 2.2 - Prob. 28ECh. 2.2 - Prob. 29ECh. 2.2 - Prob. 30ECh. 2.2 - Prob. 31ECh. 2.2 - Prob. 32ECh. 2.2 - Prob. 33ECh. 2.2 - Prob. 34ECh. 2.2 - Prob. 35ECh. 2.2 - Prob. 36ECh. 2.2 - Prob. 37ECh. 2.3 - Prob. 38UYKCh. 2.3 - Prob. 39UYKCh. 2.3 - Prob. 40ECh. 2.3 - Prob. 41ECh. 2.3 - Prob. 42ECh. 2.3 - Prob. 43ECh. 2.3 - Prob. 44ECh. 2.3 - Prob. 45ECh. 2.3 - Prob. 46ECh. 2.3 - Prob. 47ECh. 2.3 - Prob. 48ECh. 2.3 - Prob. 49ECh. 2.3 - Prob. 50ECh. 2.3 - Prob. 51ECh. 2.3 - Prob. 52ECh. 2.3 - Prob. 53ECh. 2.3 - Prob. 54ECh. 2.3 - Prob. 55ECh. 2.3 - Prob. 56ECh. 2.3 - Prob. 57ECh. 2.3 - Prob. 58ECh. 2.3 - Prob. 59ECh. 2.3 - Prob. 60ECh. 2.4 - Prob. 61UYKCh. 2.4 - Prob. 62UYKCh. 2.4 - Prob. 63UYKCh. 2.4 - Prob. 64UYKCh. 2.4 - Prob. 65ECh. 2.4 - Prob. 66ECh. 2.4 - Prob. 67ECh. 2.4 - Prob. 68ECh. 2.4 - Prob. 69ECh. 2.4 - Prob. 70ECh. 2.4 - Prob. 71ECh. 2.4 - Prob. 72ECh. 2.4 - Prob. 73ECh. 2.4 - Prob. 74ECh. 2.4 - Prob. 75ECh. 2.4 - Prob. 76ECh. 2.4 - Prob. 77ECh. 2.4 - Prob. 78ECh. 2.4 - Prob. 79ECh. 2.4 - Prob. 80ECh. 2.4 - Prob. 81ECh. 2.4 - Prob. 82ECh. 2.4 - Prob. 83ECh. 2.4 - Prob. 84ECh. 2.4 - Prob. 85ECh. 2.4 - Prob. 86ECh. 2.4 - Prob. 87ECh. 2.4 - Prob. 88ECh. 2.4 - Prob. 89ECh. 2.4 - Prob. 90ECh. 2.4 - Prob. 91ECh. 2.5 - Prob. 92UYKCh. 2.5 - Prob. 93UYKCh. 2.5 - Prob. 94ECh. 2.5 - Prob. 95ECh. 2.5 - Prob. 96ECh. 2.5 - Prob. 97ECh. 2.5 - Prob. 98ECh. 2.5 - Prob. 99ECh. 2.5 - Prob. 100ECh. 2.5 - Prob. 101ECh. 2.5 - Prob. 102ECh. 2.5 - Prob. 103ECh. 2.5 - Prob. 104ECh. 2.5 - Prob. 105ECh. 2.5 - Prob. 106ECh. 2.5 - Prob. 107ECh. 2.5 - Prob. 108ECh. 2.5 - Prob. 110ECh. 2.5 - Prob. 111ECh. 2.5 - Prob. 112ECh. 2.6 - Prob. 113UYKCh. 2.6 - Prob. 114UYKCh. 2.6 - Prob. 115UYKCh. 2.6 - Prob. 116UYKCh. 2.6 - Prob. 117UYKCh. 2.6 - Prob. 118UYKCh. 2.6 - Prob. 119ECh. 2.6 - Prob. 120ECh. 2.6 - Prob. 121ECh. 2.6 - Prob. 122ECh. 2.6 - Prob. 123ECh. 2.6 - Prob. 124ECh. 2.6 - Prob. 125ECh. 2.6 - Prob. 126ECh. 2.6 - Prob. 127ECh. 2.6 - Prob. 128ECh. 2.6 - Prob. 129ECh. 2.6 - Prob. 130ECh. 2.7 - Prob. 131ECh. 2.7 - Prob. 132ECh. 2.7 - Prob. 133ECh. 2.7 - Prob. 134ECh. 2.7 - Prob. 135ECh. 2.7 - Prob. 136ECh. 2.7 - Prob. 137ECh. 2.7 - Prob. 138ECh. 2.7 - Prob. 139ECh. 2.7 - Prob. 140ECh. 2.7 - Prob. 141ECh. 2.7 - Prob. 142ECh. 2.7 - Prob. 143ECh. 2 - Prob. 144ECh. 2 - Prob. 145ECh. 2 - Prob. 146ECh. 2 - Prob. 147ECh. 2 - Prob. 148ECh. 2 - Prob. 149ECh. 2 - Prob. 150ECh. 2 - Prob. 151ECh. 2 - Prob. 152ECh. 2 - Prob. 153ECh. 2 - Prob. 154ECh. 2 - Prob. 155ECh. 2 - Prob. 156ECh. 2 - Prob. 157ECh. 2 - Prob. 158ECh. 2 - Prob. 159ECh. 2 - Prob. 160ECh. 2 - Prob. 161ECh. 2 - Prob. 162ECh. 2 - Prob. 163ECh. 2 - Prob. 164ECh. 2 - Prob. 165ECh. 2 - Prob. 166ECh. 2 - Prob. 167ECh. 2 - Prob. 168ECh. 2 - Prob. 169ECh. 2 - Prob. 170ECh. 2 - Prob. 171ECh. 2 - Prob. 172ECh. 2 - Prob. 173ECh. 2 - Prob. 174ECh. 2 - Prob. 175ECh. 2 - Prob. 176E
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Similar questions
- Suppose the Internal Revenue Service reported that the mean tax refund for the year 2022 was $3401. Assume the standard deviation is $82.5 and that the amounts refunded follow a normal probability distribution. Solve the following three parts? (For the answer to question 14, 15, and 16, start with making a bell curve. Identify on the bell curve where is mean, X, and area(s) to be determined. 1.What percent of the refunds are more than $3,500? 2. What percent of the refunds are more than $3500 but less than $3579? 3. What percent of the refunds are more than $3325 but less than $3579?arrow_forwardA normal distribution has a mean of 50 and a standard deviation of 4. Solve the following three parts? 1. Compute the probability of a value between 44.0 and 55.0. (The question requires finding probability value between 44 and 55. Solve it in 3 steps. In the first step, use the above formula and x = 44, calculate probability value. In the second step repeat the first step with the only difference that x=55. In the third step, subtract the answer of the first part from the answer of the second part.) 2. Compute the probability of a value greater than 55.0. Use the same formula, x=55 and subtract the answer from 1. 3. Compute the probability of a value between 52.0 and 55.0. (The question requires finding probability value between 52 and 55. Solve it in 3 steps. In the first step, use the above formula and x = 52, calculate probability value. In the second step repeat the first step with the only difference that x=55. In the third step, subtract the answer of the first part from the…arrow_forwardIf a uniform distribution is defined over the interval from 6 to 10, then answer the followings: What is the mean of this uniform distribution? Show that the probability of any value between 6 and 10 is equal to 1.0 Find the probability of a value more than 7. Find the probability of a value between 7 and 9. The closing price of Schnur Sporting Goods Inc. common stock is uniformly distributed between $20 and $30 per share. What is the probability that the stock price will be: More than $27? Less than or equal to $24? The April rainfall in Flagstaff, Arizona, follows a uniform distribution between 0.5 and 3.00 inches. What is the mean amount of rainfall for the month? What is the probability of less than an inch of rain for the month? What is the probability of exactly 1.00 inch of rain? What is the probability of more than 1.50 inches of rain for the month? The best way to solve this problem is begin by a step by step creating a chart. Clearly mark the range, identifying the…arrow_forward
- Client 1 Weight before diet (pounds) Weight after diet (pounds) 128 120 2 131 123 3 140 141 4 178 170 5 121 118 6 136 136 7 118 121 8 136 127arrow_forwardClient 1 Weight before diet (pounds) Weight after diet (pounds) 128 120 2 131 123 3 140 141 4 178 170 5 121 118 6 136 136 7 118 121 8 136 127 a) Determine the mean change in patient weight from before to after the diet (after – before). What is the 95% confidence interval of this mean difference?arrow_forwardIn order to find probability, you can use this formula in Microsoft Excel: The best way to understand and solve these problems is by first drawing a bell curve and marking key points such as x, the mean, and the areas of interest. Once marked on the bell curve, figure out what calculations are needed to find the area of interest. =NORM.DIST(x, Mean, Standard Dev., TRUE). When the question mentions “greater than” you may have to subtract your answer from 1. When the question mentions “between (two values)”, you need to do separate calculation for both values and then subtract their results to get the answer. 1. Compute the probability of a value between 44.0 and 55.0. (The question requires finding probability value between 44 and 55. Solve it in 3 steps. In the first step, use the above formula and x = 44, calculate probability value. In the second step repeat the first step with the only difference that x=55. In the third step, subtract the answer of the first part from the…arrow_forward
- If a uniform distribution is defined over the interval from 6 to 10, then answer the followings: What is the mean of this uniform distribution? Show that the probability of any value between 6 and 10 is equal to 1.0 Find the probability of a value more than 7. Find the probability of a value between 7 and 9. The closing price of Schnur Sporting Goods Inc. common stock is uniformly distributed between $20 and $30 per share. What is the probability that the stock price will be: More than $27? Less than or equal to $24? The April rainfall in Flagstaff, Arizona, follows a uniform distribution between 0.5 and 3.00 inches. What is the mean amount of rainfall for the month? What is the probability of less than an inch of rain for the month? What is the probability of exactly 1.00 inch of rain? What is the probability of more than 1.50 inches of rain for the month? The best way to solve this problem is begin by creating a chart. Clearly mark the range, identifying the lower and upper…arrow_forwardProblem 1: The mean hourly pay of an American Airlines flight attendant is normally distributed with a mean of 40 per hour and a standard deviation of 3.00 per hour. What is the probability that the hourly pay of a randomly selected flight attendant is: Between the mean and $45 per hour? More than $45 per hour? Less than $32 per hour? Problem 2: The mean of a normal probability distribution is 400 pounds. The standard deviation is 10 pounds. What is the area between 415 pounds and the mean of 400 pounds? What is the area between the mean and 395 pounds? What is the probability of randomly selecting a value less than 395 pounds? Problem 3: In New York State, the mean salary for high school teachers in 2022 was 81,410 with a standard deviation of 9,500. Only Alaska’s mean salary was higher. Assume New York’s state salaries follow a normal distribution. What percent of New York State high school teachers earn between 70,000 and 75,000? What percent of New York State high school…arrow_forwardPls help asaparrow_forward
- Solve the following LP problem using the Extreme Point Theorem: Subject to: Maximize Z-6+4y 2+y≤8 2x + y ≤10 2,y20 Solve it using the graphical method. Guidelines for preparation for the teacher's questions: Understand the basics of Linear Programming (LP) 1. Know how to formulate an LP model. 2. Be able to identify decision variables, objective functions, and constraints. Be comfortable with graphical solutions 3. Know how to plot feasible regions and find extreme points. 4. Understand how constraints affect the solution space. Understand the Extreme Point Theorem 5. Know why solutions always occur at extreme points. 6. Be able to explain how optimization changes with different constraints. Think about real-world implications 7. Consider how removing or modifying constraints affects the solution. 8. Be prepared to explain why LP problems are used in business, economics, and operations research.arrow_forwardged the variance for group 1) Different groups of male stalk-eyed flies were raised on different diets: a high nutrient corn diet vs. a low nutrient cotton wool diet. Investigators wanted to see if diet quality influenced eye-stalk length. They obtained the following data: d Diet Sample Mean Eye-stalk Length Variance in Eye-stalk d size, n (mm) Length (mm²) Corn (group 1) 21 2.05 0.0558 Cotton (group 2) 24 1.54 0.0812 =205-1.54-05T a) Construct a 95% confidence interval for the difference in mean eye-stalk length between the two diets (e.g., use group 1 - group 2).arrow_forwardAn article in Business Week discussed the large spread between the federal funds rate and the average credit card rate. The table below is a frequency distribution of the credit card rate charged by the top 100 issuers. Credit Card Rates Credit Card Rate Frequency 18% -23% 19 17% -17.9% 16 16% -16.9% 31 15% -15.9% 26 14% -14.9% Copy Data 8 Step 1 of 2: Calculate the average credit card rate charged by the top 100 issuers based on the frequency distribution. Round your answer to two decimal places.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
MATLAB: An Introduction with Applications
Statistics
ISBN:9781119256830
Author:Amos Gilat
Publisher:John Wiley & Sons Inc
Probability and Statistics for Engineering and th...
Statistics
ISBN:9781305251809
Author:Jay L. Devore
Publisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...
Statistics
ISBN:9781305504912
Author:Frederick J Gravetter, Larry B. Wallnau
Publisher:Cengage Learning
Elementary Statistics: Picturing the World (7th E...
Statistics
ISBN:9780134683416
Author:Ron Larson, Betsy Farber
Publisher:PEARSON
The Basic Practice of Statistics
Statistics
ISBN:9781319042578
Author:David S. Moore, William I. Notz, Michael A. Fligner
Publisher:W. H. Freeman
Introduction to the Practice of Statistics
Statistics
ISBN:9781319013387
Author:David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:W. H. Freeman
The Shape of Data: Distributions: Crash Course Statistics #7; Author: CrashCourse;https://www.youtube.com/watch?v=bPFNxD3Yg6U;License: Standard YouTube License, CC-BY
Shape, Center, and Spread - Module 20.2 (Part 1); Author: Mrmathblog;https://www.youtube.com/watch?v=COaid7O_Gag;License: Standard YouTube License, CC-BY
Shape, Center and Spread; Author: Emily Murdock;https://www.youtube.com/watch?v=_YyW0DSCzpM;License: Standard Youtube License