Chapter 18, Problem 33CE

Ray Anderson, owner of Anderson Ski Lodge in upstate New York, is interested in forecasting the number of visitors for the upcoming year. The following data are available, by quarter, from the first quarter of 2007 to the fourth quarter of 2013. Develop a seasonal index for each quarter. How many visitors would you expect for each quarter of 2014, if Ray projects that there will be a 10% increase from the total number of visitors in 2013? Determine the trend equation, project the number of visitors for 2014, and seasonally adjust the forecast. Which forecast would you choose?

To determine

Obtain a seasonal index for each of the four quarters.

Find the number of visitors expected for each quarters of 2014 if there is 10% increase in the total number of visitors in 2013.

Obtain the trend equation.

Predict the number of visitors for 2014.

Find the seasonally adjusted forecasts.

Identify the best forecast.

Answer to Problem 33CE

The seasonal indexes for the four quarters are 1.2046, 1.0206, 0.6297, and 01.1451.

The number of visitors for each quarter of 2017 if there is 10% increase in the total number of visitors in 2013 is 255.25 visitors per quarter.

The trend equation is $\widehat{Y}=50.127+6.617t$.

The number of visitors for 2017 are 242.0171, 248.634, 255.2509, and 261.8678.

The seasonally adjusted forecasts are 291.5338, 253.7559, 160.7315, and 299.8648.

The best forecast is the fourth quarter of 2017.

Explanation of Solution

Four-year moving average:

$\text{Four-year moving average}=\frac{\text{sum of the four concequent Visitors}}{4}$.

Centered moving average:

$\text{Centered moving average}=\frac{\text{sum of the two concequent moving averages}}{2}$.

Specific seasonal index:

$\text{Specific seasonal index}=\frac{\text{Visitors}}{\text{Centered moving average}}$

Year	Quarter	Visitors	Four-quarter moving average	Centered Moving average	Specific seasonal
2007	1	86
	2	62
	3	28		70	0.4
	4	94	67.5	75	1.253333
2008	1	106	72.5	80	1.325
	2	82	77.5	85	0.964706
	3	48	82.5	91.75	0.523161
	4	114	87.5	100.75	1.131514
2009	1	140	96	109.75	1.275626
	2	120	105.5	119	1.008403
	3	82	114	126.75	0.646943
	4	154	124	132	1.166667
2010	1	162	129.5	136.75	1.184644
	2	140	134.5	141.5	0.989399
	3	100	139	147.25	0.679117
	4	174	144	154.5	1.126214
2011	1	188	150.5	162	1.160494
	2	172	158.5	168.5	1.020772
	3	128	165.5	174	0.735632
	4	198	171.5	180.25	1.098474
2012	1	208	176.5	187.25	1.110814
	2	202	184	193.25	1.045278
	3	154	190.5	200.75	0.767123
	4	220	196	210.25	1.046373
2013	1	246	205.5	219.5	1.120729
	2	240	215	228	1.052632
	3	190	224
	4	252	232

The quarterly indexes are as follows:

	I	II	III	IV
2007			0.4	1.253333
2008	1.325	0.964706	0.523161	1.131514
2009	1.275626	1.008403	0.646943	1.166667
2010	1.184644	0.989399	0.679117	1.126214
2011	1.160494	1.020772	0.735632	1.098474
2012	1.110814	1.045278	0.767123	1.046373
2013	1.120729	1.052632
Mean	1.1962	1.0135	0.6253	1.1371

Seasonal index:

$\text{Seasonal Index}=\text{Mean of the quarter}\times \text{Correction Factor}$

Here, $\text{Correction Factor}=\frac{4}{\text{Sum of the means of the quarters}}$.

Therefore, the following is obtained:

$\begin{array}{c}\text{Correction Factor}=\frac{4}{\text{1}\text{.1962+1}\text{.0135+0}\text{.6253+1}\text{.1371}}\\ =\frac{4}{3.9721}\\ =1.00703\end{array}$

The seasonal indexes are as follows:

	I	II	III	IV
2007			0.4	1.253333
2008	1.325	0.964706	0.523161	1.131514
2009	1.275626	1.008403	0.646943	1.166667
2010	1.184644	0.989399	0.679117	1.126214
2011	1.160494	1.020772	0.735632	1.098474
2012	1.110814	1.045278	0.767123	1.046373
2013	1.120729	1.052632
Mean	1.1962	1.0135	0.6253	1.1371
Seasonal Index	$\begin{array}{l}1.1962\times 1.00703\\ =1.2046\end{array}$	$\begin{array}{l}1.0135\times 1.00703\\ =1.0206\end{array}$	$\begin{array}{l}0.6253\times 1.00703\\ =0.6297\end{array}$	$\begin{array}{l}1.1371\times 1.00703\\ =1.1451\end{array}$

The total number of visitors in the year 2013 is $928\left(=246+240+190+252\right)$.

The 10% of 928 visitors is $\approx 93\left(928\times 0.10=92.8\right)$.

The number of visitors in the year 2017 is $1,021\left(=928+93\right)$.

Therefore, the number of visitors in each quarter of 2017 is $\frac{1,021}{4}=255.25$.

Trend equation:

Step-by-step procedure to obtain the regression using the Excel:

• Enter the data for Year, Visitors and t in Excel sheet.
• Go to Data Menu.
• Click on Data Analysis.
• Select Regression and click on OK.
• Select the column of Visitors under Input Y Range.
• Select the column of t under Input X Range.
• Click on OK.

Output for the regression obtained using the Excel is as follows:

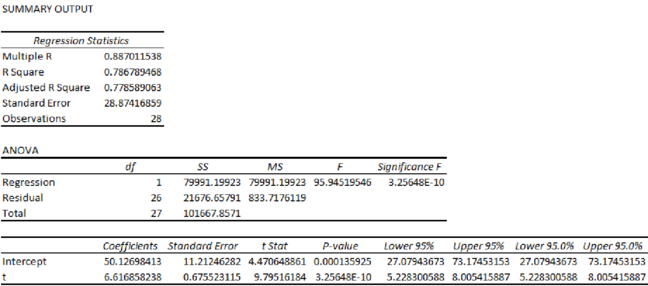
From the output, the regression equation is $\widehat{Y}=50.127+6.617t$.

Projection of the number of visitors for 2017:

The t value for the first quarter of 2014 is 29.

$\begin{array}{c}\widehat{Y}=50.127+\left(6.617\times 29\right)\\ =242.0171\end{array}$

The t value for the second quarter of 2014 is 30.

$\begin{array}{c}\widehat{Y}=50.127+\left(6.617\times 30\right)\\ =248.634\end{array}$

The t value for the third quarter of 2014 is 31.

$\begin{array}{c}\widehat{Y}=50.127+\left(6.617\times 31\right)\\ =255.2509\end{array}$

The t value for the fourth quarter of 2014 is 32.

$\begin{array}{c}\widehat{Y}=50.127+\left(6.617\times 32\right)\\ =261.8678\end{array}$

Seasonally adjusted forecast:

Estimated Visitors	Seasonal Index	$\text{Forecast}=\text{Estimated Visitors}\times \text{Seasonal Index}$
242.0171	1.2046	291.5338
248.634	1.0206	253.7559
255.2509	0.6297	160.7315
261.8678	1.1451	299.8648

The seasonal index for the fourth quarter is high when compared to the remaining three quarters. Hence, the forecast for the fourth quarter is the best.