
Concept explainers
(a)
The range ofwavenumber.
(a)
Answer to Problem 67P
Explanation of Solution
Introduction:
A sound wave form originated by tuning fork of frequency centered on
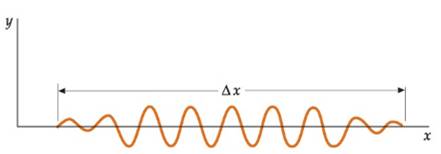
Figure 1:A sound waveform created by a tuning fork
Basically, the wave packet is the result of the superposition of the two or more waves, hence, the resultant of the super-position of the wave results in the spread of frequencies,
Let say, the v is the speed of the pulse. Therefore, the pulse spread in space can be written as
The range of frequencies
As it is well known relation between the velocity, frequency and the wave number is
Putting the value of v from equation 1 in equation 2,
Comparing equation 1 and 4, we get
k is the wave number.
Conclusion:
The wave number range is
(b)
The average value of the wavelength.
(b)
Answer to Problem 67P
Explanation of Solution
Introduction:
A wavelength
N number of cycles are there in a wave packet of length
The distance for one cycle is basically equal to the wavelength and will be
Hence, one can write
Conclusion:
The average wavelength will be
(c)
The average value of the wave number k
(c)
Answer to Problem 67P
Explanation of Solution
Introduction:
As calculated before:
N number of cycles are there in a wave packet of length
The distance for one cycle is basically equal to the wavelength and will be
Hence,
The relation between
From equation 3:
Conclusion:
The average value of the wave number kis
(d)
Therange in angular frequencies.
(d)
Answer to Problem 67P
Explanation of Solution
Introduction:
A sound wave form originated by tuning fork of frequency centered on
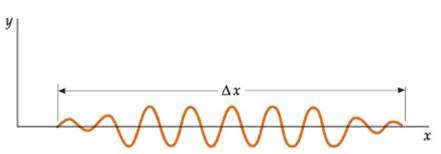
Figure 1:A sound waveform created by a tuning fork
Basically, the wave packet is the result of the superposition of the two or more waves, hence, the resultant of the super-position of the wave results in the spread of frequencies,
The relation between the range of frequencies
Conclusion:
The range in angular frequencies
(e)
The frequency in terms of N and
(e)
Answer to Problem 67P
Explanation of Solution
Introduction:
A sound wave form originated by tuning fork of frequency centered on
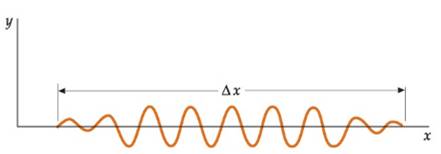
Figure 1:A sound waveform created by a tuning fork
Basically, the wave packet is the result of the superposition of the two or more waves, hence, the resultant of the super-position of the wave results in the spread of frequencies,
N number of cycles are there in a wave packet of length
The time required to complete one cycle is basically equal to the frequency and will be
Hence, one can write
Conclusion:
The frequency will be
(f)
The uncertainty in N.
(f)
Answer to Problem 67P
There is an uncertainty of
Explanation of Solution
Introduction:
A sound wave form originated by tuning fork of frequency centered on
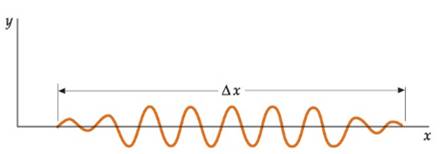
Figure 1:A sound waveform created by a tuning fork
Basically, the wave packet is the result of the superposition of the two or more waves, hence, the resultant of the super-position of the wave results in the spread of frequencies,
As it is clear from the Figure 1, there is a cycle which is not a complete one. Hence, the cycle can be either not present or may be present in the wave packet.
Therefore, there is an error or uncertainty of
Conclusion:
There is an uncertainty of
(g)
The uncertainty in wave number k
(g)
Answer to Problem 67P
Explanation of Solution
Introduction:
A sound wave form originated by tuning fork of frequency centered on
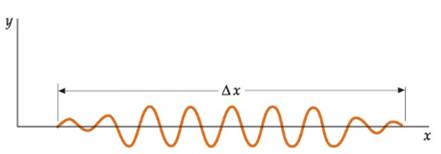
Figure 1:A sound waveform created by a tuning fork
Basically, the wave packet is the result of the superposition of the two or more waves, hence, the resultant of the super-position of the wave results in the spread of frequencies,
As calculated before:
N number of cycles are there in a wave packet of length
The distance for one cycle is basically equal to the wavelength and will be
Hence, we can write
The relation between
From equation 3 we obtain
As calculated by the previous section that the uncertainty in N is
Conclusion:
The uncertainty in k will be,
Want to see more full solutions like this?
Chapter 16 Solutions
Physics for Scientists and Engineers
- = A hanging weight, with a mass of m₁ = 0.365 kg, is attached by a rope to a block with mass m₂ 0.835 kg as shown in the figure below. The rope goes over a pulley with a mass of M = 0.350 kg. The pulley can be modeled as a hollow cylinder with an inner radius of R₁ = 0.0200 m, and an outer radius of R2 = 0.0300 m; the mass of the spokes is negligible. As the weight falls, the block slides on the table, and the coefficient of kinetic friction between the block and the table is μk = 0.250. At the instant shown, the block is moving with a velocity of v; = 0.820 m/s toward the pulley. Assume that the pulley is free to spin without friction, that the rope does not stretch and does not slip on the pulley, and that the mass of the rope is negligible. mq R₂ R₁ mi (a) Using energy methods, find the speed of the block (in m/s) after it has moved a distance of 0.700 m away from the initial position shown. m/s (b) What is the angular speed of the pulley (in rad/s) after the block has moved this…arrow_forwardno AI, pleasearrow_forwardno AI, pleasearrow_forward
- no AI, pleasearrow_forwardTwo astronauts, each having a mass of 95.5 kg, are connected by a 10.0-m rope of negligible mass. They are isolated in space, moving in circles around the point halfway between them at a speed of 4.60 m/s. Treating the astronauts as particles, calculate each of the following. CG × d (a) the magnitude of the angular momentum of the system kg m2/s (b) the rotational energy of the system KJ By pulling on the rope, the astronauts shorten the distance between them to 5.00 m. (c) What is the new angular momentum of the system? kg m2/s (d) What are their new speeds? m/s (e) What is the new rotational energy of the system? KJ (f) How much work is done by the astronauts in shortening the rope? KJarrow_forwardA uniform horizontal disk of radius 5.50 m turns without friction at w = 2.55 rev/s on a vertical axis through its center, as in the figure below. A feedback mechanism senses the angular speed of the disk, and a drive motor at A ensures that the angular speed remain constant while a m = 1.20 kg block on top of the disk slides outward in a radial slot. The block starts at the center of the disk at time t = 0 and moves outward with constant speed v = 1.25 cm/s relative to the disk until it reaches the edge at t = 360 s. The sliding block experiences no friction. Its motion is constrained to have constant radial speed by a brake at B, producing tension in a light string tied to the block. (a) Find the torque as a function of time that the drive motor must provide while the block is sliding. Hint: The torque is given by t = 2mrvw. t N.m (b) Find the value of this torque at t = 360 s, just before the sliding block finishes its motion. N.m (c) Find the power which the drive motor must…arrow_forward
- (a) A planet is in an elliptical orbit around a distant star. At its closest approach, the planet is 0.670 AU from the star and has a speed of 54.0 km/s. When the planet is at its farthest distance from the star of 36.0 AU, what is its speed (in km/s)? (1 AU is the average distance from the Earth to the Sun and is equal to 1.496 × 1011 m. You may assume that other planets and smaller objects in the star system exert negligible forces on the planet.) km/s (b) What If? A comet is in a highly elliptical orbit around the same star. The comet's greatest distance from the star is 25,700 times larger than its closest distance to the star. The comet's speed at its greatest distance is 2.40 x 10-2 km/s. What is the speed (in km/s) of the comet at its closest approach? km/sarrow_forwardYou are attending a county fair with your friend from your physics class. While walking around the fairgrounds, you discover a new game of skill. A thin rod of mass M = 0.505 kg and length = 2.70 m hangs from a friction-free pivot at its upper end as shown in the figure. Pivot Velcro M Incoming Velcro-covered ball m The front surface of the rod is covered with Velcro. You are to throw a Velcro-covered ball of mass m = 1.25 kg at the rod in an attempt to make it swing backward and rotate all the way across the top. The ball must stick to the rod at all times after striking it. If you cause the rod to rotate over the top position (that is, rotate 180° opposite of its starting position), you win a stuffed animal. Your friend volunteers to try his luck. He feels that the most torque would be applied to the rod by striking it at its lowest end. While he prepares to aim at the lowest point on the rod, you calculate how fast he must throw the ball to win the stuffed animal with this…arrow_forward56 is not the correct answer!arrow_forward
- 81 SSM Figure 29-84 shows a cross section of an infinite conducting sheet carrying a current per unit x-length of 2; the current emerges perpendicularly out of the page. (a) Use the Biot-Savart law and symmetry to show that for all points B •P x B P'. Figure 29-84 Problem 81. P above the sheet and all points P' below it, the magnetic field B is parallel to the sheet and directed as shown. (b) Use Ampere's law to prove that B = ½µλ at all points P and P'.arrow_forward(λvacuum =640nm) red light (λ vacuum = 640 nm) and green light perpendicularly on a soap film (n=1.31) A mixture of (a vacuum = 512 nm) shines that has air on both side. What is the minimum nonzero thickness of the film, so that destructive interference to look red in reflected light? nm Causes itarrow_forwardSuppose the inteference pattern shown in the figure below is produced by monochromatic light passing through a diffraction grating, that has 260 lines/mm, and onto a screen 1.40m away. What is the wavelength of light if the distance between the dashed lines is 180cm? nmarrow_forward
 University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning
Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College
College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill





