Connect Math hosted by ALEKS Access Card 52 Weeks for Math in Our World
Connect Math hosted by ALEKS Access Card 52 Weeks for Math in Our World
3rd Edition
ISBN: 9781259232848
Author: David Sobecki, Allan Bluman
Publisher: McGraw-Hill Education
bartleby

Concept explainers

Inverse Normal Distribution
The method used for finding the corresponding z-critical value in a normal distribution using the known probability is said to be an inverse normal distribution. The inverse normal distribution is a continuous probability distribution with a family of tw…
Mean, Median, Mode
It is a descriptive summary of a data set. It can be defined by using some of the measures. The central tendencies do not provide information regarding individual data from the dataset. However, they give a summary of the data set. The central tendency o…
Z-Scores
A z-score is a unit of measurement used in statistics to describe the position of a raw score in terms of its distance from the mean, measured with reference to standard deviation from the mean. Z-scores are useful in statistics because they allow compar…
bartleby

Videos

Textbook Question
Book Icon
Chapter 15.1, Problem 61E

Exercises 61–66 use the mathematical system described next.

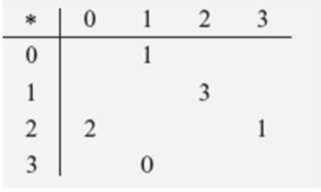
Many ceiling fans have a four-way switch on a pull chain, with positions off, high speed, medium speed, and low speed. We can build a mathematical system to describe the positions using 0 for off, 1 for high speed, 2 for medium speed, and 3 for low speed. For example, if the fan is off and you pull the chain once, it goes to high speed, so if we use + to represent the operation of combining positions by pulling the chain, 0 + 1 = 1. If it’s on medium speed and you pull the chain three times the result is also high speed (medium → low → off → high), so 2 + 3 = 1.

61.    Fill in the operational table we’ve started below for the mathematical system described by the ceiling fan pull chain.

Chapter 15.1, Problem 61E, Exercises 6166 use the mathematical system described next. Many ceiling fans have a four-way switch

Expert Solution & Answer
Check Mark

Want to see the full answer?

Check out a sample textbook solution
Blurred answer
Previous
Chapter 15.1, Problem 60E
Next
Chapter 15.1, Problem 62E
Students have asked these similar questions
I need help with this problem and an explanation of the solution for the image described below. (Statistics: Engineering Probabilities)
12. [-/1 Points] DETAILS MY NOTES SESSCALCET2 6.3.508.XP. ASK YOUR TEA Make a substitution to express the integrand as a rational function and then evaluate the integral. (Remember to use absolute values where appropriate. Use C for the constant of integration.) x + 16 dx X Need Help? Read It SUBMIT ANSWER 13. [-/1 Points] DETAILS MY NOTES SESSCALCET2 6.3.512.XP. ASK YOUR TEA Make a substitution to express the integrand as a rational function and then evaluate the integral. (Remember to use absolute values where appropriate. Use C for the constant of integration.) dx 8)(2x + 1) Need Help? Read It SUBMIT ANSWER 14. [-/1 Points] DETAILS MY NOTES SESSCALCET2 6.3.518.XP. Find the area of the region under the given curve from 1 to 5. y = x² +7 6x - x² Need Help? Read It ASK YOUR TEA
Lakshmi planted 20 begonias, but her neighbor’s dog ate 7 of them. What percent of the begonias did the dog eat?

Chapter 15 Solutions

Connect Math hosted by ALEKS Access Card 52 Weeks for Math in Our World

Ch. 15.1 - Use Table 15-1 to find the result of each...Ch. 15.1 - Which properties does the following system...Ch. 15.1 - Prob. 3TTOCh. 15.1 - Prob. 4TTOCh. 15.1 - Prob. 1ECh. 15.1 - Prob. 2ECh. 15.1 - Prob. 3ECh. 15.1 - Prob. 4ECh. 15.1 - Prob. 5ECh. 15.1 - Prob. 6E
Ch. 15.1 - Prob. 7ECh. 15.1 - Prob. 8ECh. 15.1 - Prob. 9ECh. 15.1 - Prob. 10ECh. 15.1 - Prob. 11ECh. 15.1 - Prob. 12ECh. 15.1 - For Exercises 724, use the elements C, D, E, and...Ch. 15.1 - For Exercises 724, use the elements C, D, E, and...Ch. 15.1 - For Exercises 724, use the elements C, D, E, and...Ch. 15.1 - For Exercises 724, use the elements C, D, E, and...Ch. 15.1 - For Exercises 724, use the elements C, D, E, and...Ch. 15.1 - For Exercises 724, use the elements C, D, E, and...Ch. 15.1 - Prob. 19ECh. 15.1 - Prob. 20ECh. 15.1 - For Exercises 724, use the elements C, D, E, and...Ch. 15.1 - Prob. 22ECh. 15.1 - Prob. 23ECh. 15.1 - Prob. 24ECh. 15.1 - Prob. 25ECh. 15.1 - Prob. 26ECh. 15.1 - Prob. 27ECh. 15.1 - Prob. 28ECh. 15.1 - Prob. 29ECh. 15.1 - For Exercises 2540, use the elements and the...Ch. 15.1 - Prob. 31ECh. 15.1 - Prob. 32ECh. 15.1 - For Exercises 2540, use the elements and the...Ch. 15.1 - For Exercises 2540, use the elements and the...Ch. 15.1 - For Exercises 2540, use the elements and the...Ch. 15.1 - Prob. 36ECh. 15.1 - Prob. 37ECh. 15.1 - Prob. 38ECh. 15.1 - For Exercises 2540, use the elements and the...Ch. 15.1 - Prob. 40ECh. 15.1 - Prob. 41ECh. 15.1 - Prob. 42ECh. 15.1 - Prob. 43ECh. 15.1 - Prob. 44ECh. 15.1 - Prob. 45ECh. 15.1 - Prob. 46ECh. 15.1 - Prob. 47ECh. 15.1 - For Exercises 4150, determine which properties the...Ch. 15.1 - Prob. 49ECh. 15.1 - Prob. 50ECh. 15.1 - Prob. 51ECh. 15.1 - Prob. 52ECh. 15.1 - Prob. 53ECh. 15.1 - Prob. 54ECh. 15.1 - Prob. 55ECh. 15.1 - Prob. 56ECh. 15.1 - For exercises 5160, determine whether the given...Ch. 15.1 - Prob. 58ECh. 15.1 - For exercises 5160, determine whether the given...Ch. 15.1 - Prob. 60ECh. 15.1 - Exercises 6166 use the mathematical system...Ch. 15.1 - Prob. 62ECh. 15.1 - Prob. 63ECh. 15.1 - Prob. 64ECh. 15.1 - Prob. 65ECh. 15.1 - Prob. 66ECh. 15.1 - Prob. 67ECh. 15.1 - Prob. 68ECh. 15.1 - Prob. 69ECh. 15.1 - Prob. 70ECh. 15.1 - Prob. 71ECh. 15.1 - Prob. 72ECh. 15.1 - Prob. 73ECh. 15.1 - Prob. 74ECh. 15.1 - Prob. 75ECh. 15.1 - Prob. 76ECh. 15.1 - Prob. 77ECh. 15.1 - Prob. 78ECh. 15.1 - Prob. 79ECh. 15.1 - Prob. 80ECh. 15.1 - Prob. 81ECh. 15.1 - Prob. 82ECh. 15.1 - Exercises 8184 are based on the classic game of...Ch. 15.1 - Exercises 8184 are based on the classic game of...Ch. 15.1 - Suppose that a mathematical system consists of the...Ch. 15.2 - Using the 12-hour clock, find these sums. (a)3+12...Ch. 15.2 - Prob. 2TTOCh. 15.2 - Prob. 3TTOCh. 15.2 - Prob. 4TTOCh. 15.2 - Prob. 1ECh. 15.2 - Prob. 2ECh. 15.2 - Prob. 3ECh. 15.2 - Prob. 4ECh. 15.2 - Prob. 5ECh. 15.2 - Prob. 6ECh. 15.2 - Prob. 7ECh. 15.2 - Prob. 8ECh. 15.2 - Prob. 9ECh. 15.2 - Prob. 10ECh. 15.2 - Prob. 11ECh. 15.2 - Prob. 12ECh. 15.2 - Prob. 13ECh. 15.2 - Prob. 14ECh. 15.2 - Prob. 15ECh. 15.2 - Prob. 16ECh. 15.2 - For Exercises 920, find the equivalent number on...Ch. 15.2 - For Exercises 920, find the equivalent number on...Ch. 15.2 - Prob. 19ECh. 15.2 - Prob. 20ECh. 15.2 - For Exercises 2130, perform the additions on the...Ch. 15.2 - Prob. 22ECh. 15.2 - For Exercises 2130, perform the additions on the...Ch. 15.2 - Prob. 24ECh. 15.2 - For Exercises 2130, perform the additions on the...Ch. 15.2 - Prob. 26ECh. 15.2 - For Exercises 2130, perform the additions on the...Ch. 15.2 - Prob. 28ECh. 15.2 - For Exercises 2130, perform the additions on the...Ch. 15.2 - Prob. 30ECh. 15.2 - For Exercises 3140, perform the subtractions on...Ch. 15.2 - Prob. 32ECh. 15.2 - For Exercises 3140, perform the subtractions on...Ch. 15.2 - Prob. 34ECh. 15.2 - For Exercises 3140, perform the subtractions on...Ch. 15.2 - Prob. 36ECh. 15.2 - For Exercises 3140, perform the subtractions on...Ch. 15.2 - Prob. 38ECh. 15.2 - For Exercises 3140, perform the subtractions on...Ch. 15.2 - Prob. 40ECh. 15.2 - For Exercises 4150, perform the multiplications on...Ch. 15.2 - Prob. 42ECh. 15.2 - For Exercises 4150, perform the multiplications on...Ch. 15.2 - Prob. 44ECh. 15.2 - For Exercises 4150, perform the multiplications on...Ch. 15.2 - Prob. 46ECh. 15.2 - For Exercises 4150, perform the multiplications on...Ch. 15.2 - Prob. 48ECh. 15.2 - For Exercises 4150, perform the multiplications on...Ch. 15.2 - Prob. 50ECh. 15.2 - In Exercises 5162, perform the indicated operation...Ch. 15.2 - Prob. 52ECh. 15.2 - In Exercises 5162, perform the indicated operation...Ch. 15.2 - Prob. 54ECh. 15.2 - In Exercises 5162, perform the indicated operation...Ch. 15.2 - Prob. 56ECh. 15.2 - Prob. 57ECh. 15.2 - Prob. 58ECh. 15.2 - Prob. 59ECh. 15.2 - Prob. 60ECh. 15.2 - In Exercises 5162, perform the indicated operation...Ch. 15.2 - Prob. 62ECh. 15.2 - For Exercises 6370, find the additive inverse for...Ch. 15.2 - Prob. 64ECh. 15.2 - Prob. 65ECh. 15.2 - Prob. 66ECh. 15.2 - Prob. 67ECh. 15.2 - For Exercises 6370, find the additive inverse for...Ch. 15.2 - Prob. 69ECh. 15.2 - For Exercises 6370, find the additive inverse for...Ch. 15.2 - For Exercises 7176, find the multiplicative...Ch. 15.2 - Prob. 72ECh. 15.2 - For Exercises 7176, find the multiplicative...Ch. 15.2 - Prob. 74ECh. 15.2 - Prob. 75ECh. 15.2 - Prob. 76ECh. 15.2 - Prob. 77ECh. 15.2 - Prob. 78ECh. 15.2 - Prob. 79ECh. 15.2 - Prob. 80ECh. 15.2 - Prob. 81ECh. 15.2 - Prob. 82ECh. 15.2 - Prob. 83ECh. 15.2 - Prob. 84ECh. 15.2 - Prob. 85ECh. 15.2 - Prob. 86ECh. 15.2 - Prob. 87ECh. 15.2 - Prob. 88ECh. 15.2 - For Exercises 8190, find the value of y using the...Ch. 15.2 - Prob. 90ECh. 15.2 - Prob. 91ECh. 15.2 - Prob. 92ECh. 15.2 - Prob. 93ECh. 15.2 - Prob. 94ECh. 15.2 - Prob. 95ECh. 15.2 - Prob. 96ECh. 15.2 - Prob. 97ECh. 15.2 - Prob. 98ECh. 15.2 - Prob. 99ECh. 15.2 - Prob. 100ECh. 15.2 - Prob. 101ECh. 15.2 - Prob. 102ECh. 15.2 - Prob. 103ECh. 15.2 - Prob. 104ECh. 15.2 - Prob. 105ECh. 15.2 - Prob. 106ECh. 15.2 - Prob. 107ECh. 15.2 - Prob. 108ECh. 15.2 - Prob. 109ECh. 15.2 - Prob. 110ECh. 15.2 - Prob. 111ECh. 15.2 - Prob. 112ECh. 15.2 - Prob. 113ECh. 15.2 - Prob. 114ECh. 15.2 - Prob. 115ECh. 15.2 - Prob. 116ECh. 15.2 - Prob. 117ECh. 15.2 - Prob. 118ECh. 15.2 - Prob. 119ECh. 15.2 - Prob. 120ECh. 15.2 - Prob. 121ECh. 15.3 - Prob. 1TTOCh. 15.3 - Prob. 2TTOCh. 15.3 - Prob. 3TTOCh. 15.3 - Prob. 4TTOCh. 15.3 - Prob. 5TTOCh. 15.3 - Find all natural number solutions to 4x 24 mod 8.Ch. 15.3 - Prob. 1ECh. 15.3 - Prob. 2ECh. 15.3 - Prob. 3ECh. 15.3 - Prob. 4ECh. 15.3 - Prob. 5ECh. 15.3 - Prob. 6ECh. 15.3 - Prob. 7ECh. 15.3 - Prob. 8ECh. 15.3 - Prob. 9ECh. 15.3 - Prob. 10ECh. 15.3 - For Exercises 516, find the values of each number...Ch. 15.3 - For Exercises 516, find the values of each number...Ch. 15.3 - Prob. 13ECh. 15.3 - Prob. 14ECh. 15.3 - Prob. 15ECh. 15.3 - Prob. 16ECh. 15.3 - Prob. 17ECh. 15.3 - Prob. 18ECh. 15.3 - Prob. 19ECh. 15.3 - Prob. 20ECh. 15.3 - Prob. 21ECh. 15.3 - For Exercises 1746, perform the following...Ch. 15.3 - Prob. 23ECh. 15.3 - Prob. 24ECh. 15.3 - Prob. 25ECh. 15.3 - Prob. 26ECh. 15.3 - Prob. 27ECh. 15.3 - Prob. 28ECh. 15.3 - Prob. 29ECh. 15.3 - Prob. 30ECh. 15.3 - Prob. 31ECh. 15.3 - Prob. 32ECh. 15.3 - Prob. 33ECh. 15.3 - Prob. 34ECh. 15.3 - For Exercises 1746, perform the following...Ch. 15.3 - Prob. 36ECh. 15.3 - Prob. 37ECh. 15.3 - For Exercises 1746, perform the following...Ch. 15.3 - Prob. 39ECh. 15.3 - Prob. 40ECh. 15.3 - Prob. 41ECh. 15.3 - Prob. 42ECh. 15.3 - Prob. 43ECh. 15.3 - Prob. 44ECh. 15.3 - Prob. 45ECh. 15.3 - Prob. 46ECh. 15.3 - Prob. 47ECh. 15.3 - Prob. 48ECh. 15.3 - Prob. 49ECh. 15.3 - Prob. 50ECh. 15.3 - Prob. 51ECh. 15.3 - Prob. 52ECh. 15.3 - Prob. 53ECh. 15.3 - For Exercises 4754, find all natural number...Ch. 15.3 - Prob. 55ECh. 15.3 - Prob. 56ECh. 15.3 - Prob. 57ECh. 15.3 - Prob. 58ECh. 15.3 - Prob. 59ECh. 15.3 - Prob. 60ECh. 15.3 - Prob. 61ECh. 15.3 - Prob. 62ECh. 15.3 - Prob. 63ECh. 15.3 - Prob. 64ECh. 15.3 - Prob. 65ECh. 15.3 - Prob. 66ECh. 15.3 - Prob. 67ECh. 15.3 - Prob. 68ECh. 15.3 - Prob. 69ECh. 15.3 - Prob. 70ECh. 15.3 - Prob. 71ECh. 15.3 - Prob. 72ECh. 15.3 - Prob. 73ECh. 15.3 - Prob. 74ECh. 15.3 - Prob. 75ECh. 15.3 - Prob. 76ECh. 15.3 - Prob. 77ECh. 15.3 - Prob. 78ECh. 15.3 - Prob. 79ECh. 15.3 - Prob. 80ECh. 15.3 - Prob. 81ECh. 15.3 - Write a congruence that solves each conversion...Ch. 15.3 - Prob. 83ECh. 15.3 - Prob. 85ECh. 15.3 - Prob. 86ECh. 15.3 - Prob. 87ECh. 15.3 - Prob. 88ECh. 15.3 - Prob. 89ECh. 15.3 - Consider the congruence x2 4 mod 5. (a)Check that...Ch. 15.3 - Prob. 91ECh. 15.3 - For each congruence, find all possible values for...Ch. 15 - For Exercises 111, use the elements A, B, C and...Ch. 15 - Prob. 2RECh. 15 - For Exercises 111, use the elements A, B, C and...Ch. 15 - Prob. 4RECh. 15 - Prob. 5RECh. 15 - Prob. 6RECh. 15 - Prob. 7RECh. 15 - Prob. 8RECh. 15 - Prob. 9RECh. 15 - Prob. 10RECh. 15 - Prob. 11RECh. 15 - Prob. 12RECh. 15 - For Exercises 1216, determine if the given system...Ch. 15 - Prob. 14RECh. 15 - Prob. 15RECh. 15 - Prob. 16RECh. 15 - Prob. 17RECh. 15 - Prob. 18RECh. 15 - Prob. 19RECh. 15 - Prob. 20RECh. 15 - Prob. 21RECh. 15 - Prob. 22RECh. 15 - For Exercises 2328, perform the indicated...Ch. 15 - Prob. 24RECh. 15 - Prob. 25RECh. 15 - Prob. 26RECh. 15 - Prob. 27RECh. 15 - Prob. 28RECh. 15 - Prob. 29RECh. 15 - Prob. 30RECh. 15 - Prob. 31RECh. 15 - Prob. 32RECh. 15 - Prob. 33RECh. 15 - Prob. 34RECh. 15 - For Exercises 3142, find the equivalent number for...Ch. 15 - Prob. 36RECh. 15 - Prob. 37RECh. 15 - Prob. 38RECh. 15 - Prob. 39RECh. 15 - Prob. 40RECh. 15 - Prob. 41RECh. 15 - Prob. 42RECh. 15 - Prob. 43RECh. 15 - Prob. 44RECh. 15 - Prob. 45RECh. 15 - Prob. 46RECh. 15 - Prob. 47RECh. 15 - Prob. 48RECh. 15 - Prob. 49RECh. 15 - Prob. 50RECh. 15 - Prob. 51RECh. 15 - Prob. 52RECh. 15 - Prob. 53RECh. 15 - Prob. 54RECh. 15 - Prob. 55RECh. 15 - Prob. 56RECh. 15 - Prob. 57RECh. 15 - Prob. 58RECh. 15 - Prob. 59RECh. 15 - Prob. 60RECh. 15 - Prob. 61RECh. 15 - Prob. 62RECh. 15 - Prob. 63RECh. 15 - Prob. 64RECh. 15 - Prob. 65RECh. 15 - Prob. 66RECh. 15 - Prob. 1CTCh. 15 - Prob. 2CTCh. 15 - Prob. 3CTCh. 15 - Prob. 4CTCh. 15 - Prob. 5CTCh. 15 - Prob. 6CTCh. 15 - Prob. 7CTCh. 15 - Prob. 8CTCh. 15 - Prob. 9CTCh. 15 - Prob. 10CTCh. 15 - Prob. 11CTCh. 15 - Prob. 12CTCh. 15 - Prob. 13CTCh. 15 - Prob. 14CTCh. 15 - Prob. 15CTCh. 15 - Prob. 16CTCh. 15 - Prob. 17CTCh. 15 - Prob. 18CTCh. 15 - Prob. 19CTCh. 15 - Prob. 20CTCh. 15 - Prob. 21CTCh. 15 - Prob. 22CTCh. 15 - Prob. 23CTCh. 15 - Prob. 24CTCh. 15 - An entire baseball league is signed up for an...Ch. 15 - Prob. 26CT
Knowledge Booster
Background pattern image
Math
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Text book image
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Text book image
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
SEE MORE TEXTBOOKS
The Shape of Data: Distributions: Crash Course Statistics #7; Author: CrashCourse;https://www.youtube.com/watch?v=bPFNxD3Yg6U;License: Standard YouTube License, CC-BY
Shape, Center, and Spread - Module 20.2 (Part 1); Author: Mrmathblog;https://www.youtube.com/watch?v=COaid7O_Gag;License: Standard YouTube License, CC-BY
Shape, Center and Spread; Author: Emily Murdock;https://www.youtube.com/watch?v=_YyW0DSCzpM;License: Standard Youtube License