
Concept explainers
Refer to the Buena School District bus data.
- a. Suppose we consider a bus “old” if it has been in service more than 8 years. At the .01 significance level, can we conclude that less than 40% of the district’s buses are old? Report the p-value.
- b. Find the
median maintenance cost and the median age of the buses. Organize the data into a two-by-twocontingency table , with buses above and below the median of each variable. Determine whether the age of the bus is related to the amount of the maintenance cost. Use the .05 significance level. - c. Is there a relationship between the maintenance cost and the manufacturer of the bus? Use the breakdown in part (b) for the buses above and below the median maintenance cost and the bus manufacturers to create a contingency table. Use the .05 significance level.
a.
Check whether one can conclude that less than 40% of the district buses are old.
Find the p-value.
Answer to Problem 64DE
It cannot be concluded that less than 40% of the district buses are old.
The p-value is 0.181.
Explanation of Solution
Let
The null and alternative hypotheses are stated as follows:
The sample proportion of the old buses can be obtained as follows:
Thus, the sample proportion of the old buses is 0.35.
The sample size n is 80 and
Step-by-step procedure to find the test statistic using the MINITAB software:
- Choose Stat > Basic Statistics > 1 Proportion.
- Choose Summarized data.
- In Number of events, enter 28. In Number of trials, enter 80.
- Enter Hypothesized proportion as 0.40.
- Check Options, enter Confidence level as 99.0.
- Choose less than in alternative.
- Select Method as Normal approximation.
- Click OK in all dialogue boxes.
Output is obtained as follows:
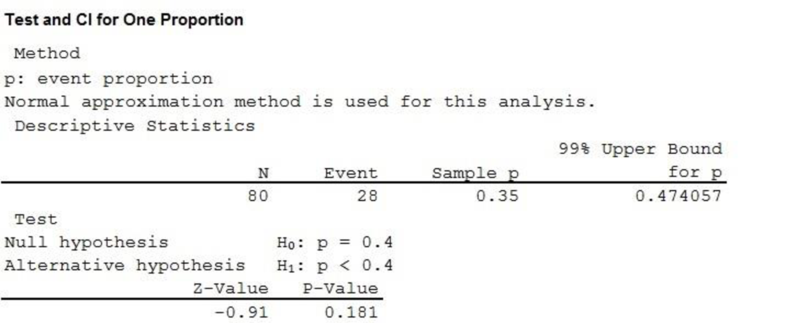
Thus, the value of the test statistic is –0.91and the p-value is 0.181.
Decision rule:
If the p-value is less than the significance level, reject the null. Otherwise, fail to reject the null hypothesis.
Conclusion:
The significance level is 0.01 and the p-value is 0.181. Here, the p-value is greater than the significance level 0.01. By the rejection rule, fail to reject the null hypothesis.
Therefore, one cannot conclude that less than 40% of the district buses are old.
b.
Find the median maintenance cost.
Find the median age of the buses.
Construct a two-way contingency table for the maintenance cost and age of the buses.
Check whether there is a relationship between the age of the buses and maintenance cost.
Answer to Problem 64DE
The median maintenance cost is $456.
The median age of the bus is 4.5 years.
The age of the bus is related to the amount of the maintenance cost.
Explanation of Solution
The median maintenance cost for the bus is calculated as follows:
First, arrange the data into ascending or descending order and then, find the median for the maintenance cost. Since there is even number of observations in the maintenance cost variable, the median is the average of the 40th and 41th observations. That is,
Thus, the median maintenance cost is $456.
The median age of the bus can be obtained as follows:
First, arrange the data into ascending or descending order and then, find the median of the bus. Therefore, the median is the average of the 40th and 41th observations. That is,
Thus, the median age of the bus is 4.5 years.
The contingency table for the maintenance cost and age of the buses is as follows:
| Maintenance cost | Age | ||
| Below median | Above median | Total | |
| Below median | 29 | 11 | 40 |
| Above median | 13 | 27 | 40 |
| Total | 42 | 38 | 80 |
The null and alternative hypotheses are stated below:
The number of degrees of freedom for a contingency table is obtained as follows:
Therefore, the number of degrees of freedom is 1.
Step-by-step procedure to find the test statistic using the MINITAB software:
- Choose Stat > Tables > Chi-Square Test for Association.
- Choose Summarized data in a two-way table.
- In Columns containing the table, enter the columns of Less than median age, and More than median age.
- Under Labels for the table, in Rows enter Maintenance cost and in the columns enter the Age.
- Click OK.
Output obtained using Minitab is as follows:
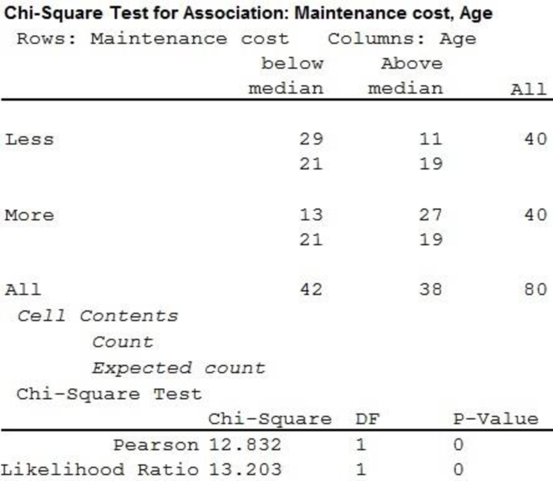
From the above output, the value of the test statistic is 12.832 and the p-value is 0.000.
Conclusion:
The significance level is 0.05 and the p-value is 0.000.
Here, the p-value is less than the significance level 0.05. By the rejection rule, reject the null hypothesis at the 0.05 significance level.
Thus, it can be concluded that the age of the bus is related to the amount of the maintenance cost.
c.
Check whether there is a relationship between the maintenance cost and manufacturer of the bus.
Answer to Problem 64DE
There is a relationship between the maintenance cost and the manufacturer of the bus.
Explanation of Solution
The contingency table for the maintenance cost and manufacturer of the bus is as follows:
| Maintenance cost | Manufacturer | |||
| Bluebird | Keiser | Thompson | Total | |
| Below median | 26 | 14 | 0 | 40 |
| Above median | 21 | 11 | 8 | 40 |
| Total | 47 | 25 | 8 | 80 |
The null and alternative hypotheses are stated below:
The number of degrees of freedom is obtained as follows:
Therefore, the number of degrees of freedom is 1.
Step-by-step procedure to find the test statistic using the MINITAB software:
- Choose Stat > Tables > Chi-Square Test for Association.
- Choose Summarized data in a two-way table.
- In Columns containing the table, enter the columns of below median manufacturer, and above median manufacturer.
- Under Labels for the table, in Rows enter Maintenance cost and in the columns enter the Manufacturer.
- Click OK
Output obtained using Minitab is as follows:
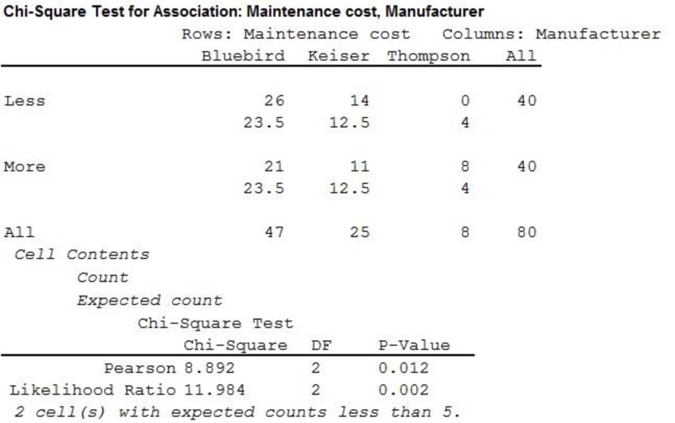
From the above output, the value of the test statistic is 8.892 and the p-value is 0.012.
Conclusion:
The significance level is 0.05 and the p-value is 0.012.
Here, the p-value is less than the significance level 0.05. By the rejection rule, reject the null hypothesis at the 0.05 significance level.
Therefore, there is a relationship between the maintenance cost and the manufacturer.
Want to see more full solutions like this?
Chapter 15 Solutions
Loose Leaf for Statistical Techniques in Business and Economics (Mcgraw-hill/Irwin Series in Operations and Decision Sciences)
- (a) What is a bimodal histogram? (b) Explain the difference between left-skewed, symmetric, and right-skewed histograms. (c) What is an outlierarrow_forward(a) Test the hypothesis. Consider the hypothesis test Ho = : against H₁o < 02. Suppose that the sample sizes aren₁ = 7 and n₂ = 13 and that $² = 22.4 and $22 = 28.2. Use α = 0.05. Ho is not ✓ rejected. 9-9 IV (b) Find a 95% confidence interval on of 102. Round your answer to two decimal places (e.g. 98.76).arrow_forwardLet us suppose we have some article reported on a study of potential sources of injury to equine veterinarians conducted at a university veterinary hospital. Forces on the hand were measured for several common activities that veterinarians engage in when examining or treating horses. We will consider the forces on the hands for two tasks, lifting and using ultrasound. Assume that both sample sizes are 6, the sample mean force for lifting was 6.2 pounds with standard deviation 1.5 pounds, and the sample mean force for using ultrasound was 6.4 pounds with standard deviation 0.3 pounds. Assume that the standard deviations are known. Suppose that you wanted to detect a true difference in mean force of 0.25 pounds on the hands for these two activities. Under the null hypothesis, 40 = 0. What level of type II error would you recommend here? Round your answer to four decimal places (e.g. 98.7654). Use a = 0.05. β = i What sample size would be required? Assume the sample sizes are to be equal.…arrow_forward
- = Consider the hypothesis test Ho: μ₁ = μ₂ against H₁ μ₁ μ2. Suppose that sample sizes are n₁ = 15 and n₂ = 15, that x1 = 4.7 and X2 = 7.8 and that s² = 4 and s² = 6.26. Assume that o and that the data are drawn from normal distributions. Use απ 0.05. (a) Test the hypothesis and find the P-value. (b) What is the power of the test in part (a) for a true difference in means of 3? (c) Assuming equal sample sizes, what sample size should be used to obtain ẞ = 0.05 if the true difference in means is - 2? Assume that α = 0.05. (a) The null hypothesis is 98.7654). rejected. The P-value is 0.0008 (b) The power is 0.94 . Round your answer to four decimal places (e.g. Round your answer to two decimal places (e.g. 98.76). (c) n₁ = n2 = 1 . Round your answer to the nearest integer.arrow_forwardConsider the hypothesis test Ho: = 622 against H₁: 6 > 62. Suppose that the sample sizes are n₁ = 20 and n₂ = 8, and that = 4.5; s=2.3. Use a = 0.01. (a) Test the hypothesis. Round your answers to two decimal places (e.g. 98.76). The test statistic is fo = i The critical value is f = Conclusion: i the null hypothesis at a = 0.01. (b) Construct the confidence interval on 02/022 which can be used to test the hypothesis: (Round your answer to two decimal places (e.g. 98.76).) iarrow_forward2011 listing by carmax of the ages and prices of various corollas in a ceratin regionarrow_forward
- س 11/ أ . اذا كانت 1 + x) = 2 x 3 + 2 x 2 + x) هي متعددة حدود محسوبة باستخدام طريقة الفروقات المنتهية (finite differences) من جدول البيانات التالي للدالة (f(x . احسب قيمة . ( 2 درجة ) xi k=0 k=1 k=2 k=3 0 3 1 2 2 2 3 αarrow_forward1. Differentiate between discrete and continuous random variables, providing examples for each type. 2. Consider a discrete random variable representing the number of patients visiting a clinic each day. The probabilities for the number of visits are as follows: 0 visits: P(0) = 0.2 1 visit: P(1) = 0.3 2 visits: P(2) = 0.5 Using this information, calculate the expected value (mean) of the number of patient visits per day. Show all your workings clearly. Rubric to follow Definition of Random variables ( clearly and accurately differentiate between discrete and continuous random variables with appropriate examples for each) Identification of discrete random variable (correctly identifies "number of patient visits" as a discrete random variable and explains reasoning clearly.) Calculation of probabilities (uses the probabilities correctly in the calculation, showing all steps clearly and logically) Expected value calculation (calculate the expected value (mean)…arrow_forwardif the b coloumn of a z table disappeared what would be used to determine b column probabilitiesarrow_forward
- Construct a model of population flow between metropolitan and nonmetropolitan areas of a given country, given that their respective populations in 2015 were 263 million and 45 million. The probabilities are given by the following matrix. (from) (to) metro nonmetro 0.99 0.02 metro 0.01 0.98 nonmetro Predict the population distributions of metropolitan and nonmetropolitan areas for the years 2016 through 2020 (in millions, to four decimal places). (Let x, through x5 represent the years 2016 through 2020, respectively.) x₁ = x2 X3 261.27 46.73 11 259.59 48.41 11 257.96 50.04 11 256.39 51.61 11 tarrow_forwardIf the average price of a new one family home is $246,300 with a standard deviation of $15,000 find the minimum and maximum prices of the houses that a contractor will build to satisfy 88% of the market valuearrow_forward21. ANALYSIS OF LAST DIGITS Heights of statistics students were obtained by the author as part of an experiment conducted for class. The last digits of those heights are listed below. Construct a frequency distribution with 10 classes. Based on the distribution, do the heights appear to be reported or actually measured? Does there appear to be a gap in the frequencies and, if so, how might that gap be explained? What do you know about the accuracy of the results? 3 4 555 0 0 0 0 0 0 0 0 0 1 1 23 3 5 5 5 5 5 5 5 5 5 5 5 5 6 6 8 8 8 9arrow_forward
 Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL


