
(a)
The linear programming problem to maximize the profit if the factors are given as below,
| Resource | Regular | Premium | Supreme | Resource Availability |
| Raw gas | ||||
| Production time | ||||
| Storage | ||||
| Profit |
(a)
Answer to Problem 2P
Solution:
The linear programming problem to maximize the profit is,
Subject to the constraints:
Explanation of Solution
Given Information:
The factors are given as below,
| Resource | Regular | Premium | Supreme | Resource Availability |
| Raw gas | ||||
| Production time | ||||
| Storage | ||||
| Profit |
Assume x be the amount of regular resource, y be the amount of premium resource and z be the amount of supreme resource
Therefore, total amount of raw gas is
But the resource availability is
Now, the total production time from the provided table is
But the resource production time is
Now, regular resource can store up to 9 tones. Therefore,
And, premium resource can store up to 6 tones. Therefore,
Also, supreme resource can store up to 5 tones. Therefore,
Since, the amount of resource cannot be negative. Therefore, the positivity constraints are,
Now, the company makes profits of
Subject to the constraints:
(b)
The solution of the linear programming problem,
Subject to the constraints:
By the Simplex method.
(b)
Answer to Problem 2P
Solution:
The values of variables are
Explanation of Solution
Given Information:
The linear programming problem,
Subject to the constraints:
Consider the provided linear programming problem,
Subject to the constraints:
First convert the above problem to standard form by adding slack variables.
As the constraints are subjected to less than condition, non- negative slack variables are added to reach equality.
Let the slack variables be
Thus, the linear programming model would be:
Subject to the constraints:
The above linear programming models consist of three non-basic variables
Now the apply the Simplex method and solve the above problem as:
| Basic | x | y | z | Solution | Intercept |
||||||
| 1 | -150 | -175 | -250 | 0 | 0 | 0 | 0 | 0 | 0 | ||
| 0 | 7 | 11 | 15 | 1 | 0 | 0 | 0 | 0 | 154 | 10.2667 | |
| 0 | 10 | 8 | 12 | 0 | 1 | 0 | 0 | 0 | 80 | 6.66667 | |
| 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 9 | ∞ | |
| 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 6 | ∞ | |
| 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 5 | 5 |
The negative minimum, P is
The minimum ratio is 5 and it corresponds to basis variable S5. So, the leaving variable is S5.
Therefore, the pivot element is 1.
| Basic | x | y | z | Solution | Intercept |
||||||
| 1 | -150 | -175 | 0 | 0 | 0 | 0 | 0 | 250 | 1250 | ||
| 0 | 7 | 11 | 0 | 1 | 0 | 0 | 0 | -15 | 79 | 7.18182 | |
| 0 | 10 | 8 | 0 | 0 | 1 | 0 | 0 | -12 | 20 | 2.5 | |
| 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 9 | ∞ | |
| 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 6 | 6 | |
| z | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 5 | ∞ |
The negative minimum, P is
The minimum ratio is 2.5 and it corresponds to basis variable S2. So, the leaving variable is S2.
Therefore, the pivot element is 8.
| Basic | x | y | z | Solution | Intercept |
||||||
| 1 | 68.75 | 0 | 0 | 0 | 21.88 | 0 | 0 | -12.5 | 1687.5 | ||
| 0 | -6.75 | 0 | 0 | 1 | -1.375 | 0 | 0 | 1.5 | 51.5 | 34.3333 | |
| y | 0 | 1.25 | 1 | 0 | 0 | 0.125 | 0 | 0 | -1.5 | 2.5 | -1.66667 |
| 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 9 | ∞ | |
| 0 | -1.25 | 0 | 0 | 0 | -0.125 | 0 | 1 | 1.5 | 3.5 | 2.33333 | |
| z | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 5 | 5 |
The negative minimum, P is
The minimum positive ratio is 2.33333 and it corresponds to basis variable S4. So, the leaving variable is S4.
Therefore, the pivot element is 1.5.
| Basic | x | y | z | Solution | ||||||
| 1 | 58.3333 | 0 | 0 | 0 | 20.83 | 0 | 8.33 | 0 | 1716.7 | |
| 0 | -5.5 | 0 | 0 | 1 | -1.25 | 0 | -1 | 0 | 48 | |
| y | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 6 |
| 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 9 | |
| 0 | -0.8333 | 0 | 0 | 0 | -0.083 | 0 | 0.67 | 1 | 2.3333 | |
| z | 0 | 0.83333 | 0 | 1 | 0 | 0.083 | 0 | -0.67 | 0 | 2.6667 |
Since
Hence, the values of variables are
(c)
The solution of the linear programming problem,
Subject to the constraints:
By the use of software.
(c)
Answer to Problem 2P
Solution:
The maximum profit is 1716.7 with
Explanation of Solution
Given Information:
The linear programming problem,
Subject to the constraints:
Use excel solver as below, to solve the linear programming,
Step 1: Enter the coefficients of x, y and z for each constraint as below,
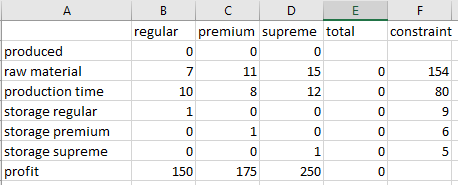
Step 2: Use formulas in column E to find total are as below,
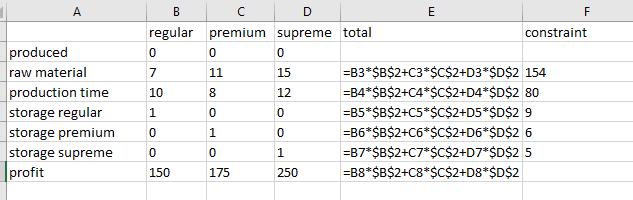
Step 3: click on Solver button under the Data Ribbon. Set the values in the Solver dialogue box as below:
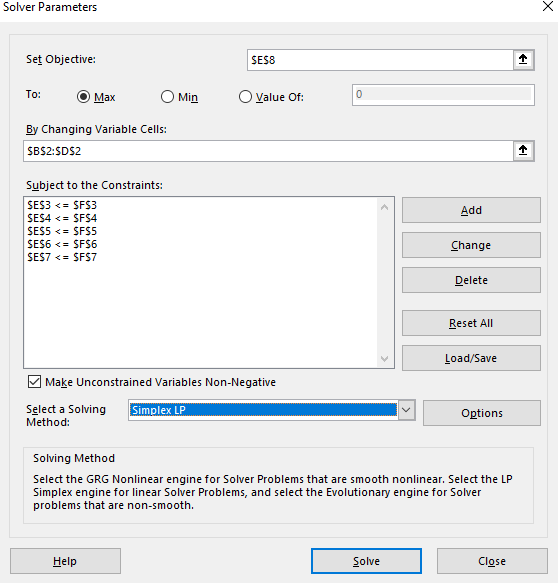
Step 4: Press the solve button.
The result obtained as,
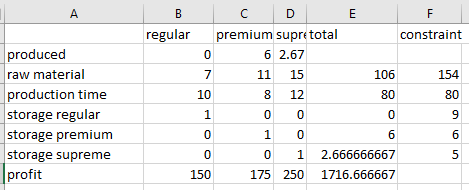
Hence, the maximum value profit is 1716.7 with
(d)
The constraint among increasing raw material, storage or production time that gives the maximum profit.
(d)
Answer to Problem 2P
Solution:
The increasing production time will give the maximum profit.
Explanation of Solution
Given Information:
The linear programming problem,
Subject to the constraints:
To obtain the maximum profit, the shadow price should be high.
Use excel as below to find the shadow price by generating the sensitivity report,
Follow same steps up to the step 4 of part (d) then select the report as sensitivity as below,
The sensitivity report for the linear programming problem is as follows,
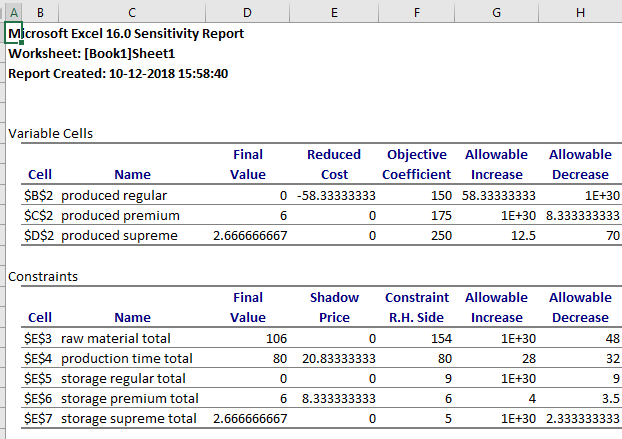
From the above sensitivity report, it is observed that the production time has a high shadow price.
Hence, the production time will give the maximum profit.
Want to see more full solutions like this?
Chapter 15 Solutions
EBK NUMERICAL METHODS FOR ENGINEERS
- Consider the following mixed-integer linear program. Max 3x1 + 4x2 s.t. 4x1 + 7x2 ≤ 28 8x1 + 5x2 ≤ 40 x1, x2 ≥ and x1 integer (a) Graph the constraints for this problem. Indicate on your graph all feasible mixed-integer solutions. On the coordinate plane the horizontal axis is labeled x1 and the vertical axis is labeled x2. A region bounded by a series of connected line segments, and several horizontal lines are on the graph. The series of line segments connect the approximate points (0, 4), (3.889, 1.778), and (5, 0). The region is above the horizontal axis, to the right of the vertical axis, and below the line segments. At each integer value between 0 and 4 on the vertical axis, a horizontal line extends out from the vertical axis to the series of connect line segments. On the coordinate plane the horizontal axis is labeled x1 and the vertical axis is labeled x2. A region bounded by a series of connected line segments, and several…arrow_forwardConsider the nonlinear optimization model stated below. Min s.t. 2x²-18x + 2XY + y² - 14Y + 53 x + 4Y ≤ 8 (a) Find the minimum solution to this problem. |at (X, Y) = (b) If the right-hand side of the constraint is increased from 8 to 9, how much do you expect the objective function to change? Based on the dual value on the constraint X + 4Y ≤ 8, we expect the optimal objective function value to decrease by (c) Resolve the problem with a new right-hand side of the constraint of 9. How does the actual change compare with your estimate? If we resolve the problem with a new right-hand-side of 9 the new optimal objective function value is| , so the actual change is a decrease of rather than what we expected in part (b).arrow_forwardStatement:If 2 | a and 3| a, then 6 a. So find three integers, and at least one integer should be negative. For each of your examples, determine if the statement is true or false.arrow_forward
- Statement: If 4 | a and 6 | a, then 24 | a. So find three integers, and at least one integer should be negative. For each of your examples, determine if the statement is true or false.arrow_forward2) dassify each critical point of the given plane autovers system x'=x-2x²-2xy y' = 4y-Sy³-7xyarrow_forward24.2. Show that, for any constant zo Є C, (a). e* = e²o Σ j=0 (2 - 20); j! |z|arrow_forward25.4. (a). Show that when 0 < || < 4, 1 1 8 zn 4z - z2 4z +Σ 4n+2* (b). Show that, when 0 < |z1|<2, n=() 2 1 8 (z - 1)(z - 3) - 3 2(z - 1) 3 Σ (2-1)" 27+2 n=0 (c). Show that, when 2<|z|< ∞, 1 z4+4z2 -*()*. n=0arrow_forward. Expand sinh z in Taylor's series at zo = πi, and show that lim sinh: καπί κ - п - - 1.arrow_forward24.3. Show that 8 (a). =(+1)(z+1)*, |+1|<1, j=0 8 (b). sin³ z j=0 (-1) 3(1-9) 4 (2j+1)! 22j+1, |<∞,arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,






