
Concept explainers
Sketching a Vector Field In Exercises 1 and 2, find
To calculate:
Answer to Problem 1RE
Solution:
Explanation of Solution
Given:
Formula used:
Calculation:
Using (1), the magnitude of a vector function
The divergence of a vector field
We start by making a table showing the vector field at several points. The table shown is a small sample. The vector field lines at many other points should be calculated to get a representative vector field, which can be obtained by using a computer algebra system.
x |
-2 |
-1 |
0 |
0 |
1 |
2 |
y |
1 |
-1 |
-1 |
1 |
-1 |
-1 |
z |
1 |
1 |
1 |
1 |
1 |
1 |
Next, we draw line segments at the points to represent the vector field
The sketch of the representative vectors, is shown below
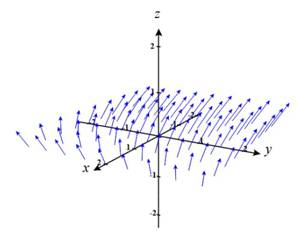
Interpretation: The vector field is irrotational in nature and has a positive divergence
Want to see more full solutions like this?
Chapter 15 Solutions
CALCULUS: EARLY TRANSCENDENTAL FUNCTIO
- A 20 foot ladder rests on level ground; its head (top) is against a vertical wall. The bottom of the ladder begins by being 12 feet from the wall but begins moving away at the rate of 0.1 feet per second. At what rate is the top of the ladder slipping down the wall? You may use a calculator.arrow_forwardExplain the focus and reasons for establishment of 12.4.1(root test) and 12.4.2(ratio test)arrow_forwarduse Integration by Parts to derive 12.6.1arrow_forward
- Explain the relationship between 12.3.6, (case A of 12.3.6) and 12.3.7arrow_forwardExplain the key points and reasons for the establishment of 12.3.2(integral Test)arrow_forwardUse 12.4.2 to determine whether the infinite series on the right side of equation 12.6.5, 12.6.6 and 12.6.7 converges for every real number x.arrow_forward
- use Corollary 12.6.2 and 12.6.3 to derive 12.6.4,12.6.5, 12.6.6 and 12.6.7arrow_forwardExplain the focus and reasons for establishment of 12.5.1(lim(n->infinite) and sigma of k=0 to n)arrow_forwardExplain the focus and reasons for establishment of 12.5.3 about alternating series. and explain the reason why (sigma k=1 to infinite)(-1)k+1/k = 1/1 - 1/2 + 1/3 - 1/4 + .... converges.arrow_forward
 Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning
Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning

