
Calculate the Riemann sum for the given
a) Lower-left vertex
b) Midpoint of rectangle
Then calculate the exact value of the double integral.
Answer to Problem 1CRE
Solution:
(a) The Riemann sum for the given double integral using lower-left vertices is 240.
(b)The Riemann sum for the given double integral using midpoints is 510.
And the exact value of the double integral is 520.
Explanation of Solution
Given:
The integral:
Formulas:
Where
Calculations:
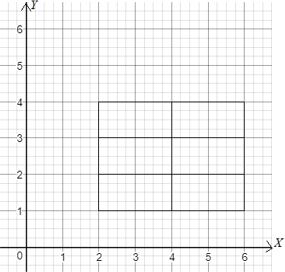
From the given integral, we can observe that and . Since our aim is to find , we need to divide the rectangle into subrectangles. The length and width of each subrectangle are calculated as follows:
Therefore, the area of each subrectangle is .
The subrectangles are shown in Image 1.
Image 1:
(a) Using Lower-left vertex
Here, we use the lower-left vertices of each subrectangleto find the Riemann sum . Notice that the lower-left vertices are and are shown in Image 2.
Image 2:
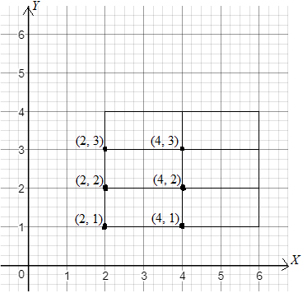
Thus,
(b) Using Midpoint of Rectangle:
Here, we use the midpoints of each subrectangle to find the Riemann sum . Notice that the midpoints are and are shown in Image 3.
Image 3:
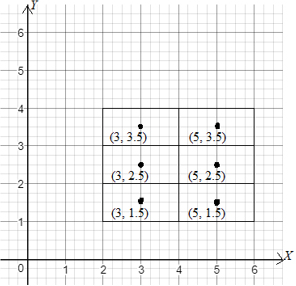
Thus,
To calculate the exact value of the integral:
Conclusion:
Thus,
(a) The Riemann sum for the given double integral using lower-left vertices is 240.
(b)The Riemann sum for the given double integral using midpoints is 510.
And the exact value of the double integral is 520.
Want to see more full solutions like this?
Chapter 15 Solutions
CALCULUS:EARLY TRANS.-SAPLINGPLUS
- Calculus lll May I please have the statements with blank lines completed; furthermore, may I please have the text box completed? Thank youarrow_forwardCalculus lll May I please have the statements completed for the following text lines and box? Thank you so much,arrow_forwardCalculus lll May I please have the solution for the following exercise? Thank you so mucharrow_forward
- Calculus lll May I please have the statement completed for the following box? Thank you so much,arrow_forwardCalculus lll May I please have the solution for the following exercise? Thank you so mucharrow_forwardUse a graphing calculator to find where the curves intersect and to find the area between the curves. y=ex, y=-x²-4x a. The left point of intersection is (Type integers or decimals rounded to the nearest thousandth as needed. Type an ordered pair.)arrow_forward
- Find the area between the curves. x= -5, x=3, y=2x² +9, y=0 The area between the curves is (Round to the nearest whole number as needed.)arrow_forwardcan you solve these questions with step by step with clear explaination pleasearrow_forwardFind the area between the following curves. x=-1, x=3, y=x-1, and y=0 The area between the curves is (Simplify your answer.)arrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





