
Concept explainers
DATA In your physics lab, an oscillator is attached to one end of a horizontal string. The other end of the string passes over a frictionless pulley. You suspend a mass M from the free end of the string, producing tension Mg in the string. The oscillator produces transverse waves of frequency f on the string. You don’t vary this frequency during the experiment, but you try strings with three different linear mass densities μ. You also keep a fixed distance between the end of the string where the oscillator is attached and the point where the string is in contact with the pulley’s rim. To produce standing waves on the string, you vary M; then you measure the node-to-node distance d for each standing-wave pattern and obtain the following data:
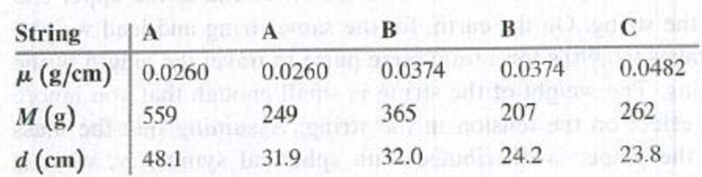
(a) Explain why you obtain only certain values of d. (b) Graph μd2 (in kg · m) versus M (in kg). Explain why the data plotted this way should fall close to a straight line. (c) Use the slope of the best straight-line fit to the data to determine the frequency f of the waves produced on the siring by the oscillator. Take g = 9.80 m/s2. (d) For string A (μ = 0.0260 g/cm), what value of M (in grams) would be required to produce a standing wave with a node-to-node distance of 24.0 cm? Use the value of f that you calculated in part (c).
Want to see the full answer?
Check out a sample textbook solution
Chapter 15 Solutions
MASTERINGPHYSICS W/ETEXT ACCESS CODE 6
Additional Science Textbook Solutions
Human Anatomy & Physiology (2nd Edition)
Microbiology: An Introduction
Anatomy & Physiology (6th Edition)
Introductory Chemistry (6th Edition)
Campbell Biology (11th Edition)
Campbell Essential Biology with Physiology (5th Edition)
- Close-up view etermine; The volume of the object given that the initial level of water in the measuring cylinder 23cm3. The density of the object. simple cell made by dipping copper and zinc plates into dilute sulfuric acid solution. A bull onnected across the plates using a wire. State what constitute current flow through the wire The bulb connected across is observed to light for some time and then goes out. State t possible asons for this observation. State two ways in which the processes named in question (b) above can be minimized t the bulb light for a longer period. ead is rated 80Ah. Determine the current that can be drawn continuouslyarrow_forwardAnswers with -1.828, -1.31 or 939.3 are not correct.arrow_forwardThree slits, each separated from its neighbor by d = 0.06 mm, are illuminated by a coherent light source of wavelength 550 nm. The slits are extremely narrow. A screen is located L = 2.5 m from the slits. The intensity on the centerline is 0.05 W. Consider a location on the screen x = 1.72 cm from the centerline. a) Draw the phasors, according to the phasor model for the addition of harmonic waves, appropriate for this location. b) From the phasor diagram, calculate the intensity of light at this location.arrow_forward
- A Jamin interferometer is a device for measuring or for comparing the indices of refraction of gases. A beam of monochromatic light is split into two parts, each of which is directed along the axis of a separate cylindrical tube before being recombined into a single beam that is viewed through a telescope. Suppose we are given the following, • Length of each tube is L = 0.4 m. • λ= 598 nm. Both tubes are initially evacuated, and constructive interference is observed in the center of the field of view. As air is slowly let into one of the tubes, the central field of view changes dark and back to bright a total of 198 times. (a) What is the index of refraction for air? (b) If the fringes can be counted to ±0.25 fringe, where one fringe is equivalent to one complete cycle of intensity variation at the center of the field of view, to what accuracy can the index of refraction of air be determined by this experiment?arrow_forward1. An arrangement of three charges is shown below where q₁ = 1.6 × 10-19 C, q2 = -1.6×10-19 C, and q3 3.2 x 10-19 C. 2 cm Y 93 92 91 X 3 cm (a) Calculate the magnitude and direction of the net force on q₁. (b) Sketch the direction of the forces on qiarrow_forward(Figure 1)In each case let w be the weight of the suspended crate full of priceless art objects. The strut is uniform and also has weight w Find the direction of the force exerted on the strut by the pivot in the arrangement (a). Express your answer in degrees. Find the tension Tb in the cable in the arrangement (b). Express your answer in terms of w. Find the magnitude of the force exerted on the strut by the pivot in the arrangement (b). Express your answer in terms of w.arrow_forward
- (Figure 1)In each case let ww be the weight of the suspended crate full of priceless art objects. The strut is uniform and also has weight w. Find the direction of the force exerted on the strut by the pivot in the arrangement (b). Express your answer in degrees.arrow_forwardA 70.0 cm, uniform, 40.0 N shelf is supported horizontally by two vertical wires attached to the sloping ceiling (Figure 1). A very small 20.0 N tool is placed on the shelf midway between the points where the wires are attached to it. Find the tension in the left-hand wire. Express your answer with the appropriate units.arrow_forwardFind the total bind Mev. binding energy for 13 Carbon, 6C (atomic mass = 13.0033554)arrow_forward
- What is the 27 energy absorbed in this endothermic Auclear reaction 2] Al + 'n → 27 Mg + ! H? (The atom mass of "Al is 26.981539u. and that of 11 Mg is 26.984341u) MeVarrow_forwardWhat is the energy released in this nuclear reaction 1 F + "', H-1 O+ He? 19 19 16 (The atomic mass of 1F is 18.998403 u, and that of 20 is 15.9949154) MeV.arrow_forwardWhat is the energy released in this B+ nuclear reaction خالد 2½ Al w/ Mg + ie? (The atomic mass of 11 Al is 23.9999394 and that > of 12 Mg is 23.985041 u) MeV.arrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning
Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





