Calculus
Calculus
11th Edition
ISBN: 9781337275576
Author: Larson, Ron, Edwards, Bruce
Publisher: Cengage Learning,
bartleby

Videos

Textbook Question
Book Icon
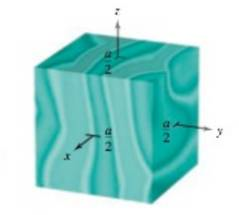
Chapter 14.6, Problem 54E

Moments of Inertia In Exercises 53- 56, find I x , I y , and I z for the solid of given density. Use a computer algebra system to evaluate the triple integrals.

(a) ρ = ( x , y , z ) = k

(b) ρ = ( x , y , z ) = k ( x 2 + y 2 )

Chapter 14.6, Problem 54E, Moments of Inertia In Exercises 53- 56, find Ix,Iy, and Iz for the solid of given density. Use a

Expert Solution & Answer
Check Mark
bartleby

Trending nowThis is a popular solution!

See solution
Check out sample textbook solution
Blurred answer
Previous
Chapter 14.6, Problem 53E
Next
Chapter 14.6, Problem 55E
Students have asked these similar questions
Subject-advance maths
Determine the type of points on the X (u, v) = (u, v, u?) surface. Differential geometry
Potential Theorem Consider the scalar (potential) function +(x,y,z)=axy' +z³ Calculate the magnitude of the conservative force associated to o at point P(a,2,b). Take a = 18 and b = -2 %3D Answer:

Chapter 14 Solutions

Calculus

Ch. 14.1 - CONCEPT CHECK Iterated Integral Explain what is...Ch. 14.1 - Prob. 2ECh. 14.1 - Question: Evaluate the integral: 0x(2xy)dyCh. 14.1 - Evaluating an IntegralIn Exercises 310, evaluate...Ch. 14.1 - Evaluating an IntegralIn Exercises 310, evaluate...Ch. 14.1 - Evaluating an IntegralIn Exercises 310, evaluate...Ch. 14.1 - Evaluating an Integral In Exercises 3-10, evaluate...Ch. 14.1 - Evaluating an IntegralIn Exercises 310, evaluate...Ch. 14.1 - Evaluating an Integral In Exercises 3-10, evaluate...Ch. 14.1 - Evaluating an Integral In Exercises 3-10, evaluate...
Ch. 14.1 - Prob. 11ECh. 14.1 - Prob. 12ECh. 14.1 - Prob. 13ECh. 14.1 - Prob. 14ECh. 14.1 - Prob. 15ECh. 14.1 - Prob. 16ECh. 14.1 - Prob. 17ECh. 14.1 - Prob. 18ECh. 14.1 - Evaluating an Iterated Integral In Exercises...Ch. 14.1 - Evaluating an Iterated Integral In Exercises...Ch. 14.1 - Prob. 21ECh. 14.1 - Prob. 22ECh. 14.1 - Evaluating an Iterated Integral In Exercises...Ch. 14.1 - Prob. 24ECh. 14.1 - Prob. 25ECh. 14.1 - Prob. 26ECh. 14.1 - Prob. 27ECh. 14.1 - Prob. 28ECh. 14.1 - Prob. 29ECh. 14.1 - Evaluating an Improper Iterated Integral In...Ch. 14.1 - Prob. 31ECh. 14.1 - Evaluating an Improper Iterated Integral In...Ch. 14.1 - Finding the Area of a Region In Exercises 33-36,...Ch. 14.1 - Prob. 34ECh. 14.1 - Prob. 35ECh. 14.1 - Finding the Area of a Region In Exercises 33-36,...Ch. 14.1 - Finding the Area of a Region In Exercises 37-42,...Ch. 14.1 - Prob. 38ECh. 14.1 - Prob. 39ECh. 14.1 - Prob. 40ECh. 14.1 - Prob. 41ECh. 14.1 - Prob. 42ECh. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Prob. 44ECh. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Prob. 48ECh. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Prob. 50ECh. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Prob. 52ECh. 14.1 - Prob. 53ECh. 14.1 - Prob. 54ECh. 14.1 - Prob. 55ECh. 14.1 - Prob. 56ECh. 14.1 - Prob. 57ECh. 14.1 - Prob. 58ECh. 14.1 - Prob. 59ECh. 14.1 - Prob. 60ECh. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Prob. 65ECh. 14.1 - Changing the Order of Integration In Exercises...Ch. 14.1 - Prob. 67ECh. 14.1 - Prob. 68ECh. 14.1 - Prob. 69ECh. 14.1 - HOW DO YOU SEE IT? Use each order of integration...Ch. 14.1 - Prob. 71ECh. 14.1 - Prob. 72ECh. 14.1 - Prob. 73ECh. 14.1 - Prob. 74ECh. 14.1 - Prob. 75ECh. 14.1 - Evaluating an Iterated Integral Using Technology...Ch. 14.1 - Prob. 77ECh. 14.1 - Comparing Different Orders of Integration Using...Ch. 14.1 - Prob. 79ECh. 14.1 - Prob. 80ECh. 14.2 - CONCEPT CHECK Approximating the Volume of a Solid...Ch. 14.2 - Prob. 2ECh. 14.2 - Approximation In Exercises 3-6, approximate the...Ch. 14.2 - Approximation In Exercises 3-6, approximate the...Ch. 14.2 - Prob. 5ECh. 14.2 - Prob. 6ECh. 14.2 - Prob. 7ECh. 14.2 - Prob. 8ECh. 14.2 - Prob. 9ECh. 14.2 - Evaluating a Double IntegralIn Exercises 712,...Ch. 14.2 - Prob. 11ECh. 14.2 - Evaluating a Double Integral In Exercises 712,...Ch. 14.2 - Evaluating a Double Integral In Exercises 1320,...Ch. 14.2 - Evaluating a Double IntegralIn Exercises 1320, set...Ch. 14.2 - Evaluating a Double IntegralIn Exercises 1320, set...Ch. 14.2 - Evaluating a Double IntegralIn Exercises 1320, set...Ch. 14.2 - Prob. 17ECh. 14.2 - Prob. 18ECh. 14.2 - Evaluating a Double IntegralIn Exercises 1320, set...Ch. 14.2 - Prob. 20ECh. 14.2 - Prob. 21ECh. 14.2 - Finding Volume In Exercises 21-26, use a double...Ch. 14.2 - Prob. 23ECh. 14.2 - Finding Volume In Exercises 21-26, use a double...Ch. 14.2 - Prob. 25ECh. 14.2 - Finding Volume In Exercises 21-26, use a double...Ch. 14.2 - Prob. 27ECh. 14.2 - Prob. 28ECh. 14.2 - Finding Volume In Exercises 29-34, set up and...Ch. 14.2 - Prob. 30ECh. 14.2 - Prob. 31ECh. 14.2 - Finding Volume In Exercises 29-34, set up and...Ch. 14.2 - Prob. 33ECh. 14.2 - Finding Volume In Exercises 29-34, set up and...Ch. 14.2 - Prob. 35ECh. 14.2 - Prob. 36ECh. 14.2 - Prob. 37ECh. 14.2 - Prob. 38ECh. 14.2 - Prob. 39ECh. 14.2 - Volume of a Region Bounded by Two Surfaces In...Ch. 14.2 - Prob. 41ECh. 14.2 - Prob. 42ECh. 14.2 - Prob. 43ECh. 14.2 - Prob. 44ECh. 14.2 - Prob. 45ECh. 14.2 - Prob. 46ECh. 14.2 - Prob. 47ECh. 14.2 - Prob. 48ECh. 14.2 - Prob. 49ECh. 14.2 - Prob. 50ECh. 14.2 - Prob. 51ECh. 14.2 - Prob. 52ECh. 14.2 - Prob. 53ECh. 14.2 - Prob. 54ECh. 14.2 - Average Value In Exercises 51-56. find the average...Ch. 14.2 - Prob. 56ECh. 14.2 - Prob. 57ECh. 14.2 - Prob. 58ECh. 14.2 - Prob. 59ECh. 14.2 - Prob. 60ECh. 14.2 - Prob. 61ECh. 14.2 - Prob. 62ECh. 14.2 - Prob. 63ECh. 14.2 - Prob. 64ECh. 14.2 - Prob. 65ECh. 14.2 - Prob. 66ECh. 14.2 - Prob. 67ECh. 14.2 - Prob. 68ECh. 14.2 - Prob. 69ECh. 14.2 - Prob. 70ECh. 14.2 - Maximizing a Double Integral Determine the region...Ch. 14.2 - Minimizing a Double Integral Determine the region...Ch. 14.2 - Prob. 73ECh. 14.2 - Prob. 74ECh. 14.2 - Prob. 75ECh. 14.2 - Prob. 76ECh. 14.3 - CONCEPT CHECK Choosing a Coordinate System In...Ch. 14.3 - CONCEPT CHECK Choosing a Coordinate SystemIn...Ch. 14.3 - Prob. 3ECh. 14.3 - Prob. 4ECh. 14.3 - Describing a Region In Exercises 58, use polar...Ch. 14.3 - Describing a Region In Exercises 58, use polar...Ch. 14.3 - Prob. 7ECh. 14.3 - Describing a Region In Exercises 58, use polar...Ch. 14.3 - Prob. 9ECh. 14.3 - Evaluating a Double Integral in Exercises 9-16,...Ch. 14.3 - Prob. 11ECh. 14.3 - Evaluating a Double Integral in Exercises 9-16,...Ch. 14.3 - Prob. 13ECh. 14.3 - Prob. 14ECh. 14.3 - Prob. 15ECh. 14.3 - Prob. 16ECh. 14.3 - Converting to Polar Coordinates: In Exercises...Ch. 14.3 - Prob. 18ECh. 14.3 - Converting to Polar Coordinates: In Exercises...Ch. 14.3 - Prob. 20ECh. 14.3 - Converting to Polar Coordinates In Exercises...Ch. 14.3 - Converting to Polar Coordinates: In Exercises...Ch. 14.3 - Prob. 23ECh. 14.3 - Converting to Polar Coordinates: In Exercises...Ch. 14.3 - Prob. 25ECh. 14.3 - Converting to Polar Coordinates: In Exercises...Ch. 14.3 - Prob. 27ECh. 14.3 - Converting to Polar Coordinates: In Exercises 27...Ch. 14.3 - Prob. 29ECh. 14.3 - Prob. 30ECh. 14.3 - Converting to Polar Coordinates In Exercises 2932,...Ch. 14.3 - Prob. 32ECh. 14.3 - Prob. 33ECh. 14.3 - Prob. 34ECh. 14.3 - Prob. 35ECh. 14.3 - Prob. 36ECh. 14.3 - Prob. 37ECh. 14.3 - Prob. 38ECh. 14.3 - Prob. 39ECh. 14.3 - Prob. 40ECh. 14.3 - Prob. 41ECh. 14.3 - Prob. 42ECh. 14.3 - Prob. 43ECh. 14.3 - Prob. 44ECh. 14.3 - AreaIn Exercises 4146, use a double integral to...Ch. 14.3 - AreaIn Exercises 4146, use a double integral to...Ch. 14.3 - Prob. 47ECh. 14.3 - Prob. 48ECh. 14.3 - Area: In Exercises 4752, sketch a graph of the...Ch. 14.3 - Area: In Exercises 4752, sketch a graph of the...Ch. 14.3 - Prob. 51ECh. 14.3 - Area: In Exercises, 4752, sketch a graph of the...Ch. 14.3 - Prob. 53ECh. 14.3 - Prob. 54ECh. 14.3 - Prob. 55ECh. 14.3 - Prob. 56ECh. 14.3 - Volume Determine the diameter of a hole that is...Ch. 14.3 - Prob. 58ECh. 14.3 - Prob. 59ECh. 14.3 - Prob. 60ECh. 14.3 - Prob. 61ECh. 14.3 - True or False? In Exercises 61 and 62, determine...Ch. 14.3 - Prob. 63ECh. 14.3 - Prob. 64ECh. 14.3 - Prob. 65ECh. 14.3 - Prob. 66ECh. 14.3 - Prob. 67ECh. 14.3 - Area Show that the area of the polar sector R (see...Ch. 14.4 - Mass of a Planar Lamina Explain when you should...Ch. 14.4 - Moment of InertiaDescribe what the moment of...Ch. 14.4 - Finding the Mass of a Lamina In Exercises 3-6,...Ch. 14.4 - Finding the Mass of a Lamina In Exercises 3-6,...Ch. 14.4 - Finding the Mass of a Lamina In Exercises 3-6,...Ch. 14.4 - Prob. 6ECh. 14.4 - Prob. 7ECh. 14.4 - Prob. 8ECh. 14.4 - Prob. 9ECh. 14.4 - Prob. 10ECh. 14.4 - Prob. 11ECh. 14.4 - Prob. 12ECh. 14.4 - Prob. 13ECh. 14.4 - Prob. 14ECh. 14.4 - Prob. 15ECh. 14.4 - Prob. 16ECh. 14.4 - Prob. 17ECh. 14.4 - Prob. 18ECh. 14.4 - Prob. 19ECh. 14.4 - Prob. 20ECh. 14.4 - Prob. 21ECh. 14.4 - Prob. 22ECh. 14.4 - Prob. 23ECh. 14.4 - Prob. 24ECh. 14.4 - Finding the Center of Mass Using Technology In...Ch. 14.4 - Prob. 26ECh. 14.4 - Prob. 27ECh. 14.4 - Prob. 28ECh. 14.4 - Prob. 29ECh. 14.4 - Prob. 30ECh. 14.4 - Prob. 31ECh. 14.4 - Prob. 32ECh. 14.4 - Finding the Radius of Gyration About Each Axis in...Ch. 14.4 - Prob. 34ECh. 14.4 - Prob. 35ECh. 14.4 - Prob. 36ECh. 14.4 - Prob. 37ECh. 14.4 - Finding Moments of Inertia and Radii of Gyration...Ch. 14.4 - Prob. 39ECh. 14.4 - Prob. 40ECh. 14.4 - Prob. 41ECh. 14.4 - Prob. 42ECh. 14.4 - Prob. 43ECh. 14.4 - Prob. 44ECh. 14.4 - Prob. 45ECh. 14.4 - Prob. 46ECh. 14.4 - Prob. 47ECh. 14.4 - HOW DO YOU SEE IT? The center of mass of the...Ch. 14.4 - Prob. 49ECh. 14.5 - CONCEPT CHECK Surface Area What is the...Ch. 14.5 - CONCEPT CHECK Numerical Integration Write a double...Ch. 14.5 - Finding Surface AreaIn Exercises 316, find the...Ch. 14.5 - Finding Surface AreaIn Exercises 316, find the...Ch. 14.5 - Finding Surface Area In Exercises 3-16, find the...Ch. 14.5 - Prob. 6ECh. 14.5 - Finding Surface AreaIn Exercises 316, find the...Ch. 14.5 - Prob. 8ECh. 14.5 - Prob. 9ECh. 14.5 - Prob. 10ECh. 14.5 - Prob. 11ECh. 14.5 - Prob. 12ECh. 14.5 - Prob. 13ECh. 14.5 - Prob. 14ECh. 14.5 - Prob. 15ECh. 14.5 - Prob. 16ECh. 14.5 - Finding Surface Area In Exercises 17-20, find the...Ch. 14.5 - Finding Surface Area In Exercises 17-20, find the...Ch. 14.5 - Finding Surface Area In Exercises 17-20, find the...Ch. 14.5 - Prob. 20ECh. 14.5 - Prob. 21ECh. 14.5 - Prob. 22ECh. 14.5 - Prob. 23ECh. 14.5 - Prob. 24ECh. 14.5 - Prob. 25ECh. 14.5 - Prob. 26ECh. 14.5 - Prob. 27ECh. 14.5 - Prob. 28ECh. 14.5 - Prob. 29ECh. 14.5 - Prob. 30ECh. 14.5 - Prob. 31ECh. 14.5 - HOW DO YOU SEE IT? Consider the surface...Ch. 14.5 - Prob. 33ECh. 14.5 - Prob. 34ECh. 14.5 - Product DesignA company produces a spherical...Ch. 14.5 - Modeling Data A company builds a ware house with...Ch. 14.5 - Prob. 37ECh. 14.5 - Prob. 38ECh. 14.6 - CONCEPT CHECK Triple Integrals What does Q=QdV...Ch. 14.6 - Prob. 2ECh. 14.6 - Evaluating a Triple Iterated Integral In Exercises...Ch. 14.6 - Evaluating a Triple Iterated Integral In Exercises...Ch. 14.6 - Prob. 5ECh. 14.6 - Prob. 6ECh. 14.6 - Prob. 7ECh. 14.6 - Prob. 8ECh. 14.6 - Prob. 9ECh. 14.6 - Prob. 10ECh. 14.6 - Evaluating a Triple Iterated Integral Using...Ch. 14.6 - Evaluating a Triple Iterated Integral Using...Ch. 14.6 - Setting Up a Triple IntegralIn Exercises 13-18,...Ch. 14.6 - Setting Up a Triple IntegralIn Exercises 13-18,...Ch. 14.6 - Setting Up a Triple IntegralIn Exercises 13-18,...Ch. 14.6 - Setting Up a Triple IntegralIn Exercises 13-18,...Ch. 14.6 - Prob. 17ECh. 14.6 - Prob. 18ECh. 14.6 - Volume In Exercises 19-24, use a triple integral...Ch. 14.6 - Volume In Exercises 19-24, use a triple integral...Ch. 14.6 - Volume In Exercises 19-24, use a triple integral...Ch. 14.6 - Volume In Exercises 19-24, use a triple integral...Ch. 14.6 - Volume In Exercises 19-24, use a triple integral...Ch. 14.6 - Volume In Exercises 19-24, use a triple integral...Ch. 14.6 - Changing the Order of integration In Exercises...Ch. 14.6 - Changing the Order of integration In Exercises...Ch. 14.6 - Prob. 27ECh. 14.6 - Prob. 28ECh. 14.6 - Changing the Order of Integration In Exercises...Ch. 14.6 - Changing the Order of integration In Exercises...Ch. 14.6 - Orders of Integration In Exercises 31-34, write a...Ch. 14.6 - Orders of Integration In Exercises 31-34, write a...Ch. 14.6 - Prob. 33ECh. 14.6 - Prob. 34ECh. 14.6 - Prob. 35ECh. 14.6 - Orders of Integration In Exercises 35 and 36, the...Ch. 14.6 - Prob. 37ECh. 14.6 - Prob. 38ECh. 14.6 - Prob. 39ECh. 14.6 - Center of Mass In Exercises 37-40, find the mass...Ch. 14.6 - Center of Mass In Exercises 41 and 42, set up the...Ch. 14.6 - Prob. 42ECh. 14.6 - Think About It The center of mass of a solid of...Ch. 14.6 - Prob. 44ECh. 14.6 - Think About It The center of mass of a solid of...Ch. 14.6 - Think About It The center of mass of a solid of...Ch. 14.6 - Centroid In Exercises 47-52, find the centroid of...Ch. 14.6 - Centroid In Exercises 47-52, find the centroid of...Ch. 14.6 - Prob. 49ECh. 14.6 - Centroid In Exercises 47-52, find the centroid of...Ch. 14.6 - Prob. 51ECh. 14.6 - Prob. 52ECh. 14.6 - Moments of Inertia In Exercises 53- 56, find...Ch. 14.6 - Moments of Inertia In Exercises 53- 56, find...Ch. 14.6 - Moments of Inertia In Exercises 53- 56, find...Ch. 14.6 - Prob. 56ECh. 14.6 - Prob. 57ECh. 14.6 - Prob. 58ECh. 14.6 - Moments of Inertia In Exercises 59 and 60, set up...Ch. 14.6 - Moments of Inertia In Exercises 59 and 60, set up...Ch. 14.6 - Prob. 61ECh. 14.6 - Prob. 62ECh. 14.6 - Prob. 63ECh. 14.6 - Prob. 64ECh. 14.6 - Prob. 65ECh. 14.6 - Prob. 66ECh. 14.6 - Prob. 67ECh. 14.6 - Prob. 68ECh. 14.6 - Prob. 69ECh. 14.6 - Prob. 70ECh. 14.6 - Prob. 71ECh. 14.6 - Prob. 72ECh. 14.6 - Prob. 73ECh. 14.7 - CONCEPT CHECK Volume Explain why triple integrals...Ch. 14.7 - CONCEPT CHECK Differential of Volume What is the...Ch. 14.7 - Prob. 3ECh. 14.7 - Prob. 4ECh. 14.7 - Prob. 5ECh. 14.7 - Prob. 6ECh. 14.7 - Prob. 7ECh. 14.7 - Prob. 8ECh. 14.7 - Prob. 9ECh. 14.7 - Prob. 10ECh. 14.7 - Prob. 11ECh. 14.7 - VolumeIn Exercises 1114, sketch the solid region...Ch. 14.7 - Volume In Exercises 11-14, sketch the solid region...Ch. 14.7 - Volume In Exercises 11-14, sketch the solid region...Ch. 14.7 - Prob. 15ECh. 14.7 - Volume In Exercises 15-20, use cylindrical...Ch. 14.7 - VolumeIn Exercises 1520, use cylindrical...Ch. 14.7 - Volume In Exercises 15-20, use cylindrical...Ch. 14.7 - VolumeIn Exercises 1520, use cylindrical...Ch. 14.7 - Volume In Exercises 15-20, use cylindrical...Ch. 14.7 - Prob. 21ECh. 14.7 - Prob. 22ECh. 14.7 - Prob. 23ECh. 14.7 - Prob. 24ECh. 14.7 - Prob. 27ECh. 14.7 - Prob. 29ECh. 14.7 - VolumeIn Exercises 3134, use spherical coordinates...Ch. 14.7 - VolumeIn Exercises 3134, use spherical coordinates...Ch. 14.7 - Prob. 33ECh. 14.7 - Prob. 34ECh. 14.7 - Prob. 35ECh. 14.7 - MassIn Exercises 35 and 36, use spherical...Ch. 14.7 - Prob. 37ECh. 14.7 - Center of MassIn Exercises 37 and 38, use...Ch. 14.7 - Prob. 39ECh. 14.7 - Prob. 40ECh. 14.7 - Converting CoordinatesIn Exercises 4144, convert...Ch. 14.7 - Converting CoordinatesIn Exercises 4144, convert...Ch. 14.7 - Converting CoordinatesIn Exercises 4144, convert...Ch. 14.7 - Prob. 45ECh. 14.7 - HOW DO YOU SEE IT? The solid is bounded below by...Ch. 14.7 - Prob. 47ECh. 14.8 - CONCEPT CHECK JacobianDescribe how to find the...Ch. 14.8 - CONCEPT CHECK Change of VariableWhen is it...Ch. 14.8 - Prob. 3ECh. 14.8 - Prob. 4ECh. 14.8 - Prob. 5ECh. 14.8 - Prob. 6ECh. 14.8 - Prob. 7ECh. 14.8 - Prob. 8ECh. 14.8 - Prob. 9ECh. 14.8 - Prob. 10ECh. 14.8 - Prob. 11ECh. 14.8 - Using a Transformation In Exercises 11-14, sketch...Ch. 14.8 - Prob. 13ECh. 14.8 - Using a Transformation In Exercises 11-14, sketch...Ch. 14.8 - Prob. 15ECh. 14.8 - Prob. 16ECh. 14.8 - Prob. 17ECh. 14.8 - Evaluating a Double Integral Using a Change of...Ch. 14.8 - Evaluating a Double Integral Using a Change of...Ch. 14.8 - Evaluating a Double Integral Using a Change of...Ch. 14.8 - Evaluating a Double Integral Using a Change of...Ch. 14.8 - Evaluating a Double Integral Using a Change of...Ch. 14.8 - Prob. 23ECh. 14.8 - Prob. 24ECh. 14.8 - Finding Volume Using a Change of Variables In...Ch. 14.8 - Finding Volume Using a Change of Variables In...Ch. 14.8 - Prob. 27ECh. 14.8 - Finding Volume Using a Change of Variables In...Ch. 14.8 - Prob. 29ECh. 14.8 - Prob. 30ECh. 14.8 - Prob. 31ECh. 14.8 - Prob. 32ECh. 14.8 - Prob. 33ECh. 14.8 - VolumeUse the result of Exercise 33 to find the...Ch. 14.8 - Prob. 35ECh. 14.8 - Prob. 36ECh. 14.8 - Prob. 37ECh. 14.8 - Prob. 38ECh. 14.8 - Prob. 39ECh. 14.8 - Prob. 40ECh. 14.8 - Prob. 41ECh. 14 - Prob. 1RECh. 14 - Prob. 2RECh. 14 - Prob. 3RECh. 14 - Prob. 4RECh. 14 - Prob. 5RECh. 14 - Prob. 6RECh. 14 - Prob. 7RECh. 14 - Prob. 8RECh. 14 - Prob. 9RECh. 14 - Prob. 10RECh. 14 - Prob. 11RECh. 14 - Prob. 12RECh. 14 - Prob. 13RECh. 14 - Prob. 14RECh. 14 - Prob. 15RECh. 14 - Prob. 16RECh. 14 - Finding Volume In Exercises 17-20, use a double...Ch. 14 - Prob. 18RECh. 14 - Prob. 19RECh. 14 - Prob. 20RECh. 14 - Prob. 21RECh. 14 - Prob. 22RECh. 14 - Prob. 23RECh. 14 - Prob. 24RECh. 14 - Converting to Polar CoordinatesIn Exercises 25 and...Ch. 14 - Prob. 26RECh. 14 - VolumeIn Exercises 27 and 28, use a double...Ch. 14 - Prob. 28RECh. 14 - Prob. 29RECh. 14 - Prob. 30RECh. 14 - Prob. 31RECh. 14 - Prob. 32RECh. 14 - Area and VolumeConsider the region R in the xy...Ch. 14 - Converting to Polar Coordinates Write the sum of...Ch. 14 - Prob. 35RECh. 14 - Prob. 36RECh. 14 - Finding the Center of MassIn Exercises 3740, find...Ch. 14 - Prob. 38RECh. 14 - Prob. 39RECh. 14 - Prob. 40RECh. 14 - Prob. 41RECh. 14 - Prob. 42RECh. 14 - Prob. 43RECh. 14 - Finding Surface AreaIn Exercises 4346, find the...Ch. 14 - Prob. 45RECh. 14 - Prob. 46RECh. 14 - Building DesignA new auditorium is built with a...Ch. 14 - Prob. 48RECh. 14 - Prob. 49RECh. 14 - Prob. 50RECh. 14 - Prob. 51RECh. 14 - Prob. 52RECh. 14 - Prob. 53RECh. 14 - Prob. 54RECh. 14 - VolumeIn Exercises 55 and 56, use a triple...Ch. 14 - Prob. 56RECh. 14 - Prob. 57RECh. 14 - Prob. 59RECh. 14 - Prob. 60RECh. 14 - Prob. 61RECh. 14 - Prob. 62RECh. 14 - Prob. 63RECh. 14 - Prob. 64RECh. 14 - Prob. 65RECh. 14 - Prob. 66RECh. 14 - VolumeIn Exercises 67 and 68, use cylindrical...Ch. 14 - Prob. 68RECh. 14 - Prob. 69RECh. 14 - Prob. 70RECh. 14 - Prob. 71RECh. 14 - Prob. 72RECh. 14 - Finding a JcobianIn Exercises 7174, find the...Ch. 14 - Prob. 74RECh. 14 - Prob. 75RECh. 14 - Evaluating a Double Integral Using a Change of...Ch. 14 - Prob. 77RECh. 14 - Prob. 78RECh. 14 - Prob. 1PSCh. 14 - Prob. 2PSCh. 14 - Prob. 3PSCh. 14 - Prob. 4PSCh. 14 - Prob. 5PSCh. 14 - Prob. 6PSCh. 14 - Prob. 7PSCh. 14 - Prob. 8PSCh. 14 - Prob. 9PSCh. 14 - Prob. 10PSCh. 14 - Prob. 11PSCh. 14 - Prob. 12PSCh. 14 - Prob. 14PSCh. 14 - Prob. 15PSCh. 14 - Prob. 16PSCh. 14 - Prob. 18PS
Knowledge Booster
Background pattern image
Calculus
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
Calculus: Early Transcendentals
Calculus
ISBN:9781285741550
Author:James Stewart
Publisher:Cengage Learning
Text book image
Thomas' Calculus (14th Edition)
Calculus
ISBN:9780134438986
Author:Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:PEARSON
Text book image
Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:9780134763644
Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:PEARSON
Text book image
Calculus: Early Transcendentals
Calculus
ISBN:9781319050740
Author:Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:W. H. Freeman
Text book image
Precalculus
Calculus
ISBN:9780135189405
Author:Michael Sullivan
Publisher:PEARSON
Text book image
Calculus: Early Transcendental Functions
Calculus
ISBN:9781337552516
Author:Ron Larson, Bruce H. Edwards
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
Introduction to Triple Integrals; Author: Mathispower4u;https://www.youtube.com/watch?v=CPR0ZD0IYVE;License: Standard YouTube License, CC-BY