
University Calculus: Early Transcendentals (4th Edition)
4th Edition
ISBN: 9780134995540
Author: Joel R. Hass, Christopher E. Heil, Przemyslaw Bogacki, Maurice D. Weir, George B. Thomas Jr.
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Textbook Question
Chapter 14.4, Problem 2E
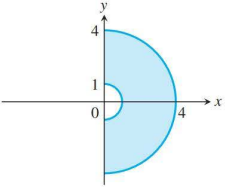
In Exercises 1-8, describe the given region in polar coordinates.
2.
Expert Solution & Answer
Learn your wayIncludes step-by-step video

schedule01:10
Students have asked these similar questions
Does the series converge or diverge
Does the series converge or diverge
Diverge or conver
Chapter 14 Solutions
University Calculus: Early Transcendentals (4th Edition)
Ch. 14.1 - In Exercises 1-14. evaluate the iterated...Ch. 14.1 - Prob. 2ECh. 14.1 - Prob. 3ECh. 14.1 - In Exercises 1-14, evaluate the iterated...Ch. 14.1 - In Exercises 1-14, evaluate the iterated...Ch. 14.1 - In Exercises 1-14, evaluate the iterated...Ch. 14.1 - In Exercises 1-14, evaluate the iterated integral....Ch. 14.1 - In Exercises 1-14, evaluate the iterated...Ch. 14.1 - In Exercises 1-14, evaluate the iterated integral....Ch. 14.1 - Prob. 10E
Ch. 14.1 - In Exercises 1-14. evaluate the iterated integral....Ch. 14.1 - In Exercises 1-14. evaluate the iterated...Ch. 14.1 - In Exercises 1–14, evaluate the iterated...Ch. 14.1 - In Exercises 1–14, evaluate the iterated...Ch. 14.1 - Find all values of the constant c so that
Ch. 14.1 - Prob. 16ECh. 14.1 - In Exercises 17-24, evaluate the double integral...Ch. 14.1 - In Exercises 17-24, evaluate the double integral...Ch. 14.1 - In Exercises 17-24, evaluate the double integral...Ch. 14.1 - Prob. 20ECh. 14.1 - In Exercises 17–24, evaluate the double integral...Ch. 14.1 - In Exercises 17–24, evaluate the double integral...Ch. 14.1 - In Exercises 17–24, evaluate the double integral...Ch. 14.1 - In Exercises 17–24, evaluate the double integral...Ch. 14.1 - In Exercises 25 and 26, integrate f over the given...Ch. 14.1 - In Exercises 25 and 26, integrate f over the given...Ch. 14.1 - In Exercises 27 and 28, sketch the solid whose...Ch. 14.1 - Prob. 28ECh. 14.1 - Find the volume of the region hounded above by the...Ch. 14.1 - Find the volume of the region bounded above by the...Ch. 14.1 - Prob. 31ECh. 14.1 - Prob. 32ECh. 14.1 - Prob. 33ECh. 14.1 - Prob. 34ECh. 14.1 - Find a value of the constant k so that
Ch. 14.1 - Prob. 36ECh. 14.1 - Prob. 37ECh. 14.1 - Prob. 38ECh. 14.1 - Prob. 39ECh. 14.1 - Prob. 40ECh. 14.2 - In Exercises 1-8, sketch the described regions of...Ch. 14.2 - Prob. 2ECh. 14.2 - Prob. 3ECh. 14.2 - In Exercises 1-8, sketch the described regions of...Ch. 14.2 - In Exercises 1-8, sketch the described regions of...Ch. 14.2 - In Exercises 1-8, sketch the described regions of...Ch. 14.2 - In Exercises 1-8, sketch the described regions of...Ch. 14.2 - In Exercises 1-8, sketch the described regions of...Ch. 14.2 - In Exercises 9–18, write an iterated integral for ...Ch. 14.2 - In Exercises 9–18, write an iterated integral for ...Ch. 14.2 - In Exercises 9–18, write an iterated integral for ...Ch. 14.2 - In Exercises 9–18, write an iterated integral for ...Ch. 14.2 - In Exercises 9–18, write an iterated integral for ...Ch. 14.2 - Prob. 14ECh. 14.2 - In Exercises 9–18, write an iterated integral for ...Ch. 14.2 - In Exercises 9-18, write an iterated integral for...Ch. 14.2 - In Exercises 9-18, write an iterated integral for...Ch. 14.2 - In Exercises 9–18, write an iterated integral for ...Ch. 14.2 - Finding Regions of Integration and Double...Ch. 14.2 - Finding Regions of Integration and Double...Ch. 14.2 - In Exercises 19–24, sketch the region of...Ch. 14.2 - Prob. 22ECh. 14.2 - In Exercises 19–24, sketch the region of...Ch. 14.2 - Prob. 24ECh. 14.2 - In Exercises 25-28, integrate f over the given...Ch. 14.2 - Prob. 26ECh. 14.2 - Prob. 27ECh. 14.2 - In Exercises 25–28, integrate f over the given...Ch. 14.2 - Prob. 29ECh. 14.2 - Prob. 30ECh. 14.2 - Each of Exercises 29–32 gives an integral over a...Ch. 14.2 - Prob. 32ECh. 14.2 - In Exercises 33–46, sketch the region of...Ch. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - Prob. 40ECh. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - Prob. 44ECh. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - Prob. 46ECh. 14.2 - In Exercises 33-46, sketch the region of...Ch. 14.2 - Prob. 48ECh. 14.2 - In Exercises 47-56, sketch the region of...Ch. 14.2 - Prob. 50ECh. 14.2 - In Exercises 47-56, sketch the region of...Ch. 14.2 - Prob. 52ECh. 14.2 - In Exercises 47-56, sketch the region of...Ch. 14.2 - Prob. 54ECh. 14.2 - In Exercises 47–56, sketch the region of...Ch. 14.2 - Prob. 56ECh. 14.2 - Find the volume of the region bounded above by the...Ch. 14.2 - Prob. 58ECh. 14.2 - Find the volume of the solid whose base is the...Ch. 14.2 - Prob. 60ECh. 14.2 - Find the volume of the solid in the first octant...Ch. 14.2 - Prob. 62ECh. 14.2 - Find the volume of the wedge cut from the first...Ch. 14.2 - Prob. 64ECh. 14.2 - Find the volume of the solid that is bounded on...Ch. 14.2 - Prob. 66ECh. 14.2 - In Exercises 67 and 68, sketch the region of...Ch. 14.2 - Prob. 68ECh. 14.2 - Prob. 69ECh. 14.2 - Prob. 70ECh. 14.2 - Prob. 71ECh. 14.2 - Prob. 72ECh. 14.2 - In Exercises 73 and 74, approximate the double...Ch. 14.2 - Prob. 74ECh. 14.2 - Circular sector Integrate over the smaller sector...Ch. 14.2 - Unbounded region Integrate f(x, y) = 1/ [(x2 –...Ch. 14.2 - Noncircular cylinder A solid right (noncircular)...Ch. 14.2 - Prob. 78ECh. 14.2 - Maximizing a double integral What region R in the...Ch. 14.2 - Minimizing a double integral What region R in the...Ch. 14.2 - Is it possible to evaluate the integral of a...Ch. 14.2 - How would you evaluate the double integral of a...Ch. 14.2 - Prob. 83ECh. 14.2 - Prob. 84ECh. 14.3 - In Exercises 1-12, sketch the region bounded by...Ch. 14.3 - Prob. 2ECh. 14.3 - In Exercises 1-12, sketch the region bounded by...Ch. 14.3 - In Exercises 1-12, sketch the region bounded by...Ch. 14.3 - In Exercises 1-12, sketch the region bounded by...Ch. 14.3 - Prob. 6ECh. 14.3 - In Exercises 1-12, sketch the region bounded by...Ch. 14.3 - Prob. 8ECh. 14.3 - In Exercises 1-12, sketch the region bounded by...Ch. 14.3 - Prob. 10ECh. 14.3 - Prob. 11ECh. 14.3 - In Exercises 1-12, sketch the region bounded by...Ch. 14.3 - The integrals and sums of integrals in Exercises...Ch. 14.3 - Prob. 14ECh. 14.3 - The integrals and sums of integrals in Exercises...Ch. 14.3 - The integrals and sums of integrals in Exercises...Ch. 14.3 - Prob. 17ECh. 14.3 - Prob. 18ECh. 14.3 - Find the average value of f(x, y) = sin(x + y)...Ch. 14.3 - Which do you think will be larger, the average...Ch. 14.3 - Find the average height of the paraboloid z = x2 +...Ch. 14.3 - Find the average value of f(x, y) = 1/(xy) over...Ch. 14.3 - Geometric area Find the area of the region
using...Ch. 14.3 - Prob. 24ECh. 14.3 - Bacterium population If f(x, y) = (10,000ey)/ (1 +...Ch. 14.3 - Prob. 26ECh. 14.3 - Average temperature in Texas According to the...Ch. 14.3 - Prob. 28ECh. 14.3 - Suppose f(x, y) is continuous over a region R in...Ch. 14.3 - Prob. 30ECh. 14.4 - In Exercises 1-8, describe the given region in...Ch. 14.4 - In Exercises 1-8, describe the given region in...Ch. 14.4 - In Exercises 1-8, describe the given region in...Ch. 14.4 - In Exercises 1-8, describe the given region in...Ch. 14.4 - In Exercises 1-8, describe the given region in...Ch. 14.4 - In Exercises 1-8, describe the given region in...Ch. 14.4 - In Exercises 1-8, describe the given region in...Ch. 14.4 - In Exercises 1-8, describe the given region in...Ch. 14.4 -
In Exercises 9-22, change the Cartesian integral...Ch. 14.4 - In Exercises 9-22, change the Cartesian integral...Ch. 14.4 - In Exercises 9-22, change the Cartesian integral...Ch. 14.4 - In Exercises 9-22, change the Cartesian integral...Ch. 14.4 - In Exercises 9-22, change the Cartesian integral...Ch. 14.4 - In Exercises 9-22, change the Cartesian integral...Ch. 14.4 - In Exercises 9-22, change the Cartesian integral...Ch. 14.4 - In Exercises 9-22, change the Cartesian integral...Ch. 14.4 - In Exercises 9-22, change the Cartesian integral...Ch. 14.4 - Prob. 18ECh. 14.4 - In Exercises 9-22, change the Cartesian integral...Ch. 14.4 - Prob. 20ECh. 14.4 - In Exercises 9–22, change the Cartesian integral...Ch. 14.4 - In Exercises 9–22, change the Cartesian integral...Ch. 14.4 - In Exercises 23-26, sketch the region of...Ch. 14.4 - In Exercises 23–26, sketch the region of...Ch. 14.4 - In Exercises 23–26, sketch the region of...Ch. 14.4 - In Exercises 23–26, sketch the region of...Ch. 14.4 - Find the area of the region cut from the first...Ch. 14.4 - Prob. 28ECh. 14.4 - One leaf of a rose Find the area enclosed by one...Ch. 14.4 - Prob. 30ECh. 14.4 - Prob. 31ECh. 14.4 - Overlapping cardioids Find the area of the region...Ch. 14.4 - In polar coordinates, the average value of a...Ch. 14.4 - Prob. 34ECh. 14.4 - In polar coordinates, the average value of a...Ch. 14.4 - Prob. 36ECh. 14.4 - Converting to a polar integral Integrate over the...Ch. 14.4 - Prob. 38ECh. 14.4 - Volume of noncircular right cylinder The region...Ch. 14.4 - Prob. 40ECh. 14.4 - Prob. 41ECh. 14.4 - Prob. 42ECh. 14.4 - Prob. 43ECh. 14.4 - Area formula in polar coordinates Use the double...Ch. 14.4 - Prob. 45ECh. 14.4 - Prob. 46ECh. 14.4 - Evaluate the integral , where R is the region...Ch. 14.4 - Prob. 48ECh. 14.5 - Evaluate the integral in Example 3, taking F(x, y,...Ch. 14.5 - Prob. 2ECh. 14.5 - Volume of tetrahedron Write six different iterated...Ch. 14.5 - Prob. 4ECh. 14.5 - Volume enclosed by paraboloids Let D be the region...Ch. 14.5 - Prob. 6ECh. 14.5 - Evaluate the integrals in Exercises 7–20.
7.
Ch. 14.5 - Evaluate the integrals in Exercises 7–20.
8.
Ch. 14.5 - Evaluate the integrals in Exercises 7–20.
9.
Ch. 14.5 - Evaluate the integrals in Exercises 7–20.
10.
Ch. 14.5 - Evaluate the integrals in Exercises 7–20.
11.
Ch. 14.5 - Evaluate the integrals in Exercises 7–20.
12.
Ch. 14.5 - Evaluate the integrals in Exercises 7–20.
13.
Ch. 14.5 - Prob. 14ECh. 14.5 - Evaluate the integrals in Exercises 7–20.
15.
Ch. 14.5 - Prob. 16ECh. 14.5 - Evaluate the integrals in Exercises 7–20.
17.
Ch. 14.5 - Evaluate the integrals in Exercises 7–20.
18.
Ch. 14.5 - Evaluate the integrals in Exercises 7–20.
19.
Ch. 14.5 - Prob. 20ECh. 14.5 - Here is the region of integration of the integral...Ch. 14.5 - Here is the region of integration of the...Ch. 14.5 - Find the volumes of the regions in Exercises...Ch. 14.5 - Find the volumes of the regions in Exercises...Ch. 14.5 - Find the volumes of the regions in Exercises...Ch. 14.5 - Find the volumes of the regions in Exercises 2336....Ch. 14.5 - Find the volumes of the regions in Exercises 2336....Ch. 14.5 - Prob. 28ECh. 14.5 - Find the volumes of the regions in Exercises...Ch. 14.5 - Find the volumes of the regions in Exercises...Ch. 14.5 - Find the volumes of the regions in Exercises...Ch. 14.5 - Prob. 32ECh. 14.5 - Find the volumes of the regions in Exercises...Ch. 14.5 - Prob. 34ECh. 14.5 - The region cut from the solid elliptical cylinder...Ch. 14.5 - Prob. 36ECh. 14.5 - In Exercises 37–40, find the average value of F(x,...Ch. 14.5 - Prob. 38ECh. 14.5 - In Exercises 37–40, find the average value of F(x,...Ch. 14.5 - Prob. 40ECh. 14.5 - Evaluate the integrals in Exercises 41–44 by...Ch. 14.5 - Evaluate the integrals in Exercises 41–44 by...Ch. 14.5 - Evaluate the integrals in Exercises 41–44 by...Ch. 14.5 - Evaluate the integrals in Exercises 41–44 by...Ch. 14.5 - Finding an upper limit of an iterated integral...Ch. 14.5 - Prob. 46ECh. 14.5 - Minimizing a triple integral What domain D in...Ch. 14.5 - Maximizing a triple integral What domain D in...Ch. 14.6 - Finding a center of mass find the center of mass...Ch. 14.6 - Prob. 2ECh. 14.6 - Finding a centroid Find the centroid of the region...Ch. 14.6 - Prob. 4ECh. 14.6 - Prob. 5ECh. 14.6 - Finding a centroid Find the centroid of the region...Ch. 14.6 - Prob. 7ECh. 14.6 - Prob. 8ECh. 14.6 - The centroid of an infinite region Find the...Ch. 14.6 - Prob. 10ECh. 14.6 - Prob. 11ECh. 14.6 - Prob. 12ECh. 14.6 - Finding a center of mass Find the center of mass...Ch. 14.6 - Prob. 14ECh. 14.6 - Prob. 15ECh. 14.6 - Prob. 16ECh. 14.6 - Center of mass, moment of inertia Find the center...Ch. 14.6 - Prob. 18ECh. 14.6 - Prob. 19ECh. 14.6 - Prob. 20ECh. 14.6 - Moments of inertia Find the moments of inertia of...Ch. 14.6 - Prob. 22ECh. 14.6 - Center of mass and moments of inertia A solid...Ch. 14.6 - Prob. 24ECh. 14.6 - a. Center of mass Find the center of mass of a...Ch. 14.6 - Prob. 26ECh. 14.6 - Moment of inertia about a line A wedge like the...Ch. 14.6 - Prob. 28ECh. 14.6 - In Exercises 29 and 30, find
the mass of the...Ch. 14.6 - In Exercises 29 and 30, find
a. the mass of the...Ch. 14.6 - In Exercises 31 and 32, find
the mass of the...Ch. 14.6 - Prob. 32ECh. 14.6 - Mass Find the mass of the solid bounded by the...Ch. 14.6 - Prob. 34ECh. 14.7 - In Exercises 1–12, sketch the region described by...Ch. 14.7 - In Exercises 1–12, sketch the region described by...Ch. 14.7 - In Exercises 1–12, sketch the region described by...Ch. 14.7 - In Exercises 1–12, sketch the region described by...Ch. 14.7 - In Exercises 1–12, sketch the region described by...Ch. 14.7 - Prob. 6ECh. 14.7 - In Exercises 1–12, sketch the region described by...Ch. 14.7 - In Exercises 1–12, sketch the region described by...Ch. 14.7 - In Exercises 1–12, sketch the region described by...Ch. 14.7 - Prob. 10ECh. 14.7 - In Exercises 1–12, sketch the region described by...Ch. 14.7 - Prob. 12ECh. 14.7 - In Exercises 13−22, sketch the region described by...Ch. 14.7 - In Exercises 13−22, sketch the region described by...Ch. 14.7 - In Exercises 13−22, sketch the region described by...Ch. 14.7 - Prob. 16ECh. 14.7 - In Exercises 13−22, sketch the region described by...Ch. 14.7 - Prob. 18ECh. 14.7 - Prob. 19ECh. 14.7 - In Exercises 13−22, sketch the region described by...Ch. 14.7 - In Exercises 13−22, sketch the region described by...Ch. 14.7 - Prob. 22ECh. 14.7 - Evaluate the cylindrical coordinate integrals in...Ch. 14.7 - Evaluate the cylindrical coordinate integrals in...Ch. 14.7 - Evaluate the cylindrical coordinate integrals in...Ch. 14.7 - Prob. 26ECh. 14.7 - Evaluate the cylindrical coordinate integrals in...Ch. 14.7 - Prob. 28ECh. 14.7 - The integrals we have seen so far suggest that...Ch. 14.7 - Prob. 30ECh. 14.7 - Prob. 31ECh. 14.7 - Prob. 32ECh. 14.7 - Let D be the region bounded below by the plane z =...Ch. 14.7 - Let D be the region bounded below by the cone and...Ch. 14.7 - Give the limits of integration for evaluating the...Ch. 14.7 - Convert the integral
to an equivalent integral in...Ch. 14.7 - In Exercises 37–42, set up the iterated integral...Ch. 14.7 - In Exercises 37–42, set up the iterated integral...Ch. 14.7 - In Exercises 37–42, set up the iterated integral...Ch. 14.7 - In Exercises 37–42, set up the iterated integral...Ch. 14.7 - In Exercises 37–42, set up the iterated integral...Ch. 14.7 - Prob. 42ECh. 14.7 - Evaluate the spherical coordinate integrals in...Ch. 14.7 - Evaluate the spherical coordinate integrals in...Ch. 14.7 - Evaluate the spherical coordinate integrals in...Ch. 14.7 - Prob. 46ECh. 14.7 - Evaluate the spherical coordinate integrals in...Ch. 14.7 - Prob. 48ECh. 14.7 - The previous integrals suggest there are preferred...Ch. 14.7 - The previous integrals suggest there are preferred...Ch. 14.7 - The previous integrals suggest there are preferred...Ch. 14.7 - Prob. 52ECh. 14.7 - Let D be the region in Exercise 33. Set up the...Ch. 14.7 - Let D be the region bounded below by the cone and...Ch. 14.7 - In Exercises 55–60, (a) find the spherical...Ch. 14.7 - In Exercises 55–60, (a) find the spherical...Ch. 14.7 - In Exercises 55–60, (a) find the spherical...Ch. 14.7 - Prob. 58ECh. 14.7 - In Exercises 55–60, (a) find the spherical...Ch. 14.7 - In Exercises 55–60, (a) find the spherical...Ch. 14.7 - Set up triple integrals for the volume of the...Ch. 14.7 - Prob. 62ECh. 14.7 - Let D be the smaller cap cut from a solid ball of...Ch. 14.7 - Prob. 64ECh. 14.7 - Find the volumes of the solids in Exercises...Ch. 14.7 - Find the volumes of the solids in Exercises...Ch. 14.7 - Find the volumes of the solids in Exercises...Ch. 14.7 - Prob. 68ECh. 14.7 - Find the volumes of the solids in Exercises...Ch. 14.7 - Prob. 70ECh. 14.7 - Sphere and cones Find the volume of the portion of...Ch. 14.7 - Prob. 72ECh. 14.7 - Prob. 73ECh. 14.7 - Prob. 74ECh. 14.7 - Cylinder and paraboloid Find the volume of the...Ch. 14.7 - Cylinder and paraboloids Find the volume of the...Ch. 14.7 - Prob. 77ECh. 14.7 - Prob. 78ECh. 14.7 - Prob. 79ECh. 14.7 - Prob. 80ECh. 14.7 - Region trapped by paraboloids Find the volume of...Ch. 14.7 - Paraboloid and cylinder Find the volume of the...Ch. 14.7 - Prob. 83ECh. 14.7 - Prob. 84ECh. 14.7 - Prob. 85ECh. 14.7 - Prob. 86ECh. 14.7 - Find the average value of the function f(, , ) = ...Ch. 14.7 - Find the average value of the function f(ρ, ϕ, θ)...Ch. 14.7 - Prob. 89ECh. 14.7 - Prob. 90ECh. 14.7 - Prob. 91ECh. 14.7 - Prob. 92ECh. 14.7 - Prob. 93ECh. 14.7 - Prob. 94ECh. 14.7 - Prob. 95ECh. 14.7 - Prob. 96ECh. 14.7 - Prob. 97ECh. 14.7 - Prob. 98ECh. 14.7 - Variable density A solid is bounded below by the...Ch. 14.7 - Variable density A solid ball is bounded by the...Ch. 14.7 - Prob. 101ECh. 14.7 - Prob. 102ECh. 14.7 - Prob. 103ECh. 14.7 - Mass of planet’s atmosphere A spherical planet of...Ch. 14.8 - Solve the system
for x and y in terms of u and v....Ch. 14.8 - Prob. 2ECh. 14.8 - Solve the system
for x and y in terms of u and v....Ch. 14.8 - Prob. 4ECh. 14.8 - Prob. 5ECh. 14.8 - Prob. 6ECh. 14.8 - Use the transformation in Exercise 3 to evaluate...Ch. 14.8 - Prob. 8ECh. 14.8 - Let R be the region in the first quadrant of the...Ch. 14.8 - Find the Jacobian of the transformation and...Ch. 14.8 - Prob. 11ECh. 14.8 - The area of an ellipse The area πab of the ellipse...Ch. 14.8 - Prob. 13ECh. 14.8 - Prob. 14ECh. 14.8 - Prob. 15ECh. 14.8 - Prob. 16ECh. 14.8 - Prob. 17ECh. 14.8 - Prob. 18ECh. 14.8 - Prob. 19ECh. 14.8 - Prob. 20ECh. 14.8 - Prob. 21ECh. 14.8 - Prob. 22ECh. 14.8 - Prob. 23ECh. 14.8 - Substitutions in single integrals How can...Ch. 14.8 - Prob. 25ECh. 14.8 - Prob. 26ECh. 14.8 - Prob. 27ECh. 14.8 - Prob. 28ECh. 14 - Prob. 1GYRCh. 14 - Prob. 2GYRCh. 14 - Prob. 3GYRCh. 14 - How can you change a double integral in...Ch. 14 - Prob. 5GYRCh. 14 - Prob. 6GYRCh. 14 - How are double and triple integrals in rectangular...Ch. 14 - Prob. 8GYRCh. 14 - How are triple integrals in cylindrical and...Ch. 14 - Prob. 10GYRCh. 14 - How are substitutions in triple integrals pictured...Ch. 14 - Prob. 1PECh. 14 - Prob. 2PECh. 14 - Prob. 3PECh. 14 - Prob. 4PECh. 14 - Prob. 5PECh. 14 - Prob. 6PECh. 14 - Prob. 7PECh. 14 - Prob. 8PECh. 14 - Prob. 9PECh. 14 - Prob. 10PECh. 14 - Prob. 11PECh. 14 - Prob. 12PECh. 14 - Prob. 13PECh. 14 - Prob. 14PECh. 14 - Prob. 15PECh. 14 - Prob. 16PECh. 14 - Prob. 17PECh. 14 - Prob. 18PECh. 14 - Prob. 19PECh. 14 - Prob. 20PECh. 14 - Prob. 21PECh. 14 - Prob. 22PECh. 14 - Prob. 23PECh. 14 - Prob. 24PECh. 14 - Prob. 25PECh. 14 - Prob. 26PECh. 14 - Prob. 27PECh. 14 - Prob. 28PECh. 14 - Prob. 29PECh. 14 - Prob. 30PECh. 14 - Prob. 31PECh. 14 - Prob. 32PECh. 14 - Prob. 33PECh. 14 - Prob. 34PECh. 14 - Prob. 35PECh. 14 - Prob. 36PECh. 14 - Prob. 37PECh. 14 - Prob. 38PECh. 14 - Prob. 39PECh. 14 - Prob. 40PECh. 14 - Prob. 41PECh. 14 - Prob. 42PECh. 14 - Prob. 43PECh. 14 - Prob. 44PECh. 14 - Prob. 45PECh. 14 - Prob. 46PECh. 14 - Prob. 47PECh. 14 - Prob. 48PECh. 14 - Prob. 49PECh. 14 - Prob. 50PECh. 14 - Prob. 51PECh. 14 - Centroid Find the centroid of the plane region...Ch. 14 - Prob. 53PECh. 14 - Prob. 54PECh. 14 - Prob. 1AAECh. 14 - Water in a hemispherical bowl A hemispherical bowl...Ch. 14 - Prob. 3AAECh. 14 - Prob. 4AAECh. 14 - Prob. 5AAECh. 14 - Prob. 6AAECh. 14 - Prob. 7AAECh. 14 - Prob. 8AAECh. 14 - Prob. 9AAECh. 14 - Prob. 10AAECh. 14 - Prob. 11AAECh. 14 - Prob. 12AAECh. 14 - Prob. 13AAECh. 14 - Prob. 14AAECh. 14 - Minimizing polar inertia A thin plate of constant...Ch. 14 - Prob. 16AAECh. 14 - Prob. 17AAECh. 14 - Centroid of a boomerang Find the centroid of the...Ch. 14 - Prob. 19AAECh. 14 - Prob. 20AAECh. 14 - Prob. 21AAECh. 14 - Prob. 22AAECh. 14 - Prob. 23AAECh. 14 - Prob. 24AAECh. 14 - Prob. 25AAECh. 14 - Prob. 26AAECh. 14 - Prob. 27AAECh. 14 - Prob. 28AAE
Additional Math Textbook Solutions
Find more solutions based on key concepts
Constructing and Graphing Discrete Probability Distributions In Exercises 19 and 20, (a) construct a probabilit...
Elementary Statistics: Picturing the World (7th Edition)
CHECK POINT I Let p and q represent the following statements: p : 3 + 5 = 8 q : 2 × 7 = 20. Determine the truth...
Thinking Mathematically (6th Edition)
To compute the area of the circle.
Pre-Algebra Student Edition
Retirement Income Several times during the year, the U.S. Census Bureau takes random samples from the populatio...
Introductory Statistics
3. Unbiased Estimators Data Set 4 “Births” in Appendix B includes birth weights of 400 babies. If we compute th...
Elementary Statistics (13th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- Can you help explain what I did based on partial fractions decomposition?arrow_forwardSuppose that a particle moves along a straight line with velocity v (t) = 62t, where 0 < t <3 (v(t) in meters per second, t in seconds). Find the displacement d (t) at time t and the displacement up to t = 3. d(t) ds = ["v (s) da = { The displacement up to t = 3 is d(3)- meters.arrow_forwardLet f (x) = x², a 3, and b = = 4. Answer exactly. a. Find the average value fave of f between a and b. fave b. Find a point c where f (c) = fave. Enter only one of the possible values for c. c=arrow_forward
- please do Q3arrow_forwardUse the properties of logarithms, given that In(2) = 0.6931 and In(3) = 1.0986, to approximate the logarithm. Use a calculator to confirm your approximations. (Round your answers to four decimal places.) (a) In(0.75) (b) In(24) (c) In(18) 1 (d) In ≈ 2 72arrow_forwardFind the indefinite integral. (Remember the constant of integration.) √tan(8x) tan(8x) sec²(8x) dxarrow_forward
- Find the indefinite integral by making a change of variables. (Remember the constant of integration.) √(x+4) 4)√6-x dxarrow_forwarda -> f(x) = f(x) = [x] show that whether f is continuous function or not(by using theorem) Muslim_mathsarrow_forwardUse Green's Theorem to evaluate F. dr, where F = (√+4y, 2x + √√) and C consists of the arc of the curve y = 4x - x² from (0,0) to (4,0) and the line segment from (4,0) to (0,0).arrow_forward
- Evaluate F. dr where F(x, y, z) = (2yz cos(xyz), 2xzcos(xyz), 2xy cos(xyz)) and C is the line π 1 1 segment starting at the point (8, ' and ending at the point (3, 2 3'6arrow_forwardCan you help me find the result of an integral + a 炉[メをメ +炉なarrow_forward2 a Can you help me find the result of an integral a 아 x² dxarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage

Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,

Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Quadrilaterals: Missing Angles and Sides; Author: rhornfeck;https://www.youtube.com/watch?v=knVj1O0L2TM;License: Standard YouTube License, CC-BY
STD IX | State Board | Types of Quadrilateral; Author: Robomate;https://www.youtube.com/watch?v=wh0KQ4UB0EU;License: Standard YouTube License, CC-BY