
The empirical evidence for Bernoulli’s effect.
Answer to Problem 1RQ
Solution:
Snoring while sleeping is an empirical evidence of Bernoulli’s effect.
Explanation of Solution
Introduction:
Bernoulli’s principle states that fluids moving at a faster velocity have lesser pressure (In general, fluids include both liquids and gases. Air is a gas; hence, it is classified as a fluid). This falling of pressure due to increase in the velocity of a fluid is known as Bernoulli’s effect.
Explanation:
When air moves through the narrow opening above the soft palate at the back of the upper portion of the mouth, its pressure decreases. Below this soft palate, stationary air is present that exerts higher pressure on the palate. Due to this pressure difference, the palate is pushed and the opening tends to close.
The palate retakes its position when airflow stops, resulting in the opening of the passage. This phenomenon keeps on repeating. The opening and closing of the palate forms a rhythm. The palate vibrates with a rhythmic movement due to the continuous airflow, which leads to snoring.
A diagram representing the situation is given below.
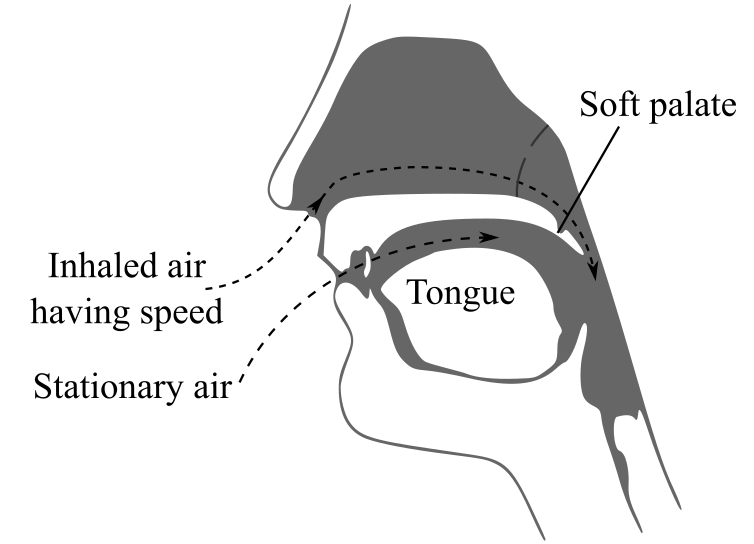
Conclusion:
Snoring while sleeping is an empirical evidence of Bernoulli’s effect.
Want to see more full solutions like this?
Chapter 14 Solutions
Pearson eText for College Physics: Explore and Apply -- Instant Access (Pearson+)
- The Dungeons & Dragons spell “Stinking Cloud” fills a 949 m^3 volume of air with a cloud of gas. The pressure of the gas is the same as the air, 101,325 Pa, and is at 29.2°C. There are 2.304x10^28 molecules of gas. What is the total internal energy of the gas?arrow_forwardThe Fiero, which is 4.70 m long, starts at 10.0˚C while in the upper atmosphere but when it goes into space the temperature would be about -270.3˚C. How much should the steel siding of the Fiero shrink due to this temperature change? The coefficient of thermal linear expansion for steel is 11.0⋅10−6⋅10^-6 C-1arrow_forwardQuestion 3 of 17 L X L L T 0.5/ In the figure above, three uniform thin rods, each of length L, form an inverted U. The vertical rods each have a mass m; the horizontal rod has a mass 3m. NOTE: Express your answer in terms of the variables given. (a) What is the x coordinate of the system's center of mass? xcom L 2 (b) What is the y coordinate of the system's center of mass? Ycom 45 L X Q Search MD bp Narrow_forward
- Sketch the harmonic on graphing paper.arrow_forwardExercise 1: (a) Using the explicit formulae derived in the lectures for the (2j+1) × (2j + 1) repre- sentation matrices Dm'm, (J/h), derive the 3 × 3 matrices corresponding to the case j = 1. (b) Verify that they satisfy the so(3) Lie algebra commutation relation: [D(Î₁/ħ), D(Î₂/h)]m'm₁ = iƊm'm² (Ĵ3/h). (c) Prove the identity 3 Dm'm,(β) = Σ (D(Ρ)D(Ρ))m'¡m; · i=1arrow_forwardSketch the harmonic.arrow_forward
- For number 11 please sketch the harmonic on graphing paper.arrow_forward# E 94 20 13. Time a) What is the frequency of the above wave? b) What is the period? c) Highlight the second cycle d) Sketch the sine wave of the second harmonic of this wave % 7 & 5 6 7 8 * ∞ Y U 9 0 0 P 150arrow_forwardShow work using graphing paperarrow_forward
- Can someone help me answer this physics 2 questions. Thank you.arrow_forwardFour capacitors are connected as shown in the figure below. (Let C = 12.0 μF.) a C 3.00 με Hh. 6.00 με 20.0 με HE (a) Find the equivalent capacitance between points a and b. 5.92 HF (b) Calculate the charge on each capacitor, taking AV ab = 16.0 V. 20.0 uF capacitor 94.7 6.00 uF capacitor 67.6 32.14 3.00 µF capacitor capacitor C ☑ με με The 3 µF and 12.0 uF capacitors are in series and that combination is in parallel with the 6 μF capacitor. What quantity is the same for capacitors in parallel? μC 32.14 ☑ You are correct that the charge on this capacitor will be the same as the charge on the 3 μF capacitor. μCarrow_forwardIn the pivot assignment, we observed waves moving on a string stretched by hanging weights. We noticed that certain frequencies produced standing waves. One such situation is shown below: 0 ст Direct Measurement ©2015 Peter Bohacek I. 20 0 cm 10 20 30 40 50 60 70 80 90 100 Which Harmonic is this? Do NOT include units! What is the wavelength of this wave in cm with only no decimal places? If the speed of this wave is 2500 cm/s, what is the frequency of this harmonic (in Hz, with NO decimal places)?arrow_forward
 College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College
College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





