
A particle P moves with constant angular speed
(a) Find the velocity vector v and show that
(b) Show that the speed
(c) Find the acceleration vector a. Show that it is proportional to r and that it points toward the origin. An acceleration with this property is called a centripetal acceleration. Show that the magnitude of the acceleration vector is
(d) Suppose that the particle has mass m. Show that the magnitude of the force F that is required to produce this motion, called a centripetal force, is
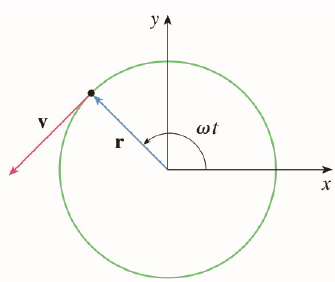
a)
To find:
The velocity vector
Answer to Problem 1P
Solution:
Explanation of Solution
1) Concept:
If
2) Given:
3) Calculation:
Consider
The velocity vector at time
Therefore, differentiating
Now
As
Since
Conclusion:
b)
To show:
The speed of particle
Answer to Problem 1P
Solution:
Explanation of Solution
1) Concept:
Speed of a particle is the magnitude of velocity vector
2) Given:
3) Calculation:
From part (a),
Therefore, as the speed of a particle is the magnitude of velocity vector
The period
At the speed
Therefore, period is
Conclusion:
The speed of particle
c)
To find:
Acceleration vector
Answer to Problem 1P
Solution:
Explanation of Solution
1) Concept:
The acceleration of a particle is
2) Calculation:
From part (a),
The acceleration of particle is
Therefore, differentiating
This concludes that acceleration is proportional to
The acceleration with this property is called centripetal acceleration.
Now, the magnitude of acceleration vector is given by
Conclusion:
The acceleration of particle is
Magnitude of acceleration vector is given by
d)
To show:
The magnitude of force required to produce this motion is
Answer to Problem 1P
Solution:
Explanation of Solution
1) Concept:
Here use Newton’s Second Law of motion.
Newton’s Second Law:
If at any time
2) Calculation:
By using Newton’s Second Law of motion,
Therefore, magnitude of force required to produce this motion is
From part (c),
Hence,
Conclusion:
The magnitude of force required to produce this motion is
Want to see more full solutions like this?
Chapter 13 Solutions
Bundle: Calculus, Loose-Leaf Version, 8th + WebAssign Printed Access Card for Stewart's Calculus, 8th Edition, Multi-Term
- New folder 10. Find the area enclosed by the loop of the curve (1- t², t-t³)arrow_forward1. Graph and find the corresponding Cartesian equation for: t X== y = t +1 2 te(-∞, ∞) 42,369 I APR 27 F5 3 MacBook Air stv A Aa T 4 DIIarrow_forwardMiddle School GP... Echo home (1) Addition and su... Google Docs Netflix Netflix New folder 9. Find the area enclosed by x = sin²t, y = cost and the y-axis.arrow_forward
- 2. Graph and find the corresponding Cartesian equation for: (4 cos 0,9 sin 0) θ ε [0, 2π) 42,369 I APR 27 3 MacBook Air 2 tv A Aaarrow_forward30 Page< 3. Find the equation of the tangent line for x = 1+12, y = 1-3 at t = 2 42,369 APR A 27 M . tv NA 1 TAGN 2 Aa 7 MacBook Air #8arrow_forwardEvaluate the following integrals as they are writtenarrow_forward
- Calculus lll May I please have the blank lines completed, and final statement defined as a result? Thank you for the support!arrow_forward3. Consider the polynomial equation 6-iz+7z² - iz³ +z = 0 for which the roots are 3i, -2i, -i, and i. (a) Verify the relations between this roots and the coefficients of the polynomial. (b) Find the annulus region in which the roots lie.arrow_forwardForce with 800 N and 400 N are acting on a machine part at 30° and 60°, respectively with the positive x axisarrow_forward
- Find the accumulated amount A, if the principal P is invested at an interest rate of r per year for t years. (Round your answer to the nearest cent.) P = $13,000, r = 6%, t = 10, compounded quarterly A = $ 31902 Need Help? Read It Watch It Viewing Saved Work Revert to Last Response SUBMIT ANSWER O/6.66 Points] DETAILS MY NOTES TANAPCALC10 5.3.003. EVIOUS ANSWERS ASK YOUR TEACHER PRACTICE ANOTHER Find the accumulated amount A, if the principal P is invested at an interest rate of r per year for t years. (Round your answer to the nearest cent.) P = $140,000, r = 8%, t = 8, compounded monthly A = $259130.20 X Need Help? Read It Watch Itarrow_forwardFind the present value of $20,000 due in 3 years at the given rate of interest. (Round your answers to the nearest cent.) (a) 2%/year compounded monthly (b) 5%/year compounded daily $ Need Help? Read It Watch It SUBMIT ANSWER [-/6.66 Points] DETAILS MY NOTES TANAPCALC10 5.3.009. ASK YOUR TEACHER PRACTICE ANC Find the accumulated amount after 3 years if $4000 is invested at 3%/year compounded continuously. (Round your answer to the nearest cent.) Need Help? Read It Watch Itarrow_forwardFind the effective rate corresponding to the given nominal rate. (Round your answers to three decimal places.) (a) 9.5%/year compounded monthly % (b) 9.5%/year compounded daily % Need Help? Read It Watch It SUBMIT ANSWER -/6.66 Points] DETAILS MY NOTES TANAPCALC10 5.3.007. ASK YOUR TEACHE Find the present value of $90,000 due in 7 years at the given rate of interest. (Round your answers to the nearest cent.) (a) 9%/year compounded semiannually (b) 9%/year compounded quarterly LAarrow_forward
 Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage LearningAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage LearningAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning


