Elementary Statistics ( 3rd International Edition ) Isbn:9781260092561
3rd Edition
ISBN: 9781259969454
Author: William Navidi Prof.; Barry Monk Professor
Publisher: McGraw-Hill Education
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Question
Chapter 13.3, Problem 21E
a.
To determine
To find:The regression equation for the data.
a.
Expert Solution
Answer to Problem 21E
The regression equation for the data is
Explanation of Solution
Given information: The data is shown below.
| 31.3 | 50 | 19 | 4.0 | 37.4 | 60 | 30 | 5.0 | 24.0 | 60 | 24 | 4.0 | 51.0 | 80 | 34 | 7.5 |
| 56.9 | 90 | 38 | 8.0 | 43.3 | 60 | 26 | 7.0 | 36.2 | 50 | 21 | 7.0 | 34.3 | 60 | 22 | 2.5 |
| 43.1 | 70 | 28 | 6.5 | 36.3 | 70 | 25 | 7.5 | 26.5 | 50 | 17 | 2.0 | 31.5 | 60 | 24 | 5.0 |
| 41.5 | 70 | 25 | 5.5 | 38.4 | 70 | 31 | 5.5 | 47.1 | 80 | 34 | 8.5 | 33.2 | 60 | 23 | 4.0 |
| 39.0 | 60 | 26 | 6.5 | 41.5 | 60 | 27 | 7.5 | 38.1 | 70 | 27 | 5.5 | 39.2 | 60 | 29 | 6.5 |
| 40.9 | 70 | 29 | 5.0 | 36.l | 60 | 23 | 6.0 | 33.5 | 60 | 24 | 2.5 | 46.7 | 70 | 27 | 7.5 |
| 35.9 | 60 | 23 | 5.5 | 38.5 | 60 | 23 | 6.0 | 43.6 | 70 | 27 | 10.0 | 30.4 | 70 | 32 | 4.0 |
| 43.5 | 70 | 28 | 5.5 | 42.4 | 60 | 24 | 9.0 | 41.0 | 80 | 32 | 6.5 | 43.2 | 60 | 25 | 5.5 |
| 47.9 | 80 | 34 | 6.5 | 46.5 | 70 | 31 | 5.5 | 50.2 | 50 | 26 | 9.0 | 30.6 | 60 | 26 | 3.5 |
| 33.8 | 70 | 26 | 4.5 | 43.1 | 80 | 32 | 6.0 | 34.4 | 50 | 22 | 4.0 | 43.3 | 70 | 28 | 7.5 |
| 41.1 | 70 | 26 | 8.0 | 50.8 | 60 | 26 | 10.0 | 47.9 | 80 | 34 | 6.5 | 32.4 | 60 | 22 | 5.5 |
| 38.7 | 70 | 26 | 8.0 | 44.2 | 70 | 28 | 4.5 | 46.2 | 50 | 21 | 10.0 | 35.5 | 60 | 22 | 4.5 |
Calculation:
The MINITAB is shown below,
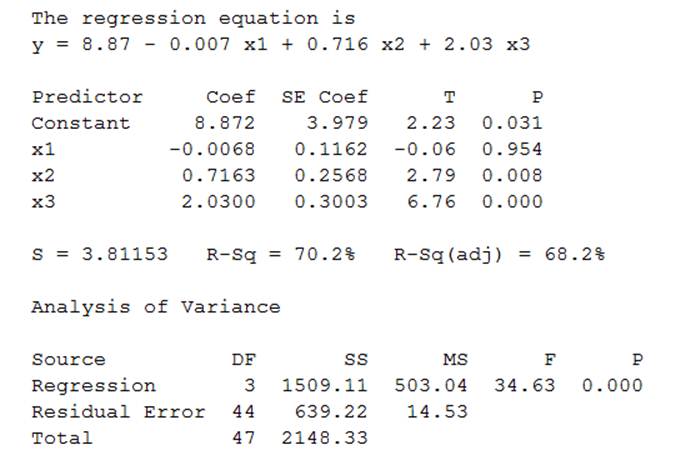
Figure-1
From Figure-1 it is clear that the regression equation is
b.
To determine
To find: The value of variable
b.
Expert Solution
Answer to Problem 21E
The value of variable
Explanation of Solution
Given information: The data is shown below.
| 31.3 | 50 | 19 | 4.0 | 37.4 | 60 | 30 | 5.0 | 24.0 | 60 | 24 | 4.0 | 51.0 | 80 | 34 | 7.5 |
| 56.9 | 90 | 38 | 8.0 | 43.3 | 60 | 26 | 7.0 | 36.2 | 50 | 21 | 7.0 | 34.3 | 60 | 22 | 2.5 |
| 43.1 | 70 | 28 | 6.5 | 36.3 | 70 | 25 | 7.5 | 26.5 | 50 | 17 | 2.0 | 31.5 | 60 | 24 | 5.0 |
| 41.5 | 70 | 25 | 5.5 | 38.4 | 70 | 31 | 5.5 | 47.1 | 80 | 34 | 8.5 | 33.2 | 60 | 23 | 4.0 |
| 39.0 | 60 | 26 | 6.5 | 41.5 | 60 | 27 | 7.5 | 38.1 | 70 | 27 | 5.5 | 39.2 | 60 | 29 | 6.5 |
| 40.9 | 70 | 29 | 5.0 | 36.l | 60 | 23 | 6.0 | 33.5 | 60 | 24 | 2.5 | 46.7 | 70 | 27 | 7.5 |
| 35.9 | 60 | 23 | 5.5 | 38.5 | 60 | 23 | 6.0 | 43.6 | 70 | 27 | 10.0 | 30.4 | 70 | 32 | 4.0 |
| 43.5 | 70 | 28 | 5.5 | 42.4 | 60 | 24 | 9.0 | 41.0 | 80 | 32 | 6.5 | 43.2 | 60 | 25 | 5.5 |
| 47.9 | 80 | 34 | 6.5 | 46.5 | 70 | 31 | 5.5 | 50.2 | 50 | 26 | 9.0 | 30.6 | 60 | 26 | 3.5 |
| 33.8 | 70 | 26 | 4.5 | 43.1 | 80 | 32 | 6.0 | 34.4 | 50 | 22 | 4.0 | 43.3 | 70 | 28 | 7.5 |
| 41.1 | 70 | 26 | 8.0 | 50.8 | 60 | 26 | 10.0 | 47.9 | 80 | 34 | 6.5 | 32.4 | 60 | 22 | 5.5 |
| 38.7 | 70 | 26 | 8.0 | 44.2 | 70 | 28 | 4.5 | 46.2 | 50 | 21 | 10.0 | 35.5 | 60 | 22 | 4.5 |
Calculation:
From Figure-1 it is clear that the regression equation is
Substitute the values in above equation.
Thus, the value of variable
c.
To determine
To find: The confidence interval.
c.
Expert Solution
Answer to Problem 21E
The confidence intervalis
Explanation of Solution
Given information: The data is shown below.
| 31.3 | 50 | 19 | 4.0 | 37.4 | 60 | 30 | 5.0 | 24.0 | 60 | 24 | 4.0 | 51.0 | 80 | 34 | 7.5 |
| 56.9 | 90 | 38 | 8.0 | 43.3 | 60 | 26 | 7.0 | 36.2 | 50 | 21 | 7.0 | 34.3 | 60 | 22 | 2.5 |
| 43.1 | 70 | 28 | 6.5 | 36.3 | 70 | 25 | 7.5 | 26.5 | 50 | 17 | 2.0 | 31.5 | 60 | 24 | 5.0 |
| 41.5 | 70 | 25 | 5.5 | 38.4 | 70 | 31 | 5.5 | 47.1 | 80 | 34 | 8.5 | 33.2 | 60 | 23 | 4.0 |
| 39.0 | 60 | 26 | 6.5 | 41.5 | 60 | 27 | 7.5 | 38.1 | 70 | 27 | 5.5 | 39.2 | 60 | 29 | 6.5 |
| 40.9 | 70 | 29 | 5.0 | 36.l | 60 | 23 | 6.0 | 33.5 | 60 | 24 | 2.5 | 46.7 | 70 | 27 | 7.5 |
| 35.9 | 60 | 23 | 5.5 | 38.5 | 60 | 23 | 6.0 | 43.6 | 70 | 27 | 10.0 | 30.4 | 70 | 32 | 4.0 |
| 43.5 | 70 | 28 | 5.5 | 42.4 | 60 | 24 | 9.0 | 41.0 | 80 | 32 | 6.5 | 43.2 | 60 | 25 | 5.5 |
| 47.9 | 80 | 34 | 6.5 | 46.5 | 70 | 31 | 5.5 | 50.2 | 50 | 26 | 9.0 | 30.6 | 60 | 26 | 3.5 |
| 33.8 | 70 | 26 | 4.5 | 43.1 | 80 | 32 | 6.0 | 34.4 | 50 | 22 | 4.0 | 43.3 | 70 | 28 | 7.5 |
| 41.1 | 70 | 26 | 8.0 | 50.8 | 60 | 26 | 10.0 | 47.9 | 80 | 34 | 6.5 | 32.4 | 60 | 22 | 5.5 |
| 38.7 | 70 | 26 | 8.0 | 44.2 | 70 | 28 | 4.5 | 46.2 | 50 | 21 | 10.0 | 35.5 | 60 | 22 | 4.5 |
Calculation:
The MINITAB output is shown below.
Figure-2
From Figure-2 it is clear that the confidence interval is
d.
To determine
To find: The prediction interval.
d.
Expert Solution
Answer to Problem 21E
The prediction intervalis
Explanation of Solution
Given information: The data is shown below.
| 31.3 | 50 | 19 | 4.0 | 37.4 | 60 | 30 | 5.0 | 24.0 | 60 | 24 | 4.0 | 51.0 | 80 | 34 | 7.5 |
| 56.9 | 90 | 38 | 8.0 | 43.3 | 60 | 26 | 7.0 | 36.2 | 50 | 21 | 7.0 | 34.3 | 60 | 22 | 2.5 |
| 43.1 | 70 | 28 | 6.5 | 36.3 | 70 | 25 | 7.5 | 26.5 | 50 | 17 | 2.0 | 31.5 | 60 | 24 | 5.0 |
| 41.5 | 70 | 25 | 5.5 | 38.4 | 70 | 31 | 5.5 | 47.1 | 80 | 34 | 8.5 | 33.2 | 60 | 23 | 4.0 |
| 39.0 | 60 | 26 | 6.5 | 41.5 | 60 | 27 | 7.5 | 38.1 | 70 | 27 | 5.5 | 39.2 | 60 | 29 | 6.5 |
| 40.9 | 70 | 29 | 5.0 | 36.l | 60 | 23 | 6.0 | 33.5 | 60 | 24 | 2.5 | 46.7 | 70 | 27 | 7.5 |
| 35.9 | 60 | 23 | 5.5 | 38.5 | 60 | 23 | 6.0 | 43.6 | 70 | 27 | 10.0 | 30.4 | 70 | 32 | 4.0 |
| 43.5 | 70 | 28 | 5.5 | 42.4 | 60 | 24 | 9.0 | 41.0 | 80 | 32 | 6.5 | 43.2 | 60 | 25 | 5.5 |
| 47.9 | 80 | 34 | 6.5 | 46.5 | 70 | 31 | 5.5 | 50.2 | 50 | 26 | 9.0 | 30.6 | 60 | 26 | 3.5 |
| 33.8 | 70 | 26 | 4.5 | 43.1 | 80 | 32 | 6.0 | 34.4 | 50 | 22 | 4.0 | 43.3 | 70 | 28 | 7.5 |
| 41.1 | 70 | 26 | 8.0 | 50.8 | 60 | 26 | 10.0 | 47.9 | 80 | 34 | 6.5 | 32.4 | 60 | 22 | 5.5 |
| 38.7 | 70 | 26 | 8.0 | 44.2 | 70 | 28 | 4.5 | 46.2 | 50 | 21 | 10.0 | 35.5 | 60 | 22 | 4.5 |
Calculation:
From Figure-12 it is clear that the
e.
To determine
To find: The percentage of variation in variable
e.
Expert Solution
Answer to Problem 21E
The percentage of variation in variable
Explanation of Solution
Given information: The data is shown below.
| 31.3 | 50 | 19 | 4.0 | 37.4 | 60 | 30 | 5.0 | 24.0 | 60 | 24 | 4.0 | 51.0 | 80 | 34 | 7.5 |
| 56.9 | 90 | 38 | 8.0 | 43.3 | 60 | 26 | 7.0 | 36.2 | 50 | 21 | 7.0 | 34.3 | 60 | 22 | 2.5 |
| 43.1 | 70 | 28 | 6.5 | 36.3 | 70 | 25 | 7.5 | 26.5 | 50 | 17 | 2.0 | 31.5 | 60 | 24 | 5.0 |
| 41.5 | 70 | 25 | 5.5 | 38.4 | 70 | 31 | 5.5 | 47.1 | 80 | 34 | 8.5 | 33.2 | 60 | 23 | 4.0 |
| 39.0 | 60 | 26 | 6.5 | 41.5 | 60 | 27 | 7.5 | 38.1 | 70 | 27 | 5.5 | 39.2 | 60 | 29 | 6.5 |
| 40.9 | 70 | 29 | 5.0 | 36.l | 60 | 23 | 6.0 | 33.5 | 60 | 24 | 2.5 | 46.7 | 70 | 27 | 7.5 |
| 35.9 | 60 | 23 | 5.5 | 38.5 | 60 | 23 | 6.0 | 43.6 | 70 | 27 | 10.0 | 30.4 | 70 | 32 | 4.0 |
| 43.5 | 70 | 28 | 5.5 | 42.4 | 60 | 24 | 9.0 | 41.0 | 80 | 32 | 6.5 | 43.2 | 60 | 25 | 5.5 |
| 47.9 | 80 | 34 | 6.5 | 46.5 | 70 | 31 | 5.5 | 50.2 | 50 | 26 | 9.0 | 30.6 | 60 | 26 | 3.5 |
| 33.8 | 70 | 26 | 4.5 | 43.1 | 80 | 32 | 6.0 | 34.4 | 50 | 22 | 4.0 | 43.3 | 70 | 28 | 7.5 |
| 41.1 | 70 | 26 | 8.0 | 50.8 | 60 | 26 | 10.0 | 47.9 | 80 | 34 | 6.5 | 32.4 | 60 | 22 | 5.5 |
| 38.7 | 70 | 26 | 8.0 | 44.2 | 70 | 28 | 4.5 | 46.2 | 50 | 21 | 10.0 | 35.5 | 60 | 22 | 4.5 |
Calculation:
From Figure-1 it is clear that the percentage of variation in variable
f.
To determine
To find:Whether the given model is useful for prediction.
f.
Expert Solution
Answer to Problem 21E
The model is useful in prediction.
Explanation of Solution
Given information: The data is shown below.
| 31.3 | 50 | 19 | 4.0 | 37.4 | 60 | 30 | 5.0 | 24.0 | 60 | 24 | 4.0 | 51.0 | 80 | 34 | 7.5 |
| 56.9 | 90 | 38 | 8.0 | 43.3 | 60 | 26 | 7.0 | 36.2 | 50 | 21 | 7.0 | 34.3 | 60 | 22 | 2.5 |
| 43.1 | 70 | 28 | 6.5 | 36.3 | 70 | 25 | 7.5 | 26.5 | 50 | 17 | 2.0 | 31.5 | 60 | 24 | 5.0 |
| 41.5 | 70 | 25 | 5.5 | 38.4 | 70 | 31 | 5.5 | 47.1 | 80 | 34 | 8.5 | 33.2 | 60 | 23 | 4.0 |
| 39.0 | 60 | 26 | 6.5 | 41.5 | 60 | 27 | 7.5 | 38.1 | 70 | 27 | 5.5 | 39.2 | 60 | 29 | 6.5 |
| 40.9 | 70 | 29 | 5.0 | 36.l | 60 | 23 | 6.0 | 33.5 | 60 | 24 | 2.5 | 46.7 | 70 | 27 | 7.5 |
| 35.9 | 60 | 23 | 5.5 | 38.5 | 60 | 23 | 6.0 | 43.6 | 70 | 27 | 10.0 | 30.4 | 70 | 32 | 4.0 |
| 43.5 | 70 | 28 | 5.5 | 42.4 | 60 | 24 | 9.0 | 41.0 | 80 | 32 | 6.5 | 43.2 | 60 | 25 | 5.5 |
| 47.9 | 80 | 34 | 6.5 | 46.5 | 70 | 31 | 5.5 | 50.2 | 50 | 26 | 9.0 | 30.6 | 60 | 26 | 3.5 |
| 33.8 | 70 | 26 | 4.5 | 43.1 | 80 | 32 | 6.0 | 34.4 | 50 | 22 | 4.0 | 43.3 | 70 | 28 | 7.5 |
| 41.1 | 70 | 26 | 8.0 | 50.8 | 60 | 26 | 10.0 | 47.9 | 80 | 34 | 6.5 | 32.4 | 60 | 22 | 5.5 |
| 38.7 | 70 | 26 | 8.0 | 44.2 | 70 | 28 | 4.5 | 46.2 | 50 | 21 | 10.0 | 35.5 | 60 | 22 | 4.5 |
Calculation:
The null hypothesis is, the model is not useful for prediction and the alternative hypothesis is, the model is useful in prediction.
From Figure-1 it is clear that the p value is less than the level of significance of
Hence, the null hypothesis is rejected.
Thus, the model is useful in prediction.
g.
To determine
To explain:The test for the hypothesis
g.
Expert Solution
Explanation of Solution
Given information: The data is shown below.
| 31.3 | 50 | 19 | 4.0 | 37.4 | 60 | 30 | 5.0 | 24.0 | 60 | 24 | 4.0 | 51.0 | 80 | 34 | 7.5 |
| 56.9 | 90 | 38 | 8.0 | 43.3 | 60 | 26 | 7.0 | 36.2 | 50 | 21 | 7.0 | 34.3 | 60 | 22 | 2.5 |
| 43.1 | 70 | 28 | 6.5 | 36.3 | 70 | 25 | 7.5 | 26.5 | 50 | 17 | 2.0 | 31.5 | 60 | 24 | 5.0 |
| 41.5 | 70 | 25 | 5.5 | 38.4 | 70 | 31 | 5.5 | 47.1 | 80 | 34 | 8.5 | 33.2 | 60 | 23 | 4.0 |
| 39.0 | 60 | 26 | 6.5 | 41.5 | 60 | 27 | 7.5 | 38.1 | 70 | 27 | 5.5 | 39.2 | 60 | 29 | 6.5 |
| 40.9 | 70 | 29 | 5.0 | 36.l | 60 | 23 | 6.0 | 33.5 | 60 | 24 | 2.5 | 46.7 | 70 | 27 | 7.5 |
| 35.9 | 60 | 23 | 5.5 | 38.5 | 60 | 23 | 6.0 | 43.6 | 70 | 27 | 10.0 | 30.4 | 70 | 32 | 4.0 |
| 43.5 | 70 | 28 | 5.5 | 42.4 | 60 | 24 | 9.0 | 41.0 | 80 | 32 | 6.5 | 43.2 | 60 | 25 | 5.5 |
| 47.9 | 80 | 34 | 6.5 | 46.5 | 70 | 31 | 5.5 | 50.2 | 50 | 26 | 9.0 | 30.6 | 60 | 26 | 3.5 |
| 33.8 | 70 | 26 | 4.5 | 43.1 | 80 | 32 | 6.0 | 34.4 | 50 | 22 | 4.0 | 43.3 | 70 | 28 | 7.5 |
| 41.1 | 70 | 26 | 8.0 | 50.8 | 60 | 26 | 10.0 | 47.9 | 80 | 34 | 6.5 | 32.4 | 60 | 22 | 5.5 |
| 38.7 | 70 | 26 | 8.0 | 44.2 | 70 | 28 | 4.5 | 46.2 | 50 | 21 | 10.0 | 35.5 | 60 | 22 | 4.5 |
The null hypothesis is, there is no relationship between
For the variable
From Figure-1 it is clear that the p value is
Hence, the null hypothesis is not rejected.
Thus, there is no linear relationship between
For the variable
From Figure-1 it is clear that the p value is
Hence, the null hypothesis is rejected.
Thus, there is alinear relationship between
For the variable
From Figure-1 it is clear that the p value is
Hence, the null hypothesis is rejected.
Thus, there is a linear relationship between
Want to see more full solutions like this?
Subscribe now to access step-by-step solutions to millions of textbook problems written by subject matter experts!
Students have asked these similar questions
Find the critical value for a left-tailed test using the F distribution with a 0.025, degrees of freedom in the numerator=12, and degrees of freedom in the
denominator = 50. A portion of the table of critical values of the F-distribution is provided.
Click the icon to view the partial table of critical values of the F-distribution.
What is the critical value?
(Round to two decimal places as needed.)
A retail store manager claims that the average daily sales of the store are $1,500.
You aim to test whether the actual average daily sales differ significantly from this claimed value.
You can provide your answer by inserting a text box and the answer must include:
Null hypothesis,
Alternative hypothesis,
Show answer (output table/summary table), and
Conclusion based on the P value.
Showing the calculation is a must. If calculation is missing,so please provide a step by step on the answers
Numerical answers in the yellow cells
Show all work
Chapter 13 Solutions
Elementary Statistics ( 3rd International Edition ) Isbn:9781260092561
Ch. 13.1 - Prob. 7ECh. 13.1 - Prob. 8ECh. 13.1 - In Exercises 9 and 10, determine whether the...Ch. 13.1 - Prob. 10ECh. 13.1 - Prob. 11ECh. 13.1 - Prob. 12ECh. 13.1 - Prob. 13ECh. 13.1 - Prob. 14ECh. 13.1 - Prob. 15ECh. 13.1 - Prob. 16E
Ch. 13.1 - Prob. 17ECh. 13.1 - Prob. 18ECh. 13.1 - Prob. 19ECh. 13.1 - Prob. 20ECh. 13.1 - Prob. 21ECh. 13.1 - Prob. 22ECh. 13.1 - Prob. 23ECh. 13.1 - Prob. 24ECh. 13.1 - Prob. 25ECh. 13.1 - Prob. 26ECh. 13.1 - Prob. 27ECh. 13.1 - Prob. 28ECh. 13.1 - Prob. 26aECh. 13.1 - Calculator display: The following TI-84 Plus...Ch. 13.1 - Prob. 28aECh. 13.1 - Prob. 29ECh. 13.1 - Prob. 30ECh. 13.1 - Confidence interval for the conditional mean: In...Ch. 13.2 - Prob. 3ECh. 13.2 - Prob. 4ECh. 13.2 - Prob. 5ECh. 13.2 - Prob. 6ECh. 13.2 - Prob. 7ECh. 13.2 - Prob. 8ECh. 13.2 - Prob. 9ECh. 13.2 - Prob. 10ECh. 13.2 - Prob. 11ECh. 13.2 - Prob. 12ECh. 13.2 - Prob. 13ECh. 13.2 - Prob. 14ECh. 13.2 - Prob. 15ECh. 13.2 - Prob. 16ECh. 13.2 - Prob. 17ECh. 13.2 - Dry up: Use the data in Exercise 26 in Section...Ch. 13.2 - Prob. 19ECh. 13.2 - Prob. 20ECh. 13.2 - Prob. 21ECh. 13.3 - Prob. 7ECh. 13.3 - Prob. 8ECh. 13.3 - Prob. 9ECh. 13.3 - In Exercises 9 and 10, determine whether the...Ch. 13.3 - Prob. 11ECh. 13.3 - Prob. 12ECh. 13.3 - Prob. 13ECh. 13.3 - For the following data set: Construct the multiple...Ch. 13.3 - Engine emissions: In a laboratory test of a new...Ch. 13.3 - Prob. 16ECh. 13.3 - Prob. 17ECh. 13.3 - Prob. 18ECh. 13.3 - Prob. 19ECh. 13.3 - Prob. 20ECh. 13.3 - Prob. 21ECh. 13.3 - Prob. 22ECh. 13.3 - Prob. 23ECh. 13 - A confidence interval for 1 is to be constructed...Ch. 13 - A confidence interval for a mean response and a...Ch. 13 - Prob. 3CQCh. 13 - Prob. 4CQCh. 13 - Prob. 5CQCh. 13 - Prob. 6CQCh. 13 - Construct a 95% confidence interval for 1.Ch. 13 - Prob. 8CQCh. 13 - Prob. 9CQCh. 13 - Prob. 10CQCh. 13 - Prob. 11CQCh. 13 - Prob. 12CQCh. 13 - Prob. 13CQCh. 13 - Prob. 14CQCh. 13 - Prob. 15CQCh. 13 - Prob. 1RECh. 13 - Prob. 2RECh. 13 - Prob. 3RECh. 13 - Prob. 4RECh. 13 - Prob. 5RECh. 13 - Prob. 6RECh. 13 - Prob. 7RECh. 13 - Prob. 8RECh. 13 - Prob. 9RECh. 13 - Prob. 10RECh. 13 - Air pollution: Following are measurements of...Ch. 13 - Icy lakes: Following are data on maximum ice...Ch. 13 - Prob. 13RECh. 13 - Prob. 14RECh. 13 - Prob. 15RECh. 13 - Prob. 1WAICh. 13 - Prob. 2WAICh. 13 - Prob. 1CSCh. 13 - Prob. 2CSCh. 13 - Prob. 3CSCh. 13 - Prob. 4CSCh. 13 - Prob. 5CSCh. 13 - Prob. 6CSCh. 13 - Prob. 7CS
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Similar questions
- Show all workarrow_forwardplease find the answers for the yellows boxes using the information and the picture belowarrow_forwardA marketing agency wants to determine whether different advertising platforms generate significantly different levels of customer engagement. The agency measures the average number of daily clicks on ads for three platforms: Social Media, Search Engines, and Email Campaigns. The agency collects data on daily clicks for each platform over a 10-day period and wants to test whether there is a statistically significant difference in the mean number of daily clicks among these platforms. Conduct ANOVA test. You can provide your answer by inserting a text box and the answer must include: also please provide a step by on getting the answers in excel Null hypothesis, Alternative hypothesis, Show answer (output table/summary table), and Conclusion based on the P value.arrow_forward
- A company found that the daily sales revenue of its flagship product follows a normal distribution with a mean of $4500 and a standard deviation of $450. The company defines a "high-sales day" that is, any day with sales exceeding $4800. please provide a step by step on how to get the answers Q: What percentage of days can the company expect to have "high-sales days" or sales greater than $4800? Q: What is the sales revenue threshold for the bottom 10% of days? (please note that 10% refers to the probability/area under bell curve towards the lower tail of bell curve) Provide answers in the yellow cellsarrow_forwardBusiness Discussarrow_forwardThe following data represent total ventilation measured in liters of air per minute per square meter of body area for two independent (and randomly chosen) samples. Analyze these data using the appropriate non-parametric hypothesis testarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Correlation Vs Regression: Difference Between them with definition & Comparison Chart; Author: Key Differences;https://www.youtube.com/watch?v=Ou2QGSJVd0U;License: Standard YouTube License, CC-BY
Correlation and Regression: Concepts with Illustrative examples; Author: LEARN & APPLY : Lean and Six Sigma;https://www.youtube.com/watch?v=xTpHD5WLuoA;License: Standard YouTube License, CC-BY