
Essential Calculus: Early Transcendentals
2nd Edition
ISBN: 9781133112280
Author: James Stewart
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Textbook Question
Chapter 13.3, Problem 1E
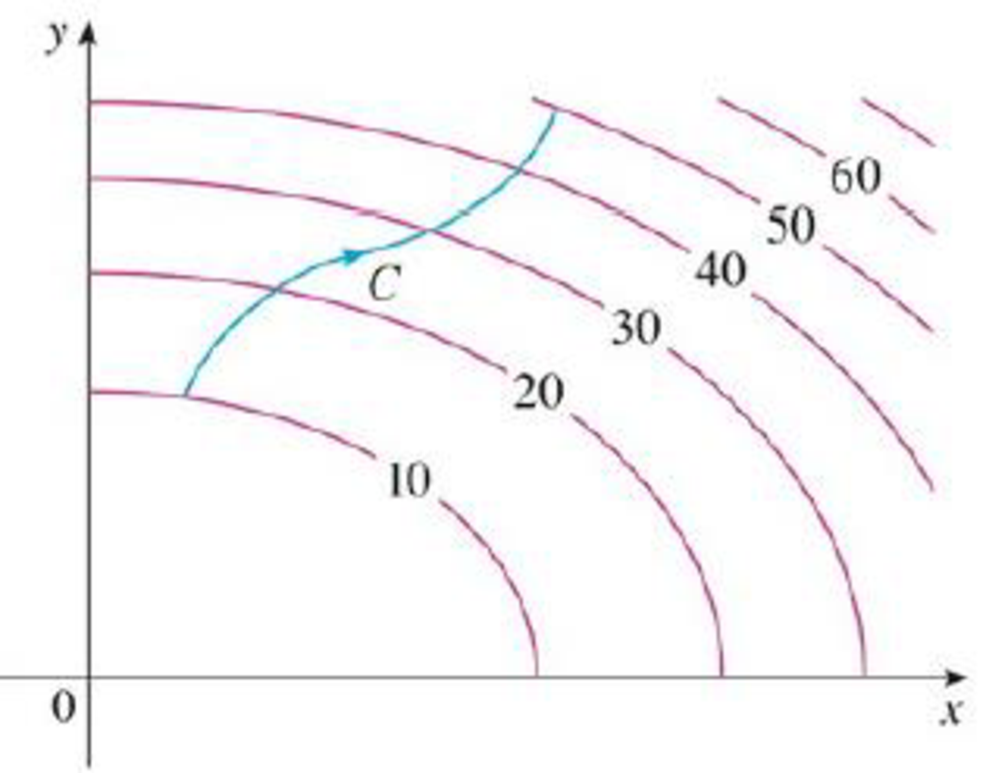
The figure shows a curve C and a contour map of a function f whose gradient is continuous. Find ∫C ∇ f · dr.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Solve this question and show steps.
u, v and w are three coplanar vectors:
⚫ w has a magnitude of 10 and points along the positive x-axis
⚫ v has a magnitude of 3 and makes an angle of 58 degrees to the positive x-
axis
⚫ u has a magnitude of 5 and makes an angle of 119 degrees to the positive x-
axis
⚫ vector v is located in between u and w
a) Draw a diagram of the three vectors placed tail-to-tail at the origin of an x-y plane.
b) If possible, find
w × (ū+v)
Support your answer mathematically or a with a written explanation.
c) If possible, find
v. (ū⋅w)
Support your answer mathematically or a with a written explanation.
d) If possible, find
u. (vxw)
Support your answer mathematically or a with a written explanation.
Note: in this question you can work with the vectors in geometric form or convert
them to algebraic vectors.
Question 3 (6 points)
u, v and w are three coplanar vectors:
⚫ w has a magnitude of 10 and points along the positive x-axis
⚫ v has a magnitude of 3 and makes an angle of 58 degrees to the positive x-
axis
⚫ u has a magnitude of 5 and makes an angle of 119 degrees to the positive x-
axis
⚫ vector v is located in between u and w
a) Draw a diagram of the three vectors placed tail-to-tail at the origin of an x-y plane.
b) If possible, find
w × (u + v)
Support your answer mathematically or a with a written explanation.
c) If possible, find
v. (ū⋅ w)
Support your answer mathematically or a with a written explanation.
d) If possible, find
u (v × w)
Support your answer mathematically or a with a written explanation.
Note: in this question you can work with the vectors in geometric form or convert
them to algebraic vectors.
Chapter 13 Solutions
Essential Calculus: Early Transcendentals
Ch. 13.1 - Sketch the vector field F by drawing a diagram...Ch. 13.1 - Sketch the vector field F by drawing a diagram...Ch. 13.1 - Prob. 3ECh. 13.1 - Prob. 4ECh. 13.1 - Prob. 5ECh. 13.1 - Prob. 6ECh. 13.1 - Prob. 7ECh. 13.1 - Sketch the vector field F by drawing a diagram...Ch. 13.1 - Prob. 9ECh. 13.1 - Sketch the vector field F by drawing a diagram...
Ch. 13.1 - Match the vector fields F with the plots labeled...Ch. 13.1 - Match the vector fields F with the plots labeled...Ch. 13.1 - Match the vector fields F with the plots labeled...Ch. 13.1 - Match the vector fields F with the plots labeled...Ch. 13.1 - Match the vector fields F on 3 with the plots...Ch. 13.1 - Match the vector fields F on 3 with the plots...Ch. 13.1 - Match the vector fields F on 3 with the plots...Ch. 13.1 - Match the vector fields F on 3 with the plots...Ch. 13.1 - Prob. 21ECh. 13.1 - Prob. 22ECh. 13.1 - Prob. 23ECh. 13.1 - Prob. 24ECh. 13.1 - Find the gradient vector field f of f and sketch...Ch. 13.1 - Find the gradient vector field f of f and sketch...Ch. 13.1 - Prob. 29ECh. 13.1 - At time t = 1, a particle is located at position...Ch. 13.1 - The flow lines (or streamlines) of a vector field...Ch. 13.1 - (a) Sketch the vector field F(x, y) = i + x j and...Ch. 13.2 - Evaluate the line integral, where C is the given...Ch. 13.2 - Evaluate the line integral, where C is the given...Ch. 13.2 - Evaluate the line integral, where C is the given...Ch. 13.2 - Evaluate the line integral, where C is the given...Ch. 13.2 - Prob. 5ECh. 13.2 - Evaluate the line integral, where C is the given...Ch. 13.2 - Prob. 7ECh. 13.2 - Evaluate the line integral, where C is the given...Ch. 13.2 - Prob. 9ECh. 13.2 - Evaluate the line integral, where C is the given...Ch. 13.2 - Prob. 11ECh. 13.2 - Prob. 12ECh. 13.2 - Prob. 13ECh. 13.2 - Prob. 14ECh. 13.2 - Evaluate the line integral, where C is the given...Ch. 13.2 - Evaluate the line integral, where C is the given...Ch. 13.2 - Let F be the vector field shown in the figure. (a)...Ch. 13.2 - The figure shows a vector field F and two curves...Ch. 13.2 - Prob. 19ECh. 13.2 - Evaluate the line integral CFdr, where C is given...Ch. 13.2 - Evaluate the line integral C F dr, where C is...Ch. 13.2 - Evaluate the line integral C F dr, where C is...Ch. 13.2 - Prob. 23ECh. 13.2 - Use a calculator or CAS to evaluate the line...Ch. 13.2 - (a) Find the work done by the force field F(x, y)...Ch. 13.2 - A thin wire is bent into the shape of a semicircle...Ch. 13.2 - A thin wire has the shape of the first-quadrant...Ch. 13.2 - Prob. 33ECh. 13.2 - Prob. 34ECh. 13.2 - Prob. 35ECh. 13.2 - Prob. 36ECh. 13.2 - Prob. 37ECh. 13.2 - Prob. 38ECh. 13.2 - Find the work done by the force field F(x, y, z) =...Ch. 13.2 - Prob. 40ECh. 13.2 - Prob. 41ECh. 13.2 - Prob. 42ECh. 13.2 - Prob. 43ECh. 13.2 - Prob. 44ECh. 13.2 - (a) Show that a constant force field does zero...Ch. 13.2 - Prob. 45ECh. 13.2 - Prob. 46ECh. 13.2 - Experiments show that a steady current I in a long...Ch. 13.3 - The figure shows a curve C and a contour map of a...Ch. 13.3 - A table of values of a function f with continuous...Ch. 13.3 - Determine whether or not F is a conservative...Ch. 13.3 - Prob. 4ECh. 13.3 - Prob. 5ECh. 13.3 - Prob. 6ECh. 13.3 - Determine whether or not F is a conservative...Ch. 13.3 - Determine whether or not F is a conservative...Ch. 13.3 - Determine whether or not F is a conservative...Ch. 13.3 - Determine whether or not F is a conservative...Ch. 13.3 - (a) Find a function f such that F = f and (b) use...Ch. 13.3 - (a) Find a function f such that F = f and (b) use...Ch. 13.3 - (a) Find a function f such that F = f and (b) use...Ch. 13.3 - Prob. 14ECh. 13.3 - Prob. 15ECh. 13.3 - (a) Find a function f such that F = f and (b) use...Ch. 13.3 - Show that the line integral is independent of path...Ch. 13.3 - Show that the line integral is independent of path...Ch. 13.3 - Find the work done by the force field F in moving...Ch. 13.3 - Find the work done by the force field F in moving...Ch. 13.3 - Is the vector field shown in the figure...Ch. 13.3 - Is the vector field shown in the figure...Ch. 13.3 - Let F = f, where f(x, y) = sin(x 2y). Find...Ch. 13.3 - Show that if the vector field F = P i + Q j + R k...Ch. 13.3 - Use Exercise 25 to show that the line integral...Ch. 13.3 - Determine whether or not the given set is (a)...Ch. 13.3 - Prob. 28ECh. 13.3 - Prob. 29ECh. 13.3 - Determine whether or not the given set is (a)...Ch. 13.3 - Let F(x, y) = yi+xjx2+y2 (a) Show that P/y=Q/x....Ch. 13.3 - (a) Suppose that F is an inverse square force...Ch. 13.4 - Evaluate the line integral by two methods: (a)...Ch. 13.4 - Evaluate the line integral by two methods: (a)...Ch. 13.4 - Evaluate the line integral by two methods: (a)...Ch. 13.4 - Evaluate the line integral by two methods: (a)...Ch. 13.4 - Use Greens Theorem to evaluate the line integral...Ch. 13.4 - Use Greens Theorem to evaluate the line integral...Ch. 13.4 - Use Greens Theorem to evaluate the line integral...Ch. 13.4 - Use Greens Theorem to evaluate the line integral...Ch. 13.4 - Use Greens Theorem to evaluate the line integral...Ch. 13.4 - Use Greens Theorem to evaluate the line integral...Ch. 13.4 - Use Greens Theorem to evaluate C F dr. (Check the...Ch. 13.4 - Use Greens Theorem to evaluate C F dr. (Check the...Ch. 13.4 - Use Greens Theorem to evaluate C F dr. (Check the...Ch. 13.4 - Use Greens Theorem to evaluate C F dr. (Check the...Ch. 13.4 - Prob. 17ECh. 13.4 - A particle starts at the point (2, 0), moves along...Ch. 13.4 - Use one of the formulas in (5) to find the area...Ch. 13.4 - If a circle C with radius 1 rolls along the...Ch. 13.4 - (a) If C is the line segment connecting the point...Ch. 13.4 - Let D be a region bounded by a simple closed path...Ch. 13.4 - Use Exercise 22 to find the centroid of a...Ch. 13.4 - Use Exercise 22 to find the centroid of the...Ch. 13.4 - A plane lamina with constant density (x, y) = ...Ch. 13.4 - Prob. 26ECh. 13.4 - Use the method of Example 5 to calculate C F dr,...Ch. 13.4 - Calculate C F dr, where F(x, y) = x2 + y, 3x y2...Ch. 13.4 - If F is the vector field of Example 5, show that C...Ch. 13.4 - Complete the proof of the special case of Greens...Ch. 13.4 - Use Greens Theorem to prove the change of...Ch. 13.5 - Find (a) the curl and (b) the divergence of the...Ch. 13.5 - Find (a) the curl and (b) the divergence of the...Ch. 13.5 - Find (a) the curl and (b) the divergence of the...Ch. 13.5 - Find (a) the curl and (b) the divergence of the...Ch. 13.5 - Find (a) the curl and (b) the divergence of the...Ch. 13.5 - Find (a) the curl and (b) the divergence of the...Ch. 13.5 - Find (a) the curl and (b) the divergence of the...Ch. 13.5 - The vector field F is shown in the xy-plane and...Ch. 13.5 - The vector field F is shown in the xy-plane and...Ch. 13.5 - Let f be a scalar field and F a vector field....Ch. 13.5 - Determine whether or not the vector field is...Ch. 13.5 - Determine whether or not the vector field is...Ch. 13.5 - Determine whether or not the vector field is...Ch. 13.5 - Determine whether or not the vector field is...Ch. 13.5 - Determine whether or not the vector field is...Ch. 13.5 - Determine whether or not the vector field is...Ch. 13.5 - Is there a vector field G on 3 such that curl G =...Ch. 13.5 - Prob. 18ECh. 13.5 - Prob. 19ECh. 13.5 - Prob. 20ECh. 13.5 - Prove the identity, assuming that the appropriate...Ch. 13.5 - Prove the identity, assuming that the appropriate...Ch. 13.5 - Prob. 23ECh. 13.5 - Prob. 24ECh. 13.5 - Prob. 25ECh. 13.5 - Prob. 26ECh. 13.5 - Prob. 27ECh. 13.5 - Prob. 28ECh. 13.5 - Prob. 29ECh. 13.5 - Let r = x i + y j + z k and r = |r|. 32. If F =...Ch. 13.5 - Prob. 31ECh. 13.5 - Prob. 32ECh. 13.5 - Prob. 33ECh. 13.5 - Prob. 34ECh. 13.5 - Prob. 35ECh. 13.5 - Maxwells equations relating the electric field E...Ch. 13.6 - Identify the surface with the given vector...Ch. 13.6 - Identify the surface with the given vector...Ch. 13.6 - Prob. 3ECh. 13.6 - Prob. 4ECh. 13.6 - Match the equations with the graphs labeled IIV...Ch. 13.6 - Match the equations with the graphs labeled IIV...Ch. 13.6 - Prob. 13ECh. 13.6 - Match the equations with the graphs labeled IIV...Ch. 13.6 - Find a parametric representation for the surface....Ch. 13.6 - Prob. 16ECh. 13.6 - Find a parametric representation for the surface....Ch. 13.6 - Find a parametric representation for the surface....Ch. 13.6 - Find a parametric representation for the surface....Ch. 13.6 - Find a parametric representation for the surface....Ch. 13.6 - Find a parametric representation for the surface....Ch. 13.6 - Find a parametric representation for the surface....Ch. 13.6 - Find parametric equations for the surface obtained...Ch. 13.6 - Find parametric equations for the surface obtained...Ch. 13.6 - The surface with parametric equations...Ch. 13.6 - Find an equation of the tangent plane to the given...Ch. 13.6 - Prob. 30ECh. 13.6 - Prob. 31ECh. 13.6 - Prob. 32ECh. 13.6 - Find the area of the surface. 39. The part of the...Ch. 13.6 - Prob. 34ECh. 13.6 - Find the area of the surface. 41. The part of the...Ch. 13.6 - Find the area of the surface. 42. The part of the...Ch. 13.6 - Prob. 37ECh. 13.6 - Prob. 38ECh. 13.6 - Prob. 39ECh. 13.6 - Prob. 41ECh. 13.6 - Find the area of the surface. 40.The part of the...Ch. 13.6 - Find the area of the surface. 48.The helicoid (or...Ch. 13.6 - Find the area of the surface. 43.The surface with...Ch. 13.6 - Find the area of the surface. 50.The part of the...Ch. 13.6 - If the equation of a surfaceSis z =f(x,y),...Ch. 13.6 - Find the area of the surface correct to four...Ch. 13.6 - Find the area of the surface correct to four...Ch. 13.6 - Find, to four decimal places, the area of the part...Ch. 13.6 - Find the area of the surface with vector equation...Ch. 13.6 - (a) Show that the parametric equations x...Ch. 13.6 - (a) Show that the parametric equationsx = acosh u...Ch. 13.6 - Find the area of the part of the spherex2+y2+ z2=...Ch. 13.6 - The figure shows the surface created when the...Ch. 13.6 - Use Definition 6 and the parametric equations for...Ch. 13.6 - Use Formula 10 to find the area of the surface...Ch. 13.6 - Use Formula 10 to find the area of the surface...Ch. 13.7 - Let S be the boundary surface of the box enclosed...Ch. 13.7 - A surface S consists of the cylinderx2+ y2=1, 1 z...Ch. 13.7 - Prob. 3ECh. 13.7 - Suppose that f(x,y,z)=g(x2+y2+z2), where g is a...Ch. 13.7 - Evaluate the surface integral. 5. s (x + y + z)...Ch. 13.7 - Evaluate the surface integral. 6. s xyz dS, Sis...Ch. 13.7 - Evaluate the surface integral. 7. s y dS,Sis the...Ch. 13.7 - Evaluate the surface integral. 8.s (x2+ y2)dS, Sis...Ch. 13.7 - Evaluate the surface integral. 9. s x2yz dS, Sis...Ch. 13.7 - Evaluate the surface integral. 10. s xz dS, S is...Ch. 13.7 - Evaluate the surface integral. 11. s x dS, S is...Ch. 13.7 - Evaluate the surface integral. 12. s y dS, S is...Ch. 13.7 - Evaluate the surface integral. Sx2z2dS, S is the...Ch. 13.7 - Evaluate the surface integral. SzdS, S is the...Ch. 13.7 - Evaluate the surface integral. 15. SydS, S is the...Ch. 13.7 - Evaluate the surface integral. 16. Sy2dS, S is the...Ch. 13.7 - Evaluate the surface integral. 17. s (x2z +...Ch. 13.7 - Evaluate the surface integral. 19. S(z+x2y)dS, S...Ch. 13.7 - Evaluate the surface integral. 19. s xz dS, S is...Ch. 13.7 - Evaluate the surface integral. 20. s (x2 + y2 +...Ch. 13.7 - Evaluate the surface integral s F dS for the...Ch. 13.7 - Evaluate the surface integral s F dS for the...Ch. 13.7 - Evaluate the surface integral s F dS for the...Ch. 13.7 - Evaluate the surface integral s F dS for the...Ch. 13.7 - Evaluate the surface integral SFdS for the given...Ch. 13.7 - Evaluate the surface integral SFdS for the given...Ch. 13.7 - Evaluate the surface integral sFdS for the given...Ch. 13.7 - Evaluate the surface integral SFdS for the given...Ch. 13.7 - Evaluate the surface integral sFdS for the given...Ch. 13.7 - Evaluate the surface integral SFdS for the given...Ch. 13.7 - Evaluate the surface integral SFdS for the given...Ch. 13.7 - Evaluate the surface integral SFdS for the given...Ch. 13.7 - Find the value of Sx2y2z2dS correct to four...Ch. 13.7 - Find a formula for s F dS similar to Formula 10...Ch. 13.7 - Find a formula for s F dS similar to Formula 10...Ch. 13.7 - Find the center of mass of the hemisphere x2 + y2...Ch. 13.7 - Find the mass of a thin funnel in the shape of a...Ch. 13.7 - (a) Give an integral expression for the moment of...Ch. 13.7 - Let S be the part of the sphere x2 + y2 + z2 = 25...Ch. 13.7 - Prob. 41ECh. 13.7 - Prob. 42ECh. 13.7 - Use Gausss Law to find the charge contained in the...Ch. 13.7 - Use Gausss Law to find the charge enclosed by the...Ch. 13.7 - The temperature at the point (x, y, z) in a...Ch. 13.7 - Prob. 46ECh. 13.7 - Let F be an inverse square field, that is, |F(r) =...Ch. 13.8 - Use Stokes Theorem to evaluate ScurlFdS. 1....Ch. 13.8 - Use Stokes Theorem to evaluate ScurlFdS. 2....Ch. 13.8 - Use Stokes Theorem to evaluate s curl F dS. 4....Ch. 13.8 - F(x, y, z) = xyz i + xy j + x2yz k. S consists of...Ch. 13.8 - Use Stokes Theorem to evaluate c F dr. In each...Ch. 13.8 - Use Stokes Theorem to evaluate c F dr. In each...Ch. 13.8 - Use Stokes Theorem to evaluate CFdr. In each case...Ch. 13.8 - Use Stokes Theorem to evaluate CFdr. In each case...Ch. 13.8 - (a) Use Stokes Theorem to evaluate c F dr, where...Ch. 13.8 - (a) Use Stokes Theorem to evaluate c F dr, where...Ch. 13.8 - Prob. 11ECh. 13.8 - Verify that Stokes Theorem is true for the given...Ch. 13.8 - Verify that Stokes Theorem is true for the given...Ch. 13.8 - Let C be a simple closed smooth curve that lies in...Ch. 13.8 - A particle moves along line segments from the...Ch. 13.8 - Evaluate c (y + sin x) dx + (z2 + cos y) dy + x3...Ch. 13.8 - Prob. 17ECh. 13.8 - Prob. 18ECh. 13.9 - Verify that the Divergence Theorem is true for the...Ch. 13.9 - Verify that the Divergence Theorem is true for the...Ch. 13.9 - Verify that the Divergence Theorem is true for the...Ch. 13.9 - Prob. 4ECh. 13.9 - Prob. 5ECh. 13.9 - Prob. 6ECh. 13.9 - Use the Divergence Theorem to calculate the...Ch. 13.9 - Use the Divergence Theorem to calculate the...Ch. 13.9 - Use the Divergence Theorem to calculate the...Ch. 13.9 - Prob. 10ECh. 13.9 - Use the Divergence Theorem to calculate the...Ch. 13.9 - Use the Divergence Theorem to calculate the...Ch. 13.9 - Prob. 13ECh. 13.9 - Prob. 14ECh. 13.9 - Use the Divergence Theorem to evaluate s F dS,...Ch. 13.9 - Prob. 18ECh. 13.9 - Prob. 19ECh. 13.9 - Prob. 20ECh. 13.9 - Prob. 21ECh. 13.9 - Prob. 22ECh. 13.9 - Prob. 23ECh. 13.9 - Prob. 24ECh. 13.9 - Prob. 25ECh. 13.9 - Prob. 26ECh. 13.9 - Prob. 27ECh. 13.9 - Prob. 28ECh. 13.9 - Prob. 29ECh. 13.9 - Prob. 30ECh. 13 - Prob. 1RCCCh. 13 - Prob. 2RCCCh. 13 - Prob. 3RCCCh. 13 - (a) Define the line integral of a vector field F...Ch. 13 - Prob. 5RCCCh. 13 - Prob. 6RCCCh. 13 - Prob. 7RCCCh. 13 - Prob. 8RCCCh. 13 - Prob. 9RCCCh. 13 - Prob. 10RCCCh. 13 - Prob. 11RCCCh. 13 - Prob. 12RCCCh. 13 - Prob. 13RCCCh. 13 - Prob. 14RCCCh. 13 - State the Divergence Theorem.Ch. 13 - In what ways are the Fundamental Theorem for Line...Ch. 13 - Prob. 1RQCh. 13 - Prob. 2RQCh. 13 - Prob. 3RQCh. 13 - Prob. 4RQCh. 13 - Prob. 5RQCh. 13 - Prob. 6RQCh. 13 - Prob. 7RQCh. 13 - Prob. 8RQCh. 13 - Prob. 9RQCh. 13 - Prob. 10RQCh. 13 - Prob. 11RQCh. 13 - Prob. 12RQCh. 13 - A vector field F, a curve C, and a point P are...Ch. 13 - Prob. 2RECh. 13 - Prob. 3RECh. 13 - Prob. 4RECh. 13 - Prob. 5RECh. 13 - Prob. 6RECh. 13 - Prob. 7RECh. 13 - Prob. 8RECh. 13 - Prob. 9RECh. 13 - Find the work done by the force field F(x, y, z) =...Ch. 13 - Prob. 11RECh. 13 - Show that F is a conservative vector field. Then...Ch. 13 - Prob. 13RECh. 13 - Show that F is a conservative and use this fact to...Ch. 13 - Verify that Greens Theorem is true for the line...Ch. 13 - Prob. 16RECh. 13 - Prob. 17RECh. 13 - Prob. 18RECh. 13 - Prob. 19RECh. 13 - Prob. 20RECh. 13 - Prob. 21RECh. 13 - If f and g are twice differentiable functions,...Ch. 13 - If f is a harmonic function, that is, 2f = 0, show...Ch. 13 - Prob. 24RECh. 13 - Find the area of the part of the surface z = x2 +...Ch. 13 - (a) Find an equation of the tangent plane at the...Ch. 13 - Prob. 27RECh. 13 - Prob. 28RECh. 13 - Prob. 29RECh. 13 - Prob. 30RECh. 13 - Prob. 31RECh. 13 - Prob. 32RECh. 13 - Prob. 33RECh. 13 - Prob. 34RECh. 13 - Verify that the Divergence Theorem is true for the...Ch. 13 - Compute the outward flux of F(x, y, z) =...Ch. 13 - Let F(x, y) = (2x3+2xy22y)i+(2y3+2x2y+2x)jx2+y2...Ch. 13 - Prob. 38RECh. 13 - If the components of F have continuous second...Ch. 13 - Prob. 39RE
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- K Find all values x = a where the function is discontinuous. For each value of x, give the limit of the function as x approaches a. Be sure to note when the limit doesn't exist. x-7 p(x) = X-7 Select the correct choice below and, if necessary, fill in the answer box(es) within your choice. (Use a comma to separate answers as needed.) OA. f is discontinuous at the single value x = OB. f is discontinuous at the single value x= OC. f is discontinuous at the two values x = OD. f is discontinuous at the two values x = The limit is The limit does not exist and is not co or - ∞. The limit for the smaller value is The limit for the larger value is The limit for the smaller value is The limit for the larger value does not exist and is not c∞ or -arrow_forwardK x3 +216 complete the table and use the results to find lim k(x). If k(x) = X+6 X-6 X -6.1 -6.01 - 6.001 - 5.999 - 5.99 -5.9 k(x) Complete the table. X -6.1 -6.01 - 6.001 - 5.999 - 5.99 - 5.9 k(x) (Round to three decimal places as needed.) Find the limit. Select the correct choice below and, if necessary, fill in the answer box within your choice.arrow_forwardSketch the slope field that represents the differential equation. × Clear Undo Redo y ४|० || 33 dy dxarrow_forward
- Sketch the slope field that represents the differential equation. × Clear Undo Redo dy 33 dx = -y "arrow_forwardMath Test 3 3 x³+y³ = Ꭹ = 9 2 2 x²+y² = 5 x+y=?arrow_forwardFor each of the following series, determine whether the absolute convergence series test determines absolute convergence or fails. For the ¿th series, if the test is inconclusive then let Mi = 4, while if the test determines absolute convergence let Mi 1 : 2: ∞ Σ(−1)"+¹ sin(2n); n=1 Σ n=1 Σ ((−1)”. COS n² 3+2n4 3: (+ 4: 5 : n=1 ∞ n 2+5n3 ПП n² 2 5+2n3 пп n² Σ(+)+ n=1 ∞ n=1 COS 4 2 3+8n3 П ηπ n- (−1)+1 sin (+727) 5 + 2m³ 4 = 8. Then the value of cos(M₁) + cos(2M2) + cos(3M3) + sin(2M) + sin(M5) is -0.027 -0.621 -1.794 -1.132 -1.498 -4.355 -2.000 2.716arrow_forward
- i need help with this question i tried by myself and so i am uploadding the question to be quided with step by step solution and please do not use chat gpt i am trying to learn thank you.arrow_forwardi need help with this question i tried by myself and so i am uploadding the question to be quided with step by step solution and please do not use chat gpt i am trying to learn thank you.arrow_forward1. 3 2 fx=14x²-15x²-9x- 2arrow_forward
- No it is not a graded assignment, its a review question but i only have the final answer not the working or explanationarrow_forwardClass, the class silues, and the class notes, whether the series does alternate and the absolute values of the terms decrease), and if the test does apply, determine whether the series converges or diverges. For the ith series, if the test does not apply the let Mi = 2, while if the test determines divergence then M¿ = 4, and if it determines convergence then M¿ = 8. 1: 2: 3 : 4: 5 : ∞ n=1 ∞ (−1)n+1. Σ(-1) +1 n=1 ∞ п 3m² +2 Σ(-1)+1 sin(2n). n=1 ∞ 2n² + 2n +3 4n2 +6 1 e-n + n² 3n23n+1 9n² +3 In(n + 1) 2n+1 Σ(-1) +1 n=1 ∞ Σ(-1)". n=1 Then the value of cos(M₁) + cos(2M2) + cos(3M3) + sin(2M4) + sin(M5) is 1.715 0.902 0.930 -1.647 -0.057 ● 2.013 1.141 4.274arrow_forward3. FCX14) = x²+3xx-y3 +.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage LearningAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage LearningAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage

Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Basic Differentiation Rules For Derivatives; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=IvLpN1G1Ncg;License: Standard YouTube License, CC-BY