
(a)
Interpretation:
The rate constant for the growth in the number of transistor on an integrated circuit has to be determined using the given plot ln N versus year.
Concept introduction:
Rate of the reaction is the change in the concentration of reactant or a product with time.
The rate law expresses the relationship of the
Rate equation for the general reaction
Order of a reaction: The sum of exponents of the concentrations in the rate law for the reaction is said to be order of a reaction.
For first order reaction,
Moore’s law states that the number of transistors per square inch on integrated circuits had doubled every year since their invention (1958).
(a)
Explanation of Solution
Given plot of ln N versus t (year) is shown below,
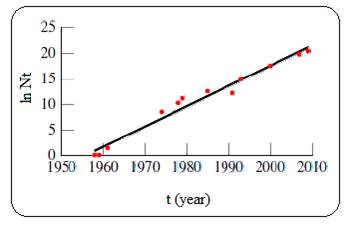
Figure 1
The plot of ln N versus t is linear for a process which follows first order kinetics. And so the given process follows first order kinetics.
The rate can be described using the equation,
Where N is the number of transistor on an integrated circuit, which is roughly doubles every 1.5 year according to the Moore’s law.
For first order reaction,
For this case, the equation can be rearranged as follows,
Comparing this equation to the straight line equation (
(b)
Interpretation:
The time required for
Concept introduction:
The rate law expresses the relationship of the rate of a reaction to the rate constant.
Rate equation for the general reaction
Order of a reaction: The sum of exponents of the concentrations in the rate law for the reaction is said to be order of a reaction.
For first order reaction,
Moore’s law states that the number of transistors per square inch on integrated circuits had doubled every year since their invention (1958).
(b)
Explanation of Solution
Given plot of ln N versus t (year) is shown below,

Figure 1
The time required for
For first order reaction,
This value is very close to the value mentioned in Moore’s law.
(c)
Interpretation:
The number of transistors on an integrated circuit
Concept introduction:
The rate law expresses the relationship of the rate of a reaction to the rate constant.
Rate equation for the general reaction
Order of a reaction: The sum of exponents of the concentrations in the rate law for the reaction is said to be order of a reaction.
For first order reaction,
Moore’s law states that the number of transistors per square inch on integrated circuits had doubled every year since their invention (1958).
(c)
Explanation of Solution
Given plot of ln N versus t (year) is shown below,

Figure 1
The time required for
For first order reaction,
For this case, the equation can be rearranged as follows,
Assume the year 1960 as
The year 2100 would corresponds to
Substituting known values in the above mentioned equation,
Thus, there will be
Want to see more full solutions like this?
Chapter 13 Solutions
CHEMISTRY-ALEKS 360 ACCESS
- One liter of chlorine gas at 1 atm and 298 K reacts completely with 1.00 L of nitrogen gas and 2.00 L of oxygen gas at the same temperature and pressure. A single gaseous product is formed, which fills a 2.00 L flask at 1.00 atm and 298 K. Use this information to determine the following characteristics of the product:(a) its empirical formula;(b) its molecular formula;(c) the most favorable Lewis formula based on formal charge arguments (the central atom is N);(d) the shape of the molecule.arrow_forwardHow does the square root mean square velocity of gas molecules vary with temperature? Illustrate this relationship by plotting the square root mean square velocity of N2 molecules as a function of temperature from T=100 K to T=300 K.arrow_forwardDraw product B, indicating what type of reaction occurs. F3C CF3 NH2 Me O .N. + B OMearrow_forward
- Benzimidazole E. State its formula. sState the differences in the formula with other benzimidazoles.arrow_forwardDraw product A, indicating what type of reaction occurs. F3C CN CF3 K2CO3, DMSO, H₂O2 Aarrow_forward19) Which metal is most commonly used in galvanization to protect steel structures from oxidation? Lead a. b. Tin C. Nickel d. Zinc 20) The following molecule is an example of a: R₁ R2- -N-R3 a. Secondary amine b. Secondary amide c. Tertiary amine d. Tertiary amidearrow_forward
- pls helparrow_forwardpls helparrow_forward35) Complete the following equation by drawing the line the structure of the products that are formed. Please note that in some cases more than one product is possible. You must draw all possible products to recive full marks! a. ethanol + 2-propanol + H2SO4 → b. OH conc. H2SO4 CH2 H3C CH + K2Cr2O7 C. d. H3C A pressure CH3 + H2 CH Pt catalystarrow_forward
 General Chemistry - Standalone book (MindTap Cour...ChemistryISBN:9781305580343Author:Steven D. Gammon, Ebbing, Darrell Ebbing, Steven D., Darrell; Gammon, Darrell Ebbing; Steven D. Gammon, Darrell D.; Gammon, Ebbing; Steven D. Gammon; DarrellPublisher:Cengage Learning
General Chemistry - Standalone book (MindTap Cour...ChemistryISBN:9781305580343Author:Steven D. Gammon, Ebbing, Darrell Ebbing, Steven D., Darrell; Gammon, Darrell Ebbing; Steven D. Gammon, Darrell D.; Gammon, Ebbing; Steven D. Gammon; DarrellPublisher:Cengage Learning Chemistry: An Atoms First ApproachChemistryISBN:9781305079243Author:Steven S. Zumdahl, Susan A. ZumdahlPublisher:Cengage Learning
Chemistry: An Atoms First ApproachChemistryISBN:9781305079243Author:Steven S. Zumdahl, Susan A. ZumdahlPublisher:Cengage Learning Living By Chemistry: First Edition TextbookChemistryISBN:9781559539418Author:Angelica StacyPublisher:MAC HIGHER
Living By Chemistry: First Edition TextbookChemistryISBN:9781559539418Author:Angelica StacyPublisher:MAC HIGHER Chemistry & Chemical ReactivityChemistryISBN:9781337399074Author:John C. Kotz, Paul M. Treichel, John Townsend, David TreichelPublisher:Cengage Learning
Chemistry & Chemical ReactivityChemistryISBN:9781337399074Author:John C. Kotz, Paul M. Treichel, John Townsend, David TreichelPublisher:Cengage Learning Chemistry & Chemical ReactivityChemistryISBN:9781133949640Author:John C. Kotz, Paul M. Treichel, John Townsend, David TreichelPublisher:Cengage Learning
Chemistry & Chemical ReactivityChemistryISBN:9781133949640Author:John C. Kotz, Paul M. Treichel, John Townsend, David TreichelPublisher:Cengage Learning Introductory Chemistry: A FoundationChemistryISBN:9781337399425Author:Steven S. Zumdahl, Donald J. DeCostePublisher:Cengage Learning
Introductory Chemistry: A FoundationChemistryISBN:9781337399425Author:Steven S. Zumdahl, Donald J. DeCostePublisher:Cengage Learning





