CALCULUS:EARLY TRANSCENDENTALS-PACKAGE
3rd Edition
ISBN: 9780135182543
Author: Briggs
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 12.4, Problem 37E
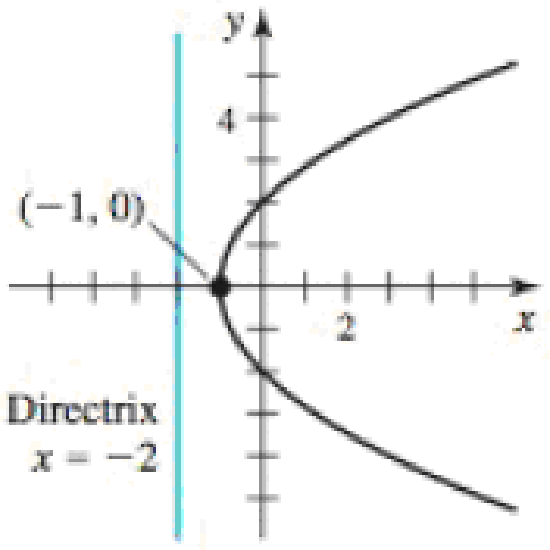
From graphs to equations Write an equation of the following parabolas.
25. 
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
(7) (12 points) Let F(x, y, z) = (y, x+z cos yz, y cos yz).
Ꮖ
(a) (4 points) Show that V x F = 0.
(b) (4 points) Find a potential f for the vector field F.
(c) (4 points) Let S be a surface in R3 for which the Stokes' Theorem is valid. Use
Stokes' Theorem to calculate the line integral
Jos
F.ds;
as denotes the boundary of S. Explain your answer.
(3) (16 points) Consider
z = uv,
u = x+y,
v=x-y.
(a) (4 points) Express z in the form z = fog where g: R² R² and f: R² →
R.
(b) (4 points) Use the chain rule to calculate Vz = (2, 2). Show all intermediate
steps otherwise no credit.
(c) (4 points) Let S be the surface parametrized by
T(x, y) = (x, y, ƒ (g(x, y))
(x, y) = R².
Give a parametric description of the tangent plane to S at the point p = T(x, y).
(d) (4 points) Calculate the second Taylor polynomial Q(x, y) (i.e. the quadratic
approximation) of F = (fog) at a point (a, b). Verify that
Q(x,y) F(a+x,b+y).
=
(6) (8 points) Change the order of integration and evaluate
(z +4ry)drdy .
So S√ ²
0
Chapter 12 Solutions
CALCULUS:EARLY TRANSCENDENTALS-PACKAGE
Ch. 12.1 - Identify the graph generated by the parametric...Ch. 12.1 - Prob. 2QCCh. 12.1 - Describe the curve generated by x = 3 + 2t, y = 12...Ch. 12.1 - Find parametric equations for the line segment...Ch. 12.1 - Use Theorem 12.1 to find the slope of the line x =...Ch. 12.1 - Use the arc length formula to find the length of...Ch. 12.1 - Explain how a pair of parametric equations...Ch. 12.1 - Prob. 2ECh. 12.1 - Prob. 3ECh. 12.1 - Give parametric equations that generate the line...
Ch. 12.1 - Find parametric equations for the complete...Ch. 12.1 - Describe the similarities between the graphs of...Ch. 12.1 - Find the slope of the parametric curve x = 2t3 +...Ch. 12.1 - Prob. 8ECh. 12.1 - Find three different pairs of parametric equations...Ch. 12.1 - Use calculus to find the arc length of the line...Ch. 12.1 - Prob. 11ECh. 12.1 - Prob. 12ECh. 12.1 - Prob. 13ECh. 12.1 - Prob. 14ECh. 12.1 - Prob. 15ECh. 12.1 - Prob. 16ECh. 12.1 - Prob. 17ECh. 12.1 - Prob. 18ECh. 12.1 - Prob. 19ECh. 12.1 - Prob. 20ECh. 12.1 - Prob. 21ECh. 12.1 - Prob. 22ECh. 12.1 - Prob. 23ECh. 12.1 - Prob. 24ECh. 12.1 - Prob. 25ECh. 12.1 - Prob. 26ECh. 12.1 - Prob. 27ECh. 12.1 - Working with parametric equations Consider the...Ch. 12.1 - Prob. 29ECh. 12.1 - Prob. 30ECh. 12.1 - Eliminating the parameter Eliminate the parameter...Ch. 12.1 - Eliminating the parameter Eliminate the parameter...Ch. 12.1 - Prob. 33ECh. 12.1 - Prob. 34ECh. 12.1 - Prob. 35ECh. 12.1 - Prob. 36ECh. 12.1 - Parametric equations of circles Find parametric...Ch. 12.1 - Parametric equations of circles Find parametric...Ch. 12.1 - Parametric equations of circles Find parametric...Ch. 12.1 - Parametric equations of circles Find parametric...Ch. 12.1 - Prob. 41ECh. 12.1 - Curves to parametric equations Find parametric...Ch. 12.1 - Curves to parametric equations Give a set of...Ch. 12.1 - Curves to parametric equations Give a set of...Ch. 12.1 - Prob. 45ECh. 12.1 - Curves to parametric equations Find parametric...Ch. 12.1 - Prob. 47ECh. 12.1 - Prob. 48ECh. 12.1 - Prob. 49ECh. 12.1 - Curves to parametric equations Find parametric...Ch. 12.1 - Curves to parametric equations Find parametric...Ch. 12.1 - Curves to parametric equations Find parametric...Ch. 12.1 - Circular motion Find parametric equations that...Ch. 12.1 - Circular motion Find parametric equations that...Ch. 12.1 - Circular motion Find parametric equations that...Ch. 12.1 - Circular motion Find parametric equations that...Ch. 12.1 - More parametric curves Use a graphing utility to...Ch. 12.1 - More parametric curves Use a graphing utility to...Ch. 12.1 - More parametric curves Use a graphing utility to...Ch. 12.1 - More parametric curves Use a graphing utility to...Ch. 12.1 - More parametric curves Use a graphing utility to...Ch. 12.1 - More parametric curves Use a graphing utility to...Ch. 12.1 - Implicit function graph Explain and carry out a...Ch. 12.1 - Air drop A plane traveling horizontally at 80 m/s...Ch. 12.1 - Air dropinverse problem A plane traveling...Ch. 12.1 - Prob. 66ECh. 12.1 - Prob. 67ECh. 12.1 - Derivatives Consider the following parametric...Ch. 12.1 - Derivatives Consider the following parametric...Ch. 12.1 - Prob. 70ECh. 12.1 - Derivatives Consider the following parametric...Ch. 12.1 - Prob. 72ECh. 12.1 - Tangent lines Find an equation of the line tangent...Ch. 12.1 - Tangent lines Find an equation of the line tangent...Ch. 12.1 - Tangent lines Find an equation of the line tangent...Ch. 12.1 - Tangent lines Find an equation of the line tangent...Ch. 12.1 - Slopes of tangent lines Find all the points at...Ch. 12.1 - Slopes of tangent lines Find all the points at...Ch. 12.1 - Slopes of tangent lines Find all the points at...Ch. 12.1 - Slopes of tangent lines Find all the points at...Ch. 12.1 - Arc length Find the arc length of the following...Ch. 12.1 - Arc length Find the arc length of the following...Ch. 12.1 - Arc length Find the arc length of the following...Ch. 12.1 - Arc length Find the arc length of the following...Ch. 12.1 - Arc length Find the arc length of the following...Ch. 12.1 - Arc length Find the arc length of the following...Ch. 12.1 - Arc length Find the arc length of the following...Ch. 12.1 - Arc length Find the arc length of the following...Ch. 12.1 - Explain why or why not Determine whether the...Ch. 12.1 - Prob. 90ECh. 12.1 - Prob. 91ECh. 12.1 - Prob. 92ECh. 12.1 - Parametric equations of ellipses Find parametric...Ch. 12.1 - Prob. 94ECh. 12.1 - Prob. 95ECh. 12.1 - Prob. 96ECh. 12.1 - Prob. 97ECh. 12.1 - Beautiful curves Consider the family of curves...Ch. 12.1 - Prob. 99ECh. 12.1 - Prob. 100ECh. 12.1 - Prob. 101ECh. 12.1 - Lissajous curves Consider the following Lissajous...Ch. 12.1 - Area under a curve Suppose the function y = h(x)...Ch. 12.1 - Area under a curve Suppose the function y = h(x)...Ch. 12.1 - Area under a curve Suppose the function y = h(x)...Ch. 12.1 - Prob. 106ECh. 12.1 - Prob. 107ECh. 12.1 - Prob. 108ECh. 12.1 - Surfaces of revolution Let C be the curve x =...Ch. 12.1 - Prob. 110ECh. 12.1 - Surfaces of revolution Let C be the curve x =...Ch. 12.1 - Prob. 112ECh. 12.1 - Prob. 113ECh. 12.1 - Prob. 114ECh. 12.2 - Which of the following coordinates represent the...Ch. 12.2 - Draw versions of Figure 12.21 with P in the...Ch. 12.2 - Give two polar coordinate descriptions of the...Ch. 12.2 - Describe the polar curves r = 12, r = 6, and r sin...Ch. 12.2 - Prob. 5QCCh. 12.2 - Prob. 6QCCh. 12.2 - Plot the points with polar coordinates (2,6) and...Ch. 12.2 - Prob. 2ECh. 12.2 - Prob. 3ECh. 12.2 - Prob. 4ECh. 12.2 - What is the polar equation of the vertical line x...Ch. 12.2 - What is the polar equation of the horizontal line...Ch. 12.2 - Prob. 7ECh. 12.2 - Prob. 8ECh. 12.2 - Graph the points with the following polar...Ch. 12.2 - Graph the points with the following polar...Ch. 12.2 - Prob. 11ECh. 12.2 - Prob. 12ECh. 12.2 - Prob. 13ECh. 12.2 - Points in polar coordinates Give two sets of polar...Ch. 12.2 - Prob. 15ECh. 12.2 - Prob. 16ECh. 12.2 - Prob. 17ECh. 12.2 - Prob. 18ECh. 12.2 - Prob. 19ECh. 12.2 - Prob. 20ECh. 12.2 - Prob. 21ECh. 12.2 - Prob. 22ECh. 12.2 - Rader Airplanes are equipped with transponders...Ch. 12.2 - Prob. 24ECh. 12.2 - Converting coordinates Express the following polar...Ch. 12.2 - Converting coordinates Express the following polar...Ch. 12.2 - Converting coordinates Express the following polar...Ch. 12.2 - Converting coordinates Express the following polar...Ch. 12.2 - Converting coordinates Express the following polar...Ch. 12.2 - Converting coordinates Express the following polar...Ch. 12.2 - Converting coordinates Express the following...Ch. 12.2 - Converting coordinates Express the following...Ch. 12.2 - Converting coordinates Express the following...Ch. 12.2 - Converting coordinates Express the following...Ch. 12.2 - Converting coordinates Express the following...Ch. 12.2 - Converting coordinates Express the following...Ch. 12.2 - Prob. 37ECh. 12.2 - Prob. 38ECh. 12.2 - Prob. 39ECh. 12.2 - Prob. 40ECh. 12.2 - Prob. 41ECh. 12.2 - Prob. 42ECh. 12.2 - Prob. 43ECh. 12.2 - Prob. 44ECh. 12.2 - Prob. 45ECh. 12.2 - Prob. 46ECh. 12.2 - Prob. 47ECh. 12.2 - Prob. 48ECh. 12.2 - Cartesian-to-polar coordinates Convert the...Ch. 12.2 - Cartesian-to-polar coordinates Convert the...Ch. 12.2 - Cartesian-to-polar coordinates Convert the...Ch. 12.2 - Cartesian-to-polar coordinates Convert the...Ch. 12.2 - Prob. 53ECh. 12.2 - Prob. 54ECh. 12.2 - Prob. 55ECh. 12.2 - Prob. 56ECh. 12.2 - Graphing polar curves Graph the following...Ch. 12.2 - Graphing polar curves Graph the following...Ch. 12.2 - Prob. 59ECh. 12.2 - Prob. 60ECh. 12.2 - Graphing polar curves Graph the following...Ch. 12.2 - Graphing polar curves Graph the following...Ch. 12.2 - Graphing polar curves Graph the following...Ch. 12.2 - Graphing polar curves Graph the following...Ch. 12.2 - Prob. 65ECh. 12.2 - Prob. 66ECh. 12.2 - Prob. 67ECh. 12.2 - Prob. 68ECh. 12.2 - Using a graphing utility Use a graphing utility to...Ch. 12.2 - Using a graphing utility Use a graphing utility to...Ch. 12.2 - Prob. 71ECh. 12.2 - Using a graphing utility Use a graphing utility to...Ch. 12.2 - Using a graphing utility Use a graphing utility to...Ch. 12.2 - Using a graphing utility Use a graphing utility to...Ch. 12.2 - Prob. 75ECh. 12.2 - Prob. 76ECh. 12.2 - Prob. 77ECh. 12.2 - Prob. 78ECh. 12.2 - Circles in general Show that the polar equation...Ch. 12.2 - Prob. 80ECh. 12.2 - Prob. 81ECh. 12.2 - Prob. 82ECh. 12.2 - Prob. 83ECh. 12.2 - Equations of circles Find equations of the circles...Ch. 12.2 - Navigating A plane is 150 miles north of a radar...Ch. 12.2 - Prob. 86ECh. 12.2 - Prob. 87ECh. 12.2 - Prob. 88ECh. 12.2 - Prob. 89ECh. 12.2 - Prob. 90ECh. 12.2 - Prob. 91ECh. 12.2 - Limiting limaon Consider the family of limaons r =...Ch. 12.2 - Prob. 93ECh. 12.2 - Prob. 94ECh. 12.2 - Prob. 95ECh. 12.2 - The lemniscate family Equations of the form r2 = a...Ch. 12.2 - The rose family Equations of the form r = a sin m...Ch. 12.2 - Prob. 98ECh. 12.2 - Prob. 99ECh. 12.2 - The rose family Equations of the form r = a sin m...Ch. 12.2 - Prob. 101ECh. 12.2 - Prob. 102ECh. 12.2 - Prob. 103ECh. 12.2 - Spirals Graph the following spirals. Indicate the...Ch. 12.2 - Enhanced butterfly curve The butterfly curve of...Ch. 12.2 - Prob. 106ECh. 12.2 - Prob. 107ECh. 12.2 - Prob. 108ECh. 12.2 - Prob. 109ECh. 12.2 - Prob. 110ECh. 12.2 - Cartesian lemniscate Find the equation in...Ch. 12.3 - Verify that if y = f() sin , then y'() =f'() sin ...Ch. 12.3 - Prob. 2QCCh. 12.3 - Prob. 3QCCh. 12.3 - Prob. 4QCCh. 12.3 - Prob. 1ECh. 12.3 - Explain why the slope of the line = /2 is...Ch. 12.3 - Explain why the slope of the line tangent to the...Ch. 12.3 - What integral must be evaluated to find the area...Ch. 12.3 - What is the slope of the line = /3?Ch. 12.3 - Prob. 6ECh. 12.3 - Find the area of the shaded region.Ch. 12.3 - Prob. 8ECh. 12.3 - Explain why the point with polar coordinates (0,...Ch. 12.3 - Prob. 10ECh. 12.3 - Slopes of tangent lines Find the slope of the line...Ch. 12.3 - Slopes of tangent lines Find the slope of the line...Ch. 12.3 - Slopes of tangent lines Find the slope of the line...Ch. 12.3 - Slopes of tangent lines Find the slope of the line...Ch. 12.3 - Slopes of tangent lines Find the slope of the line...Ch. 12.3 - Slopes of tangent lines Find the slope of the line...Ch. 12.3 - Slopes of tangent lines Find the slope of the line...Ch. 12.3 - Slopes of tangent lines Find the slope of the line...Ch. 12.3 - Slopes of tangent lines Find the slope of the line...Ch. 12.3 - Slopes of tangent lines Find the slope of the line...Ch. 12.3 - Tangent line at the origin Find the polar equation...Ch. 12.3 - Prob. 22ECh. 12.3 - Multiple tangent lines at a point a. Give the...Ch. 12.3 - Multiple tangent lines at a point a. Give the...Ch. 12.3 - Horizontal and vertical tangents Find the points...Ch. 12.3 - Horizontal and vertical tangents Find the points...Ch. 12.3 - Horizontal and vertical tangents Find the points...Ch. 12.3 - Prob. 28ECh. 12.3 - Prob. 29ECh. 12.3 - Prob. 30ECh. 12.3 - Prob. 31ECh. 12.3 - Prob. 32ECh. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Intersection points and area a. Find all the...Ch. 12.3 - Intersection points and area a. Find all the...Ch. 12.3 - Intersection points and area a. Find all the...Ch. 12.3 - Intersection points and area a. Find all the...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Areas of regions Make a sketch of the region and...Ch. 12.3 - Area of plane regions Find the areas of the...Ch. 12.3 - Area of plane regions Find the areas of the...Ch. 12.3 - Area of plane regions Find the areas of the...Ch. 12.3 - Area of plane regions Find the areas of the...Ch. 12.3 - Area of polar regions Find the area of the regions...Ch. 12.3 - Area of polar regions Find the area of the regions...Ch. 12.3 - Prob. 59ECh. 12.3 - Area of polar regions Find the area of the regions...Ch. 12.3 - Two curves, three regions Determine the...Ch. 12.3 - Prob. 62ECh. 12.3 - Arc length of polar curves Find the length of the...Ch. 12.3 - Prob. 64ECh. 12.3 - Prob. 65ECh. 12.3 - Prob. 66ECh. 12.3 - Prob. 67ECh. 12.3 - Arc length of polar curves Find the length of the...Ch. 12.3 - Arc length of polar curves Find the length of the...Ch. 12.3 - Arc length of polar curves Find the length of the...Ch. 12.3 - Prob. 71ECh. 12.3 - Prob. 72ECh. 12.3 - Prob. 73ECh. 12.3 - Prob. 74ECh. 12.3 - Prob. 75ECh. 12.3 - Prob. 76ECh. 12.3 - Prob. 77ECh. 12.3 - Prob. 78ECh. 12.3 - Prob. 79ECh. 12.3 - Prob. 80ECh. 12.3 - Regions bounded by a spiral Let Rn be the region...Ch. 12.3 - Tangents and normals Let a polar curve be...Ch. 12.3 - Prob. 83ECh. 12.3 - Prob. 84ECh. 12.3 - Grazing goat problems Consider the following...Ch. 12.3 - Grazing goat problems Consider the following...Ch. 12.3 - Prob. 87ECh. 12.4 - Verify that x2+(yp)2=y+p is equivalent to x2 =...Ch. 12.4 - Prob. 2QCCh. 12.4 - In the case that the vertices and foci are on the...Ch. 12.4 - Prob. 4QCCh. 12.4 - Prob. 5QCCh. 12.4 - Prob. 6QCCh. 12.4 - Give the property that defines all parabolas.Ch. 12.4 - Prob. 2ECh. 12.4 - Give the property that defines all hyperbolas.Ch. 12.4 - Prob. 4ECh. 12.4 - Prob. 5ECh. 12.4 - What is the equation of the standard parabola with...Ch. 12.4 - Prob. 7ECh. 12.4 - Prob. 8ECh. 12.4 - Given vertices (a, 0) and eccentricity e, what are...Ch. 12.4 - Prob. 10ECh. 12.4 - What are the equations of the asymptotes of a...Ch. 12.4 - Prob. 12ECh. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Prob. 16ECh. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Prob. 18ECh. 12.4 - Prob. 19ECh. 12.4 - Prob. 20ECh. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Prob. 23ECh. 12.4 - Prob. 24ECh. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Prob. 27ECh. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Graphing conic sections Determine whether the...Ch. 12.4 - Prob. 31ECh. 12.4 - Equations of parabolas Find an equation of the...Ch. 12.4 - Equations of parabolas Find an equation of the...Ch. 12.4 - Prob. 34ECh. 12.4 - Prob. 35ECh. 12.4 - Equations of parabolas Find an equation of the...Ch. 12.4 - From graphs to equations Write an equation of the...Ch. 12.4 - From graphs to equations Write an equation of the...Ch. 12.4 - Equations of ellipses Find an equation of the...Ch. 12.4 - Equations of ellipses Find an equation of the...Ch. 12.4 - Equations of hyperbolas Find an equation of the...Ch. 12.4 - Equations of hyperbolas Find an equation of the...Ch. 12.4 - Equations of ellipses Find an equation of the...Ch. 12.4 - Prob. 44ECh. 12.4 - Equations of hyperbolas Find an equation of the...Ch. 12.4 - Prob. 46ECh. 12.4 - Prob. 47ECh. 12.4 - Prob. 48ECh. 12.4 - From graphs to equations Write an equation of the...Ch. 12.4 - From graphs to equations Write an equation of the...Ch. 12.4 - Prob. 51ECh. 12.4 - Golden Gate Bridge Completed in 1937, San...Ch. 12.4 - Eccentricity-directrix approach Find an equation...Ch. 12.4 - Eccentricity-directrix approach Find an equation...Ch. 12.4 - Eccentricity-directrix approach Find an equation...Ch. 12.4 - Eccentricity-directrix approach Find an equation...Ch. 12.4 - Prob. 57ECh. 12.4 - Prob. 58ECh. 12.4 - Prob. 59ECh. 12.4 - Prob. 60ECh. 12.4 - Prob. 61ECh. 12.4 - Prob. 62ECh. 12.4 - Tracing hyperbolas and parabolas Graph the...Ch. 12.4 - Tracing hyperbolas and parabolas Graph the...Ch. 12.4 - Tracing hyperbolas and parabolas Graph the...Ch. 12.4 - Tracing hyperbolas and parabolas Graph the...Ch. 12.4 - Prob. 67ECh. 12.4 - Hyperbolas with a graphing utility Use a graphing...Ch. 12.4 - Tangent lines Find an equation of the tine tangent...Ch. 12.4 - Prob. 70ECh. 12.4 - Tangent lines Find an equation of the tine tangent...Ch. 12.4 - Tangent lines Find an equation of the tine tangent...Ch. 12.4 - Tangent lines for an ellipse Show that an equation...Ch. 12.4 - Prob. 74ECh. 12.4 - Prob. 75ECh. 12.4 - Prob. 76ECh. 12.4 - Another construction for a hyperbola Suppose two...Ch. 12.4 - The ellipse and the parabola Let R be the region...Ch. 12.4 - Volume of an ellipsoid Suppose that the ellipse...Ch. 12.4 - Area of a sector of a hyperbola Consider the...Ch. 12.4 - Volume of a hyperbolic cap Consider the region R...Ch. 12.4 - Prob. 82ECh. 12.4 - Prob. 83ECh. 12.4 - Prob. 84ECh. 12.4 - Prob. 85ECh. 12.4 - Prob. 86ECh. 12.4 - Prob. 87ECh. 12.4 - Prob. 88ECh. 12.4 - Shared asymptotes Suppose that two hyperbolas with...Ch. 12.4 - Focal chords A focal chord of a conic section is a...Ch. 12.4 - Focal chords A focal chord of a conic section is a...Ch. 12.4 - Focal chords A focal chord of a conic section is a...Ch. 12.4 - Prob. 93ECh. 12.4 - Prob. 94ECh. 12.4 - Confocal ellipse and hyperbola Show that an...Ch. 12.4 - Approach to asymptotes Show that the vertical...Ch. 12.4 - Prob. 97ECh. 12.4 - Prob. 98ECh. 12 - Explain why or why not Determine whether the...Ch. 12 - Prob. 2RECh. 12 - Prob. 3RECh. 12 - Eliminating the parameter Eliminate the parameter...Ch. 12 - Prob. 5RECh. 12 - Prob. 6RECh. 12 - Parametric curves and tangent lines a. Eliminate...Ch. 12 - Parametric curves and tangent lines a. Eliminate...Ch. 12 - Prob. 9RECh. 12 - Parametric curves a. Eliminate the parameter to...Ch. 12 - Parametric curves a. Eliminate the parameter to...Ch. 12 - Prob. 12RECh. 12 - Tangent lines Find an equation of the line tangent...Ch. 12 - Parametric descriptions Write parametric equations...Ch. 12 - Parametric description Write parametric equations...Ch. 12 - Parametric description Write parametric equations...Ch. 12 - Parametric description Write parametric equations...Ch. 12 - Parametric description Write parametric equations...Ch. 12 - Area bounded by parametric curves Find the area of...Ch. 12 - Area bounded by parametric curves Find the area of...Ch. 12 - Prob. 21RECh. 12 - Arc length Find the length of the following...Ch. 12 - Arc length Find the length of the following...Ch. 12 - Prob. 24RECh. 12 - Sets in polar coordinates Sketch the following...Ch. 12 - Prob. 26RECh. 12 - Prob. 27RECh. 12 - Prob. 28RECh. 12 - Prob. 29RECh. 12 - Prob. 30RECh. 12 - Polar curves Graph the following equations. 31. r...Ch. 12 - Prob. 32RECh. 12 - Prob. 33RECh. 12 - Prob. 34RECh. 12 - Polar conversion Write the equation...Ch. 12 - Polar conversion Consider the equation r = 4/(sin ...Ch. 12 - Prob. 37RECh. 12 - Prob. 38RECh. 12 - Prob. 39RECh. 12 - Slopes of tangent lines a. Find all points where...Ch. 12 - Slopes of tangent lines a. Find all points where...Ch. 12 - Prob. 42RECh. 12 - Prob. 43RECh. 12 - The region enclosed by all the leaves of the rose...Ch. 12 - Prob. 45RECh. 12 - The region inside the limaon r = 2 + cos and...Ch. 12 - Areas of regions Find the ares of the following...Ch. 12 - Prob. 48RECh. 12 - The area that is inside the cardioid r = 1 + cos ...Ch. 12 - Prob. 50RECh. 12 - Prob. 51RECh. 12 - Arc length of the polar curves Find the...Ch. 12 - Prob. 53RECh. 12 - Prob. 54RECh. 12 - Conic sections a. Determine whether the following...Ch. 12 - Prob. 56RECh. 12 - Prob. 57RECh. 12 - Tangent lines Find an equation of the line tangent...Ch. 12 - Tangent lines Find an equation of the line tangent...Ch. 12 - Prob. 60RECh. 12 - Prob. 61RECh. 12 - Prob. 62RECh. 12 - Prob. 63RECh. 12 - Prob. 64RECh. 12 - Prob. 65RECh. 12 - Prob. 66RECh. 12 - Eccentricity-directrix approach Find an equation...Ch. 12 - Prob. 68RECh. 12 - Prob. 69RECh. 12 - Prob. 70RECh. 12 - Prob. 71RECh. 12 - Prob. 72RECh. 12 - Prob. 73RECh. 12 - Prob. 74RECh. 12 - Lam curves The Lam curve described by...Ch. 12 - Prob. 76RE
Additional Math Textbook Solutions
Find more solutions based on key concepts
In hypothesis testing, the common level of significance is =0.05. Some might argue for a level of significance ...
Basic Business Statistics, Student Value Edition
Voting A random sample of likely voters showed that 49 planned to support Measure X. The margin of error is 3 p...
Introductory Statistics
The difference of −2−5 .
Pre-Algebra Student Edition
In Exercises 13–16, find the margin of error for the values of c, ?, and n.
13. c = 0.95, ? = 5.2, n = 30
Elementary Statistics: Picturing the World (7th Edition)
Answer the following regarding the English alphabet. a. Determine the ratio of vowels to consonants. b. What is...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- (10) (16 points) Let R>0. Consider the truncated sphere S given as x² + y² + (z = √15R)² = R², z ≥0. where F(x, y, z) = −yi + xj . (a) (8 points) Consider the vector field V (x, y, z) = (▼ × F)(x, y, z) Think of S as a hot-air balloon where the vector field V is the velocity vector field measuring the hot gasses escaping through the porous surface S. The flux of V across S gives the volume flow rate of the gasses through S. Calculate this flux. Hint: Parametrize the boundary OS. Then use Stokes' Theorem. (b) (8 points) Calculate the surface area of the balloon. To calculate the surface area, do the following: Translate the balloon surface S by the vector (-15)k. The translated surface, call it S+ is part of the sphere x² + y²+z² = R². Why do S and S+ have the same area? ⚫ Calculate the area of S+. What is the natural spherical parametrization of S+?arrow_forward(1) (8 points) Let c(t) = (et, et sint, et cost). Reparametrize c as a unit speed curve starting from the point (1,0,1).arrow_forward(9) (16 points) Let F(x, y, z) = (x² + y − 4)i + 3xyj + (2x2 +z²)k = - = (x²+y4,3xy, 2x2 + 2²). (a) (4 points) Calculate the divergence and curl of F. (b) (6 points) Find the flux of V x F across the surface S given by x² + y²+2² = 16, z ≥ 0. (c) (6 points) Find the flux of F across the boundary of the unit cube E = [0,1] × [0,1] x [0,1].arrow_forward
- (8) (12 points) (a) (8 points) Let C be the circle x² + y² = 4. Let F(x, y) = (2y + e²)i + (x + sin(y²))j. Evaluate the line integral JF. F.ds. Hint: First calculate V x F. (b) (4 points) Let S be the surface r² + y² + z² = 4, z ≤0. Calculate the flux integral √(V × F) F).dS. Justify your answer.arrow_forwardDetermine whether the Law of Sines or the Law of Cosines can be used to find another measure of the triangle. a = 13, b = 15, C = 68° Law of Sines Law of Cosines Then solve the triangle. (Round your answers to four decimal places.) C = 15.7449 A = 49.9288 B = 62.0712 × Need Help? Read It Watch Itarrow_forward(4) (10 points) Evaluate √(x² + y² + z²)¹⁄² exp[}(x² + y² + z²)²] dV where D is the region defined by 1< x² + y²+ z² ≤4 and √√3(x² + y²) ≤ z. Note: exp(x² + y²+ 2²)²] means el (x²+ y²+=²)²]¸arrow_forward
- (2) (12 points) Let f(x,y) = x²e¯. (a) (4 points) Calculate Vf. (b) (4 points) Given x directional derivative 0, find the line of vectors u = D₁f(x, y) = 0. (u1, 2) such that the - (c) (4 points) Let u= (1+3√3). Show that Duƒ(1, 0) = ¦|▼ƒ(1,0)| . What is the angle between Vf(1,0) and the vector u? Explain.arrow_forwardFind the missing values by solving the parallelogram shown in the figure. (The lengths of the diagonals are given by c and d. Round your answers to two decimal places.) a b 29 39 66.50 C 17.40 d 0 54.0 126° a Ꮎ b darrow_forward(5) (10 points) Let D be the parallelogram in the xy-plane with vertices (0, 0), (1, 1), (1, 1), (0, -2). Let f(x,y) = xy/2. Use the linear change of variables T(u, v)=(u,u2v) = (x, y) 1 to calculate the integral f(x,y) dA= 0 ↓ The domain of T is a rectangle R. What is R? |ǝ(x, y) du dv. |ð(u, v)|arrow_forward
- 2 Anot ined sove in peaper PV+96252 Q3// Find the volume of the region between the cylinder z = y2 and the xy- plane that is bounded by the planes x=1, x=2,y=-2,andy=2. vertical rect a Q4// Draw and Evaluate Soxy-2sin (ny2)dydx D Lake tarrow_forwardDetermine whether the Law of Sines or the Law of Cosines can be used to find another measure of the triangle. B 13 cm 97° Law of Sines Law of Cosines A 43° Then solve the triangle. (Round your answers to two decimal places.) b = x C = A = 40.00arrow_forwardFind the missing values by solving the parallelogram shown in the figure. (The lengths of the diagonals are given by c and d. Round your answers to two decimal places.) a 29 b 39 d Ꮎ 126° a Ꮎ b darrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Finding The Focus and Directrix of a Parabola - Conic Sections; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=KYgmOTLbuqE;License: Standard YouTube License, CC-BY