
(a)
To find:
The sum of squared errors, SSE.
Answer to Problem 9E
Solution:
The equation of the least squares regression line is
Squared error (SSE) is
Explanation of Solution
Given Information:
The numbers of parking tickets students received during one semester and their monthly
| Parking Tickets and Monthly Income | ||||||||||
| Number of Tickets, |
10 | 8 | 3 | 2 | 0 | 5 | 4 | 2 | 1 | 0 |
| Monthly Income (in Dollars), |
4000 | 3800 | 1500 | 2000 | 870 | 2500 | 1800 | 1200 | 1400 | |
The least Squares regression line is the line for which the average variation from the data is the smallest, also called the line of best fit, given by
Where ![]() is the slope of the least-squares regression line for paired data from a sample and
is the slope of the least-squares regression line for paired data from a sample and![]() is the
is the ![]() -intercept for the regression line.
-intercept for the regression line.
The sum of squared errors (SSE) for a regression line is the sum of the squares of the residuals.
Formula used:
The equation of least-squares regression line is given by
Where![]() , is the slope of the least-squares regression line given as,
, is the slope of the least-squares regression line given as,
![]() intercept given as,
intercept given as,
Here n is the number of data pairs in the sample, ![]() is the
is the ![]() value of the explanatory variable and
value of the explanatory variable and ![]() is the
is the ![]() value of response variable.
value of response variable.
The sum of squared errors (SSE) for a regression line is the sum of the squares of the residuals is given by,
Here ![]() is the
is the ![]() observed value of the response variable and
observed value of the response variable and ![]() is the predicted variable of
is the predicted variable of ![]() using the list square regression model.
using the list square regression model.
Calculation:
The table of the numbers of parking tickets students received during one semester and
their monthly Incomes is given by,
| Number of Tickets, |
Monthly Income (in Dollars), |
|||
| 10 | 4000 | 40000 | 100 | 16000000 |
| 8 | 3800 | 30400 | 64 | 14440000 |
| 3 | 1500 | 4500 | 9 | 2250000 |
| 2 | 2000 | 4000 | 4 | 4000000 |
| 0 | 870 | 0 | 0 | 756900 |
| 5 | 2500 | 12500 | 25 | 6250000 |
| 4 | 1800 | 7200 | 16 | 3240000 |
| 2 | 1000 | 2000 | 4 | 1000000 |
| 1 | 1200 | 1200 | 1 | 1440000 |
| 0 | 1400 | 0 | 0 | 1960000 |
Where,
Substitute 10 for![]() , 8 for
, 8 for![]() , 3 for
, 3 for![]() … 0 for
… 0 for ![]() in the above equation,
in the above equation,
Substitute ![]() for
for![]() ,
, ![]() for
for![]() ,
,![]() for
for![]() …
…![]() for
for ![]() in the above equation,
in the above equation,
In order to calculate the value of ![]() and 4000 for
and 4000 for ![]() in
in
Proceed in the same manner to calculate
In order to calculate the value of![]() , substitute 10 for
, substitute 10 for ![]() in
in![]() .
.
Proceed in the same manner to calculate![]() for the rest of the data and refer table for the rest of the
for the rest of the data and refer table for the rest of the ![]() values calculated.
values calculated.
In order to calculate the value of ![]() substitute 4000 for
substitute 4000 for ![]() in
in ![]() .
.
Proceed in the same manner to calculate![]() for the rest of the data and refer table for the rest of the
for the rest of the data and refer table for the rest of the ![]() values calculated.
values calculated.
The slope of the least-squares regression line is given as,
Substitute 35 for ![]() in the above equation of
in the above equation of![]() ,
,
The ![]() -intercept of regression line is given as,
-intercept of regression line is given as,
Substitute 35 for
The equation of least-squares regression line is,
The table of the numbers of parking tickets students received during one semester and
their monthly incomes is given by,
| Number of Tickets, |
Monthly Income (in Dollars), |
Predicted value, |
Residual, |
Squared error, |
| 10 | 4000 | 4047.87 | -47.87 | 2291.5369 |
| 8 | 3800 | 3419.91 | 380.09 | 144468.4081 |
| 3 | 1500 | 1850.01 | -350.01 | 122507.0001 |
| 2 | 2000 | 1536.03 | 463.97 | 215268.1609 |
| 0 | 870 | 908.07 | -38.07 | 1449.3249 |
| 5 | 2500 | 2477.97 | 22.03 | 485.3209 |
| 4 | 1800 | 2163.99 | -363.99 | 132488.7201 |
| 2 | 1000 | 1536.03 | -536.03 | 287328.1609 |
| 1 | 1200 | 1222.05 | -22.05 | 486.2025 |
| 0 | 1400 | 908.07 | 491.93 | 241995.1249 |
The predicted values ![]() are obtained by substituting the values of
are obtained by substituting the values of ![]() in the fitted regression line,
in the fitted regression line,
For![]() , substitute 10 for
, substitute 10 for ![]() in the above equation,
in the above equation,
Similarly the other values of ![]() are obtained and are shown in the third column of the table:
are obtained and are shown in the third column of the table:
The residuals ![]() and
and ![]() .
.
Substitute 4000 for ![]() and 4047.87 for
and 4047.87 for ![]() in above equation,
in above equation,
Similarly the other values of
The squared errors are obtained as,
Substitute ![]() for
for ![]() in
in
Thus,
Similarly the other values of
The sum of squared errors (SSE) for a regression line is,
From the above table SSE is given by,
Thus, the sum of squared error (SSE) is 114767.96.
Conclusion:
The equation of least-squares regression line is,
The sum of squared errors (SSE) is 1148767.96.
(b)
To find:
The Standard error of estimate, ![]() .
.
Answer to Problem 9E
Solution:
The Standard error of estimate ![]() is 378.94.
is 378.94.
Explanation of Solution
Given Information:
The numbers of parking tickets students received during one semester and their monthly
| Parking Tickets and Monthly Income | ||||||||||
| Number of Tickets, |
10 | 8 | 3 | 2 | 0 | 5 | 4 | 2 | 1 | 0 |
| Monthly Income (in Dollars), |
4000 | 3800 | 1500 | 2000 | 870 | 2500 | 1800 | 1000 | 1200 | 1400 |
Incomes is,
Formula used:
The standard error of estimate is a measure of the deviation of the sample data points from the regression line and is given by:
Here SSE is the sum of squared error and ![]() is the number of paired data set in the sample,
is the number of paired data set in the sample, ![]() is the
is the ![]() observed value of the response variable,
observed value of the response variable,
![]() is the predicted variable of
is the predicted variable of ![]() using the list square regression model.
using the list square regression model.
Calculation:
The formula of Standard error of estimate is,
From part a substitute ![]() for SSE and
for SSE and![]() for
for ![]() in the above equation of Standard error of estimate.
in the above equation of Standard error of estimate.
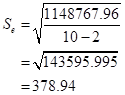
Conclusion:
Thus, the Standard error of estimate ![]() is 378.94.
is 378.94.
(c)
To Construct:
A 95% prediction interval for the given value of explanatory variable, ![]() .
.
Answer to Problem 9E
Solution:
The prediction interval is (2032.14, 3551.76).
Explanation of Solution
The prediction interval is a confidence interval for an individual value of the response
Variable y, at a given fixed value of the explanatory variable, x.
Formula used:
The formula to calculate the margin of error of a prediction interval for an individual value of the response variable, y, is given by,
n is the number of data pairs in the sample.
The formula to calculate the Prediction interval is given by,
or
Where
margin of error
The formula to calculate arithmetic mean is given by,
Where
Variables.
Calculation:
Arithmetic Mean is given as,
From part a substitute 10 for![]() , 10 for
, 10 for![]() , 8 for
, 8 for ![]() …..0 for
…..0 for ![]() in the above equation of arithmetic mean,
in the above equation of arithmetic mean,
Since the level of confidence is 95%,
Then using the t-distribution table, the critical value for this test
For ![]() -distribution with 8 degrees of freedom,
-distribution with 8 degrees of freedom,
The formula to calculate the margin of error of a prediction interval is,
Substitute 1.86 for![]() , 3.5 for
, 3.5 for![]() , 10 for
, 10 for![]() , 35 for
, 35 for![]() , 223 for
, 223 for![]() , 6 for
, 6 for ![]() and
and ![]() for
for ![]() in the above equation of margin of error,
in the above equation of margin of error,
Predicted value ![]() for
for ![]() is
is
![]()
The prediction interval is:
Substitute 2791.95for ![]() and 759.81 for
and 759.81 for ![]() in the above formula,
in the above formula,
The prediction interval is
Conclusion:
Thus, the prediction interval is
(d)
To Construct:
A 95% confidence interval for the ![]() intercept of regression line.
intercept of regression line.
Answer to Problem 9E
Solution:
The 95% confidence interval for the ![]() intercept
intercept ![]() is (496.45, 1319.69).
is (496.45, 1319.69).
Explanation of Solution
Given Information:
The numbers of parking tickets students received during one semester and their monthly
Incomes is,
| Parking Tickets and Monthly Income | ||||||||||
| Number of Tickets, x | 10 | 8 | 3 | 2 | 0 | 5 | 4 | 2 | 1 | 0 |
| Monthly Income | 4000 | 3800 | 1500 | 2000 | 870 | 2500 | 1800 | 1000 | 1200 | 1400 |
| (in Dollars), y | ||||||||||
Calculation:
To generate the regression table in excel follow the given steps:
1. Under data tab, choose data analytics and then select regression.
2. Select the input Y ![]() and select the input X range and enter the range of the given
and select the input X range and enter the range of the given ![]() data.
data.
3.Choose 95% confidence interval and click OK.
The following table will appear.
SUMMARY OUTPUT
| Regression Statistics | |||||
| Multiple R | 0.94662523 | ||||
| R Square | 0.89609937 | ||||
| Adjusted R Square | 0.88311172 | ||||
| Standard Error | 378.940622 | ||||
| Observations | 10 | ||||
ANOVA |
|||||
| df | SS | MS | F | Significance F | |
| Regression | 1 | 9907642.04 | 9907642 | 68.99665 | 3.32835E-05 |
| Residual | 8 | 1148767.96 | 143596 | ||
| Total | 9 | 11056410 | |||
| Coefficients | Standard Error | t Stat | P-value | Lower 95.0% | Upper 95.0% | |
| Intercept | 908.0696517 | 178.5009634 | 5.087197 | 0.000945 | 496.4456924 | 1319.69361 |
| X | 313.9800995 | 37.79968083 | 8.306422 | 3.33E-05 | 226.8138793 | 401.14632 |
From the above result,
1. Multiple R is the absolute value of the ![]() .
.
2.
3. Standard Error is the standard error of the estimate, ![]() .
.
4. The intersection of the Residual row and the SS column is the sum of squared errors, SSE.
5. The Lower 95.0% and the Upper 95.0% columns give the lower and upper endpoints of the 95% confidence intervals for the ![]() intercept and slope.
intercept and slope.
6. The coefficient columns gives the values for the coefficients, that is, the
![]() intercept and slope, of the regression line.
intercept and slope, of the regression line.
The row labeled Intercept is the row for the values corresponding to the ![]() intercept. The last two values in this row are the lower and upper endpoints for a 95% confidence for the
intercept. The last two values in this row are the lower and upper endpoints for a 95% confidence for the ![]() intercept of the regression line,
intercept of the regression line,
Thus, the 95% confidence interval for
Confidence Interval=(496.45, 1319.69).
Conclusion:
Thus, the 95% confidence interval for the ![]() intercept
intercept ![]() is(496.45, 1319.69).
is(496.45, 1319.69).
(e)
To Construct:
A 95% confidence interval for the slope of regression line.
Answer to Problem 9E
Solution:
Thus, the 95% confidence interval for the slope
Explanation of Solution
Given Information:
The numbers of parking tickets students received during one semester and their monthly
Incomes is,
| Parking Tickets and Monthly Income | ||||||||||
| Number of Tickets, x | 10 | 8 | 3 | 2 | 0 | 5 | 4 | 2 | 1 | 0 |
| Monthly Income | 4000 | 3800 | 1500 | 2000 | 870 | 2500 | 1800 | 1000 | 1200 | 1400 |
| (in Dollars), y | ||||||||||
Calculation:
To generate the regression table in excel follow the given steps:
1. Under data tab, choose data analytics and then select regression.
2. Select the input Y range and enter the range of the given ![]() and select the input X range and enter the range of the given
and select the input X range and enter the range of the given ![]() data.
data.
3.Choose 95% confidence interval and click OK.
The following table will appear.
SUMMARY OUTPUT
| Regression Statistics | ||||||
| Multiple R | 0.946625253 | |||||
| R Square | 0.89609937 | |||||
| Adjusted R Square | 0.883111792 | |||||
| Standard Error | 378.940622 | |||||
| Observations | 10 | |||||
| ANOVA | ||||||
| df | SS | MS | F | Significance F | ||
| Regression | 1 | 9907642.04 | 9907642 | 68.99665 | 3.32835E-05 | |
| Residual | 8 | 1148767.96 | 143596 | |||
| Total | 9 | 11056410 | ||||
| Coefficients | Standard Error | t Stat | P-value | Lower 95.0% | Upper 95.0% | |
| Intercept | 908.0696517 | 178.5009634 | 5.087197 | 0.000945 | 496.4456924 | 1319.69361 |
| X | 313.9800995 | 37.79968083 | 8.306422 | 3.33E-05 | 226.8138793 | 401.14632 |
From the above result,
1. Multiple R is the absolute value of the ![]() .
.
2.
3. Standard Error is the standard error of the estimate, ![]() .
.
4. The intersection of the Residual row and the SS column is the sum of squared errors, SSE.
5. The Lower 95.0% and the Upper 95.0% columns give the lower and upper endpoints of the 95% confidence intervals for the ![]() intercept and slope.
intercept and slope.
6. The coefficient columns gives the values for the coefficients, that is, the
![]() intercept and slope, of the regression line.
intercept and slope, of the regression line.
The row labeled ![]() is the row for the values corresponding to the slope of regression line. The last two values in this row are the lower and upper endpoints for a 95% confidence for the slope of the regression line,
is the row for the values corresponding to the slope of regression line. The last two values in this row are the lower and upper endpoints for a 95% confidence for the slope of the regression line,
Thus, the 95% confidence interval for
Confidence Interval=(226.81, 401.15).
Conclusion:
Thus, the 95% confidence interval for the slope ![]() is(226.81, 401.15).
is(226.81, 401.15).
Want to see more full solutions like this?
Chapter 12 Solutions
Beginning Statistics
- A survey of 250 young professionals found that two-thirds of them use their cell phones primarily for e-mail. Can you conclude statistically that the population proportion who use cell phones primarily for e-mail is less than 0.72? Use a 95% confidence interval. Question content area bottom Part 1 The 95% confidence interval is [ ], [ ] As 0.72 is ▼ above the upper limit within the limits below the lower limit of the confidence interval, we ▼ can cannot conclude that the population proportion is less than 0.72. (Use ascending order. Round to four decimal places as needed.)arrow_forwardI need help with this problem and an explanation of the solution for the image described below. (Statistics: Engineering Probabilities)arrow_forwardI need help with this problem and an explanation of the solution for the image described below. (Statistics: Engineering Probabilities)arrow_forward
- I need help with this problem and an explanation of the solution for the image described below. (Statistics: Engineering Probabilities)arrow_forwardQuestions An insurance company's cumulative incurred claims for the last 5 accident years are given in the following table: Development Year Accident Year 0 2018 1 2 3 4 245 267 274 289 292 2019 255 276 288 294 2020 265 283 292 2021 263 278 2022 271 It can be assumed that claims are fully run off after 4 years. The premiums received for each year are: Accident Year Premium 2018 306 2019 312 2020 318 2021 326 2022 330 You do not need to make any allowance for inflation. 1. (a) Calculate the reserve at the end of 2022 using the basic chain ladder method. (b) Calculate the reserve at the end of 2022 using the Bornhuetter-Ferguson method. 2. Comment on the differences in the reserves produced by the methods in Part 1.arrow_forwardQuestions An insurance company's cumulative incurred claims for the last 5 accident years are given in the following table: Development Year Accident Year 0 2018 1 2 3 4 245 267 274 289 292 2019 255 276 288 294 2020 265 283 292 2021 263 278 2022 271 It can be assumed that claims are fully run off after 4 years. The premiums received for each year are: Accident Year Premium 2018 306 2019 312 2020 318 2021 326 2022 330 You do not need to make any allowance for inflation. 1. (a) Calculate the reserve at the end of 2022 using the basic chain ladder method. (b) Calculate the reserve at the end of 2022 using the Bornhuetter-Ferguson method. 2. Comment on the differences in the reserves produced by the methods in Part 1.arrow_forward
- From a sample of 26 graduate students, the mean number of months of work experience prior to entering an MBA program was 34.67. The national standard deviation is known to be18 months. What is a 90% confidence interval for the population mean? Question content area bottom Part 1 A 9090% confidence interval for the population mean is left bracket nothing comma nothing right bracketenter your response here,enter your response here. (Use ascending order. Round to two decimal places as needed.)arrow_forwardA test consists of 10 questions made of 5 answers with only one correct answer. To pass the test, a student must answer at least 8 questions correctly. (a) If a student guesses on each question, what is the probability that the student passes the test? (b) Find the mean and standard deviation of the number of correct answers. (c) Is it unusual for a student to pass the test by guessing? Explain.arrow_forwardIn a group of 40 people, 35% have never been abroad. Two people are selected at random without replacement and are asked about their past travel experience. a. Is this a binomial experiment? Why or why not? What is the probability that in a random sample of 2, no one has been abroad? b. What is the probability that in a random sample of 2, at least one has been abroad?arrow_forward
- Questions An insurance company's cumulative incurred claims for the last 5 accident years are given in the following table: Development Year Accident Year 0 2018 1 2 3 4 245 267 274 289 292 2019 255 276 288 294 2020 265 283 292 2021 263 278 2022 271 It can be assumed that claims are fully run off after 4 years. The premiums received for each year are: Accident Year Premium 2018 306 2019 312 2020 318 2021 326 2022 330 You do not need to make any allowance for inflation. 1. (a) Calculate the reserve at the end of 2022 using the basic chain ladder method. (b) Calculate the reserve at the end of 2022 using the Bornhuetter-Ferguson method. 2. Comment on the differences in the reserves produced by the methods in Part 1.arrow_forwardTo help consumers in purchasing a laptop computer, Consumer Reports calculates an overall test score for each computer tested based upon rating factors such as ergonomics, portability, performance, display, and battery life. Higher overall scores indicate better test results. The following data show the average retail price and the overall score for ten 13-inch models (Consumer Reports website, October 25, 2012). Brand & Model Price ($) Overall Score Samsung Ultrabook NP900X3C-A01US 1250 83 Apple MacBook Air MC965LL/A 1300 83 Apple MacBook Air MD231LL/A 1200 82 HP ENVY 13-2050nr Spectre XT 950 79 Sony VAIO SVS13112FXB 800 77 Acer Aspire S5-391-9880 Ultrabook 1200 74 Apple MacBook Pro MD101LL/A 1200 74 Apple MacBook Pro MD313LL/A 1000 73 Dell Inspiron I13Z-6591SLV 700 67 Samsung NP535U3C-A01US 600 63 a. Select a scatter diagram with price as the independent variable. b. What does the scatter diagram developed in part (a) indicate about the relationship…arrow_forwardTo the Internal Revenue Service, the reasonableness of total itemized deductions depends on the taxpayer’s adjusted gross income. Large deductions, which include charity and medical deductions, are more reasonable for taxpayers with large adjusted gross incomes. If a taxpayer claims larger than average itemized deductions for a given level of income, the chances of an IRS audit are increased. Data (in thousands of dollars) on adjusted gross income and the average or reasonable amount of itemized deductions follow. Adjusted Gross Income ($1000s) Reasonable Amount ofItemized Deductions ($1000s) 22 9.6 27 9.6 32 10.1 48 11.1 65 13.5 85 17.7 120 25.5 Compute b1 and b0 (to 4 decimals).b1 b0 Complete the estimated regression equation (to 2 decimals). = + x Predict a reasonable level of total itemized deductions for a taxpayer with an adjusted gross income of $52.5 thousand (to 2 decimals). thousand dollarsWhat is the value, in dollars, of…arrow_forward
 MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman





