Finite Mathematics and Application Calculus (Looseleaf) - Text Only
Finite Mathematics and Application Calculus (Looseleaf) - Text Only
7th Edition
ISBN: 9781337291484
Author: Waner
Publisher: Cengage
bartleby

Concept explainers

Unitary Method
The word “unitary” comes from the word “unit”, which means a single and complete entity. In this method, we find the value of a unit product from the given number of products, and then we solve for the other number of products.
Speed, Time, and Distance
Imagine you and 3 of your friends are planning to go to the playground at 6 in the evening. Your house is one mile away from the playground and one of your friends named Jim must start at 5 pm to reach the playground by walk. The other two friends are 3 …
Profit and Loss
The amount earned or lost on the sale of one or more items is referred to as the profit or loss on that item.
Units and Measurements
Measurements and comparisons are the foundation of science and engineering. We, therefore, need rules that tell us how things are measured and compared. For these measurements and comparisons, we perform certain experiments, and we will need the experime…
bartleby

Videos

Question
Book Icon
Chapter 12.3, Problem 75E

(a)

To determine

The nearest year in which the rate of change of yearly industrial output reaches a maximum where the graph shows the yearly industrial output (measured in billions of Zonars) of the city-state of Utarek on mars over 7-year period

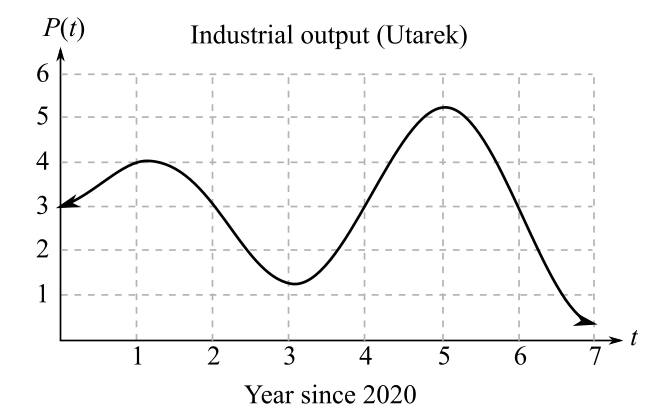
Finite Mathematics and Application Calculus (Looseleaf) - Text Only, Chapter 12.3, Problem 75E , additional homework tip 1

(b)

To determine

The nearest year in which the rate of change of yearly industrial output reaches a minimum where the graph shows the yearly industrial output (measured in billions of Zonars) of the city-state of Utarek on mars over 7-year period

Finite Mathematics and Application Calculus (Looseleaf) - Text Only, Chapter 12.3, Problem 75E , additional homework tip 2

(c)

To determine

The nearest year in which the graph first change from concave up to concave down and the correct option from the options about the result a) in that year the rate of change of industrial output reached a minimum compared to the nearby years b) in that year the rate of change of industrial output reached a maximum compared to the nearby years; where the graph shows the yearly industrial output (measured in billions of Zonars) of the city-state of Utarek on mars over 7-year period

Finite Mathematics and Application Calculus (Looseleaf) - Text Only, Chapter 12.3, Problem 75E , additional homework tip 3

Expert Solution & Answer
Check Mark

Want to see the full answer?

Check out a sample textbook solution
Blurred answer
Previous
Chapter 12.3, Problem 74E
Next
Chapter 12.3, Problem 76E
Students have asked these similar questions
Explain the focus and reasons for establishment of 12.4.1(root test) and 12.4.2(ratio test)
use Integration by Parts to derive 12.6.1
Explain the relationship between 12.3.6, (case A of 12.3.6) and 12.3.7

Chapter 12 Solutions

Finite Mathematics and Application Calculus (Looseleaf) - Text Only

Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 112, locate and classify all extrema...
Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 112, locate and classify all extrema...Ch. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - Prob. 15ECh. 12.1 - Prob. 16ECh. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - Prob. 19ECh. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - Prob. 24ECh. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - Prob. 26ECh. 12.1 - Prob. 27ECh. 12.1 - Prob. 28ECh. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - Prob. 32ECh. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - Prob. 34ECh. 12.1 - Prob. 35ECh. 12.1 - Prob. 36ECh. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - Prob. 38ECh. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - Prob. 40ECh. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - Prob. 42ECh. 12.1 - In Exercises 13-44, find the exact location of all...Ch. 12.1 - Prob. 44ECh. 12.1 - In Exercises 4548, use graphing technology and the...Ch. 12.1 - Prob. 46ECh. 12.1 - Prob. 47ECh. 12.1 - Prob. 48ECh. 12.1 - In Exercises 4956 the graph of the derivative of a...Ch. 12.1 - Prob. 50ECh. 12.1 - In Exercises 4956 the graph of the derivative of a...Ch. 12.1 - Prob. 52ECh. 12.1 - In Exercises 4956 the graph of the derivative of a...Ch. 12.1 - Prob. 54ECh. 12.1 - Prob. 55ECh. 12.1 - Prob. 56ECh. 12.1 - Prob. 57ECh. 12.1 - Prob. 58ECh. 12.1 - Draw the graph of a function that has stationary...Ch. 12.1 - Prob. 60ECh. 12.1 - Prob. 61ECh. 12.1 - Prob. 62ECh. 12.1 - We said that if f is continuous on a closed...Ch. 12.1 - Prob. 64ECh. 12.1 - Prob. 65ECh. 12.1 - Prob. 66ECh. 12.2 - In Exercises 1-8, solve the given optimization...Ch. 12.2 - In Exercises 1-8, solve the given optimization...Ch. 12.2 - In Exercises 1-8, solve the given optimization...Ch. 12.2 - In Exercises 1-8, solve the given optimization...Ch. 12.2 - In Exercises 1-8, solve the given optimization...Ch. 12.2 - Prob. 6ECh. 12.2 - Prob. 7ECh. 12.2 - Prob. 8ECh. 12.2 - For a rectangle with perimeter 20 to have the...Ch. 12.2 - Prob. 10ECh. 12.2 - Advertising Costs The cost, in thousands of...Ch. 12.2 - Advertising Costs The cost, in billions of...Ch. 12.2 - Average Cost: iPhones Assume that it costs Apple...Ch. 12.2 - Prob. 14ECh. 12.2 - Prob. 15ECh. 12.2 - Prob. 16ECh. 12.2 - Prob. 17ECh. 12.2 - Prob. 18ECh. 12.2 - Fences I would like to create a rectangular...Ch. 12.2 - Fences I would like to create a rectangular orchid...Ch. 12.2 - Prob. 21ECh. 12.2 - Prob. 22ECh. 12.2 - Fences (Compare Exercise 19.) For tax reasons I...Ch. 12.2 - Fences (Compare Exercise 20.) For reasons too...Ch. 12.2 - Revenue Hercules Films is deciding on the price of...Ch. 12.2 - Prob. 26ECh. 12.2 - Prob. 27ECh. 12.2 - Projected Revenue: Smartphones Worldwide annual...Ch. 12.2 - Revenue: Monorail Service The demand for monorail...Ch. 12.2 - Prob. 30ECh. 12.2 - Prob. 31ECh. 12.2 - Prob. 32ECh. 12.2 - Prob. 33ECh. 12.2 - Prob. 34ECh. 12.2 - Profit: Smartphones (Compare Exercise 27.)...Ch. 12.2 - Projected Profit: Smartphones (Compare Exercise...Ch. 12.2 - Prob. 37ECh. 12.2 - Profit Because of sales by a competing company,...Ch. 12.2 - Prob. 39ECh. 12.2 - Metal Drums A company manufactures cylindrical...Ch. 12.2 - Tin Cans A company manufactures cylindrical tin...Ch. 12.2 - Prob. 42ECh. 12.2 - Prob. 43ECh. 12.2 - Prob. 44ECh. 12.2 - Box Design A packaging company is going to make...Ch. 12.2 - Prob. 46ECh. 12.2 - Prob. 47ECh. 12.2 - Carry-on Dimensions American Airlines requires...Ch. 12.2 - Prob. 49ECh. 12.2 - Prob. 50ECh. 12.2 - Package Dimensions The U.S. Postal Service (USPS)...Ch. 12.2 - Prob. 52ECh. 12.2 - Prob. 53ECh. 12.2 - Cellphone Revenues (Refer to Exercise 53.) If we...Ch. 12.2 - Prob. 55ECh. 12.2 - Prob. 56ECh. 12.2 - Prob. 57ECh. 12.2 - Prob. 58ECh. 12.2 - Prob. 59ECh. 12.2 - Prob. 60ECh. 12.2 - Average Profit The FeatureRich Software Company...Ch. 12.2 - Prob. 62ECh. 12.2 - Prob. 63ECh. 12.2 - Resource Allocation Your company is the largest...Ch. 12.2 - Prob. 65ECh. 12.2 - Prob. 66ECh. 12.2 - Prob. 67ECh. 12.2 - Prison Population The prison population in the...Ch. 12.2 - Prob. 69ECh. 12.2 - Prob. 70ECh. 12.2 - Prob. 71ECh. 12.2 - Prob. 72ECh. 12.2 - Prob. 73ECh. 12.2 - Prob. 74ECh. 12.2 - Prob. 75ECh. 12.2 - Agriculture Two years ago, your orange orchard...Ch. 12.2 - Prob. 77ECh. 12.2 - Prob. 78ECh. 12.2 - Prob. 79ECh. 12.2 - Prob. 80ECh. 12.2 - Prob. 81ECh. 12.2 - Prob. 82ECh. 12.2 - Prob. 83ECh. 12.2 - Prob. 84ECh. 12.2 - Prob. 85ECh. 12.2 - Explain how you would solve an optimization...Ch. 12.3 - In Exercises 1-10, calculate d2ydx2. [HINT: See...Ch. 12.3 - Prob. 2ECh. 12.3 - In Exercises 1-10, calculate d2ydx2. [HINT: See...Ch. 12.3 - Prob. 4ECh. 12.3 - In Exercises 1-10, calculate d2ydx2. [HINT: See...Ch. 12.3 - Prob. 6ECh. 12.3 - In Exercises 1-10, calculate d2ydx2. [HINT: See...Ch. 12.3 - In Exercises 1-10, calculate d2ydx2. [HINT: See...Ch. 12.3 - In Exercises 1-10, calculate d2ydx2. [HINT: See...Ch. 12.3 - Prob. 10ECh. 12.3 - In Exercises 1116 the position s of a point (in...Ch. 12.3 - In Exercises 1116 the position s of a point (in...Ch. 12.3 - Prob. 13ECh. 12.3 - In Exercises 1116 the position s of a point (in...Ch. 12.3 - In Exercises 1116 the position s of a point (in...Ch. 12.3 - Prob. 16ECh. 12.3 - Prob. 17ECh. 12.3 - Prob. 18ECh. 12.3 - Prob. 19ECh. 12.3 - Prob. 20ECh. 12.3 - Prob. 21ECh. 12.3 - Prob. 22ECh. 12.3 - In Exercises 1724 the graph of a function is...Ch. 12.3 - Prob. 24ECh. 12.3 - Prob. 25ECh. 12.3 - Prob. 26ECh. 12.3 - Prob. 27ECh. 12.3 - Prob. 28ECh. 12.3 - Prob. 29ECh. 12.3 - Prob. 30ECh. 12.3 - Prob. 31ECh. 12.3 - Prob. 32ECh. 12.3 - Prob. 33ECh. 12.3 - In Exercises 3344, find the x-coordinates of all...Ch. 12.3 - Prob. 35ECh. 12.3 - Prob. 36ECh. 12.3 - Prob. 37ECh. 12.3 - Prob. 38ECh. 12.3 - Prob. 39ECh. 12.3 - Prob. 40ECh. 12.3 - Prob. 41ECh. 12.3 - Prob. 42ECh. 12.3 - Prob. 43ECh. 12.3 - Prob. 44ECh. 12.3 - Prob. 45ECh. 12.3 - Prob. 46ECh. 12.3 - Prob. 47ECh. 12.3 - Prob. 48ECh. 12.3 - Prob. 49ECh. 12.3 - Prob. 50ECh. 12.3 - Prob. 51ECh. 12.3 - Prob. 52ECh. 12.3 - Prob. 53ECh. 12.3 - Prob. 54ECh. 12.3 - Acceleration on Mars If a stone is dropped from a...Ch. 12.3 - Prob. 56ECh. 12.3 - Motion in a Straight Line The position of a...Ch. 12.3 - Motion in a Straight Line The position of a...Ch. 12.3 - Bottled Water Sales Annual sales of bottled water...Ch. 12.3 - Bottled Water Sales Annual U.S. per capita sales...Ch. 12.3 - Embryo Development The daily oxygen consumption of...Ch. 12.3 - Embryo Development The daily oxygen consumption of...Ch. 12.3 - Inflation The following graph shows the...Ch. 12.3 - Prob. 64ECh. 12.3 - Inflation The following graph shows the...Ch. 12.3 - Inflation The following graph shows the...Ch. 12.3 - Prob. 67ECh. 12.3 - Scientific Research: 19832003 The percentage of...Ch. 12.3 - Prob. 69ECh. 12.3 - Prob. 70ECh. 12.3 - Prob. 71ECh. 12.3 - Prob. 72ECh. 12.3 - Epidemics The following graph shows the total...Ch. 12.3 - Prob. 74ECh. 12.3 - Prob. 75ECh. 12.3 - Prob. 76ECh. 12.3 - Prob. 77ECh. 12.3 - Prob. 78ECh. 12.3 - Prob. 79ECh. 12.3 - Prob. 80ECh. 12.3 - Oil Imports from Mexico Daily oil production in...Ch. 12.3 - Oil Imports from Mexico Repeat Exercises 81 using...Ch. 12.3 - Prob. 83ECh. 12.3 - Prob. 84ECh. 12.3 - Prob. 85ECh. 12.3 - Prob. 86ECh. 12.3 - Prob. 87ECh. 12.3 - Prob. 88ECh. 12.3 - Prob. 89ECh. 12.3 - Prob. 90ECh. 12.3 - Prob. 91ECh. 12.3 - Prob. 92ECh. 12.3 - Prob. 93ECh. 12.3 - Prob. 94ECh. 12.3 - Prob. 95ECh. 12.3 - Prob. 96ECh. 12.3 - Prob. 97ECh. 12.3 - Prob. 98ECh. 12.4 - In Exercises 126, sketch the graph of the given...Ch. 12.4 - In Exercises 126, sketch the graph of the given...Ch. 12.4 - In Exercises 126, sketch the graph of the given...Ch. 12.4 - Prob. 4ECh. 12.4 - Prob. 5ECh. 12.4 - Prob. 6ECh. 12.4 - Prob. 7ECh. 12.4 - Prob. 8ECh. 12.4 - Prob. 9ECh. 12.4 - Prob. 10ECh. 12.4 - Prob. 11ECh. 12.4 - Prob. 12ECh. 12.4 - In Exercises 126, sketch the graph of the given...Ch. 12.4 - Prob. 14ECh. 12.4 - Prob. 15ECh. 12.4 - Prob. 16ECh. 12.4 - Prob. 17ECh. 12.4 - Prob. 18ECh. 12.4 - Prob. 19ECh. 12.4 - In Exercises 126, sketch the graph of the given...Ch. 12.4 - Prob. 21ECh. 12.4 - Prob. 22ECh. 12.4 - Prob. 23ECh. 12.4 - Prob. 24ECh. 12.4 - Prob. 25ECh. 12.4 - Prob. 26ECh. 12.4 - Prob. 27ECh. 12.4 - Prob. 28ECh. 12.4 - Prob. 29ECh. 12.4 - Prob. 30ECh. 12.4 - Prob. 31ECh. 12.4 - Prob. 32ECh. 12.4 - Prob. 33ECh. 12.4 - Prob. 34ECh. 12.4 - Consumer Price Index The following graph shows the...Ch. 12.4 - Prob. 36ECh. 12.4 - Prob. 37ECh. 12.4 - Prob. 38ECh. 12.4 - Average Cost: iPhone Assume that is costs Apple...Ch. 12.4 - Average Cost: PlayStation 4s Assume that it costs...Ch. 12.4 - Prob. 41ECh. 12.4 - Subprime Mortgage Debt during the Housing Bubble...Ch. 12.4 - Prob. 43ECh. 12.4 - Prob. 44ECh. 12.4 - Prob. 45ECh. 12.4 - Prob. 46ECh. 12.4 - Prob. 47ECh. 12.4 - Prob. 48ECh. 12.4 - Prob. 49ECh. 12.4 - Prob. 50ECh. 12.5 - Rewrite the statements and questions in Exercises...Ch. 12.5 - Rewrite the statements and questions in Exercises...Ch. 12.5 - Rewrite the statements and questions in Exercises...Ch. 12.5 - Rewrite the statements and questions in Exercises...Ch. 12.5 - Prob. 5ECh. 12.5 - Prob. 6ECh. 12.5 - Prob. 7ECh. 12.5 - Prob. 8ECh. 12.5 - Sunspots The area of a circular sunspot is growing...Ch. 12.5 - Puddles The radius of a circular puddle is growing...Ch. 12.5 - Prob. 11ECh. 12.5 - Prob. 12ECh. 12.5 - End of the Earth In 5 billion years the Sun will...Ch. 12.5 - Prob. 14ECh. 12.5 - Sliding Ladders The base of a 50-foot ladder is...Ch. 12.5 - Prob. 16ECh. 12.5 - Prob. 17ECh. 12.5 - Prob. 18ECh. 12.5 - Prob. 19ECh. 12.5 - Average Cost Repeat Exercise 19, using the revised...Ch. 12.5 - Prob. 21ECh. 12.5 - Supply The number of retro portable CD players you...Ch. 12.5 - Revenue You can now sell 50 cups of lemonade per...Ch. 12.5 - Prob. 24ECh. 12.5 - Prob. 25ECh. 12.5 - Oil Expenditures Daily oil imports to the United...Ch. 12.5 - Resource Allocation Your company manufactures...Ch. 12.5 - Prob. 28ECh. 12.5 - Prob. 29ECh. 12.5 - Prob. 30ECh. 12.5 - Prob. 31ECh. 12.5 - Demand The demand equation for rubies at Royal...Ch. 12.5 - Prob. 33ECh. 12.5 - Prob. 34ECh. 12.5 - Baseball A baseball diamond is a square with side...Ch. 12.5 - Prob. 36ECh. 12.5 - Movement along a Graph A point on the graph of...Ch. 12.5 - Prob. 38ECh. 12.5 - Prob. 39ECh. 12.5 - Prob. 40ECh. 12.5 - Prob. 41ECh. 12.5 - Prob. 42ECh. 12.5 - Cones A right circular conical vessel is being...Ch. 12.5 - More Cones A circular conical vessel is being...Ch. 12.5 - Prob. 45ECh. 12.5 - Prob. 46ECh. 12.5 - Prob. 47ECh. 12.5 - Computers vs. Income Refer back to the model in...Ch. 12.5 - Prob. 49ECh. 12.5 - Prob. 50ECh. 12.5 - Prob. 51ECh. 12.5 - Prob. 52ECh. 12.5 - Prob. 53ECh. 12.5 - Prob. 54ECh. 12.5 - Prob. 55ECh. 12.5 - Prob. 56ECh. 12.5 - Prob. 57ECh. 12.5 - Prob. 58ECh. 12.5 - Prob. 59ECh. 12.5 - Prob. 60ECh. 12.5 - Prob. 61ECh. 12.5 - Prob. 62ECh. 12.6 - Demand for Oranges The weekly sales of Honolulu...Ch. 12.6 - Demand for Oranges Repeat Exercise 1 for weekly...Ch. 12.6 - Demand for Smartphones Worldwide annual sales of...Ch. 12.6 - Prob. 4ECh. 12.6 - Prob. 5ECh. 12.6 - Monorail Services The demand for monorail service...Ch. 12.6 - Tissues The consumer demand equation for tissues...Ch. 12.6 - Bodybuilding The consumer demand curve for...Ch. 12.6 - T-Shirts The Physics Club sells E=mc2 T-shirts at...Ch. 12.6 - Comics The demand curve for original Iguanawoman...Ch. 12.6 - E-Readers The demand for Amazons Kindle e-reader...Ch. 12.6 - Monorail Service on Mars The demand for monorail...Ch. 12.6 - Corn In the 1930s the economist Henry Schultz...Ch. 12.6 - Demand for Fried Chicken A fried chicken franchise...Ch. 12.6 - Paint-By-Number The estimated monthly sales of...Ch. 12.6 - Prob. 16ECh. 12.6 - Prob. 17ECh. 12.6 - Prob. 18ECh. 12.6 - Hyperbolic Demand Functions A general hyperbolic...Ch. 12.6 - Quadratic Demand Functions A general quadratic...Ch. 12.6 - Modeling Linear Demand You have been hired as a...Ch. 12.6 - Modeling Linear Demand You have been hired as a...Ch. 12.6 - Modeling Exponential Demand As the new owner of a...Ch. 12.6 - Prob. 24ECh. 12.6 - Income Elasticity of Demand: Live Drama The...Ch. 12.6 - Prob. 26ECh. 12.6 - Income Elasticity of Demand: Broadband in 2010 The...Ch. 12.6 - Prob. 28ECh. 12.6 - Income Elasticity of Demand: Computer Usage in the...Ch. 12.6 - Prob. 30ECh. 12.6 - Prob. 31ECh. 12.6 - Price Elasticity of Supply Given a supply equation...Ch. 12.6 - Income Elasticity of Demand (based on a question...Ch. 12.6 - Prob. 34ECh. 12.6 - Prob. 35ECh. 12.6 - Prob. 36ECh. 12.6 - Prob. 37ECh. 12.6 - Prob. 38ECh. 12.6 - Prob. 39ECh. 12.6 - Prob. 40ECh. 12 - In Exercises 18, find all the relative and...Ch. 12 - Prob. 2RECh. 12 - Prob. 3RECh. 12 - Prob. 4RECh. 12 - Prob. 5RECh. 12 - Prob. 6RECh. 12 - Prob. 7RECh. 12 - Prob. 8RECh. 12 - Prob. 9RECh. 12 - Prob. 10RECh. 12 - Prob. 11RECh. 12 - Prob. 12RECh. 12 - Prob. 13RECh. 12 - Prob. 14RECh. 12 - Prob. 15RECh. 12 - Prob. 16RECh. 12 - Prob. 17RECh. 12 - Prob. 18RECh. 12 - Prob. 19RECh. 12 - Prob. 20RECh. 12 - Prob. 21RECh. 12 - Prob. 22RECh. 12 - Revenue Demand for the latest best-seller at...Ch. 12 - Prob. 24RECh. 12 - Prob. 25RECh. 12 - Prob. 26RECh. 12 - Office Space Although still a sophomore at...Ch. 12 - Prob. 28RECh. 12 - Box Design The sales department at...Ch. 12 - Prob. 30RECh. 12 - Prob. 31RECh. 12 - Prob. 32RECh. 12 - Prob. 33RECh. 12 - Prob. 34RECh. 12 - Elasticity of Demand (Compare Exercise 23.) Demand...Ch. 12 - Prob. 36RECh. 12 - Prob. 37RECh. 12 - Prob. 38RE
Knowledge Booster
Background pattern image
Calculus
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Text book image
College Algebra
Algebra
ISBN:9781938168383
Author:Jay Abramson
Publisher:OpenStax
Text book image
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Text book image
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Text book image
Functions and Change: A Modeling Approach to Coll...
Algebra
ISBN:9781337111348
Author:Bruce Crauder, Benny Evans, Alan Noell
Publisher:Cengage Learning
Text book image
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
Use of ALGEBRA in REAL LIFE; Author: Fast and Easy Maths !;https://www.youtube.com/watch?v=9_PbWFpvkDc;License: Standard YouTube License, CC-BY
Compound Interest Formula Explained, Investment, Monthly & Continuously, Word Problems, Algebra; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=P182Abv3fOk;License: Standard YouTube License, CC-BY
Applications of Algebra (Digit, Age, Work, Clock, Mixture and Rate Problems); Author: EngineerProf PH;https://www.youtube.com/watch?v=Y8aJ_wYCS2g;License: Standard YouTube License, CC-BY