MATH IN OUR WORLD (LOOSELEAF)-W/ACCESS
3rd Edition
ISBN: 9781259969690
Author: sobecki
Publisher: MCG
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 12, Problem 1RE
Use this information for Exercises 1–4: the preference ballots for an election for the best speaker in a contest are shown. There are three candidates: Peterson (P), Quintana (Q), and Ross (R).
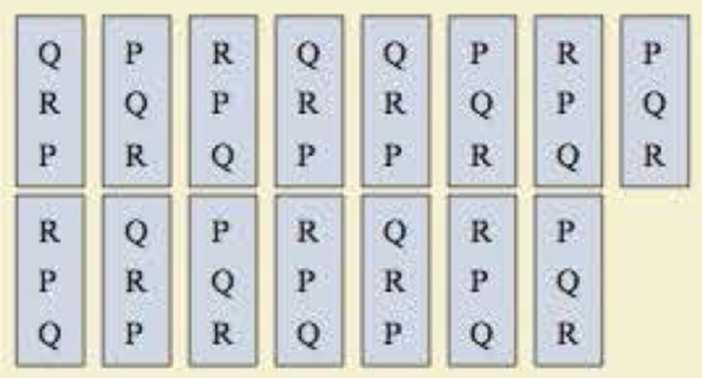
1. Construct a preference table for the results of the election.
Expert Solution & Answer
To determine
To construct: The preference table for the results in the election.
Answer to Problem 1RE
The preference table for the results in the election are listed below.
| Number of votes | 5 | 5 | 5 |
| First choice | Q | P | R |
| Second choice | R | Q | P |
| Third choice | P | R | Q |
Explanation of Solution
Given:
The three candidates are Peterson (P), Quintana (Q) and Ross (R).
The preference table of the best speaker in a contest for the election are listed below.
| Q | P | R | Q | Q | P | R | P | R | Q | P | R | Q | R | P |
| R | Q | P | R | R | Q | P | Q | P | R | Q | P | R | P | Q |
| P | R | Q | P | P | R | Q | R | Q | P | R | Q | P | Q | R |
Calculation:
The choices are Peterson (P), Quintana (Q) and Ross (R).
From the ballot preference table, it is noticed that the candidate order QRP occurs 5 times, the candidate order PQR occurs 5 times and the candidate order RPQ occurs 5 times.
That is, the orders of QRP, PQR and RPQ is 5, 5 and 5.
Therefore, the preference table of the results in the election are listed below.
| Number of votes | 5 | 5 | 5 |
| First choice | Q | P | R |
| Second choice | R | Q | P |
| Third choice | P | R | Q |
Want to see more full solutions like this?
Subscribe now to access step-by-step solutions to millions of textbook problems written by subject matter experts!
Students have asked these similar questions
The Rosetown community association plans to meet once each month. The association members are deciding what day of the week to meet by holding a vote.
The choices are Monday (M), Tuesday (T), and Friday (F). Instead of voting for only one day, each member ranks the three days in order of preference. Each
member's ballot gives the member's first, second, and third choice for a monthly meeting day. Here are the ballots submitted.
Baliot 1
Ballot 2
Ballot 3
Ballot 4
Ballot 5
Ballot 6
Balot 7
Ballot 8
Ballot 9
1st
M.
14
M
F.
M.
1st
2n
2n
2nd
2nd
M
M
T.
2nd
2
2nd
2nd
2nd
M.
3rd
3rd
3rd
F
3rd
3rd
M
3rd
3rd
M.
3rd
Ballot 10
Ballot 11
Ballot 12
Ballot 13
Balot 14
Ballot 15
Ballot 16
1st
F
M
1st
T.
M
14
2nd
T.
2nd
T.
2nd
2ne
20
2n M
2nd
F
M
3rd
M
3rd
3rd
M
3rd
3
3rd
Construct a preference table to summarize the ballots. You can add or remove columns in the table as needed. Note: The individual ballots above will highlight
when clicked. (Highlighting the ballots can help you with your…
The students in a class get to decide on the color of the classroom. The two choices are blue and yellow. Polling shows yellow is preferred by a small
majority, 53% for yellow to 47% for blue. However, before the vote is held, a new color choice is added: red. Not a lot of people like red, but there are
some yellow supporters who would actually vote for red. The results of the vote are below.
Blue
Yellow
Red
46%
44%
10%
What statement below is correct about adding a third choice to the plurality vote?
O a
Ob
Oc
d
Red did not win the vote, but it draws enough support away from yellow to make blue the winner, even though a majority of
students preferred yellow over blue when there were two choices.
Red did not win the vote, but it had no effect on drawing enough support away from yellow to make blue the winner, even though
a majority of students preferred yellow over blue when there were two choices.
Red did not win the vote, so because of this, yellow should win the vote over blue.…
A Senior High School class held an election for class president. Instead of just voting for one candidate, the students needed to rank all the candidates according to preference. The table presents the results.
RANKINGS
Anton
2
3
1
3
4
2
Maria
4
1
3
4
1
3
Shelly
1
2
2
2
3
4
Troy
3
4
4
1
2
1
Number of Votes
36
53
41
27
31
45
1. Who is the winner by plurality? 2. What is the majority number of votes?
Chapter 12 Solutions
MATH IN OUR WORLD (LOOSELEAF)-W/ACCESS
Ch. 12.1 - The Student Activities Committee at Camden College...Ch. 12.1 - An election was held for the chairperson of the...Ch. 12.1 - Prob. 3TTOCh. 12.1 - Prob. 4TTOCh. 12.1 - Prob. 1ECh. 12.1 - Prob. 2ECh. 12.1 - Prob. 3ECh. 12.1 - Explain the head-to head comparison criterion.Ch. 12.1 - 5. What is a fairness criterion for an election?
Ch. 12.1 - Prob. 6E
Ch. 12.1 - Prob. 7ECh. 12.1 - Prob. 8ECh. 12.1 - Prob. 9ECh. 12.1 - Prob. 10ECh. 12.1 - Prob. 11ECh. 12.1 - Prob. 12ECh. 12.1 - Prob. 13ECh. 12.1 - Prob. 14ECh. 12.1 - Prob. 15ECh. 12.1 - For Exercises 15–18, rewrite the preference table...Ch. 12.1 - Prob. 17ECh. 12.1 - Prob. 18ECh. 12.1 - Using the election results given in Exercise 9,...Ch. 12.1 - 20. Using the election results given in Exercise...Ch. 12.1 - Prob. 21ECh. 12.1 - Prob. 22ECh. 12.1 - Using the Internet as a resource, look up the...Ch. 12.1 - Suppose that an election has seven candidates, and...Ch. 12.1 - Prob. 25ECh. 12.1 - Prob. 26ECh. 12.1 - Prob. 27ECh. 12.1 - Prob. 28ECh. 12.1 - Prob. 29ECh. 12.1 - Prob. 30ECh. 12.1 - Prob. 31ECh. 12.1 - Prob. 32ECh. 12.2 - Prob. 1TTOCh. 12.2 - Prob. 2TTOCh. 12.2 - Prob. 3TTOCh. 12.2 - If the one voter who listed softball last in the...Ch. 12.2 - Prob. 1ECh. 12.2 - Prob. 2ECh. 12.2 - Prob. 3ECh. 12.2 - Prob. 4ECh. 12.2 - Prob. 5ECh. 12.2 - Can a candidate that gets the least first-place...Ch. 12.2 - A gaming club holds a vote to decide what type of...Ch. 12.2 - The McKees Point Yacht Club Board of Directors...Ch. 12.2 - Prob. 9ECh. 12.2 - A local police union is holding an election for a...Ch. 12.2 - Students at a college were asked to rank three...Ch. 12.2 - Prob. 12ECh. 12.2 - Prob. 13ECh. 12.2 - Prob. 14ECh. 12.2 - Prob. 15ECh. 12.2 - Prob. 16ECh. 12.2 - Prob. 17ECh. 12.2 - Does the election in Exercise 12 violate the...Ch. 12.2 - An English department is voting for a new...Ch. 12.2 - The Association of Self-Employed Working Persons...Ch. 12.2 - Prob. 21ECh. 12.2 - Prob. 22ECh. 12.2 - Suppose that all 4 voters from the last column of...Ch. 12.2 - Suppose that 2 of the 4 voters from the second...Ch. 12.2 - If 2 of the voters from column 1 in Exercise 21...Ch. 12.2 - If the 3 voters in column 4 in Exercise 22 change...Ch. 12.2 - Construct a preference table for an election...Ch. 12.2 - Construct a preference table for an election...Ch. 12.2 - Construct a preference table for an election so...Ch. 12.2 - If the candidates on a preference ballot are...Ch. 12.2 - If the candidates on a preference ballot are...Ch. 12.2 - In an election with four candidates, how many...Ch. 12.2 - Based on your answers to Exercise 32, explain why...Ch. 12.2 - One way to avoid the issue described in Exercises...Ch. 12.2 - Lets talk about a modified Borda count method....Ch. 12.3 - Prob. 1TTOCh. 12.3 - Prob. 2TTOCh. 12.3 - Does the election in Try This One 2 violate the...Ch. 12.3 - Prob. 4TTOCh. 12.3 - Explain how to determine the winner of an election...Ch. 12.3 - Prob. 2ECh. 12.3 - Describe Arrows impossibility theorem. How is it...Ch. 12.3 - Prob. 4ECh. 12.3 - Prob. 5ECh. 12.3 - Which of the five voting methods we studied do you...Ch. 12.3 - If all of the voters in an approval voting...Ch. 12.3 - Fill in the table below, which summarizes our five...Ch. 12.3 - Prob. 9ECh. 12.3 - Prob. 10ECh. 12.3 - Prob. 11ECh. 12.3 - Prob. 12ECh. 12.3 - Prob. 13ECh. 12.3 - Prob. 14ECh. 12.3 - Prob. 15ECh. 12.3 - Prob. 16ECh. 12.3 - The McKees Point Yacht Club Board of Directors...Ch. 12.3 - The students in Dr. Lees math class are asked to...Ch. 12.3 - If Professor Donovan was unable to serve as...Ch. 12.3 - If the travel company from Exercise 14 loses its...Ch. 12.3 - If the West Oak Golf Club is unavailable and the...Ch. 12.3 - If a room for Dr. Lees final exam was not...Ch. 12.3 - A sports committee of students needs to choose a...Ch. 12.3 - Prob. 24ECh. 12.3 - Prob. 25ECh. 12.3 - Prob. 26ECh. 12.3 - Prob. 27ECh. 12.3 - Prob. 28ECh. 12.3 - Prob. 29ECh. 12.3 - Construct a preference table so that one candidate...Ch. 12.3 - Prob. 31ECh. 12.3 - Prob. 32ECh. 12.3 - Prob. 33ECh. 12.3 - Evaluate each voting method we studied if there...Ch. 12.3 - Suppose that in an election for city council,...Ch. 12.3 - Prob. 36ECh. 12.3 - Devise a method for breaking ties when using...Ch. 12.3 - Prob. 38ECh. 12.4 - Prob. 1TTOCh. 12.4 - Prob. 2TTOCh. 12.4 - Prob. 3TTOCh. 12.4 - Prob. 4TTOCh. 12.4 - Prob. 5TTOCh. 12.4 - Assign the 30 seats from Try This One 5 using...Ch. 12.4 - Prob. 7TTOCh. 12.4 - Prob. 8TTOCh. 12.4 - Prob. 1ECh. 12.4 - Prob. 2ECh. 12.4 - Prob. 3ECh. 12.4 - Describe how to find the upper and lower quotas...Ch. 12.4 - Prob. 5ECh. 12.4 - Prob. 6ECh. 12.4 - Prob. 7ECh. 12.4 - Prob. 8ECh. 12.4 - Prob. 9ECh. 12.4 - Prob. 10ECh. 12.4 - In Exercises 912, find the standard divisor for...Ch. 12.4 - Prob. 12ECh. 12.4 - Prob. 13ECh. 12.4 - Prob. 14ECh. 12.4 - Prob. 15ECh. 12.4 - Prob. 16ECh. 12.4 - Prob. 17ECh. 12.4 - Prob. 18ECh. 12.4 - Prob. 19ECh. 12.4 - Prob. 20ECh. 12.4 - Prob. 21ECh. 12.4 - Prob. 22ECh. 12.4 - Prob. 23ECh. 12.4 - Prob. 24ECh. 12.4 - Prob. 25ECh. 12.4 - For Exercises 2628 find: (a)The standard divisor....Ch. 12.4 - Prob. 27ECh. 12.4 - Prob. 28ECh. 12.4 - Prob. 29ECh. 12.4 - Prob. 30ECh. 12.4 - Prob. 31ECh. 12.4 - Prob. 32ECh. 12.4 - Prob. 33ECh. 12.4 - Prob. 34ECh. 12.4 - Prob. 35ECh. 12.4 - Prob. 36ECh. 12.4 - Prob. 37ECh. 12.4 - Prob. 38ECh. 12.4 - Prob. 39ECh. 12.4 - Prob. 40ECh. 12.4 - Prob. 41ECh. 12.4 - Prob. 42ECh. 12.4 - Prob. 43ECh. 12.5 - Prob. 1TTOCh. 12.5 - Try This One 2
A county with three districts has...Ch. 12.5 - Prob. 3TTOCh. 12.5 - Prob. 1ECh. 12.5 - Prob. 2ECh. 12.5 - Prob. 3ECh. 12.5 - Prob. 4ECh. 12.5 - What is the quota rule? Which apportionment...Ch. 12.5 - Prob. 6ECh. 12.5 - Prob. 7ECh. 12.5 - Prob. 8ECh. 12.5 - Prob. 9ECh. 12.5 - Prob. 10ECh. 12.5 - Prob. 11ECh. 12.5 - Prob. 12ECh. 12.5 - The table shows the enrollment at two campuses of...Ch. 12.5 - Prob. 14ECh. 12.5 - Prob. 15ECh. 12.5 - Prob. 16ECh. 12.5 - Write an essay explaining why many people feel...Ch. 12.5 - 18. Which do you think is more serious: violating...Ch. 12 - Use this information for Exercises 14: the...Ch. 12 - Use this information for Exercises 14: the...Ch. 12 - Use this information for Exercises 14: the...Ch. 12 - Prob. 4RECh. 12 - Prob. 5RECh. 12 - Prob. 6RECh. 12 - Prob. 7RECh. 12 - Prob. 8RECh. 12 - Prob. 9RECh. 12 - Prob. 10RECh. 12 - Prob. 11RECh. 12 - Prob. 12RECh. 12 - Prob. 13RECh. 12 - Prob. 14RECh. 12 - Use this information for Exercises 917: a large...Ch. 12 - Prob. 16RECh. 12 - Use this information for Exercises 917: a large...Ch. 12 - Prob. 18RECh. 12 - Prob. 19RECh. 12 - Prob. 20RECh. 12 - Prob. 21RECh. 12 - Prob. 22RECh. 12 - Prob. 23RECh. 12 - Prob. 24RECh. 12 - Prob. 25RECh. 12 - Prob. 26RECh. 12 - Prob. 27RECh. 12 - Prob. 28RECh. 12 - Prob. 29RECh. 12 - A community college bought 15 laptop computers to...Ch. 12 - Prob. 31RECh. 12 - Prob. 32RECh. 12 - Prob. 33RECh. 12 - Prob. 34RECh. 12 - Prob. 35RECh. 12 - Repeat exercise 30 using the Huntington-Hill...Ch. 12 - Prob. 37RECh. 12 - Prob. 38RECh. 12 - Prob. 39RECh. 12 - Prob. 40RECh. 12 - Prob. 41RECh. 12 - Prob. 42RECh. 12 - Prob. 43RECh. 12 - Prob. 1CTCh. 12 - Prob. 2CTCh. 12 - Prob. 3CTCh. 12 - Prob. 4CTCh. 12 - Prob. 5CTCh. 12 - Prob. 6CTCh. 12 - Prob. 7CTCh. 12 - Prob. 8CTCh. 12 - Use this information for Exercises 512: a small...Ch. 12 - Prob. 10CTCh. 12 - Prob. 11CTCh. 12 - Prob. 12CTCh. 12 - Prob. 13CTCh. 12 - An airline offers nonstop flights from Fort...Ch. 12 - Prob. 15CTCh. 12 - Repeat Problem 14 using Websters method.Ch. 12 - Repeat Problem 14 using the Huntington-Hill...Ch. 12 - Prob. 18CTCh. 12 - Prob. 19CTCh. 12 - Prob. 20CTCh. 12 - Prob. 21CT
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- The managers of Prints Alive (a local silk-screening business) are planning a party for their 30 employees. There are three possible locations for the party: the warehouse (W), the park (P), or the beach (B). The employees are asked to rank these choices in order of preference, and the results are summarized in the table. Use the instant runoff method to determine the winner. the parkthe beach the warehouse Number of Ballots Cast 2 12 13 3 1st choice P P B W 2nd choice B W W B 3rd choice W B P Parrow_forwardNeed help with the first three questions, and please explain step by step how to do it the easiest way possible.arrow_forwardUse the Condorcet method to determine the winner of the election. Four students are running for president of the school: • Ariana (A), • Brett (B), • Carlos (C), and • DeeDee (D). The 5 clubs and their members were asked to rank all candidates. Number of Voters Preference Order 1st to last 19 A C D 15 В D A C 11 D A C В 7 D В A D A В a A b В C d No Condorcet winner O O O Oarrow_forward
- Mr. Abdul Rheman's class held an election for class president. Instead of just voting for one candidate, students need to rank all the candidates according to their preference. The results are shown below: Rankings X 3 2 1 2 1 Y 1 1 1 3 3 1 2 2 Number of 7 8 6 Students Winner by Plurality.arrow_forwardAn election is held to choose the chair of a department at a university. The candidates are Professors Argand, Brandt, Chavez, Dietz, and Epstein (A, B, C, D, and E for short). The following table gives the preference schedule for the election. Use the table to complete parts (a) through (c). Number of Voters 4 3 6. 3 4 1st choice B B A A 2nd choice A C D A D 3rd choice 4th choice D A D B. B. E B B 5th choice E A D E (a) How many people voted in this election? voters (Type a whole number.) (b) How many first-place votes are needed for a majority? The smallest number for a majority is votes. (e) Which candidate had the fewest last-place votes? B. Aarrow_forwardA Senior High School class held an election for class president. Instead of just voting for one candidate, the students needed to rank all the candidates according to preference. The table presents the results. RANKINGS Rey 2 3 1 3 4 2 Allyson 4 1 3 4 1 3 Michelle 1 2 2 2 3 4 Carlo 3 4 4 1 2 1 Number of Votes 36 53 41 27 31 45 1.What is Total Number of Votes 2. what is the ajority number of Votes 3. who is the winner by Plurality 4. who is the winner by Borda Count Methodarrow_forward
- Mr. Abdul Rheman's class held an election for class president. Instead of just voting for one candidate, students need to rank all the candidates according to their preference. The results are shown below: Rankings 2 1 2 3 1 Y 2 1 2 3 1 3 1 3 1 2 2 Number of 8 7 7 8 6. 7 Students Number of Votes for the Winner by Plurality.arrow_forwardDetermines who wins the plurality vote and who wins the rank voting or preferential voting method in the election. 1. There are 650 students in a school, and they all get to vote for the school president. There are 3 candidates: Rios, • Millie, • Charles. Each student will receive a ballot and they will rank the candidates in or of 1", 2nd, and 3rd choice. Remember on the preferential vote the winning candidate must have 1 more than half the votes. See the results of the election below. Votes 225 175 250 225 1¹ Millie Charles Rios 250 and a. The person who has the largest number of first place votes is b. The person who has the fewest number of first place votes is c. The candidate we eliminate is d. Fill in the new table. 1 Charles Millie Millie Rios Rios Charles 2rd Is there a majority of votes for a candidate now? If yes, who is that candidate, and how many the first place votes does that candidate have? f. Who won the plurality vote or single candidate voting method? Explain how…arrow_forwardEmployees at the Me, My Mop and I cleaning service are choosing a new company logo. The choices are a mop (M), a broom and dustpan (B), and a vacuum cleaner (V). Each employee ranks the three choices from first to third. The preference table below shows the results of the ballots. Number of Votes First Second Third 20 3 M V V M B B 17 8 10 19 V M B B B M M M V V B V Determine the winner using the plurality method. O A. broom and dustpan B. mop O C. vacuumarrow_forward
- I'm attaching the questions I need help with, I attempted and got them wrong but I did the other questions.arrow_forwardEmployees at the Me, My Mop and I cleaning service are choosing a new company logo. The choices are a mop (M), a broom and dustpan (B), and a vacuum cleaner (V). Each employee ranks the three choices from first to third. The preference table below shows the results of the ballots. Number of Votes 15 11 10 14 7 20 First M V V B В Second V В V M Third B B M M V V Determine the winner using the plurality method. O A. mop O B. vacuum C. broom and dustpanarrow_forwardThe Science club is selecting its representative for a competition. The members rank the 4 candidates given in the preference table below: Votes 2 1 8 4 1st Berlin Anton Dianne Charles 2nd Charles Berlin Charles Berlin 3rd Dianne Charles Anton Dianne 4th Anton Dianne Berlin Anton Who is the Borda count winner? Group of answer choices Dianne Anton Berlin Charlesarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
12. Searching and Sorting; Author: MIT OpenCourseWare;https://www.youtube.com/watch?v=6LOwPhPDwVc;License: Standard YouTube License, CC-BY
Algorithms and Data Structures - Full Course for Beginners from Treehouse; Author: freeCodeCamp.org;https://www.youtube.com/watch?v=8hly31xKli0;License: Standard Youtube License