
Concept explainers
a.
Find the missing quantities.
a.
Answer to Problem 106SE
The filled
| Predictor | Coefficient | SE Coefficient | T | P | |||
| Constant | 517.46 | 11.76 | 44.0017 | 0 | |||
| 11.4720 | 0.314 | 36.50 | 0 | ||||
| –8.1378 | 0.1969 | –41.3296 | 0 | ||||
| 10.8565 | 0.6652 | 16.3207 | 0 | ||||
| Analysis of Variance | |||||||
| Source | DF | SS | MS | F | P | ||
| Regression | 3 | 347,300 | 115,767 | 1,102.543 | 0 | ||
| Residual Error | 16 | 1,683 | 105 | ||||
| Total | 19 | 348,983 | |||||
Explanation of Solution
Calculation:
An incomplete regression analysis table is given.
Multiple linear regression model:
A multiple linear regression model is given as
The t-statistic is obtained as,
The test statistic
In the given problem the regression equation is
Hence, there are 3 regressors and
Now, using the given information it can be found that,
| Predictor | Coefficient | SE Coefficient | |
| Constant | 517.46 | 11.76 | |
| 11.4720 | 36.50 | ||
| –8.1378 | 0.1969 | ||
| 10.8565 | 0.6652 |
The total observation is 19. Thus,
In the given problem, the degrees of freedom for t-statistic is,
P- value:
Software procedure:
Step by step procedure to obtain the P- value using the MINITAB software is given below:
- Choose Graph > Probability Distribution Plot choose View Probability > OK.
- From Distribution, choose ‘t’ distribution.
- Enter Degree of freedom as 15.
- Click the Shaded Area tab.
- Choose X value and Right tail for the region of the curve to shade.
- Enter the X value as 44.0017.
- Click OK.
Output using the MINITAB software is given below:
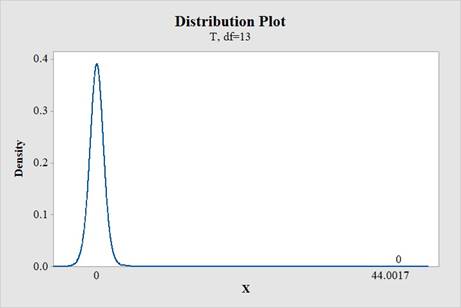
P- value:
Software procedure:
Step by step procedure to obtain the P- value using the MINITAB software is given below:
- Choose Graph > Probability Distribution Plot choose View Probability > OK.
- From Distribution, choose ‘t’ distribution.
- Enter Degree of freedom as 15.
- Click the Shaded Area tab.
- Choose X value and Right tail for the region of the curve to shade.
- Enter the X value as 36.50.
- Click OK.
Output using the MINITAB software is given below:
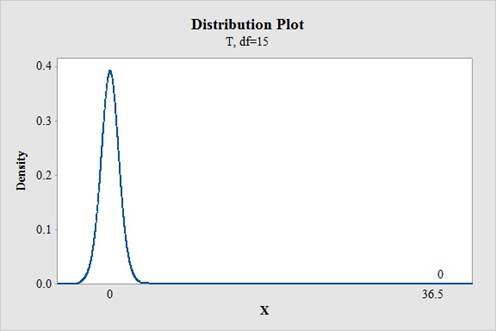
P- value:
Software procedure:
Step by step procedure to obtain the P- value using the MINITAB software is given below:
- Choose Graph > Probability Distribution Plot choose View Probability > OK.
- From Distribution, choose ‘t’ distribution.
- Enter Degree of freedom as 15.
- Click the Shaded Area tab.
- Choose X value and Left tail for the region of the curve to shade.
- Enter the X value as –41.3296.
- Click OK.
Output using the MINITAB software is given below:
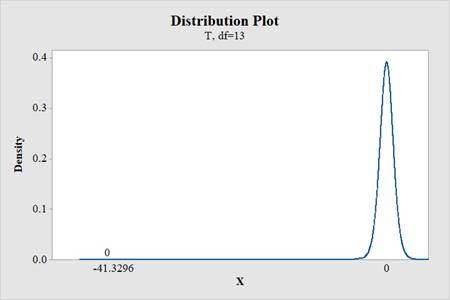
P- value:
Software procedure:
Step by step procedure to obtain the P- value using the MINITAB software is given below:
- Choose Graph > Probability Distribution Plot choose View Probability > OK.
- From Distribution, choose ‘t’ distribution.
- Enter Degree of freedom as 15.
- Click the Shaded Area tab.
- Choose X value and Right tail for the region of the curve to shade.
- Enter the X value as 16.3207.
- Click OK.
Output using the MINITAB software is given below:
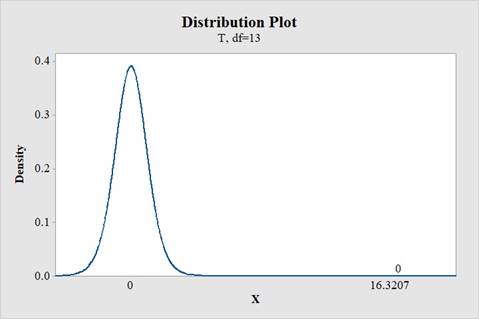
Hence, the corresponding P-values are,
| Predictor | P-value | |
| Constant | 44.0017 | 0 |
| 36.50 | 0 | |
| –41.3296 | 0 | |
| 16.3207 | 0 |
The F-statistic is obtained as,
The F-statistic follows F distribution with numerator degrees of freedom k and denominator degrees of freedom
It is also known that,
Now, it is given that
Hence,
Coefficient of multiple determination
The coefficient of multiple determination,
Thus,
Hence,
Adjusted coefficient of multiple determination
The adjusted coefficient of multiple determination,
Thus,
Hence,
Now, the regression degrees of freedom is
Hence,
Hence,
The enumerator degrees of freedom of F is 3 and the denominator degrees of freedom is 16.
P- value:
Software procedure:
Step by step procedure to obtain the P- value using the MINITAB software is given below:
- Choose Graph > Probability Distribution Plot choose View Probability > OK.
- From Distribution, choose ‘F’ distribution.
- Enter Numerator degree of freedom as 3.
- Enter Denominator degree of freedom as 16.
- Click the Shaded Area tab.
- Choose X value and Right tail for the region of the curve to shade.
- Enter the X value as 1,102.543.
- Click OK.
Output using the MINITAB software is given below:
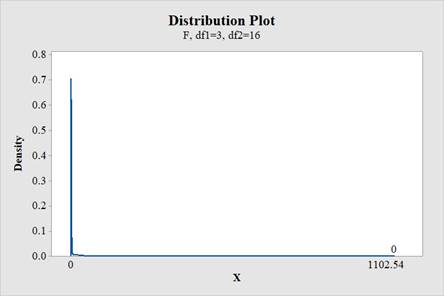
Hence, the filled regression analysis table is,
| Predictor | Coefficient | SE Coefficient | T | P | |||
| Constant | 517.46 | 11.76 | 44.0017 | 0 | |||
| 11.4720 | 0.314 | 36.50 | 0 | ||||
| –8.1378 | 0.1969 | –41.3296 | 0 | ||||
| 10.8565 | 0.6652 | 16.3207 | 0 | ||||
| Analysis of Variance | |||||||
| Source | DF | SS | MS | F | P | ||
| Regression | 3 | 347,300 | 115,767 | 1,102.543 | 0 | ||
| Residual Error | 16 | 1,683 | 105 | ||||
| Total | 19 | 348,983 | |||||
b.
Explain whether the model is significant at
Also explain whether the model is significant at
b.
Answer to Problem 106SE
The test for the statistical significance of the regression at level of significance
Explanation of Solution
Calculation:
Multiple regression model:
The regression model of the response variable y on k regressor variables,
Here, there are 3 regressors.
The model would be of the form:
The parameter of the interest:
The parameters of the interest are the slope coefficients
For level of significance
Hypotheses:
Null hypothesis:
That is, there is no statistical significance of the regression.
Alternative hypothesis:
That is, there is statistical significance of the regression.
Level of significance:
Assume that, the level of significance is
P-value:
From part (a), it is found that the P-value for the F-test of the regression is
Decision rule:
If
If,
Conclusion:
Here, the P-value is less than the level of significance.
That is,
By rejection rule, reject the null hypothesis.
Hence, there is sufficient evidence of statistical significance of the regression.
For level of significance
Hypotheses:
Null hypothesis:
That is, there is no statistical significance of the regression.
Alternative hypothesis:
That is, there is statistical significance of the regression.
Level of significance:
Assume that, the level of significance is
P-value:
From part (a), it is found that the P-value for the F-test of the regression is
Decision rule:
If
If,
Conclusion:
Here, the P-value is less than the level of significance.
That is,
By rejection rule, reject the null hypothesis.
Hence, there is sufficient evidence of statistical significance of the regression.
Conclusion:
Hence, there are enough evidence that there is statistical significance of at least one of the regressors for any of the given values
c.
Explain about the contribution of the individual regressors to the model.
c.
Answer to Problem 106SE
The tests for the statistical significance of the regressors at level of significance
Explanation of Solution
Calculation:
Test for the statistical significance of
The parameter of the interest:
The parameter of the interest is the slope coefficient
Hypotheses:
Null hypothesis:
That is, there is no statistical significance of the coefficient of
Alternative hypothesis:
That is, there is statistical significance of the coefficient of
Level of significance:
Assume that level of significance is
P-value:
From part (a), the P-value for the t-test of the coefficient of
Decision rule:
If
If,
Conclusion:
Here, the P-value is less than the level of significance.
That is,
By rejection rule, reject the null hypothesis.
Hence, there is sufficient evidence of statistical significance of the coefficient of
Test for the statistical significance of
The parameter of the interest:
The parameter of the interest is the slope coefficient
Hypotheses:
Null hypothesis:
That is, there is no statistical significance of
Alternative hypothesis:
That is, there is statistical significance of the coefficient of
Level of significance:
Assume that, the level of significance is
P-value:
From part (a), the P-value for the t-test of the coefficient of
Decision rule:
If
If,
Conclusion:
Here, the P-value is less than the level of significance.
That is,
By rejection rule, reject the null hypothesis.
Hence, there is sufficient evidence of statistical significance of the coefficient of
Test for the statistical significance of
The parameter of the interest:
The parameter of the interest is the slope coefficient
Hypotheses:
Null hypothesis:
That is, there is no statistical significance of
Alternative hypothesis:
That is, there is statistical significance of the coefficient of
Level of significance:
Assume that, the level of significance is
P-value:
From part (a), the P-value for the t-test of the coefficient of
Decision rule:
If
If,
Conclusion:
Here, the P-value is less than the level of significance.
That is,
By rejection rule, reject the null hypothesis.
Hence, there is sufficient evidence of statistical significance of the coefficient of
Want to see more full solutions like this?
Chapter 12 Solutions
Applied Statistics and Probability for Engineers
- To help consumers in purchasing a laptop computer, Consumer Reports calculates an overall test score for each computer tested based upon rating factors such as ergonomics, portability, performance, display, and battery life. Higher overall scores indicate better test results. The following data show the average retail price and the overall score for ten 13-inch models (Consumer Reports website, October 25, 2012). Brand & Model Price ($) Overall Score Samsung Ultrabook NP900X3C-A01US 1250 83 Apple MacBook Air MC965LL/A 1300 83 Apple MacBook Air MD231LL/A 1200 82 HP ENVY 13-2050nr Spectre XT 950 79 Sony VAIO SVS13112FXB 800 77 Acer Aspire S5-391-9880 Ultrabook 1200 74 Apple MacBook Pro MD101LL/A 1200 74 Apple MacBook Pro MD313LL/A 1000 73 Dell Inspiron I13Z-6591SLV 700 67 Samsung NP535U3C-A01US 600 63 a. Select a scatter diagram with price as the independent variable. b. What does the scatter diagram developed in part (a) indicate about the relationship…arrow_forwardTo the Internal Revenue Service, the reasonableness of total itemized deductions depends on the taxpayer’s adjusted gross income. Large deductions, which include charity and medical deductions, are more reasonable for taxpayers with large adjusted gross incomes. If a taxpayer claims larger than average itemized deductions for a given level of income, the chances of an IRS audit are increased. Data (in thousands of dollars) on adjusted gross income and the average or reasonable amount of itemized deductions follow. Adjusted Gross Income ($1000s) Reasonable Amount ofItemized Deductions ($1000s) 22 9.6 27 9.6 32 10.1 48 11.1 65 13.5 85 17.7 120 25.5 Compute b1 and b0 (to 4 decimals).b1 b0 Complete the estimated regression equation (to 2 decimals). = + x Predict a reasonable level of total itemized deductions for a taxpayer with an adjusted gross income of $52.5 thousand (to 2 decimals). thousand dollarsWhat is the value, in dollars, of…arrow_forwardK The mean height of women in a country (ages 20-29) is 63.7 inches. A random sample of 65 women in this age group is selected. What is the probability that the mean height for the sample is greater than 64 inches? Assume σ = 2.68. The probability that the mean height for the sample is greater than 64 inches is (Round to four decimal places as needed.)arrow_forward
- In a survey of a group of men, the heights in the 20-29 age group were normally distributed, with a mean of 69.6 inches and a standard deviation of 4.0 inches. A study participant is randomly selected. Complete parts (a) through (d) below. (a) Find the probability that a study participant has a height that is less than 68 inches. The probability that the study participant selected at random is less than 68 inches tall is 0.4. (Round to four decimal places as needed.) 20 2arrow_forwardPEER REPLY 1: Choose a classmate's Main Post and review their decision making process. 1. Choose a risk level for each of the states of nature (assign a probability value to each). 2. Explain why each risk level is chosen. 3. Which alternative do you believe would be the best based on the maximum EMV? 4. Do you feel determining the expected value with perfect information (EVWPI) is worthwhile in this situation? Why or why not?arrow_forwardQuestions An insurance company's cumulative incurred claims for the last 5 accident years are given in the following table: Development Year Accident Year 0 2018 1 2 3 4 245 267 274 289 292 2019 255 276 288 294 2020 265 283 292 2021 263 278 2022 271 It can be assumed that claims are fully run off after 4 years. The premiums received for each year are: Accident Year Premium 2018 306 2019 312 2020 318 2021 326 2022 330 You do not need to make any allowance for inflation. 1. (a) Calculate the reserve at the end of 2022 using the basic chain ladder method. (b) Calculate the reserve at the end of 2022 using the Bornhuetter-Ferguson method. 2. Comment on the differences in the reserves produced by the methods in Part 1.arrow_forward
- You are provided with data that includes all 50 states of the United States. Your task is to draw a sample of: o 20 States using Random Sampling (2 points: 1 for random number generation; 1 for random sample) o 10 States using Systematic Sampling (4 points: 1 for random numbers generation; 1 for random sample different from the previous answer; 1 for correct K value calculation table; 1 for correct sample drawn by using systematic sampling) (For systematic sampling, do not use the original data directly. Instead, first randomize the data, and then use the randomized dataset to draw your sample. Furthermore, do not use the random list previously generated, instead, generate a new random sample for this part. For more details, please see the snapshot provided at the end.) Upload a Microsoft Excel file with two separate sheets. One sheet provides random sampling while the other provides systematic sampling. Excel snapshots that can help you in organizing columns are provided on the next…arrow_forwardThe population mean and standard deviation are given below. Find the required probability and determine whether the given sample mean would be considered unusual. For a sample of n = 65, find the probability of a sample mean being greater than 225 if μ = 224 and σ = 3.5. For a sample of n = 65, the probability of a sample mean being greater than 225 if μ=224 and σ = 3.5 is 0.0102 (Round to four decimal places as needed.)arrow_forward***Please do not just simply copy and paste the other solution for this problem posted on bartleby as that solution does not have all of the parts completed for this problem. Please answer this I will leave a like on the problem. The data needed to answer this question is given in the following link (file is on view only so if you would like to make a copy to make it easier for yourself feel free to do so) https://docs.google.com/spreadsheets/d/1aV5rsxdNjHnkeTkm5VqHzBXZgW-Ptbs3vqwk0SYiQPo/edit?usp=sharingarrow_forward
- The data needed to answer this question is given in the following link (file is on view only so if you would like to make a copy to make it easier for yourself feel free to do so) https://docs.google.com/spreadsheets/d/1aV5rsxdNjHnkeTkm5VqHzBXZgW-Ptbs3vqwk0SYiQPo/edit?usp=sharingarrow_forwardThe following relates to Problems 4 and 5. Christchurch, New Zealand experienced a major earthquake on February 22, 2011. It destroyed 100,000 homes. Data were collected on a sample of 300 damaged homes. These data are saved in the file called CIEG315 Homework 4 data.xlsx, which is available on Canvas under Files. A subset of the data is shown in the accompanying table. Two of the variables are qualitative in nature: Wall construction and roof construction. Two of the variables are quantitative: (1) Peak ground acceleration (PGA), a measure of the intensity of ground shaking that the home experienced in the earthquake (in units of acceleration of gravity, g); (2) Damage, which indicates the amount of damage experienced in the earthquake in New Zealand dollars; and (3) Building value, the pre-earthquake value of the home in New Zealand dollars. PGA (g) Damage (NZ$) Building Value (NZ$) Wall Construction Roof Construction Property ID 1 0.645 2 0.101 141,416 2,826 253,000 B 305,000 B T 3…arrow_forwardRose Par posted Apr 5, 2025 9:01 PM Subscribe To: Store Owner From: Rose Par, Manager Subject: Decision About Selling Custom Flower Bouquets Date: April 5, 2025 Our shop, which prides itself on selling handmade gifts and cultural items, has recently received inquiries from customers about the availability of fresh flower bouquets for special occasions. This has prompted me to consider whether we should introduce custom flower bouquets in our shop. We need to decide whether to start offering this new product. There are three options: provide a complete selection of custom bouquets for events like birthdays and anniversaries, start small with just a few ready-made flower arrangements, or do not add flowers. There are also three possible outcomes. First, we might see high demand, and the bouquets could sell quickly. Second, we might have medium demand, with a few sold each week. Third, there might be low demand, and the flowers may not sell well, possibly going to waste. These outcomes…arrow_forward
 MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman





