Problem 1SP: Refer to the graph of f ( x ) = x 2 + k to determine the value k . Problem 2SP: Graph the functions f , g , and h on the same coordinate system. f ( x ) = x 2 g ( x ) = x 2 +... Problem 3SP: Refer to the graph of f ( x ) = ( x − h ) 2 to determine the value of h . Problem 4SP: Graph the functions f , g , and h on the same coordinate system. f ( x ) = x 2 g ( x ) = ( x + 3... Problem 5SP: 5. Graph the functions on the same coordinate system.
Problem 6SP: 6. Graph the functions on the same coordinate system.
Problem 7SP: Given the function defined by g ( x ) = 3 ( x + 1 ) 2 − 3 a. Identify the vertex. b. Sketch the... Problem 8SP: Given the function defined by h ( x ) = − 1 2 ( x − 4 ) 2 + 2 a. Identify the vertex. b. Sketch the... Problem 1PE: a. The graph of a quadratic function, f ( x ) = a x 2 + b x + c = 0 , is a ___________. b. The... Problem 2PE: For Exercises 2–8, solve the equations.
2.
Problem 3PE: For Exercises 2–8, solve the equations.
3.
Problem 4PE: For Exercises 2–8, solve the equations. 2 a + 2 = a + 1 Problem 5PE: For Exercises 2–8, solve the equations. 5 t ( t − 2 ) = − 3 Problem 6PE: For Exercises 2–8, solve the equations.
6.
Problem 7PE: For Exercises 2–8, solve the equations.
7.
Problem 8PE: For Exercises 2–8, solve the equations.
8.
Problem 9PE: Describe how the value of k affects the graph of a function defined by f ( x ) = k 2 + k Problem 10PE: For Exercises 10–17, graph the functions. (See Examples 1–2.)
10.
Problem 11PE: For Exercises 10–17, graph the functions. (See Examples 1–2.)
11.
Problem 12PE: For Exercises 10–17, graph the functions. (See Examples 1–2.) p ( x ) = x 2 − 3 Problem 13PE: For Exercises 10–17, graph the functions. (See Examples 1–2.) q ( x ) = x 2 − 4 Problem 14PE: For Exercises 10–17, graph the functions. (See Examples 1–2.)
14.
Problem 15PE: For Exercises 10–17, graph the functions. (See Examples 1–2.) S ( x ) = x 2 + 3 2 Problem 16PE: For Exercises 10–17, graph the functions. (See Examples 1–2.) M ( x ) = x 2 − 5 4 Problem 17PE: For Exercises 10–17, graph the functions. (See Examples 1–2.) n ( x ) = x 2 − 1 3 Problem 18PE: Describe how the value of h affects the graph of a function defined by f ( x ) = ( x − h ) 2 . Problem 19PE: For Exercises 19–26, graph the functions. (See Examples 3–4.) r ( x ) = ( x + 1 ) 2 Problem 20PE: For Exercises 19–26, graph the functions. (See Examples 3–4.)
20.
Problem 21PE: For Exercises 19–26, graph the functions. (See Examples 3–4.)
21.
Problem 22PE: For Exercises 19–26, graph the functions. (See Examples 3–4.) L ( x ) = ( x − 4 ) 2 Problem 23PE: For Exercises 19–26, graph the functions. (See Examples 3–4.) A ( x ) ( x + 3 4 ) 2 Problem 24PE: For Exercises 19–26, graph the functions. (See Examples 3–4.)
24.
Problem 25PE: For Exercises 19–26, graph the functions. (See Examples 3–4.) W ( x ) = ( x − 1.25 ) 2 Problem 26PE: For Exercises 19–26, graph the functions. (See Examples 3–4.) V ( x ) = ( x − 2.5 ) 2 Problem 27PE: Describe how the value of a affects the graph of a function defined by f ( x ) = a x 2 , where a ≠ 0... Problem 28PE: 28. How do you determine whether the graph of a function defined by opens upward or downward?
Problem 29PE: For Exercises 29–36, graph the functions. (See Examples 5–6.)
29.
Problem 30PE: For Exercises 29–36, graph the functions. (See Examples 5–6.) g ( x ) = 3 x 2 Problem 31PE: For Exercises 29–36, graph the functions. (See Examples 5–6.) h ( x ) = 1 4 x 2 Problem 32PE: For Exercises 29–36, graph the functions. (See Examples 5–6.) f ( x ) = 1 5 x 2 Problem 33PE: For Exercises 29–36, graph the functions. (See Examples 5–6.) c ( x ) = − x 2 Problem 34PE: For Exercises 29–36, graph the functions. (See Examples 5–6.) g ( x ) = − 4 x 2 Problem 35PE: For Exercises 29–36, graph the functions. (See Examples 5–6.) v ( x ) = − 1 5 x 2 Problem 36PE: For Exercises 29–36, graph the functions. (See Examples 5–6.) f ( x ) = − 1 4 x 2 Problem 37PE: For Exercises 37–44, match the function with its graph. f ( x ) = − 1 4 x 2 Problem 38PE: For Exercises 37–44, match the function with its graph.
38.
Problem 39PE: For Exercises 37–44, match the function with its graph. k ( x ) = ( x − 3 ) 2 Problem 40PE: For Exercises 37–44, match the function with its graph. h ( x ) = 1 4 x 2 Problem 41PE: For Exercises 37–44, match the function with its graph. t ( x ) x 2 + 2 Problem 42PE: For Exercises 37–44, match the function with its graph.
42.
Problem 43PE: For Exercises 37–44, match the function with its graph.
43.
Problem 44PE: For Exercises 37–44, match the function with its graph. n ( x ) = − ( x − 2 ) 2 + 3 Problem 45PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 46PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 47PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 48PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 49PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 50PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 51PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 52PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 53PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 54PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 55PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 56PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 57PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 58PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 59PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 60PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 61PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 62PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 63PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 64PE: For Exercises 45–64, graph the parabola and the axis of symmetry. Label the coordinates of the... Problem 65PE: Compare the graphs of the following equations to the graph of y = x 2 . a. y = x 2 + 3 b. y = ( x +... Problem 66PE: 66. Compare the graphs of the following equations to the graph of.
a.
b.
c.
Problem 67PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 68PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 69PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 70PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 71PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 72PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 73PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 74PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 75PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 76PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 77PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 78PE: For Exercises 67–78, write the coordinates of the vertex and determine if the vertex is a maximum... Problem 79PE: 79. True or false: The function defined by has a maximum value but no minimum value.
Problem 80PE: 80. True or false: The function defined by has a maximum value but no minimum value.
Problem 81PE: 81. True or false: If the vertex represents a minimum point, then the minimum value is −2.
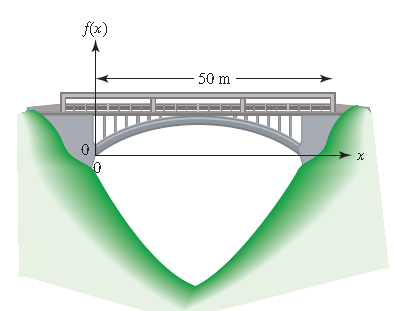
Problem 82PE: True or false: If the vertex ( − 2 , 8 ) represents a maximum point, then the maximum value is 8. Problem 83PE: A suspension bridge is 120 ft long. Its supporting cable hangs in a shapethat resembles a parabola.... Problem 84PE: A 50-m bridge over a crevasse is supported by a parabolic arch. The function defined by... Problem 85PE: The staging platform for a fireworks display is 6 ft above ground, and the mortars leave the... format_list_bulleted



 Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
 College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell




