Chapter 11.3, Problem 71E

a.

To determine

To find: a formula for the instantaneous rate of change of the balloon.

Answer to Problem 71E

  $s\text{'}(t)=-32t+64$ .

Explanation of Solution

Given information: A water balloon is thrown upward from the top of an 80-foot

building with an initial velocity of 64 feet per second. The height or displacement s (in feet) of the balloon can be modeled by the position function $s(t)=-16t^2+64t+80$, where t is the time in second since it was thrown.

Calculation:

  $\begin{array}{l}s(t)=-16t^2+64t+80\\ s\text{'}(t)=-32t+64\end{array}$

b.

To determine

To find: the average rate of change of the balloon after the first three second of flight.

Answer to Problem 71E

  $\text{Average}\text{rate}\text{of}\text{change}=16\text{ft/sec}$

Explanation of Solution

Given information: $s(t)=-16t^2+64t+80$

Calculation:

  $\begin{array}{l}s(0)=80,s(3)=128\\ \text{Average}\text{rate}\text{of}\text{change}=\frac{128-80}{3}=\frac{48}{3}=16\text{ft/sec}\text{.}\end{array}$

c.

To determine

To find: the time at which the balloon reaches its maximum height.

Answer to Problem 71E

The time at which the balloon reaches its maximum height is 2 seconds.

Explanation of Solution

Given information: $s(t)=-16t^2+64t+80$

Calculation:

  $\begin{array}{l}s(t)=-16t^2+64t+80\\ s\text{'}(t)=-32t+64=0\Rightarrow t=2\text{seconds}\end{array}$

d.

To determine

To find: the velocity of the balloon as it impacts the ground.

Answer to Problem 71E

The velocity of the balloon as it impacts the ground is -96 ft/sec.

Explanation of Solution

Given information: $s(t)=-16t^2+64t+80$

Calculation:

  $\begin{array}{l}\text{At}\text{ground}s(t)=0\\ s(t)=-16t^2+64t+80=0\Rightarrow t=5\\ s\text{'}(t)=-32t+64\Rightarrow s\text{'}(5)=-32(5)+64=-96\text{ft/sec}\end{array}$

e.

To determine

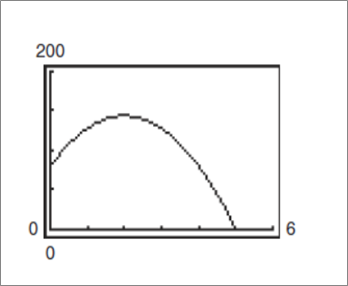
To graph: the model using a graphing utility.

Explanation of Solution

Given information: $s(t)=-16t^2+64t+80$

Calculation:

The graph of the model using the graphing utility is shown below.

  $$