Chapter 11.2, Problem 9P

For Problems 5-14, please provide the following information.

(a) What is the level of significance? State the null and alternate hypotheses.

(b) Find the value of the chi-square statistic for the sample. Are all the expected frequencies greater than 5? What sampling distribution will you use? What are the degrees of freedom?

(c) Find or estimate the P-value of the sample test statistic.

(d) Based on your answers in parts (a)-(c), will you reject or fail to reject the null hypothesis that the population fits the specified distribution of categories?

(e) Interpret your conclusion in the context of the application

Meteorology: Normal Distribution The following problem is based on information from the National Oceanic and Atmospheric Administration (NOAA) Environment Data Service. Let be a random variable that represents the average daily temperature (in degrees Fahrenheit ) in July in the town of Kit Carson, Colorado. The x distribution has a mean $\mu$ of approximately $\text{75°F}$ and standard deviation $\sigma$ of approximately $\text{8°F}$. A 20-year study (620 July days) gave the entries in the rightmost column of the following table.

I	II	III	IV
Region under Normal Curve	$x°\text{F}$	Expected % from Noraml Curve	Observed Number of Days in 20 Years
$\mu -3\sigma \le x<\mu -2\sigma$	$51\le x<59$	2.35%	16
$\mu -2\sigma \le x<\mu -\sigma$	$59\le x<67$	13.5%	78
$\mu -\sigma \le x<\mu$	$67\le x<75$	34%	212
$\mu \le x<\mu +\sigma$	$75\le x<83$	34%	221
$\mu +\sigma \le x<\mu +2\sigma$	$83\le x<91$	13.5%	81
$\mu +2\sigma \le x<\mu +3\sigma$	$91\le x<99$	2.35%	12

(i) Remember that $\mu \text{ = 75 and }\sigma \text{ = 8}$. Examine Figure 7-3 in Chapter 7. Write a brief explanation fur Columns I, II, and III in the context of this problem.

(ii) Use a 19 level of significance to test the claim that the average daily. July temperature follows a normal distribution with $\mu \text{ = 75 and }\sigma \text{= K}\text{.}$

To determine

To explain: The columns I, II and III in the context of this problem.

Explanation of Solution

Let, X represents the average daily temperature (in degrees Fahrenheit), which follows the normal distribution with $\mu =75$ and $\sigma =8$.

The below graph shows normal curve with $\mu =75$ and $\sigma =8$. $$

We can see that, 2.35% of the data values will lie within 2 standard deviation below the mean and 3 standard deviation below the mean, 13.55% of the data values will lie within 1 standard deviation below the mean and 2 standard deviation below the mean, 34.0% of the data values will lie within mean and 1 standard deviation below the mean and mean.

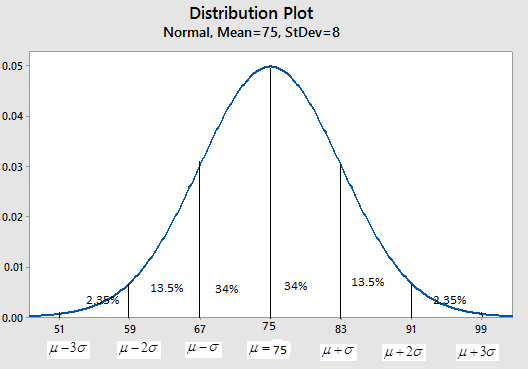
By empirical rule, approximately 68% of the data values lie within 1 standard deviation on each side of the mean, approximately 95% of the data values lies within 2 standard deviations on each side of the mean and approximately 99.7% of the data values lies within 3 standard deviations on each side of the mean.

To determine

The level of significance and state the null and alternative hypotheses.

Answer to Problem 9P

Solution: The level of significance is 0.01. $H_0$: The average daily July temperature follows a normal distributionand $H_1$: The average daily July temperature does not follow a normal distribution.

Explanation of Solution

The level of significance, $\alpha =0.01$.

The null hypothesis for testing is defined as,

$H_0$: The average daily July temperature follows a normal distribution.

The alternative hypothesis is defined as,

$H_1$: The average daily July temperature does not follow a normal distribution.

To determine

To test: The value of chi-square statistic for the sample, whether all the expected frequencies are greater than 5 and also explain the sampling distribution to be used and find degrees of freedom.

Answer to Problem 9P

Solution: The value of chi-square statistic for the sample is 1.5590. Expected frequencies are greater than 5. We have to use chi-square distribution and degrees of freedom are 5.

Explanation of Solution

Calculation: To find the ${\chi }^2$ statistic for the sample by using MINITAB software is as:

Step 1: Go to Stat > Tables > Chi-square Goodness-of-fit-test.

Step 2: Select ‘Days_20_yrs’ in ‘Observed counts’.

Step 3: Choose ‘Normal_curve’ in ‘Proportions specified by Historical counts’. Then click on OK.

The Minitab output is:

Chi-Square Goodness-of-Fit Test

Category	Observed	Historical Counts	Test Proportion	Expected	Contribution to Chi-Square
1	16	0.0235	0.023571	14.614	0.131481
2	78	0.1350	0.135406	83.952	0.421963
3	212	0.3400	0.341023	211.434	0.001514
4	221	0.3400	0.341023	211.434	0.432771
5	81	0.1350	0.135406	83.952	0.103791
6	12	0.0235	0.023571	14.614	0.467513

Chi-Square Test

N	DF	Chi-Sq	P-Value
620	5	1.55903	0.906

Therefore, the obtained Chi-square test statistics is 1.5590.

The obtained expected frequencies are:

Expected Frequencies

14.614

83.952

211.434

211.434

83.952

14.614

Therefore, all expected frequencies are greater than 5.

The chi-square distribution will use in this study and the degrees of freedom is 5.

Conclusion: ${\chi }^2\text{statistic}\approx 1.559$ and expected frequencies are greater than 5. We have to use chi-square distribution and degrees of freedom are 5.

To determine

The P-value of the sample statistic.

Answer to Problem 9P

Solution: The P-value of sample statistic is 0.906.

Explanation of Solution

Calculation: The Minitab output obtained in above part (b) also gives the P-value. So, the estimate P-valuefor the sample test statistic is 0.906.

To determine

Whether we reject or fail to reject the null hypothesis.

Answer to Problem 9P

Solution: We failed to reject the null hypothesis is at 1% level of significance.

Explanation of Solution

The obtained results in part (a), (b) and (c) are,

$\alpha =0.01$

${\chi }^2\text{statistic}\approx 1.5590$ with5 degrees of freedom.

Since, the P-value(0.906) is less than 0.01, hence, we failed to reject the null hypothesis at $\alpha =0.01$. Therefore we can conclude that the data are not statistically significant at 1% level of significance.

To determine

To explain: The conclusion in the context of application.

Answer to Problem 9P

Solution: We have insufficient evidence to conclude that the average daily July temperature does not follow a normal distribution at 1% level of significance.

Explanation of Solution

From above part, we failed to reject the null hypothesis of independence at $\alpha =0.01$. Therefore, we can conclude that the data are not statistically significant at 1% level of significance.

We have insufficient evidence to conclude that the average daily July temperature does not follow a normal distributionat 1% level of significance.