
Concept explainers
(a)
The interpretation of
Answer to Problem 1P
Solution:
The program is stated as follows.
Explanation of Solution
The given expressions are,
Multiply the above expressions.
Write the MATLAB code to determine the interpretation of
MATLAB Code:
syms x
S1 = x.^2.*(4.*x.^2-8.*x-3)+3.*(8.*x-9)
S2 = (2.*x-3).^2+4.*x
a = simplify(S1.*S2)
Save the MATLAB script with the name, Chapter11_56830_11_1Pa.m in the current folder. Execute the script by typing the script name at the command window to get the code to determine the interpretation of
Result:

Conclusion:
Therefore, the required program is executed above.
(b)
The interpretation of
Answer to Problem 1P
Solution:
The program is stated as follows.
Explanation of Solution
The given expressions are,
Divide the above expressions.
Write the MATLAB code to determine the interpretation of
MATLAB Code:
syms x
S1 = x.^2.*(4.*x.^2-8.*x-3)+3.*(8.*x-9)
S2 = (2.*x-3).^2+4.*x
b = simplify(S1./S2)
Save the MATLAB script with the name, Chapter11_56830_11_1Pb.m in the current folder. Execute the script by typing the script name at the command window to get the code to determine the interpretation of
Result:
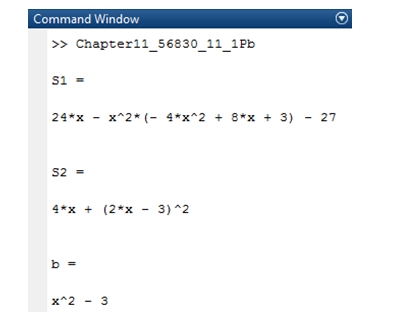
Conclusion:
Therefore, the required program is executed above.
(c)
The interpretation of
Answer to Problem 1P
Solution:
The program is stated as follows.
Explanation of Solution
The given expressions are,
Subtract the above expressions.
Write the MATLAB code to determine the interpretation of
MATLAB Code:
syms x
S1 = x.^2.*(4.*x.^2-8.*x-3)+3.*(8.*x-9)
S2 = (2.*x-3).^2+4.*x
b = simplify(S1-S2)
Save the MATLAB script with the name, Chapter11_56830_11_1Pc.m in the current folder. Execute the script by typing the script name at the command window to get the code to determine the interpretation of
Result:

Conclusion:
Therefore, the required program is executed above.
(d)
The numerical value of the result from part (c) for
Answer to Problem 1P
Solution:
The program is stated as follows.
Explanation of Solution
The given expressions are,
Subtract the above expressions.
Substitute
Write the MATLAB code to determine the numerical value of the result from part (c) for
MATLAB Code:
syms x
S1 = x.^2.*(4.*x.^2-8.*x-3)+3.*(8.*x-9)
S2 = (2.*x-3).^2+4.*x
c = simplify(S1-S2);
d = subs(c,7)
Save the MATLAB script with the name, Chapter11_56830_11_1Pd.m in the current folder. Execute the script by typing the script name at the command window to get the code to determine the numerical value of the result from part (c) for
Result:
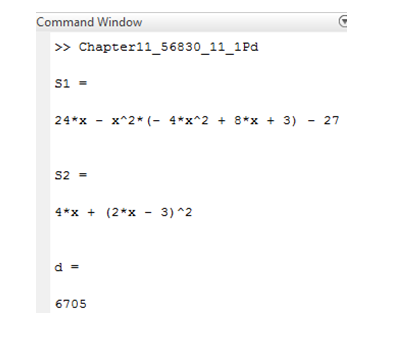
Conclusion:
Therefore, the required program is executed above.
Want to see more full solutions like this?
Chapter 11 Solutions
MATLAB: An Introduction with Applications
- Harvard University California Institute of Technology Massachusetts Institute of Technology Stanford University Princeton University University of Cambridge University of Oxford University of California, Berkeley Imperial College London Yale University University of California, Los Angeles University of Chicago Johns Hopkins University Cornell University ETH Zurich University of Michigan University of Toronto Columbia University University of Pennsylvania Carnegie Mellon University University of Hong Kong University College London University of Washington Duke University Northwestern University University of Tokyo Georgia Institute of Technology Pohang University of Science and Technology University of California, Santa Barbara University of British Columbia University of North Carolina at Chapel Hill University of California, San Diego University of Illinois at Urbana-Champaign National University of Singapore McGill…arrow_forwardName Harvard University California Institute of Technology Massachusetts Institute of Technology Stanford University Princeton University University of Cambridge University of Oxford University of California, Berkeley Imperial College London Yale University University of California, Los Angeles University of Chicago Johns Hopkins University Cornell University ETH Zurich University of Michigan University of Toronto Columbia University University of Pennsylvania Carnegie Mellon University University of Hong Kong University College London University of Washington Duke University Northwestern University University of Tokyo Georgia Institute of Technology Pohang University of Science and Technology University of California, Santa Barbara University of British Columbia University of North Carolina at Chapel Hill University of California, San Diego University of Illinois at Urbana-Champaign National University of Singapore…arrow_forwardA company found that the daily sales revenue of its flagship product follows a normal distribution with a mean of $4500 and a standard deviation of $450. The company defines a "high-sales day" that is, any day with sales exceeding $4800. please provide a step by step on how to get the answers in excel Q: What percentage of days can the company expect to have "high-sales days" or sales greater than $4800? Q: What is the sales revenue threshold for the bottom 10% of days? (please note that 10% refers to the probability/area under bell curve towards the lower tail of bell curve) Provide answers in the yellow cellsarrow_forward
- Find the critical value for a left-tailed test using the F distribution with a 0.025, degrees of freedom in the numerator=12, and degrees of freedom in the denominator = 50. A portion of the table of critical values of the F-distribution is provided. Click the icon to view the partial table of critical values of the F-distribution. What is the critical value? (Round to two decimal places as needed.)arrow_forwardA retail store manager claims that the average daily sales of the store are $1,500. You aim to test whether the actual average daily sales differ significantly from this claimed value. You can provide your answer by inserting a text box and the answer must include: Null hypothesis, Alternative hypothesis, Show answer (output table/summary table), and Conclusion based on the P value. Showing the calculation is a must. If calculation is missing,so please provide a step by step on the answers Numerical answers in the yellow cellsarrow_forwardShow all workarrow_forward
 MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman





