
Concept explainers
BIO Human-Powered Centrifuge
Space travel is fraught with hazards, not the least of which are the many side effects of prolonged weightlessness, including weakened muscles, bone loss, decreased coordination, and unsteady balance. If you are fortunate enough to go on a trip to Mars, which could take more than a year each way, you might be a bit “weak in the knees” by the time you arrive. This could lead to problems when you try to take your first “small step” on the surface.
To counteract these effects, NASA is looking into ways to provide astronauts with “portable gravity” on long space flights One method under consideration is the human-powered centrifuge, which not only subjects the astronauts to artificial gravity, but also gives them aerobic exercise. The device is basically a rotating, circular platform on which two astronauts lie supine along a diameter, head-to-head at the center, with their feet at opposite rims, as shown in the accompanying photo. The radius of the platform in this test model is 6.25 ft. As one astronaut pedals to rotate the platform, the astronaut facing the other direction can exercise in the artificial gravity. Alternatively, a third astronaut on a stationary bicycle can provide the rotation for the other two. While the astronauts’ feet are at the outer rim of the platform, their heads are near the center of the platform, and their hearts are 4.50 feet from the rim, which means that different parts of the astronauts’ bodies will experience different “gravitational” accelerations.

Human-powered centrifuge.
Figure 10-43 shows the centripetal acceleration (in g) produced by a rotating platform at four different radii. Notice that the acceleration increases as the square of the angular speed. Also indicated in Figure 10-43 are acceleration levels corresponding to 1, 3, and 5 gs. It is thought that enhanced gravitational effects may be desirable because the astronauts will experience the artificial gravity for only relatively brief periods of time during the flight.
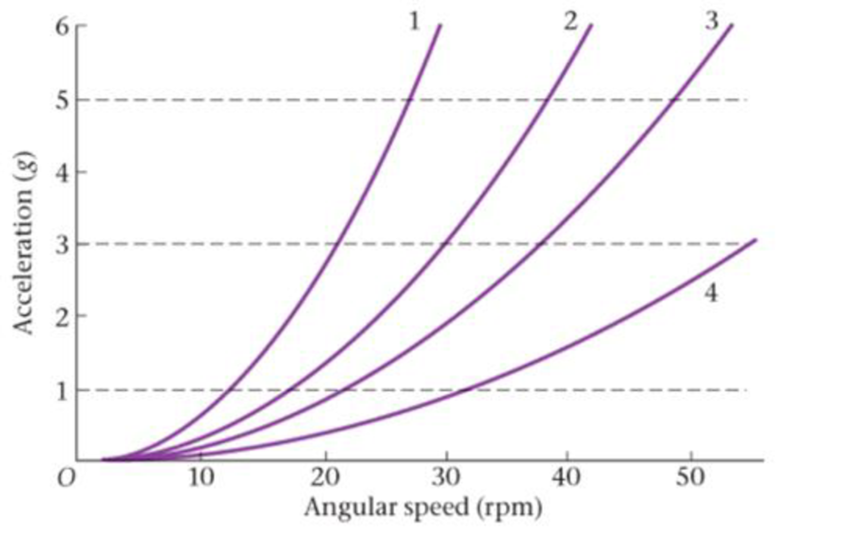
Figure 10-43
Problems 94, 95, 96, and 97
95. • A particular experimental protocol calls for measuring the physiological parameters during exercise with 2.00g at the feet. What angular speed in rpm must the platform in this test model have in order to produce this centripetal acceleration at the feet?
- A. 3.20 rpm
- B. 26.5 rpm
- C. 30.6 rpm
- D. 57.8 rpm
Want to see the full answer?
Check out a sample textbook solution
Chapter 10 Solutions
Physics (5th Edition)
Additional Science Textbook Solutions
Campbell Biology (11th Edition)
Brock Biology of Microorganisms (15th Edition)
Biology: Life on Earth (11th Edition)
Organic Chemistry (8th Edition)
Microbiology: An Introduction
Genetic Analysis: An Integrated Approach (3rd Edition)
- Suppose there are two transformers between your house and the high-voltage transmission line that distributes the power. In addition, assume your house is the only one using electric power. At a substation the primary of a step-down transformer (turns ratio = 1:23) receives the voltage from the high-voltage transmission line. Because of your usage, a current of 51.1 mA exists in the primary of the transformer. The secondary is connected to the primary of another step-down transformer (turns ratio = 1:36) somewhere near your house, perhaps up on a telephone pole. The secondary of this transformer delivers a 240-V emf to your house. How much power is your house using? Remember that the current and voltage given in this problem are rms values.arrow_forwardThe human eye is most sensitive to light having a frequency of about 5.5 × 1014 Hz, which is in the yellow-green region of the electromagnetic spectrum. How many wavelengths of this light can fit across a distance of 2.2 cm?arrow_forwardA one-dimensional harmonic oscillator of mass m and angular frequency w is in a heat bath of temperature T. What is the root mean square of the displacement of the oscillator? (In the expressions below k is the Boltzmann constant.) Select one: ○ (KT/mw²)1/2 ○ (KT/mw²)-1/2 ○ kT/w O (KT/mw²) 1/2In(2)arrow_forward
- Two polarizers are placed on top of each other so that their transmission axes coincide. If unpolarized light falls on the system, the transmitted intensity is lo. What is the transmitted intensity if one of the polarizers is rotated by 30 degrees? Select one: ○ 10/4 ○ 0.866 lo ○ 310/4 01/2 10/2arrow_forwardBefore attempting this problem, review Conceptual Example 7. The intensity of the light that reaches the photocell in the drawing is 160 W/m², when 0 = 18°. What would be the intensity reaching the photocell if the analyzer were removed from the setup, everything else remaining the same? Light Photocell Polarizer Insert Analyzerarrow_forwardThe lifetime of a muon in its rest frame is 2.2 microseconds. What is the lifetime of the muon measured in the laboratory frame, where the muon's kinetic energy is 53 MeV? It is known that the rest energy of the muon is 106 MeV. Select one: O 4.4 microseconds O 6.6 microseconds O 3.3 microseconds O 1.1 microsecondsarrow_forward
- The Lagrangian of a particle performing harmonic oscil- lations is written in the form L = ax² - Bx² - yx, where a, and are constants. What is the angular frequency of oscillations? A) √2/a B) √(+2a)/B C) √√Ba D) B/αarrow_forwardThe mean temperature of the Earth is T=287 K. What would the new mean temperature T' be if the mean distance between the Earth and the Sun was increased by 2%? Select one: ○ 293 K O 281 K ○ 273 K 284 Karrow_forwardTwo concentric current-carrying wire loops of radius 3 cm and 9 cm lie in the same plane. The currents in the loops flow in the same direction and are equal in magnitude. The magnetic field at the common center of the loops is 50 mT. What would be the value of magnetic field at the center if the direction of the two currents was opposite to each other (but their value is kept constant)? Select one: ○ 20 mT ○ 10 mT O 15 mT ○ 25 mTarrow_forward
- An ideal coil of inductivity 50 mH is connected in series with a resistor of 50 ohm. This system is connected to a 4.5 V battery for a long time. What is the current in the circuit? Select one: O 45 mA ○ 90 mA 00 mA O 150 mAarrow_forwardThere are two thin-walled spherical shells made from the same material, the radius of the smaller shell is half of the radius of the larger one. The thickness of the walls is the same. Denote the moment of inertia (with respect to the center) of the larger shell by I₁, and that of the smaller one by 12. What is the ratio I₁/12? Select one: ○ 8 O 16 O 4 ○ 32arrow_forwardA swimming pool has dimensions 20.0 m X 20.0 m and a flat bottom. The pool is filled to a depth of 3.00 m with fresh water. By what force does the water push each of the sidewalls? Density of water is 1000 kg/m³. Select one: ○ ~ 900 KN о ~ 2 ~ 1800 kN 600 kN 1500 kNarrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning
Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





