Intermediate Algebra
19th Edition
ISBN: 9780998625720
Author: Lynn Marecek
Publisher: OpenStax College
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 10, Problem 449PT
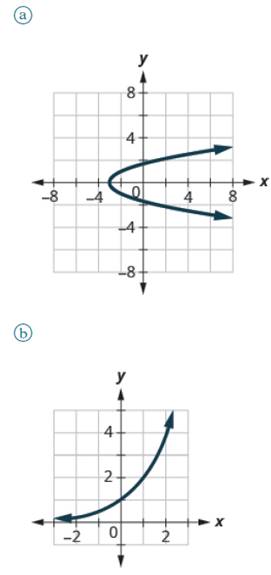
Determine whether each graph is the graph of a function and if so, is it one-to-one.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
EXERCICE 2: 6.5 points
Le plan complexe est rapporté à un repère orthonormé (O, u, v ).Soit [0,[.
1/a. Résoudre dans l'équation (E₁): z2-2z+2 = 0. Ecrire les solutions sous forme exponentielle.
I
b. En déduire les solutions de l'équation (E2): z6-2 z³ + 2 = 0.
1-2
2/ Résoudre dans C l'équation (E): z² - 2z+1+e2i0 = 0. Ecrire les solutions sous forme
exponentielle.
3/ On considère les points A, B et C d'affixes respectives: ZA = 1 + ie 10, zB = 1-ie 10 et zc = 2.
a.
Déterminer l'ensemble EA décrit par le point A lorsque e varie sur
[0, 1.
b. Calculer l'affixe du milieu K du segment [AB].
C.
Déduire l'ensemble EB décrit par le point B lorsque varie sur
[0,¹ [.
d. Montrer que OACB est un parallelogramme.
e.
Donner une mesure de l'angle orienté (OA, OB) puis déterminer pour que OACB soit un
carré.
2
Use grouping to factor: 10x + 13x + 3 = 0
Identify A B and C in the chart below feach responce in
2
Use grouping to factor: 10x² + 13x + 3 = 0
Identify A, B, and C in the chart below. (each re
Chapter 10 Solutions
Intermediate Algebra
Ch. 10.1 - For functions f(x)=3x2 g(x)=5x+1, find (a) (fg)(x)...Ch. 10.1 - For functions f(x)=4x3, and g(x)=6x5, find (a)...Ch. 10.1 - For function f(x)=x29, and g(x)=2x+5, find (a)...Ch. 10.1 - For function f(x)=x2+1, and g(x)=3x5, find (a)...Ch. 10.1 - For each set of ordered pairs, determine if it...Ch. 10.1 - For each set of ordered pairs, determine if it...Ch. 10.1 - Determine (a) whether each graph is the graph of a...Ch. 10.1 - Determine (a) whether each graph is the graph of a...Ch. 10.1 - Find the inverse of {(0,4),(1,7),(2,10),(3,13)}....Ch. 10.1 - Find the inverse of {(1,4),(2,1),(3,0),(4,2)}....
Ch. 10.1 - Graph, on the same coordinate system, the inverse...Ch. 10.1 - Graph, on the same coordinate system, the inverse...Ch. 10.1 - Verify the functions are inverse functions....Ch. 10.1 - Verify the functions are inverse functions....Ch. 10.1 - Verify the inverse of the function f(x)=5x3.Ch. 10.1 - Verify the inverse of the function f(x)=8x+5.Ch. 10.1 - Verify the inverse of the function f(x)=3x25.Ch. 10.1 - Verify the inverse of the function f(x)=6x74.Ch. 10.1 - In the following exercises, find (a) (fg)(x), (b)...Ch. 10.1 - In the following exercises, find (a) (fg)(x), (b)...Ch. 10.1 - In the following exercises, find (a) (fg)(x), (b)...Ch. 10.1 - In the following exercises, find (a) (fg)(x), (b)...Ch. 10.1 - In the following exercises, find (a) (fg)(x), (b)...Ch. 10.1 - In the following exercises, find (a) (fg)(x), (b)...Ch. 10.1 - In the following exercises, find (a) (fg)(x), (b)...Ch. 10.1 - In the following exercises, find (a) (fg)(x), (b)...Ch. 10.1 - In the following exercises, find the values...Ch. 10.1 - In the following exercises, find the values...Ch. 10.1 - In the following exercises, find the values...Ch. 10.1 - In the following exercises, find the values...Ch. 10.1 - In the following exercises, determine if the set...Ch. 10.1 - In the following exercises, determine if the set...Ch. 10.1 - In the following exercises, determine if the set...Ch. 10.1 - In the following exercises, determine if the set...Ch. 10.1 - In the following exercises, determine whether each...Ch. 10.1 - In the following exercises, determine whether each...Ch. 10.1 - In the following exercises, determine whether each...Ch. 10.1 - In the following exercises, determine whether each...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, graph on the same...Ch. 10.1 - In the following exercises, graph on the same...Ch. 10.1 - In the following exercises, graph on the same...Ch. 10.1 - In the following exercises, graph on the same...Ch. 10.1 - In the following exercises, determine whether or...Ch. 10.1 - In the following exercises, determine whether or...Ch. 10.1 - In the following exercises, determine whether or...Ch. 10.1 - In the following exercises, determine whether or...Ch. 10.1 - In the following exercises, determine whether or...Ch. 10.1 - In the following exercises, determine whether or...Ch. 10.1 - In the following exercises, determine whether or...Ch. 10.1 - In the following exercises, determine whether or...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - In the following exercises, find the inverse of...Ch. 10.1 - Explain how the graph of the inverse of a function...Ch. 10.1 - Explain how to find the inverse of a function from...Ch. 10.2 - Graph: f(x)=4x.Ch. 10.2 - Graph: g(x)=5x.Ch. 10.2 - Graph: f(x)=(14)x.Ch. 10.2 - Graph: g(x)=(15)x.Ch. 10.2 - On the same coordinate system, graph: f(x)=2x and...Ch. 10.2 - On the same coordinate system, graph: f(x)=3x and...Ch. 10.2 - On the same coordinate system, graph: f(x)=3x and...Ch. 10.2 - On the same coordinate system, graph: f(x)=4x and...Ch. 10.2 - Solve: 33x2=81.Ch. 10.2 - Solve: 7x3=7.Ch. 10.2 - Solve: ex2ex=e2.Ch. 10.2 - Solve: ex2ex=e6.Ch. 10.2 - Angela invested $15,000 in a savings account. If...Ch. 10.2 - Allan invested $10,000 in a mutual fund. If the...Ch. 10.2 - Another researcher at the Center for Disease...Ch. 10.2 - Maria, a biologist is observing the growth pattern...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each function in...Ch. 10.2 - In the following exercises, graph each function in...Ch. 10.2 - In the following exercises, graph each function in...Ch. 10.2 - In the following exercises, graph each function in...Ch. 10.2 - In the following exercises, graph each function in...Ch. 10.2 - In the following exercises, graph each function in...Ch. 10.2 - In the following exercises, graph each function in...Ch. 10.2 - In the following exercises, graph each function in...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, graph each exponential...Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, solve each equation....Ch. 10.2 - In the following exercises, match the graphs to...Ch. 10.2 - In the following exercises, match the graphs to...Ch. 10.2 - In the following exercises, match the graphs to...Ch. 10.2 - In the following exercises, match the graphs to...Ch. 10.2 - In the following exercises, match the graphs to...Ch. 10.2 - In the following exercises, match the graphs to...Ch. 10.2 - In the following exercises, use an exponential...Ch. 10.2 - In the following exercises, use an exponential...Ch. 10.2 - In the following exercises, use an exponential...Ch. 10.2 - In the following exercises, use an exponential...Ch. 10.2 - In the following exercises, use an exponential...Ch. 10.2 - In the following exercises, use an exponential...Ch. 10.2 - In the following exercises, use an exponential...Ch. 10.2 - In the following exercises, use an exponential...Ch. 10.2 - Explain how you can distinguish between...Ch. 10.2 - Compare and contrast the graphs of y=x2 and y=2x.Ch. 10.2 - What happens to an exponential function as the...Ch. 10.3 - Convert to logarithmic form: (a) 32=9 (b) 712=7...Ch. 10.3 - Convert to logarithmic form: (a) 43=64 (b) 413=43...Ch. 10.3 - Convert to exponential form: (a) 3=log464 (b)...Ch. 10.3 - Convert to exponential form: (a) 3=log327 (b)...Ch. 10.3 - Find the value of x: (a) logx64=2 (b) log5x=3 (c)...Ch. 10.3 - Find the value of x: (a) logx81=2 (b) log3x=5 (c)...Ch. 10.3 - Find the exact value logarithm without using a...Ch. 10.3 - Find the exact value logarithm without using a...Ch. 10.3 - Graph: y=log3x.Ch. 10.3 - Graph: y=log5x.Ch. 10.3 - Graph: y=log12x.Ch. 10.3 - Graph: y=log14x.Ch. 10.3 - Solve: (a) loga121=2 (b) lnx=7Ch. 10.3 - Solve: (a) loga64=3 (b) lnx=9Ch. 10.3 - Solve: (a) log2(5x1)=6 (b) lne3x=6Ch. 10.3 - Solve: (a) log3(4x+3)=3 (b) lne4x=4Ch. 10.3 - What is the decibel level of one of the new quiet...Ch. 10.3 - What is the decibel level heavy city traffic with...Ch. 10.3 - In 1906, San Francisco experienced an intense...Ch. 10.3 - In 2014, Chile experienced an intense earthquake...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert form...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, convert each...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the value of x in...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, find the exact value...Ch. 10.3 - In the following exercises, graph each logarithmic...Ch. 10.3 - In the following exercises, graph each logarithmic...Ch. 10.3 - In the following exercises, graph each logarithmic...Ch. 10.3 - In the following exercises, graph each logarithmic...Ch. 10.3 - In the following exercises, graph each logarithmic...Ch. 10.3 - In the following exercises, graph each logarithmic...Ch. 10.3 - In the following exercises, graph each logarithmic...Ch. 10.3 - In the following exercises, graph each logarithmic...Ch. 10.3 - In the following exercises, graph each logarithmic...Ch. 10.3 - In the following exercises, graph each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, solve each logarithmic...Ch. 10.3 - In the following exercises, use a logarithmic...Ch. 10.3 - In the following exercises, use a logarithmic...Ch. 10.3 - In the following exercises, use a logarithmic...Ch. 10.3 - In the following exercises, use a logarithmic...Ch. 10.3 - In the following exercises, use a logarithmic...Ch. 10.3 - In the following exercises, use a logarithmic...Ch. 10.3 - In the following exercises, use a logarithmic...Ch. 10.3 - In the following exercises, use a logarithmic...Ch. 10.3 - In the following exercises, use a logarithmic...Ch. 10.3 - In the following exercises, use a logarithmic...Ch. 10.4 - Evaluate using the properties of logarithms: (a)...Ch. 10.4 - Evaluate using the properties of logarithms: (a)...Ch. 10.4 - Evaluate using the properties of logarithms: (a)...Ch. 10.4 - Evaluate using the properties of logarithms: (a)...Ch. 10.4 - Use the Product Property of Logarithms to write...Ch. 10.4 - Use the Product Property of Logarithms to write...Ch. 10.4 - Use the Quotient Property of Logarithms to write...Ch. 10.4 - Use the Quotient Property of Logarithms to write...Ch. 10.4 - Use the Power property of Logarithms to write each...Ch. 10.4 - Use the Power property of Logarithms to write each...Ch. 10.4 - Use the Properties of Logarithms to expand the...Ch. 10.4 - Use the Properties of Logarithms to expand the...Ch. 10.4 - Use the Properties of Logarithms to expand the...Ch. 10.4 - Use the Properties of Logarithms to expand the...Ch. 10.4 - Use the Properties of Logarithms to condense the...Ch. 10.4 - Use the Properties of Logarithms to condense the...Ch. 10.4 - Use the Properties of Logarithms to condense the...Ch. 10.4 - Use the Properties of Logarithms to condense the...Ch. 10.4 - Rounding to three decimal places, approximate...Ch. 10.4 - Rounding to three decimal places, approximate...Ch. 10.4 - In the following exercises, use the properties of...Ch. 10.4 - In the following exercises, use the properties of...Ch. 10.4 - In the following exercises, use the properties of...Ch. 10.4 - In the following exercises, use the properties of...Ch. 10.4 - In the following exercises, use the properties of...Ch. 10.4 - In the following exercises, use the properties of...Ch. 10.4 - In the following exercises, use the properties of...Ch. 10.4 - In the following exercises, use the properties of...Ch. 10.4 - In the following exercises, use the properties of...Ch. 10.4 - In the following exercises, use the properties of...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Quotient...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Properties of...Ch. 10.4 - In the following exercises, use the Change-of-Base...Ch. 10.4 - In the following exercises, use the Change-of-Base...Ch. 10.4 - In the following exercises, use the Change-of-Base...Ch. 10.4 - In the following exercises, use the Change-of-Base...Ch. 10.4 - In the following exercises, use the Change-of-Base...Ch. 10.4 - In the following exercises, use the Change-of-Base...Ch. 10.4 - Write the Product Property in your own words. Does...Ch. 10.4 - Write the Power Property in your own words. Does...Ch. 10.4 - Use an example to show that log(a+b)loga+logb ?Ch. 10.4 - Explain how to find the value of log715 using your...Ch. 10.5 - Solve: 2log3x=log336Ch. 10.5 - Solve: 3logx=log64Ch. 10.5 - Solve: log2x+log2(x2)=3Ch. 10.5 - Solve: log2x+log2(x6)=4Ch. 10.5 - Solve: log(x+2)log(4x+3)=logx.Ch. 10.5 - Solve: log(x2)log(4x+16)=log1x.Ch. 10.5 - Solve 7x=43 . Find the exact answer and then...Ch. 10.5 - Solve 8x=98. Find the exact answer and then...Ch. 10.5 - Solve 2ex2=18 . Find the exact answer and then...Ch. 10.5 - Solve 5e2x=25. Find the exact answer and then...Ch. 10.5 - Hector invests $10,000 at age 21. He hopes the...Ch. 10.5 - Rachel invests $15,000 at age 25. She hopes the...Ch. 10.5 - Researchers recorded that a certain bacteria...Ch. 10.5 - Researchers recorded that a certain bacteria...Ch. 10.5 - The half-life of magnesium-27 is 9.45 minutes. How...Ch. 10.5 - The half-life of radioactive iodine is 60 days....Ch. 10.5 - In the following exercises, solve for x. 288....Ch. 10.5 - In the following exercises, solve for x. 289....Ch. 10.5 - In the following exercises, solve for x. 290....Ch. 10.5 - In the following exercises, solve for x. 291....Ch. 10.5 - In the following exercises, solve for x. 292....Ch. 10.5 - In the following exercises, solve for x. 293. 3...Ch. 10.5 - In the following exercises, solve for x. 294....Ch. 10.5 - In the following exercises, solve for x. 295....Ch. 10.5 - In the following exercises, solve for x. 296....Ch. 10.5 - In the following exercises, solve for x. 297....Ch. 10.5 - In the following exercises, solve for x. 299....Ch. 10.5 - In the following exercises, solve for x. 299....Ch. 10.5 - In the following exercises, solve for x. 300....Ch. 10.5 - In the following exercises, solve for x. 301....Ch. 10.5 - In the following exercises, solve for x. 302....Ch. 10.5 - In the following exercises, solve for x. 303....Ch. 10.5 - In the following exercises, solve for x. 304....Ch. 10.5 - In the following exercises, solve for x. 305....Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each exponential...Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve each equation....Ch. 10.5 - In the following exercises, solve for x, giving an...Ch. 10.5 - In the following exercises, solve for x, giving an...Ch. 10.5 - In the following exercises, solve for x, giving an...Ch. 10.5 - In the following exercises, solve for x, giving an...Ch. 10.5 - In the following exercises, solve. 344. Sung Lee...Ch. 10.5 - In the following exercises, solve. 345. Alice...Ch. 10.5 - In the following exercises, solve. 346. Coralee...Ch. 10.5 - In the following exercises, solve. 347. Simone...Ch. 10.5 - In the following exercises, solve. 348....Ch. 10.5 - In the following exercises, solve. 349....Ch. 10.5 - In the following exercises, solve. 350. A virus...Ch. 10.5 - In the following exercises, solve. 351. A bacteria...Ch. 10.5 - In the following exercises, solve. 352. Carbon-14...Ch. 10.5 - In the following exercises, solve. Radioactive...Ch. 10.5 - Explain the method you would use to solve these...Ch. 10.5 - What is the difference between the equation for...Ch. 10 - In the following exercises, for each pair of...Ch. 10 - In the following exercises, for each pair of...Ch. 10 - In the following exercises, evaluate the...Ch. 10 - In the following exercises, evaluate the...Ch. 10 - In the following exercises, for each set of...Ch. 10 - In the following exercises, for each set of...Ch. 10 - In the following exercises, for each set of...Ch. 10 - In the following exercises, for each set of...Ch. 10 - In the following exercises, for each set of...Ch. 10 - In the following exercise, find the inverse of the...Ch. 10 - In the following exercise, graph the inverse of...Ch. 10 - In the following exercises, find the inverse of...Ch. 10 - In the following exercises, find the inverse of...Ch. 10 - In the following exercises, find the inverse of...Ch. 10 - In the following exercises, find the inverse of...Ch. 10 - In the following exercises, find the inverse of...Ch. 10 - In the following exercises, find the inverse of...Ch. 10 - In the following exercises, graph each of the...Ch. 10 - In the following exercises, graph each of the...Ch. 10 - In the following exercises, graph each of the...Ch. 10 - In the following exercises, graph each of the...Ch. 10 - In the following exercises, graph each of the...Ch. 10 - In the following exercises, graph each of the...Ch. 10 - In the following exercises, graph each of the...Ch. 10 - In the following exercises, solve each equation....Ch. 10 - In the following exercises, solve each equation....Ch. 10 - In the following exercises, solve each equation....Ch. 10 - In the following exercises, solve each equation....Ch. 10 - In the following exercises, solve each equation....Ch. 10 - In the following exercises, solve each equation....Ch. 10 - In the following exercises, solve. 386. Felix...Ch. 10 - In the following exercises, solve. 387. Sayed...Ch. 10 - In the following exercises, solve. 388. A...Ch. 10 - In the following exercises, solve. 389. In the...Ch. 10 - In the following exercises, convert from...Ch. 10 - In the following exercises, convert from...Ch. 10 - In the following exercises, convert from...Ch. 10 - In the following exercises, convert from...Ch. 10 - In the following exercises, convert each...Ch. 10 - In the following exercises, convert each...Ch. 10 - In the following exercises, convert each...Ch. 10 - In the following exercises, solve for x. 397....Ch. 10 - In the following exercises, solve for x. 398....Ch. 10 - In the following exercises, solve for x. 399....Ch. 10 - In the following exercises, find the exact value...Ch. 10 - In the following exercises, find the exact value...Ch. 10 - In the following exercises, find the exact value...Ch. 10 - In the following exercises, graph each logarithmic...Ch. 10 - In the following exercises, graph each logarithmic...Ch. 10 - In the following exercises, graph each logarithmic...Ch. 10 - In the following exercises, solve each logarithmic...Ch. 10 - In the following exercises, solve each logarithmic...Ch. 10 - In the following exercises, solve each logarithmic...Ch. 10 - In the following exercises, solve each logarithmic...Ch. 10 - In the following exercises, solve each logarithmic...Ch. 10 - What is the decibel level of a train whistle with...Ch. 10 - In the following exercises, use the properties of...Ch. 10 - In the following exercises, use the properties of...Ch. 10 - In the following exercises, use the properties of...Ch. 10 - In the following exercises, use the properties of...Ch. 10 - In the following exercises, use the Quotient...Ch. 10 - In the following exercises, use the Quotient...Ch. 10 - In the following exercises, use the Quotient...Ch. 10 - In the following exercises, use the Quotient...Ch. 10 - In the following exercises, use the Power Property...Ch. 10 - In the following exercises, use the Power Property...Ch. 10 - In the following exercises, use properties of...Ch. 10 - In the following exercises, use properties of...Ch. 10 - In the following exercises, use properties of...Ch. 10 - In the following exercises, use properties of...Ch. 10 - In the following exercises, use the Properties of...Ch. 10 - In the following exercises, use the Properties of...Ch. 10 - In the following exercises, use the Properties of...Ch. 10 - In the following exercises, use the Properties of...Ch. 10 - In the following exercises, rounding to three...Ch. 10 - In the following exercises, rounding to three...Ch. 10 - In the following exercises, solve for x. 432....Ch. 10 - In the following exercises, solve for x. 433....Ch. 10 - In the following exercises, solve for x. 434....Ch. 10 - In the following exercises, solve for x. 435....Ch. 10 - In the following exercises, solve for x. 436....Ch. 10 - In the following exercises, solve each exponential...Ch. 10 - In the following exercises, solve each exponential...Ch. 10 - In the following exercises, solve each exponential...Ch. 10 - In the following exercises, solve each exponential...Ch. 10 - In the following exercises, solve each exponential...Ch. 10 - Jerome invests $18,000 at age 17. He hopes the...Ch. 10 - Elise invests $4500 in an account that compounds...Ch. 10 - Researchers recorded that a certain bacteria...Ch. 10 - Mouse populations can double in 8 months (A=2A0) ....Ch. 10 - The half-life of radioactive iodine is 60 days....Ch. 10 - For the functions, f(x)=6x+1 and g(x)=8x3, find...Ch. 10 - Determine if the following set of ordered pairs...Ch. 10 - Determine whether each graph is the graph of a...Ch. 10 - Graph, on the same coordinate system, the inverse...Ch. 10 - Find the inverse of the function f(x)=x59.Ch. 10 - Graph the function g(x)=2x3.Ch. 10 - Solve the equation 22x4=64.Ch. 10 - Solve the equation ex2e4=e3x.Ch. 10 - Megan invested $21,000 in a savings account. If...Ch. 10 - Convert the equation from exponential to...Ch. 10 - Convert the equation from logarithmic equation to...Ch. 10 - Solve for x: log5x=3Ch. 10 - Evaluate log111.Ch. 10 - Evaluate log4164.Ch. 10 - Graph the function y=log3x.Ch. 10 - Solve for x: log(x239)=1Ch. 10 - What is the decibel level of a small fan with...Ch. 10 - Evaluate each. (a) 6log617 (b) log993Ch. 10 - In the following exercises, use properties of...Ch. 10 - In the following exercises, use properties of...Ch. 10 - In the following exercises, use properties of...Ch. 10 - In the following exercises, use the Properties of...Ch. 10 - In the following exercises, use the Properties of...Ch. 10 - In the following exercises, use the Properties of...Ch. 10 - In the following exercises, use the Properties of...Ch. 10 - In the following exercises, solve each exponential...Ch. 10 - In the following exercises, solve each exponential...Ch. 10 - In the following exercises, solve each exponential...Ch. 10 - In the following exercises, solve each exponential...Ch. 10 - In the following exercises, solve each exponential...
Additional Math Textbook Solutions
Find more solutions based on key concepts
29. Expected Value for Life Insurance There is a 0.9986 probability that a randomly selected 30-year-old male l...
Elementary Statistics (13th Edition)
Version 2 of the Chain Rule Use Version 2 of the Chain Rule to calculate the derivatives of the following funct...
Calculus: Early Transcendentals (2nd Edition)
Interpreting a P-Value In Exercises 3–8, the P-value for a hypothesis test is shown. Use the P-value to decide ...
Elementary Statistics: Picturing the World (7th Edition)
Simplification of 7.6.5.44.3.2 .
Pre-Algebra Student Edition
Surfing College students and surfers Rex Robinson and Sandy Hudson collected data on the self-reported numbers ...
Introductory Statistics
In track, the second lane from the inside of the track is longer than the inside lane. Use this information to ...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- 2 Use grouping to factor: 10x + 13x + 3 = 0 Identify A B and C in the chart below feach responce inarrow_forwardUse grouping to fully factor: x³ + 3x² - 16x - 48 = 0 3 2arrow_forwardName: Tay Jones Level Two Date: Algebra 3 Unit 3: Functions and Equations Practice Assessment Class: #7-OneNote 1. The function f(x) = x² is transformed in the following functions. List the vertex for each function, circle whether the function opens up or down, and why. All three parts must be correct to receive Level 2 points. You can receive points for a, b, and c. a) g(x) = -2(x+5)² Vertex: Opens Up Opens Down Why? ais negative -2 Vertex: b) g(x) = (x + 2)² - 3 c) g(x) = -4(x + 2)² + 2 Opens Up Opens Down Vertex: Opens Up Opens Down Why? 4 Ca is negative) Why? his positive 2. The graph of the function f(x) is shown below. Find the domain, range, and end behavior. Then list the values of x for which the function values are increasing and decreasing. f(x) Domain: End Behavior: As x → ∞o, f(x) -> -6 As x, f(x) -> Range: Where is it Increasing? (002] Where is it Decreasing? (1,00)arrow_forward
- Show what to do on the graph visually please!arrow_forwardThe county's new asphalt paving machine can surface 1 km of highway in 10 h. A much older machine can surface 1 km in 18 h. How long will it take them to surface 21 km of highway if they start at opposite ends and work day and night?arrow_forward3. Write a system of linear equations in slope intercept form that has exactly one solution at the point (3, 4), such that one line has positive slope (but not 1) and the other line has negative slope (but not "1). Also write your system of equations with both equations written in standard form with out any fractions 8- 7 8 5 4 3 -2- + -8-7-6-5-4-3-2-1 1 2 3 -1 2 - ° 4 -5 - -8arrow_forward
- 2. Write a system of linear equations in slope-intercept form has exactly one solution at the point (3, 4), such that both lines have negative slope (but neither one has slope of 1). Also write your system of equations with both equations written in standard form without any fractions. B 0 5 4 3 -2 1 -8-7-6-5-4-3-2 -1 12 3 -1 2 -3 -5 6 -7 -8arrow_forward4. Write a system of linear equations in slope-intercept form that has no solution, such that (3, 4), and (3,8) are solutions to the first equation, and (0, 4) is a solution to the second equation. Also write your system of equations with both equations written in standard form with out any fractions B 0 5 4 3 -2 + -8-7-6-5-4-3-2 -1 |- 1 2 3 -1 2 -3 4 -5 6 -7arrow_forwardShow how you can solve the system of equations by manipulating the algebra tiles while maintaining the balances. On this side of the page, use the addition (elimination) method. Keep track of what you did at each step by writing down the corresponding equivalent equations, as well as what you did to go from one equation to the next. 1. x + 2y = 5 x-2y=1 2. 2x+y=2 x-2y= 6arrow_forward
- e) x24 1) Which of these are equivalent to x³? For each expression that is equivalent to x², prove it by using the definition of exponents. For each that is not equivalent to x³, give an example using a specific value for x that shows that it represents a different number. a) (x5) d) f) 10-2 b) (x²) *|*arrow_forwardNow show how you can solve the system of equations by manipulating the algebra tiles while maintaining the balances, using the substitution method. Keep track of what you did at each step by writing down the corresponding equivalent equations, as well as what you did to go from one equation to the next. Δ 1. x + 2y = 5 x-2y=1 2. 2x + y = 2 x-2y= 6arrow_forward1. Write a system of two linear equations in slope-intercept form that has exactly one solution at the point (3, 4), such that both lines have positive slope (but neither one has slope of 1) Also write your system of equations with both equations written in standard form without any fractions. 8- 7 8 5 4 3 -2- + -8-7-6-5-4-3-2-1 1 2 3 -1 2 - 4 -5 -7 -8arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Graph Theory: Euler Paths and Euler Circuits; Author: Mathispower4u;https://www.youtube.com/watch?v=5M-m62qTR-s;License: Standard YouTube License, CC-BY
WALK,TRIAL,CIRCUIT,PATH,CYCLE IN GRAPH THEORY; Author: DIVVELA SRINIVASA RAO;https://www.youtube.com/watch?v=iYVltZtnAik;License: Standard YouTube License, CC-BY