Refrigeration and Air Conditioning Technology (MindTap Course List)
8th Edition
ISBN: 9781305578296
Author: John Tomczyk, Eugene Silberstein, Bill Whitman, Bill Johnson
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 10, Problem 1RQ
How can liquid refrigerant be added to the refrigeration system when the system is out of refrigerant?
Expert Solution & Answer
To determine
When the system is out of refrigerant the procedure for adding liquid refrigerant in it.
Explanation of Solution
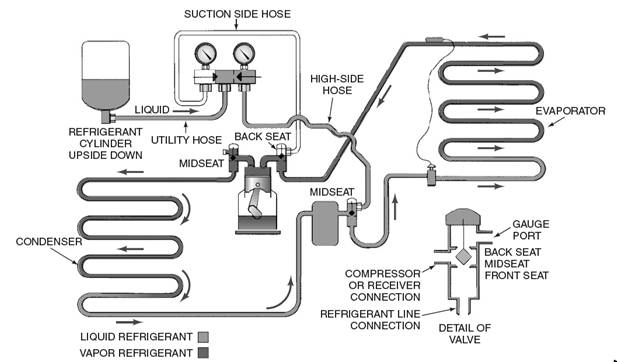
In the refrigeration system, charging a system refers to adding a refrigerant into the system. The amount and type of the charge added must be correct so that a refrigeration system can operate effectively and efficiently as it was designed to. Some refrigerant such as single-compound refrigerants may be added into the system either in the vapour or liquid states, while blended refrigerants must be charged only in the liquid state. A scale is used to determine the correct amount of refrigerant that is added to a system.
Liquid refrigerant charging of a system is normally passed in the liquid line. Liquid refrigerant can be passed into the king valve on the liquid line or receiver when a system is out of refrigerant.It moves through the evaporator and condenser. If the system is in a vacuum or has been evacuated, liquid refrigerant is allowed to enter the system until the flow has nearly stopped. After entering into the system, the liquid refrigerant will move towards the evaporator and condenser coils. While charging the system with liquid refrigerant, precautions must be taken to enter the refrigerant into the suction line of a compressor as it causes damage to the system.
Want to see more full solutions like this?
Subscribe now to access step-by-step solutions to millions of textbook problems written by subject matter experts!
Students have asked these similar questions
||!
Sign in
MMB241 - Tutorial L9.pd X PDF MMB241 - Tutorial L10.pX DE MMB241 - Tutorial L11.p x PDF Lecture W12 - Work and X
File C:/Users/KHULEKANI/Desktop/mmb241/MMB241%20-%20Tutorial%20L11.pdf
PDE Lecture W11 - Power and X
Draw
Alla | Ask Copilot
++
3
of 3
| D
6. If the 50-kg load A is hoisted by motor M so that the load has a constant velocity of 1.5
m/s, determine the power input to the motor, which operates at an efficiency € = 0.8.
1.5 m/s
2
7. The sports car has a mass of 2.3 Mg, and while it is traveling at 28 m/s the driver causes
it to accelerate at 5m/s². If the drag resistance on the car due to the wind is FD= 0.3v²N,
where v is the velocity in m/s, determine the power supplied to the engine at this instant.
The engine has a running efficiency of P = 0.68.
8. If the jet on the dragster supplies a constant thrust of T-20 kN, determine the power
generated by the jet as a function of time. Neglect drag and rolling resistance, and the loss
of fuel. The dragster has a mass of 1…
Q |
Sign in
PDE Lecture W09.pdf
PDF MMB241 - Tutorial L9.pdi X
PDF MMB241 - Tutorial L10.p X
PDF
MMB241 - Tutorial L11.p X
Lecture W12-Work and X
+
File C:/Users/KHULEKANI/Desktop/mmb241/Lecture%20W12%20-%20Work%20and%20Energy.pdf
||!
Draw
| IA | a | Ask Copilot
Class Work
+
33
of 34 D
Question 1
The engine of a 3500-N car is generating a constant power of 50 hp (horsepower)
while the car is traveling up the slope with a constant speed. If the engine is
operating with an efficiency of € 0.8, determine the speed of the car. Neglect
drag and rolling resistance. Use g 9.81 m/s² and 1 hp = 745.7 W.
10
го
Question 2
A man pushes on a 60-N crate with a force F. The force is always directed downward at an angle of 30°
from the horizontal, as shown in the figure. The magnitude of the force is gradually increased until the crate
begins to slide. Determine the crate's initial acceleration once it starts to move. Assume the coefficient of
static friction is μ = 0.6, the coefficient of kinetic…
state is
Derive an expression for the volume expansivity of a substance whose equation of
RT
P
=
v-b
a
v(v + b)TZ
where a and b are empirical constants.
Chapter 10 Solutions
Refrigeration and Air Conditioning Technology (MindTap Course List)
Ch. 10 - How can liquid refrigerant be added to the...Ch. 10 - How is the refrigerant cylinder pressure kept...Ch. 10 - Why does the refrigerant pressure decrease in a...Ch. 10 - What type of equipment normally has the...Ch. 10 - Why are digital scales preferred over other types...Ch. 10 - How does a graduated cylinder account for the...Ch. 10 - What methods besides weighing and measuring are...Ch. 10 - Two or more refrigerants combined together to...Ch. 10 - Define temperature glide, and give examples of...Ch. 10 - A portion of a refrigerant blend that is...
Ch. 10 - What is the main difference between a zeotropic...Ch. 10 - What is meant by "restricting" liquid refrigerant...Ch. 10 - What causes fractionation in certain refrigerant...Ch. 10 - When should a service technician use the...Ch. 10 - A service technician is charging an...Ch. 10 - A technician takes the following readings on an...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- For a gas whose equation of state is P(v-b)=RT, the specified heat difference Cp-Cv is equal to which of the following (show all work): (a) R (b) R-b (c) R+b (d) 0 (e) R(1+v/b)arrow_forwardof state is Derive an expression for the specific heat difference of a substance whose equation RT P = v-b a v(v + b)TZ where a and b are empirical constants.arrow_forwardTemperature may alternatively be defined as T = ди v Prove that this definition reduces the net entropy change of two constant-volume systems filled with simple compressible substances to zero as the two systems approach thermal equilibrium.arrow_forward
- Using the Maxwell relations, determine a relation for equation of state is (P-a/v²) (v−b) = RT. Os for a gas whose av Tarrow_forward(◉ Homework#8arrow_forwardHomework#8arrow_forwardBox A has a mass of 15 kilograms and is attached to the 20 kilogram Box B using the cord and pulley system shown. The coefficient of kinetic friction between the boxes and surface is 0.2 and the moment of inertia of the pulley is 0.5 kg * m^ 2. After 2 seconds, how far do the boxes move? A бро Barrow_forwardBox A has a mass of 15 kilograms and is attached to the 20 kilogram Box B using the cord and pulley system shown. The coefficient of kinetic friction between the boxes and surface is 0.2 and the moment of inertia of the pulley is 0.5 kg * m^2. Both boxes are 0.25 m long and 0.25 m high. The cord is attached to the bottom of Box A and the middle of box B. After 2 seconds, how far do the boxes move? A From бро Barrow_forwardHomework#8arrow_forwardSign in PDF Lecture W09.pdf PDF MMB241 - Tutorial L9.pdf File C:/Users/KHULEKANI/Desktop/mmb241/MMB241%20-%20Tutorial%20L9.pdf II! Draw | I│Alla | Ask Copilot + of 4 D Topic: Kinetics of Particles: - Forces in dynamic system, Free body diagram, newton's laws of motion, and equations of motion. TQ1. The 10-kg block is subjected to the forces shown. In each case, determine its velocity when t=2s if v 0 when t=0 500 N F = (201) N 300 N (b) TQ2. The 10-kg block is subjected to the forces shown. In each case, determine its velocity at s-8 m if v = 3 m/s at s=0. Motion occurs to the right. 40 N F = (2.5 s) N 200 N 30 N (b) TQ3. Determine the initial acceleration of the 10-kg smooth collar. The spring has an unstretched length of 1 m. 1 σ Q ☆ Q 6 ا الى ☑arrow_forwardSign in PDF Lecture W09.pdf PDF MMB241 - Tutorial L9.pdf File C:/Users/KHULEKANI/Desktop/mmb241/MMB241%20-%20Tutorial%20L9.pdf II! Draw | I│Alla | Ask Copilot + 4 of 4 | D TQ9. If motor M exerts a force of F (10t 2 + 100) N determine the velocity of the 25-kg crate when t kinetic friction between the crate and the plane are μs The crate is initially at rest. on the cable, where t is in seconds, 4s. The coefficients of static and 0.3 and μk = 0.25, respectively. M 3 TQ10. The spring has a stiffness k = 200 N/m and is unstretched when the 25-kg block is at A. Determine the acceleration of the block when s = 0.4 m. The contact surface between the block and the plane is smooth. 0.3 m F= 100 N F= 100 N k = 200 N/m σ Q Q ☆ ا الى 6 ☑arrow_forwardmy ID# is 016948724 please solve this problem step by steparrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
Refrigeration and Air Conditioning Technology (Mi...Mechanical EngineeringISBN:9781305578296Author:John Tomczyk, Eugene Silberstein, Bill Whitman, Bill JohnsonPublisher:Cengage Learning
Automotive Technology: A Systems Approach (MindTa...Mechanical EngineeringISBN:9781133612315Author:Jack Erjavec, Rob ThompsonPublisher:Cengage Learning
Refrigeration and Air Conditioning Technology (Mi...Mechanical EngineeringISBN:9781305578296Author:John Tomczyk, Eugene Silberstein, Bill Whitman, Bill JohnsonPublisher:Cengage LearningAutomotive Technology: A Systems Approach (MindTa...Mechanical EngineeringISBN:9781133612315Author:Jack Erjavec, Rob ThompsonPublisher:Cengage Learning
The Refrigeration Cycle Explained - The Four Major Components; Author: HVAC Know It All;https://www.youtube.com/watch?v=zfciSvOZDUY;License: Standard YouTube License, CC-BY