Homework Help is Here – Start Your Trial Now!
Math
Statistics
You want to obtain a sample to estimate a population proportion. Based on previo believe the population proportion is approximately p' = 36 % . You would like to confident that your esimate is within 1.5% of the true population proportion. How l size is required? n = Do not round mid-calculation. However, use a critical value accurate to three decimal Question Help: Message instructor

You want to obtain a sample to estimate a population proportion. Based on previo believe the population proportion is approximately p' = 36 % . You would like to confident that your esimate is within 1.5% of the true population proportion. How l size is required? n = Do not round mid-calculation. However, use a critical value accurate to three decimal Question Help: Message instructor

icon
Related questions
Topic Video
Question
You want to obtain a sample to estimate a population proportion. Based on previous evidence, you believe the population proportion is approximately \( p^* = 36\% \). You would like to be 99.9% confident that your estimate is within 1.5% of the true population proportion. How large of a sample size is required? \[ n = \] Do not round mid-calculation. However, use a critical value accurate to three decimal places. Question Help: Message instructor Submit Question
Transcribed Image Text:You want to obtain a sample to estimate a population proportion. Based on previous evidence, you believe the population proportion is approximately \( p^* = 36\% \). You would like to be 99.9% confident that your estimate is within 1.5% of the true population proportion. How large of a sample size is required? \[ n = \] Do not round mid-calculation. However, use a critical value accurate to three decimal places. Question Help: Message instructor Submit Question
Expert Solution
Step 1

Let p* denote the population proportion.

Given that p*=36%=36/100=0.36

The estimate is within 1.5% of the true population proportion.

That is, E =1.5%=1.5/100=0.015

The confidence interval is given as 99.9%

That is, c=99.9%=99.9/100=0.999

The level of significance is,

α= 1-c=1-0.999=0.001

From standard normal table at α=0.001 level of significance, the two- tailed critical value is,

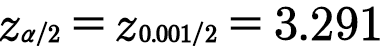
Statistics homework question answer, step 1, image 1

steps

Step by step

Solved in 2 steps with 2 images

Blurred answer
Knowledge Booster
Hypothesis Tests and Confidence Intervals for Proportions
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.